Comparing Fractions Worksheets
All About These 15 Worksheets
This series of 15 worksheets on Comparing Fractions is designed to help students master this essential math topic. Comparing fractions is a fundamental skill in math that is essential for working with fractions in many contexts, including real-life situations.
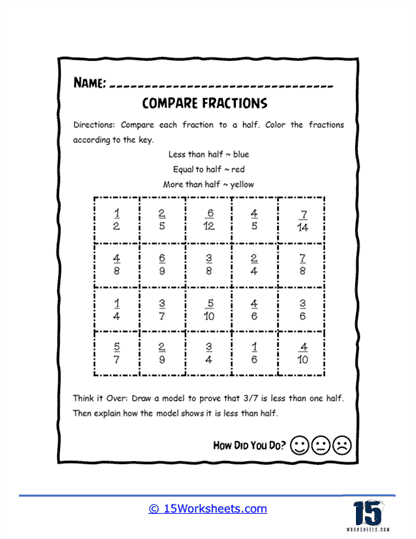
Each worksheet in the series includes a variety of problems with varying levels of difficulty. The problems require students to compare fractions with different denominators and to showcase what they’ve learned about the topic through a wide range of creative exercises.
This series is most suitable for students in grades 4 to 7. It can be used in the classroom as a supplement to the math curriculum or at home as a way for parents to help their children practice and reinforce their math skills.
Overall, these Comparing Fractions worksheets are an engaging and effective tool for helping students develop their skills and confidence in comparing fractions. With these worksheets, students can build a strong foundation in fractions and math, which will serve them well in their future studies.
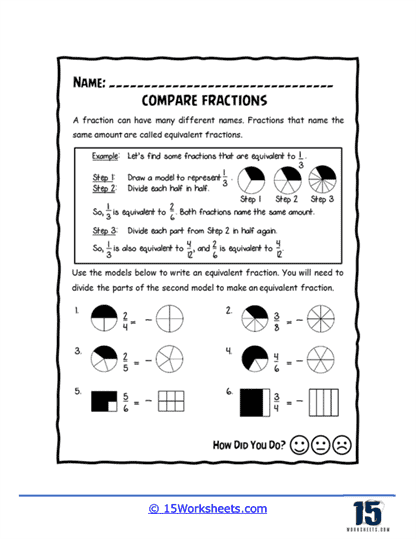
How to Compare the Value of Fractions
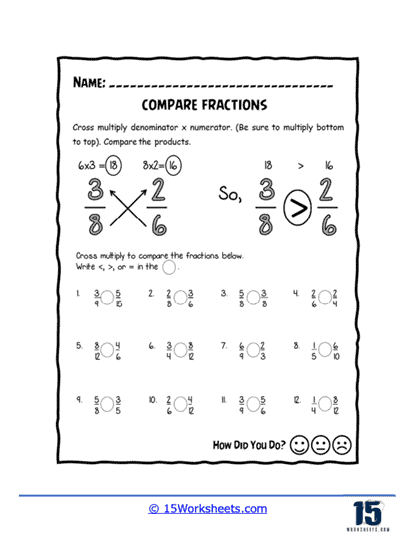
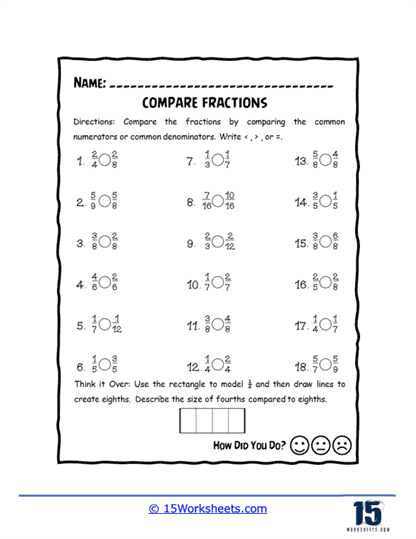
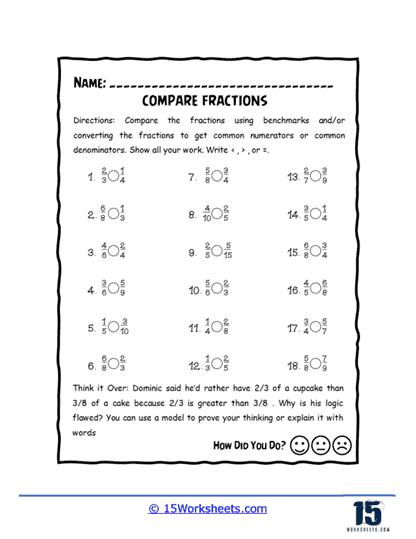
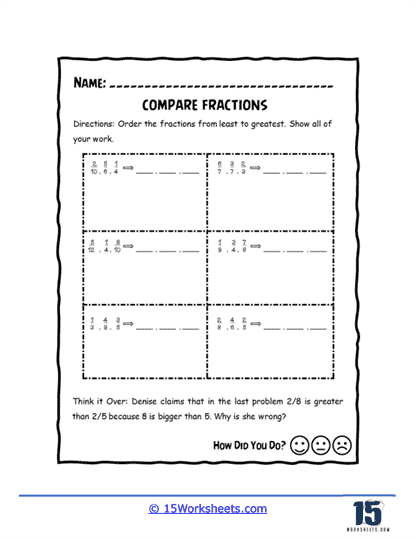
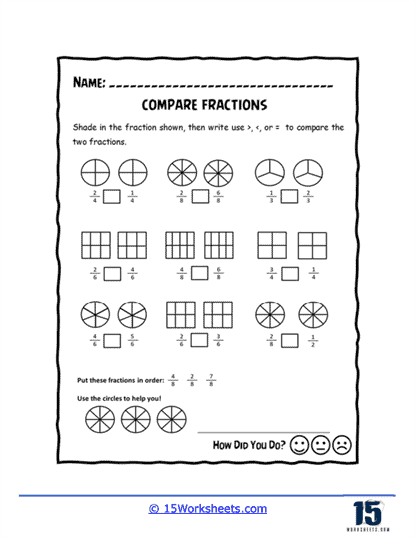
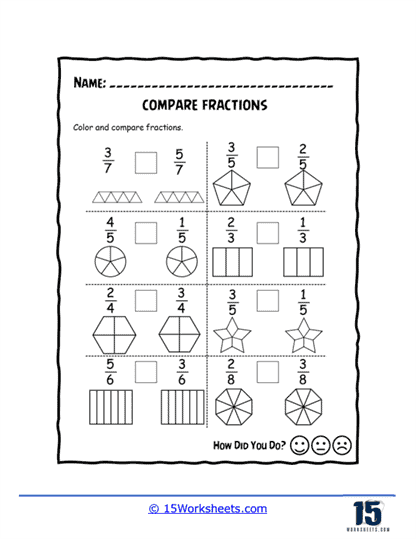
To compare the value of two or more fractions, you need to have a common denominator for each fraction. Once you have found the common denominator, you can compare the numerators of the equivalent fractions to determine which fraction is larger, smaller, or if they are equal. Follow these steps:
Find a common denominator – Use the method described in the previous answers to find a common denominator for the fractions you want to compare.
Convert each fraction to an equivalent fraction with the common denominator – Divide the least common multiple (LCM) by the original denominator, then multiply both the numerator and the denominator of the fraction by the result.
For example, let’s say you want to compare the fractions 2/3 and 3/5:
Find the LCM of 3 and 5 – The LCM is 15.
Convert the fractions to equivalent fractions with the common denominator of 15:
- For 2/3, divide 15 by 3 to get 5. Multiply both the numerator and denominator of 2/3 by 5 to get 10/15.
- For 3/5, divide 15 by 5 to get 3. Multiply both the numerator and denominator of 3/5 by 3 to get 9/15.
Compare the numerators of the equivalent fractions – Now that the fractions have the same denominator, you can compare their numerators to determine their relative values.
In our example, 10/15 and 9/15, the numerators are 10 and 9.
Since 10 > 9, we can conclude that 10/15 > 9/15.
Therefore, the original fractions 2/3 > 3/5.
Remember that you only need to find a common denominator when comparing fractions with different denominators. If the denominators are already the same, you can directly compare the numerators.
Real World Applications
Comparing fractions in the real world can be useful in various situations where we need to evaluate or compare ratios, proportions, or parts of a whole. Some examples include:
- Cooking and Baking – Recipes often involve fractions when measuring ingredients. You may need to compare fractions to adjust the recipe, scale it up or down, or substitute ingredients.
- Shopping – When comparing prices, discounts, or package sizes, you may need to compare fractions to determine the best value for your money.
- Construction and Design – In architecture or interior design, you may need to compare fractions to determine the proportions of materials, the ratio of different design elements, or the relative size of rooms or spaces.
- Time Management – In planning your day or allocating time for tasks, you may compare fractions to decide which tasks to prioritize based on the time they require, or to split your time evenly among different tasks or responsibilities.
- Finance – When comparing investment opportunities, interest rates, or financial returns, you may need to compare fractions to determine the best option for your financial goals.
- Probability and Statistics – In analyzing data or determining the likelihood of an event, you often compare fractions to evaluate the relative probabilities or proportions.
- In School – In teaching or learning mathematics, comparing fractions is a foundational skill that helps students develop a better understanding of ratios, proportions, and number relationships.
- Sports and Fitness – Comparing fractions can be useful in evaluating performance metrics, such as scoring percentages, completion rates, or success ratios, to inform training, strategy, or improvement plans.
These are just a few examples of how comparing fractions can be applied in real-world situations. The ability to compare fractions is a valuable skill that helps us make informed decisions and better understand the world around us.