Operations with Unlike Fractions Worksheets
About These 15 Worksheets
The collection of worksheets focuses on various operations with fractions that have unlike denominators. Here’s a detailed description of the skill sets that these worksheets aim to develop for students:
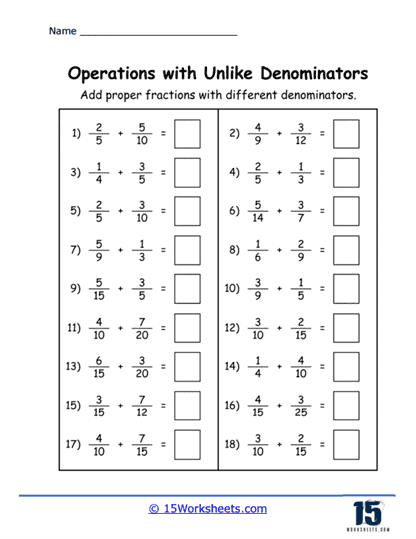
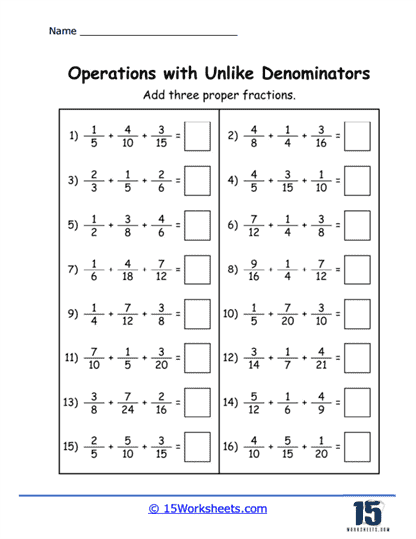
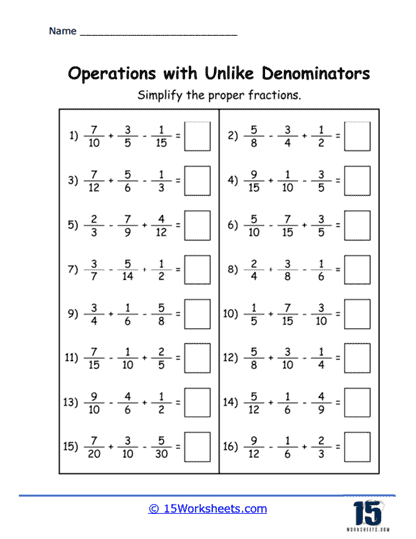
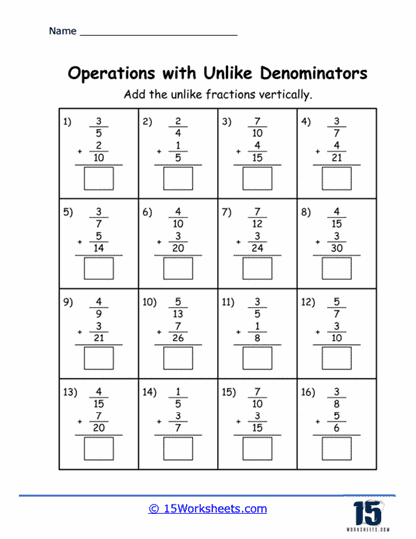
1. Addition of Fractions with Unlike Denominators
Several of the worksheets focus on teaching students how to add proper fractions with different denominators. The worksheets begin with simple problems where two fractions are added together, progressing in complexity. Some worksheets involve three fractions, and others require students to work vertically to solve these problems. This helps students practice the steps of finding a common denominator, converting fractions, and simplifying the results.
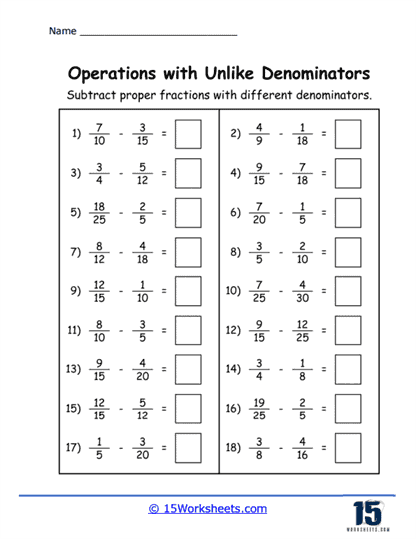
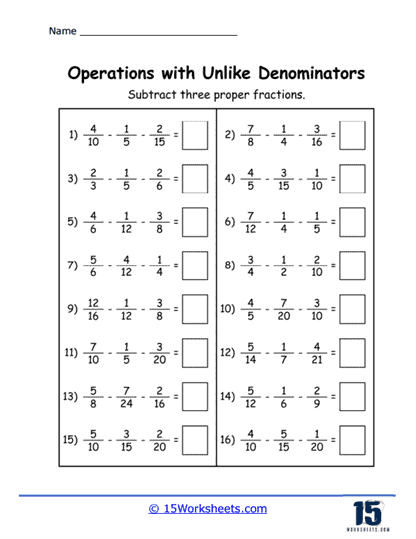
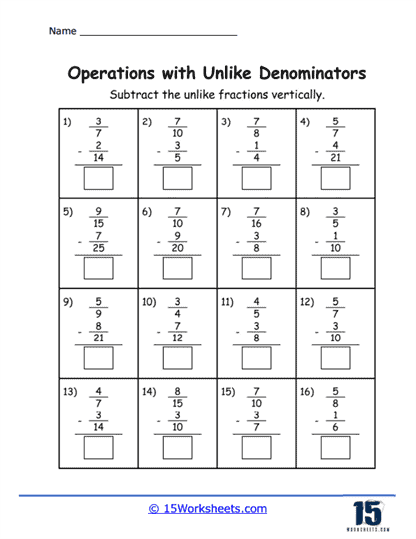
2. Subtraction of Fractions with Unlike Denominators
In addition to adding fractions, these worksheets also focus heavily on subtracting fractions with different denominators. Similar to the addition problems, students are asked to work through multiple levels of complexity-from subtracting two fractions to working with three fractions. By providing both horizontal and vertical formats, students get experience with different problem layouts, helping to reinforce their understanding of the subtraction process across unlike denominators.
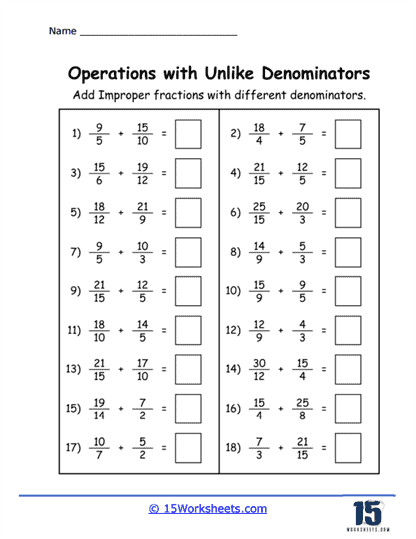
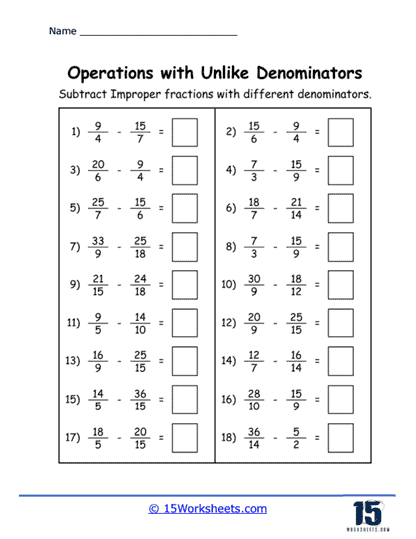
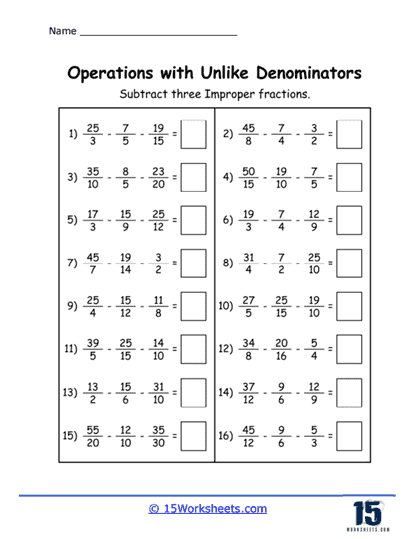
3. Operations with Improper Fractions
A more advanced set of worksheets deals with improper fractions. These problems challenge students to add or subtract improper fractions with unlike denominators. This not only reinforces their previous knowledge of operations with unlike denominators but also introduces more challenging numbers, ensuring that students can handle larger or more complex fractions.
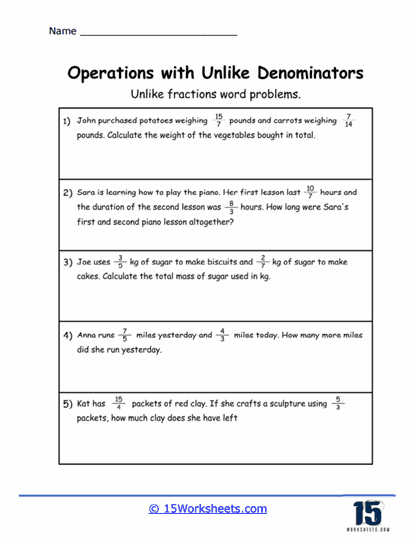
4. Word Problems Involving Fractions
One of the worksheets offers word problems that require the use of fractions. These problems push students to apply their knowledge of fractions to real-life scenarios, such as calculating weights or distances, which involve both proper and improper fractions. This builds critical thinking and problem-solving skills, as students must figure out how to set up and solve fraction equations based on the given context.
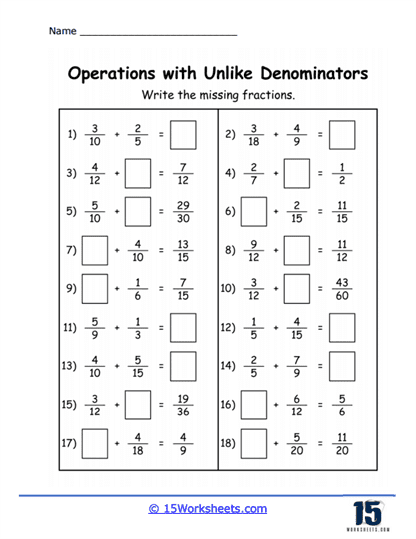
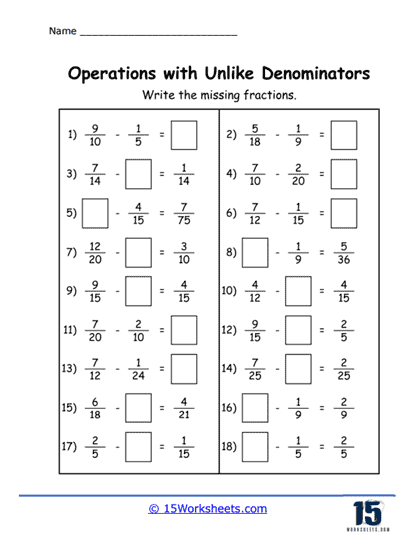
5. Finding Missing Fractions
Another set of worksheets focuses on finding missing fractions in equations. This challenges students to think about the relationship between fractions and their operations, rather than just performing a given calculation. Students are expected to solve for missing numerators or denominators, enhancing their understanding of fraction equivalency and arithmetic with fractions.
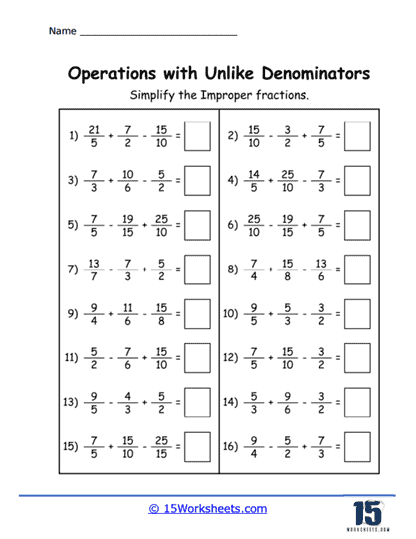
6. Simplifying Improper Fractions
Some worksheets are specifically designed for simplifying improper fractions after completing operations. This skill helps students practice reducing fractions to their simplest form, reinforcing the importance of both accuracy and efficiency in their work.
The collection of worksheets helps students build foundational skills in working with fractions, particularly those with unlike denominators. It begins by focusing on finding common denominators, a crucial step in adding and subtracting fractions. By mastering this skill, students gain a deeper understanding of number relationships and equivalencies, which are essential for handling more advanced mathematical concepts later on. As they progress, they apply this knowledge to perform operations with both proper and improper fractions, ensuring they understand how to manipulate and simplify fractions accurately.
A key strength of these worksheets is how they incorporate real-life applications through word problems. This allows students to move beyond abstract calculations, understanding how fractions can be used in everyday situations, such as measuring time or weight. These practical scenarios build problem-solving skills and encourage students to think critically about how fractions are used outside the classroom. In addition, solving equations involving fractions helps students develop algebraic thinking by focusing on the relationships between different fractions.
The gradual increase in difficulty ensures that students build confidence as they progress, starting with basic operations and working toward more complex challenges, such as multi-step word problems or simplifying improper fractions. This structured approach not only reinforces students’ procedural fluency but also prepares them for future topics in algebra and advanced math, making their learning comprehensive and adaptable to various contexts. Through consistent practice, students gain the skills needed to tackle fractions with ease and apply them in a wide range of mathematical problems.
The worksheets gradually increase in difficulty, allowing students to build their skills progressively. By the end, students should be confident in their ability to perform operations with fractions that have unlike denominators and simplify their answers appropriately. The inclusion of vertical problem-solving, three-term fractions, and word problems makes the collection comprehensive, engaging different levels of critical thinking and arithmetic operations.
How Do You Perform Operations with Unlike Fractions
Operations with unlike fractions primarily require attention to the denominators. Ensuring they are the same (or finding a common denominator) is crucial for addition and subtraction. Once that’s achieved, the operations are straightforward. On the other hand, multiplication and division do not require a common denominator but do follow their unique set of rules. Always remember to simplify the resulting fraction, if possible, to ensure your answer is in its most reduced form.
Step #1 – Finding the Least Common Denominator (LCD)
When the denominators of the fractions are not the same (i.e., they are “unlike” or “different”), the first step is to find the least common denominator (LCD). The LCD is the smallest number that both denominators can divide into evenly. It’s essentially the least common multiple (LCM) of the two denominators.
Step #2 – Rewriting the Fractions with the LCD
Once you find the LCD, you can rewrite each fraction so that they both have this common denominator.
Example: To add 1/3 and 2/5 or 1/3 + 2/5.
Step #1 – Find the LCD: The LCM of 3 and 5 is 15. So, the LCD is 15.
Step #2 – Rewrite each fraction with the LCD
For 1/3: Multiply the numerator and the denominator by 5.
1/3 x 5/5 = 5/15
For 2/5: Multiply the numerator and the denominator by 3.
2/5 c 3/3 = 6/15
The two fractions become 5/15 and 6/15, respectively.