Subtracting Mixed Numbers Worksheets
About These 15 Worksheets
A mixed number combines a whole number and a proper fraction (a fraction where the numerator is smaller than the denominator). For instance, 3 1/2, 5 3/4, and 2 2/3 are all examples of mixed numbers. In 3 1/2, 3 is the whole number, and 1/2 is the fraction.
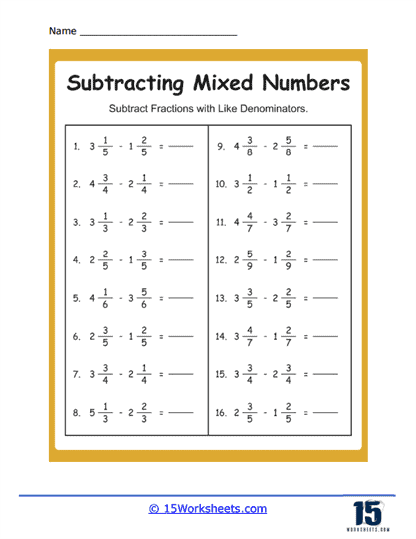
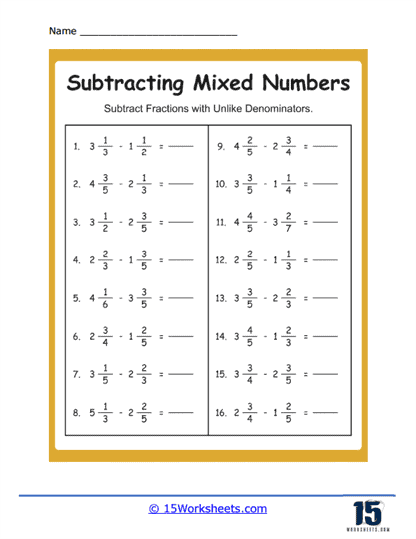
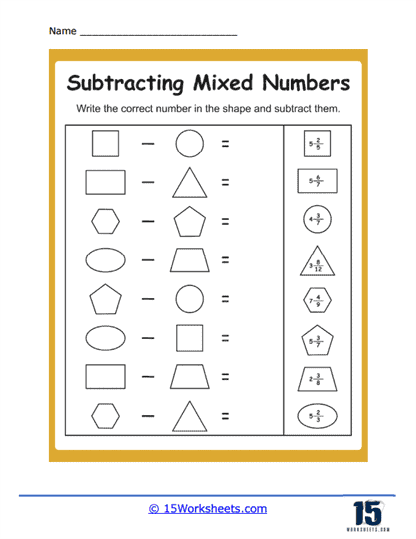
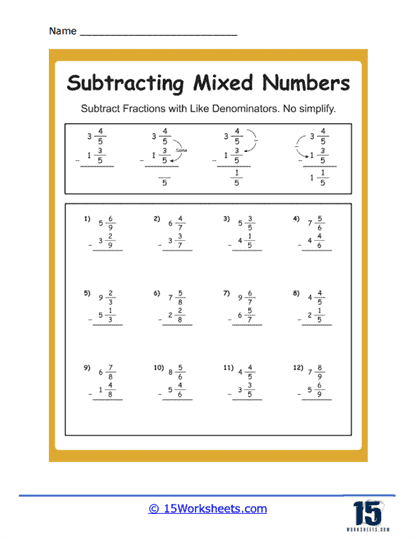
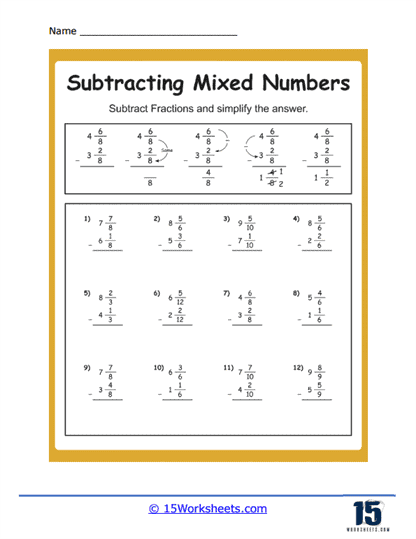
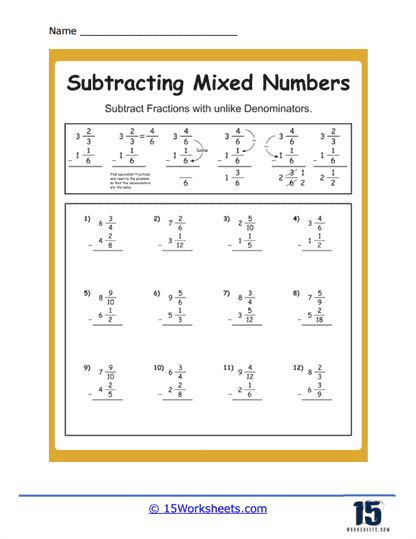
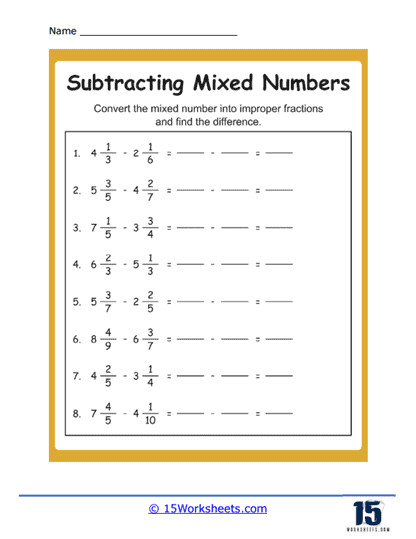
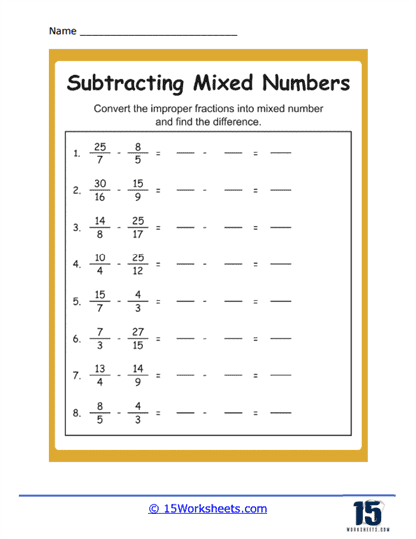
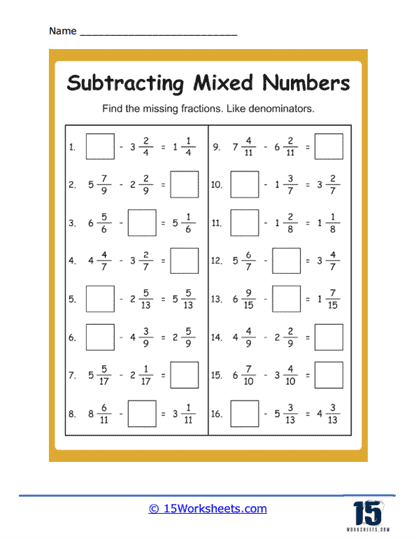
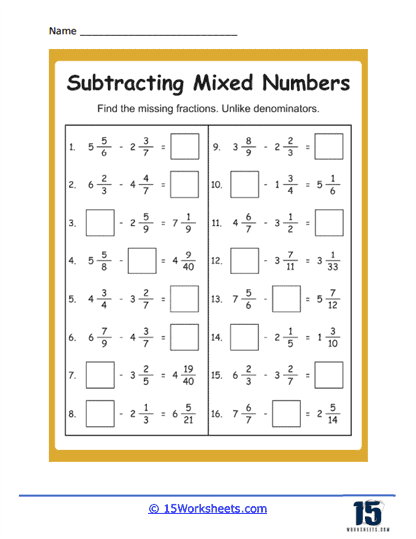
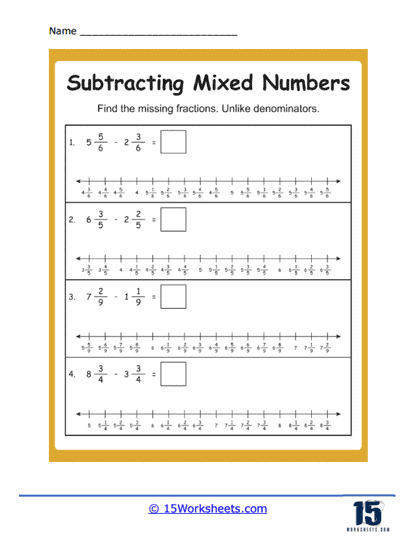
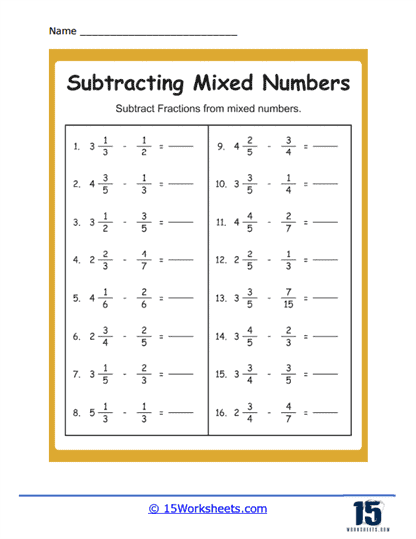
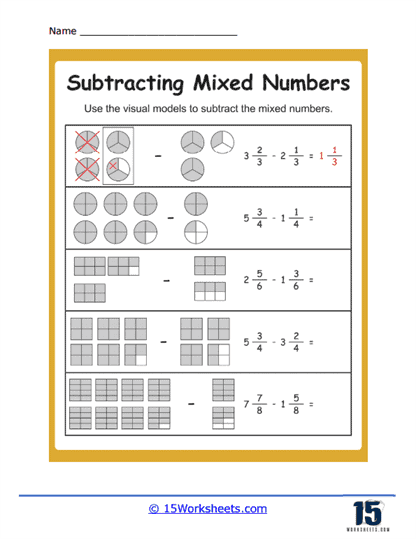
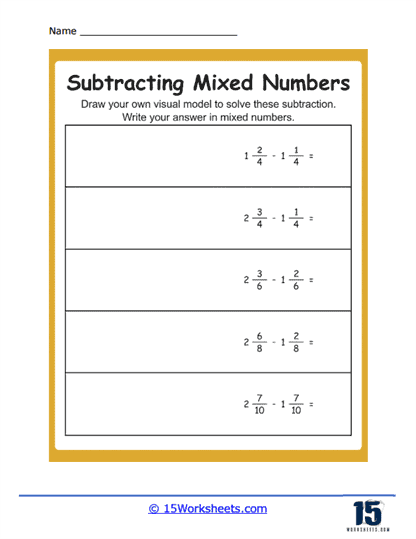
These worksheets are sheets filled with exercises that provide practice in subtracting mixed numbers. Here are some examples of exercises you might see on these worksheets:
Straightforward Subtraction – This type of exercise involves direct subtraction of two mixed numbers. For example, “Subtract 3 2/4 from 5 3/4.”
Subtraction with Borrowing – These exercises are slightly more complex because they require borrowing from the whole number. For example, “Subtract 4 3/4 from 5 1/2.” Here, you can’t subtract 3/4 from 1/2 directly, so you need to borrow 1 from the whole number 5.
Subtraction with Conversion – In this type, you need to convert the answer to the simplest form or convert an improper fraction (where the numerator is larger than the denominator) to a mixed number. For example, “Subtract 2 3/8 from 5 1/2 and simplify the result.”
By practicing these different exercises, you’re not just learning to subtract mixed numbers but also enhancing various math skills:
Problem Solving – You’re applying math concepts to solve problems, and sometimes you need to think creatively, like when you borrow during subtraction.
Number Sense – Working with mixed numbers improves your understanding of how whole numbers and fractions can interact.
Mental Math – As you become more comfortable with subtracting mixed numbers, you’ll find that you can do some calculations in your head.
Conceptual Understanding – By converting between mixed numbers and improper fractions, you’re learning about different ways to represent the same quantity.
Subtracting Mixed Numbers Worksheets can provide a foundation for more advanced math topics. When you get to algebra, you’ll see similar operations but with variables instead of numbers. If you’re comfortable with mixed numbers, you’ll find it easier to understand algebraic expressions.
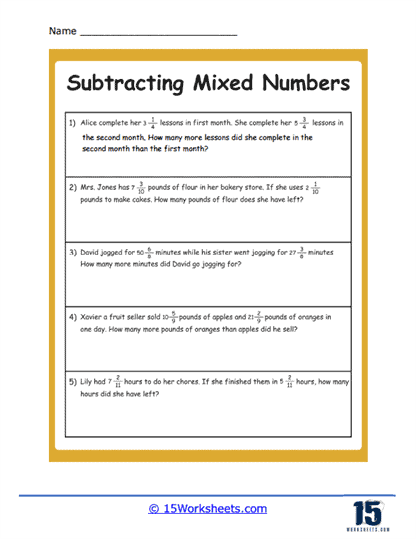
Plus, subtracting mixed numbers is a useful skill in real life. Let’s look at a few examples:
Cooking and Baking – Suppose you’re making cookies, and the recipe calls for 2 1/2 cups of sugar. But you only have 1 3/4 cups. How much more do you need? By subtracting these mixed numbers, you can find out!
Time Management – Imagine you spend 3 1/2 hours on homework and 2 1/4 hours on hobbies. How much more time do you spend on homework? Again, it’s subtraction of mixed numbers.
Sports – If you’re a runner and you run 5 1/2 miles one day and 4 3/4 miles the next day, figuring out the difference requires subtracting mixed numbers.
How Do You Subtract Mixed Numbers?
Subtracting mixed numbers can seem complex at first, but with a few steps, it can be done quite easily. Here’s how you do it:
Step 1) Subtract the fractions – Start by subtracting the fraction part of the mixed numbers.
Step 2) Subtract the whole numbers – Next, subtract the whole numbers.
Step 3) If needed, borrow from the whole number – If the fraction of the number you are subtracting from is smaller than the fraction you are subtracting, you need to “borrow” 1 from its whole number part.
Let’s illustrate this with two examples:
Example 1 – Subtract 1 3/4 from 4 1/2
First, you’ll see that you can’t subtract 3/4 from 1/2 because 3/4 is larger. So you need to borrow 1 from the whole number 4.
This borrowed 1 is equivalent to 2/2 (since 2/2 is equal to 1). So, when you add this to the existing 1/2, you get 2/2 + 1/2 = 3/2.
Now, subtract the fractions – 3/2 – 3/4. To subtract these, you need to make sure the denominators are the same. Multiply the numerator and denominator of 3/4 by 2, so the fractions become 3/2 – 6/4. Simplify 6/4 to 1 1/2. So now, you have 3/2 – 1 1/2 = 1/2.
After borrowing, your whole number for 4 1/2 becomes 3. Now, subtract the whole numbers – 3 – 1 = 2.
So, 4 1/2 – 1 3/4 = 2 1/2.
Example 2 – Subtract 2 2/3 from 5 1/2
Here also, you can’t subtract 2/3 from 1/2, so you need to borrow 1 from 5.
This 1 becomes 2/2 and when you add it to 1/2, you get 2/2 + 1/2 = 3/2.
Now, subtract the fractions – 3/2 – 2/3. To subtract, get a common denominator, which is 6 in this case. So the fractions become 9/6 – 4/6 = 5/6.
After borrowing, your whole number for 5 1/2 becomes 4. Now, subtract the whole numbers – 4 – 2 = 2.
So, 5 1/2 – 2 2/3 = 2 5/6.