Adding 3 Fractions Worksheets
About These 15 Worksheets
You can think of these worksheets like a playground for numbers, where fractions get to play and interact with each other. It’s kind of like how you might learn to play a new sport or a game. At first, you might not know all the rules or strategies, but the more you practice, the better you get. The same goes for math and fractions.
To understand what fractions are, imagine you have a whole pizza in front of you. If you divide that pizza into 4 equal parts, each part is referred to as a ‘fraction’ of the whole pizza. So, each part would be ‘1/4’ of the whole pizza. The number on the top (1) is called the ‘numerator’, which tells you how many parts we have. The number on the bottom (4) is called the ‘denominator’, which tells you into how many equal parts the whole is divided.
Now, let’s discuss the types of exercises you’ll find on Adding 3 Fractions Worksheets. They generally fall into these categories:
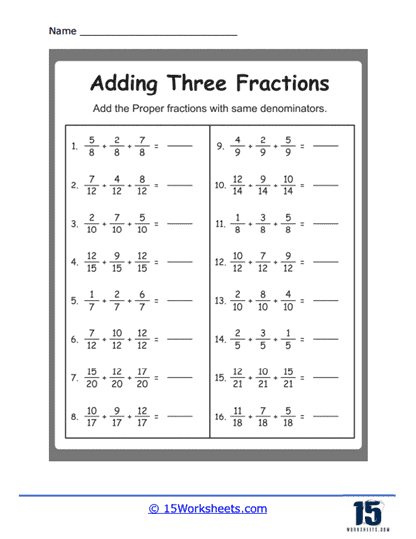
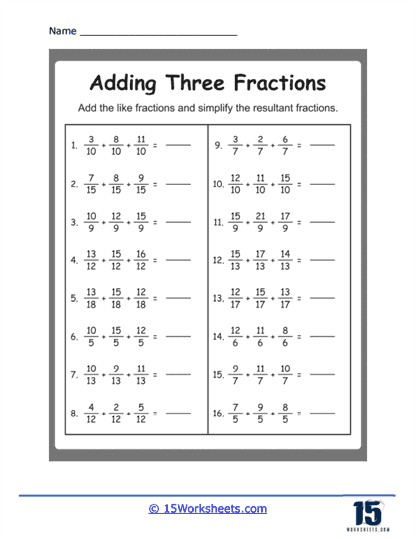
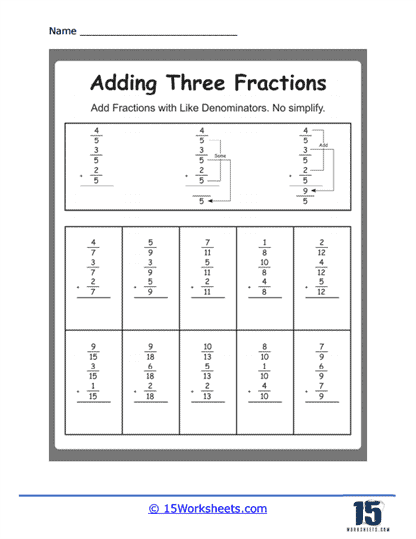
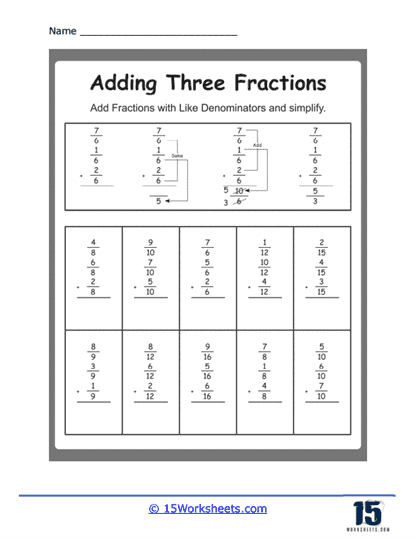
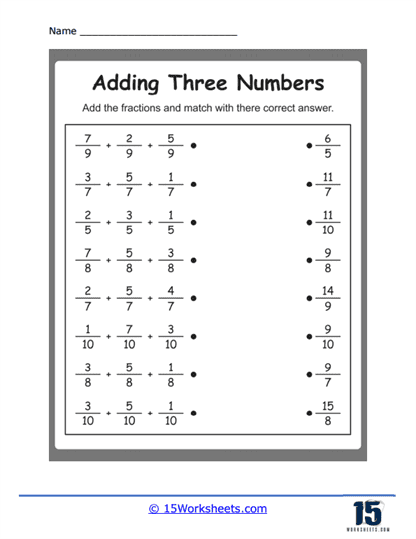
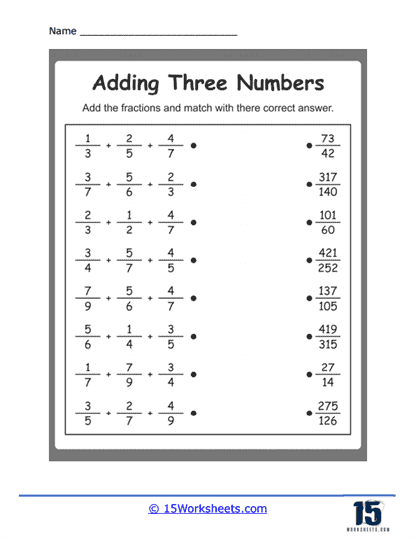
Same Denominator Exercises – These exercises involve fractions that have the same denominator, such as 1/4 + 2/4 + 3/4. These are the easiest exercises because when the denominators are the same, you just add the numerators. In this example, you’d get 6/4 which can be simplified to 1 1/2.
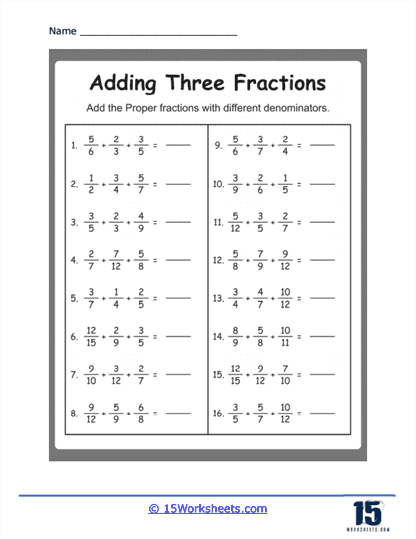
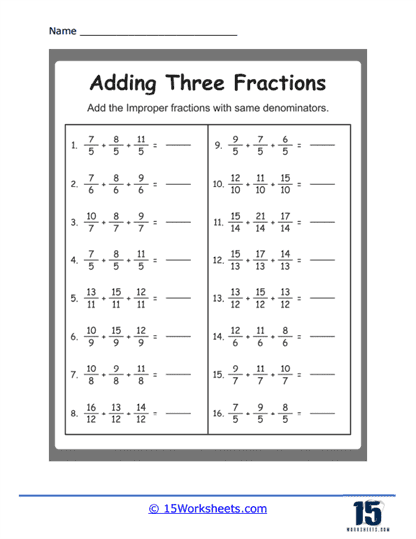
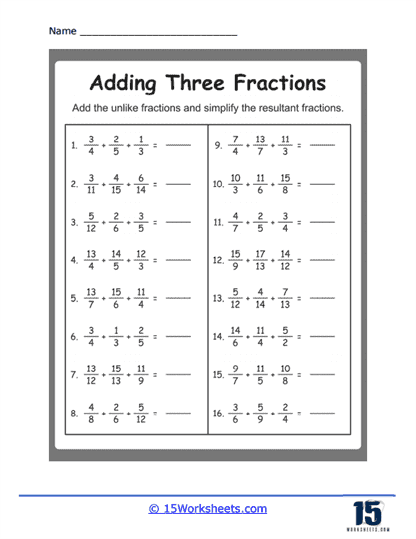
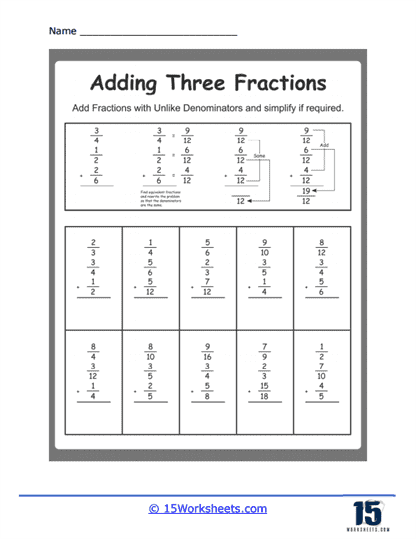
Different Denominator Exercises – These exercises involve fractions that have different denominators, such as 1/3 + 1/4 + 1/6. These are a bit more complex because you have to find a common denominator for all fractions before you can add them. This involves understanding and using multiples.
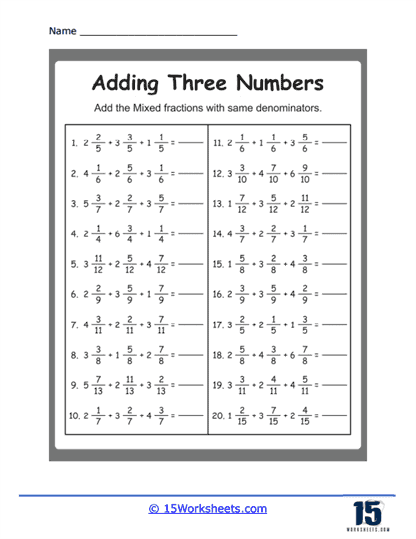
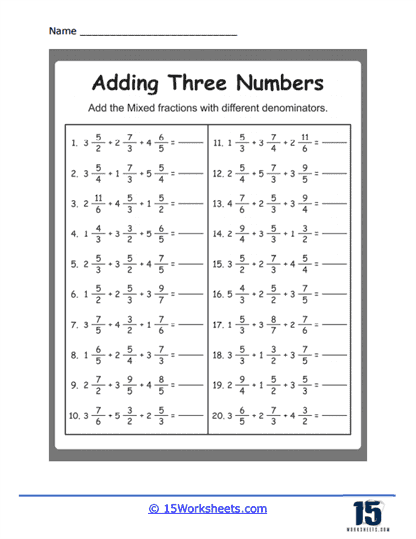
Mixed Number Exercises – These exercises include mixed numbers, which are numbers that have both a whole part and a fraction part, like 1 1/2. An example might be 1 1/3 + 2 1/2 + 3 3/4. These exercises require you to add both the whole numbers and the fractions separately, and then combine them at the end.
Adding 3 Fractions Worksheets can greatly help students improve their fraction skills and prepare them for more advanced math learning in the future. Here’s how:
Practice – The old saying “practice makes perfect” is especially true when it comes to fractions. The more exercises students complete, the more comfortable they will become with fractions. They’ll learn the rules and strategies for adding fractions, and they’ll get faster at it, too.
Understanding – Working with fractions on these worksheets can help students understand what fractions represent, how they relate to each other, and how they can be used in real life. This understanding is important for future math topics like ratios, proportions, and algebra.
Problem-Solving Skills – Many of the exercises on these worksheets require students to think critically and solve problems. They might have to figure out how to find a common denominator or how to simplify a fraction. These problem-solving skills are essential in many areas of math and science.
Building Confidence – As students work through the worksheets and see their progress, they’ll build confidence in their abilities. This confidence can encourage them to tackle more challenging math topics in the future.
Preparation for Advanced Topics – Adding fractions is a fundamental skill in math. It’s used in many advanced topics like algebra, calculus, and even in some areas of science. So, mastering fractions can set students up for success in their future studies.
These worksheets will provide you with a variety of exercises that help you understand and master the concept of fractions, build your problem-solving skills, and prepare you for more advanced math topics. Remember, like any new skill, mastering fractions takes time and practice, so be patient with yourself, keep practicing, and watch your fraction skills grow.
How Do You Add 3 Fractions?
Step 1) Identify the fractions to be added
For our example, let’s use 1/4, 1/3, and 1/2.
Step 2) Find the Least Common Denominator (LCD)
The least common denominator is the smallest multiple that all the denominators can divide into evenly.
In our example, the denominators are 4, 3, and 2. The least common multiple of these three numbers is 12. So, 12 is our least common denominator.
Step 3) Convert Each Fraction to an Equivalent Fraction with the LCD
You do this by multiplying both the numerator and denominator of each fraction by whatever number will make the denominator equal to the least common denominator.
Here’s how we do it for our fractions:
1/4 becomes 3/12 (multiply the numerator and denominator by 3)
1/3 becomes 4/12 (multiply the numerator and denominator by 4)
1/2 becomes 6/12 (multiply the numerator and denominator by 6)
Step 4) Add the Numerators
Once all fractions have the same denominator, you can add the numerators while keeping the denominator constant.
So, 3/12 + 4/12 + 6/12 equals 13/12.
Step 5) Simplify the Fraction if Necessary
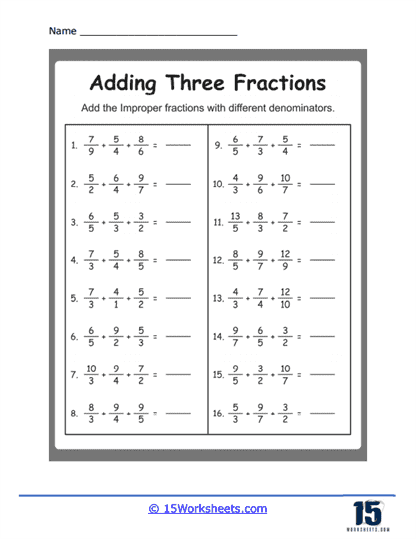
If your result is an improper fraction (the numerator is larger than the denominator), you might want to convert it to a mixed number.
In our example, 13/12 is an improper fraction. It converts to the mixed number 1 1/12 (12 goes into 13 once with 1 left over).
So, 1/4 + 1/3 + 1/2 equals 1 1/12.