Dividing Mixed Numbers Worksheets
About These 15 Worksheets
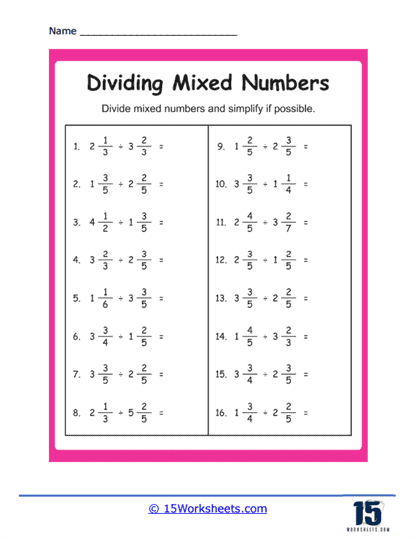
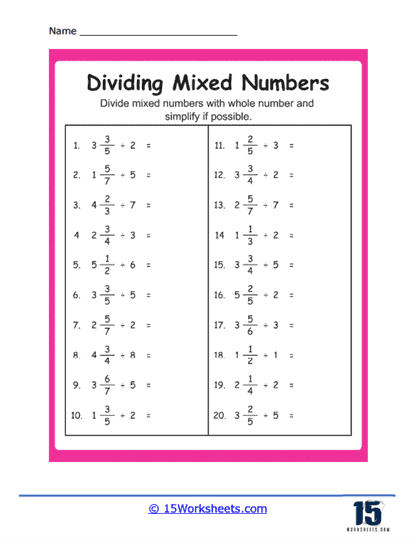
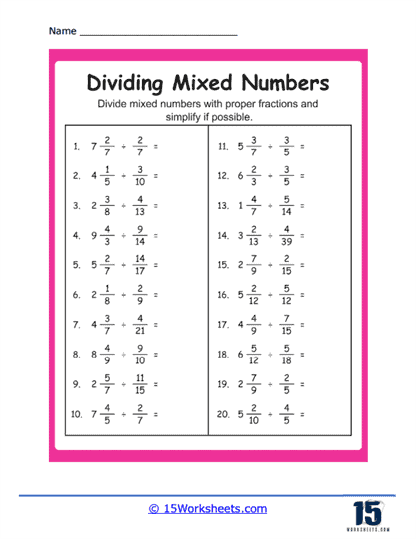
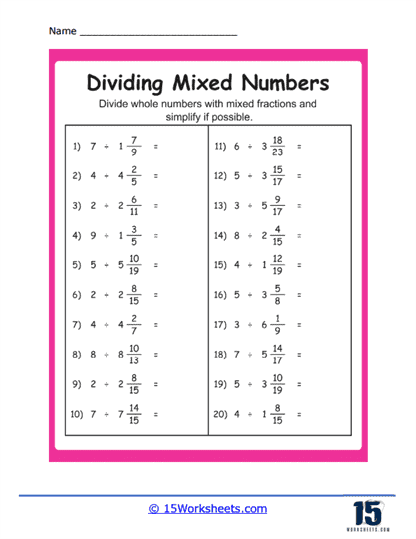
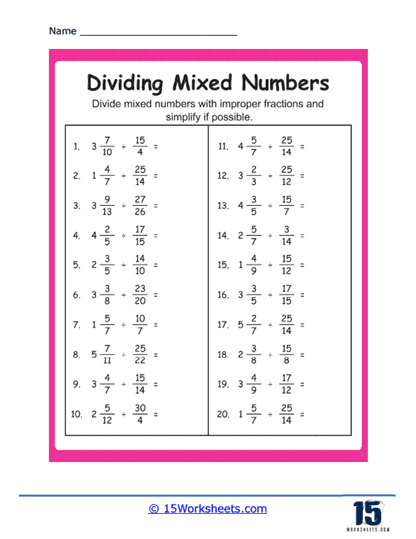
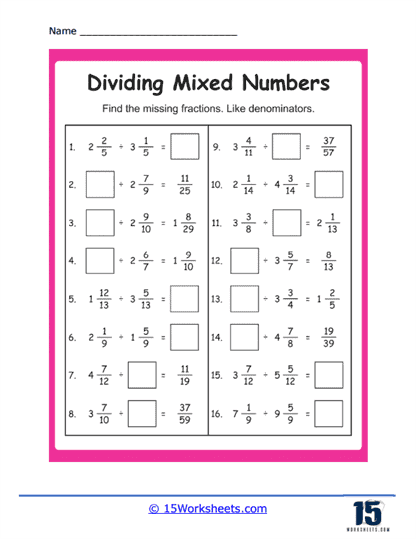
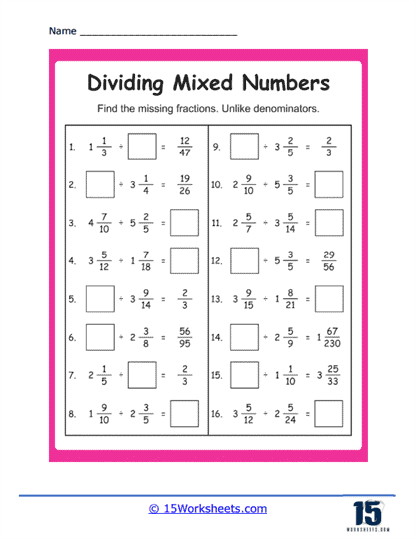
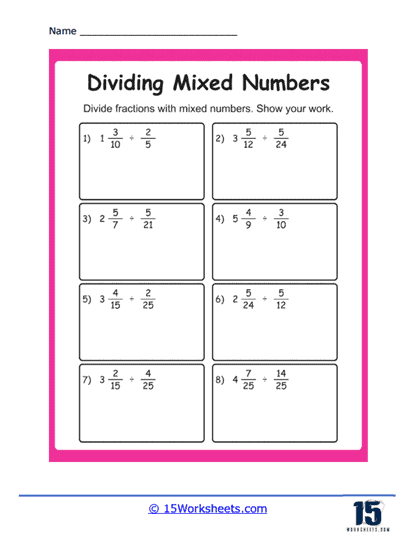
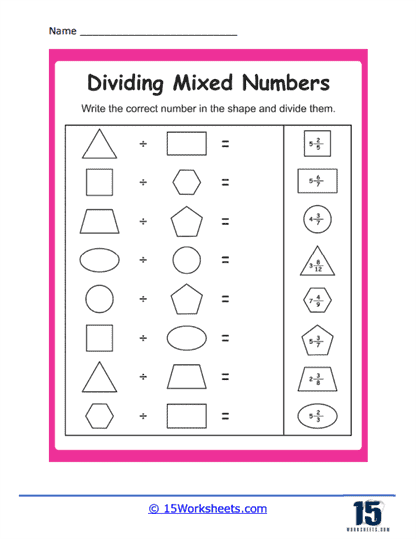
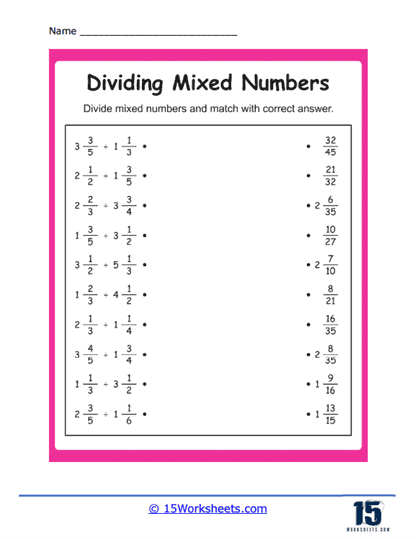
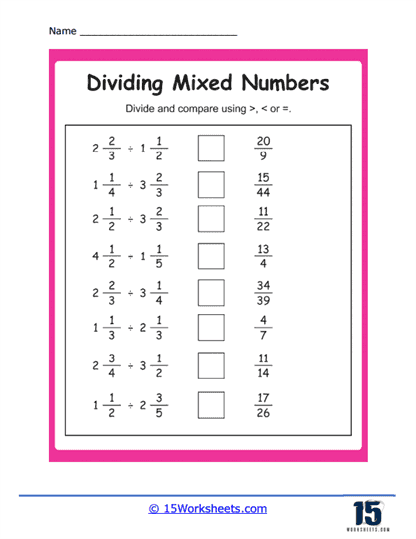
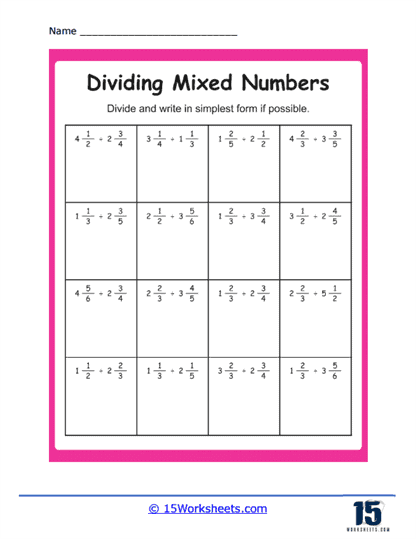
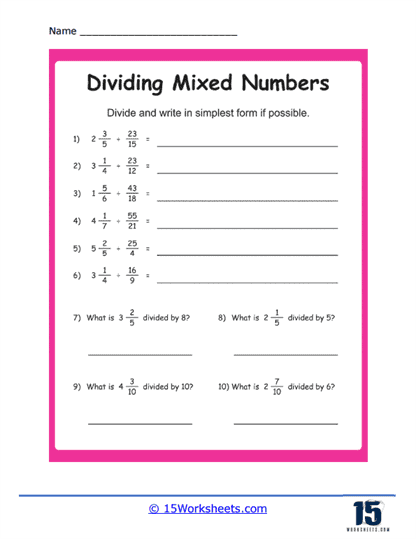
This is a collection of worksheets where the primary task involves dividing one mixed number by another. This division process isn’t as straightforward as dividing whole numbers, so practicing with worksheets can significantly help you understand and master this concept. There are several steps that you may need to do prior to performing the operation of division. This why this is considered a critical thinking activity.
So, what kind of problems can you expect to see on these worksheets?
Direct Division Problems – These are the simplest and most common. They’ll give you two mixed numbers and ask you to divide one by the other. For instance, you may need to divide 3 1/2 by 1 1/4.
Mixed Operation Problems – These problems involve more than just division. You might need to add, subtract, or multiply other numbers as part of the process. For instance, you may need to subtract 2 1/4 from 4 1/2 and then divide the result by 1 3/4.
Conversion Problems – These problems may require you to convert your answer between different forms. For example, you might have to provide your answer as an improper fraction or a decimal, even though you start with mixed numbers.
Simplification Problems – These problems may require you to simplify your answer. If the division results in an improper fraction, you’ll have to simplify it into a mixed number.
Why is this math skill so important for students to learn?
Understanding how to divide mixed numbers lays the foundation for more advanced math concepts. As you move into more advanced math, the ability to manipulate and operate with fractions becomes crucial. It’s a stepping stone towards algebra, calculus, and even statistics.
Dividing mixed numbers also enhances critical thinking and problem-solving skills. It requires several steps, each of which involves a decision point and a calculation. These processes stimulate your brain, helping to develop logical and strategic thinking skills.
Competence in dividing mixed numbers also helps to improve numerical literacy. Being comfortable with numbers, especially complex numbers like mixed numbers, is an essential part of being numerate. Numeracy is as vital as literacy in our everyday lives, from understanding finance to decoding statistics in the news.
Where can this skill be used in everyday life?
Cooking and Baking – Recipes often involve fractions, especially when they need to be adjusted for a different number of servings.
Shopping – When you’re comparing prices or figuring out discounts, you’re often working with fractions or mixed numbers.
Home Improvement – If you’re measuring for furniture or planning out a space, you might need to divide measurements.
Finance – Understanding fractions and mixed numbers can help when you’re calculating interest, tax, or other financial metrics.
Sports and Fitness – If you’re calculating pace or splitting workouts, fractions often come into play.
These worksheets are more than just a piece of paper with math problems. They are a tool for learning a vital math skill, building a foundation for more advanced concepts, enhancing critical thinking, and improving numerical literacy. Whether it’s through direct division problems, word problems, mixed operation problems, conversion problems, or simplification problems, these worksheets provide practice and insight into the world of dividing mixed numbers. It’s a skill that opens up understanding in various aspects of everyday life, making it not just a math skill, but a life skill.
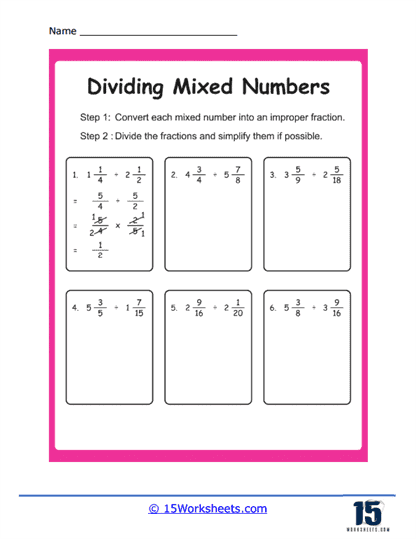
How to Divide Mixed Numbers
Suppose you have the problem of dividing 2 1/2 by 1 1/4. Here are the steps you’ll need to follow:
Step 1) Convert Mixed Numbers to Improper Fractions
An improper fraction is a fraction in which the numerator (top number) is greater than or equal to the denominator (bottom number). To convert a mixed number to an improper fraction:
Multiply the whole number by the denominator of the fraction.
Add that result to the numerator of the fraction.
The resulting number becomes your new numerator, while the denominator remains the same. For example:
Convert 2 1/2 to an improper fraction – (2 x 2 + 1)/2 = 5/2.
Convert 1 1/4 to an improper fraction – (1*4 + 1)/4 = 5/4.
Step 2) Flip the Second Fraction (Reciprocal)
The next step involves converting the division problem into a multiplication one. To do this, you “flip” the second fraction, making the numerator the denominator and vice versa. This flipped fraction is known as the reciprocal.
So, 5/4 becomes 4/5.
Step 3) Multiply the Fractions
Now, instead of dividing, we multiply the two fractions:
(5/2) x (4/5)
To multiply fractions:
Multiply the numerators together for the new numerator.
Multiply the denominators together for the new denominator.
So the multiplication looks like this:
(54) / (25) = 20/10
Step 4) Simplify the Result
The final step is to simplify the fraction, if possible. Simplify by dividing the numerator and the denominator by their greatest common divisor. If the result is an improper fraction, you may want to convert it back to a mixed number.
In our case:
20/10 = 2
So, 2 1/2 divided by 1 1/4 equals 2.