Comparing Mixed Numbers Worksheets
About These 15 Worksheets
Mixed numbers are an important part of the mathematics curriculum, especially for middle school students. They appear in many different real-life situations and are a critical stepping-stone to understanding fractions, decimals, percentages, and more complex mathematical principles.
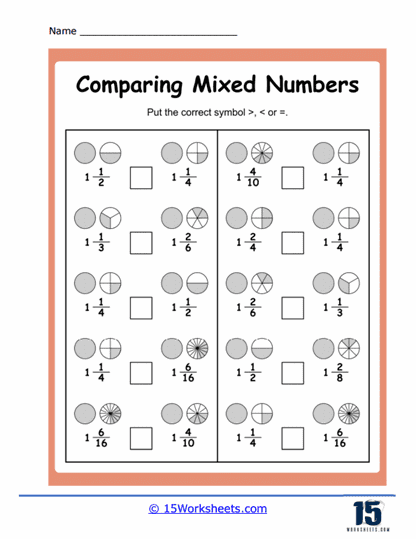
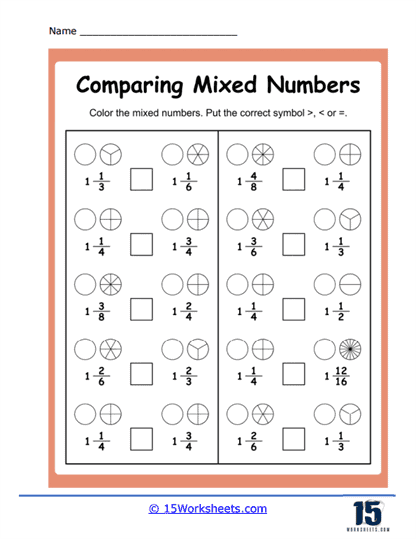
A mixed number is a whole number and a fraction combined into one “mixed” number. For example, 2 3/4 is a mixed number. We could say that it’s “2 and three-fourths.” These are worksheets that consist of various problems requiring you to compare different mixed numbers, determining whether one mixed number is larger, smaller, or equal to another. You usually denote these comparisons with symbols – “>” (greater than), “<” (less than), or “=” (equal to).
A Look At The Individual Worksheets
In the “Comparison Symbols” and “Symbols That Compare” worksheets, students become linguistic detectives, deciphering the cryptic language of “greater than,” “less than,” and “equal to.” It’s a delightful exercise in symbol literacy, where each comparison tells a story of numerical relationships.
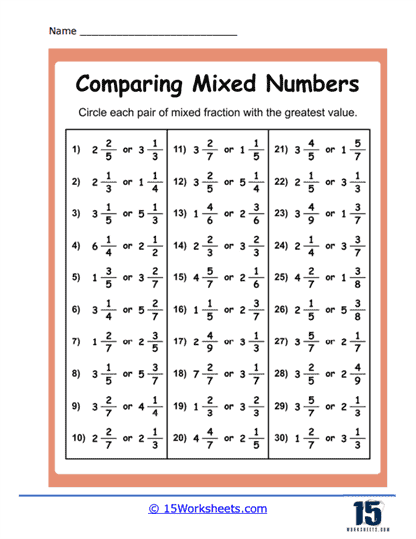
The “Compare Mixed Numbers” and “Comparing Products” worksheets challenge students to pit mixed numbers against each other in epic showdowns. It’s a mathematical version of a reality show, where numbers compete for the title of “Greatest Mixed Number,” and students are the discerning judges.
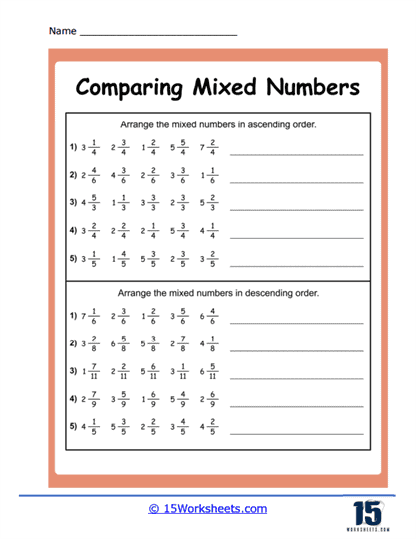
Ordering is the theme in “Ascending and Descending Order,” “Least to Most,” and “Smallest and Largest Value.” Here, students arrange mixed numbers in sequences, creating harmonious numerical symphonies. It’s a dance of digits, choreographed to perfection.
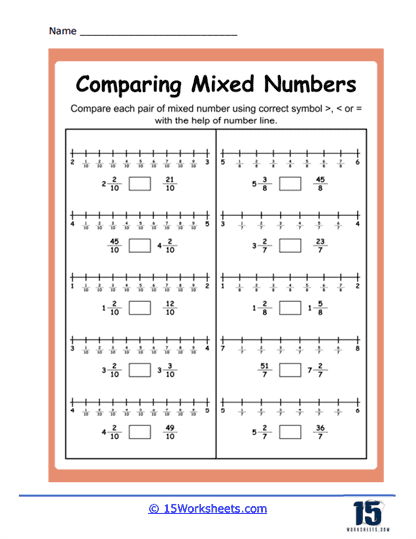
Visual learners rejoice with “Visual Mixed Numbers,” “Comparing Visual Mixed Numbers,” and “Visuals and Mixed Numbers.” These worksheets transform abstract concepts into tangible images, turning fractions into pieces of pie and mixed numbers into colorful mosaics. It’s art class meets math class in the most delightful way.
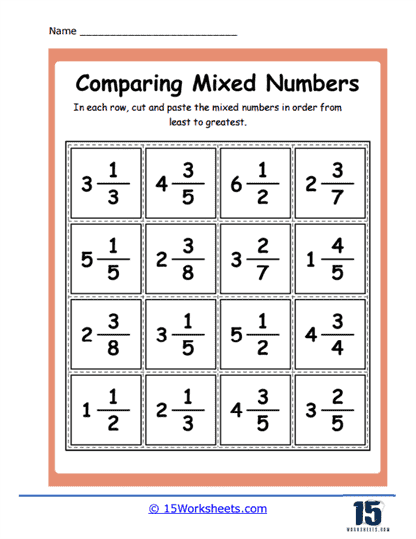
Finally, “Color and Compare” and “Growing Cut and Paste” bring creativity to the forefront. Students use colors and scissors to explore mixed numbers, making learning a hands-on adventure. It’s a reminder that math isn’t just about numbers; it’s about patterns, designs, and the joy of discovery.
Why Do We Compare Mixed Numbers?
Comparing mixed numbers is a fundamental mathematical skill for several reasons. First, it helps students understand and compare quantities, an essential concept not just in math but in various other subjects like physics, chemistry, and even economics.
It’s also a building block for more advanced mathematical concepts. Without a strong understanding of how to compare mixed numbers, it’s much more difficult to learn about and understand concepts like algebra or calculus in high school.
Moreover, developing skills in comparing mixed numbers helps in sharpening logical thinking and problem-solving skills, which are crucial, transferrable skills in everyday life.
Uses in Everyday Life
Cooking – When following a recipe, you often have to deal with mixed numbers. You might need to compare quantities to adjust the recipe or divide the meal into portions.
Shopping – Whether you’re comparing prices, discounts, or quantities, a good grasp of mixed numbers can help you make smart decisions.
Time Management – If you’re managing your time or comparing the duration of different activities, understanding mixed numbers can be helpful.
Sports – Many sports require the understanding of fractions and mixed numbers, for instance, understanding how innings work in baseball or comparing players’ stats.
Travel – When planning a trip, comparing distances, travel times, or fuel consumption often involves mixed numbers.
These worksheets were designed to improve your understanding of how to compare mixed numbers-a vital mathematical skill. Whether it’s direct comparisons, ordering, solving word problems, handling mixed problems, or tackling conversion problems, these worksheets offer a wealth of practice. It’s a skill that not only unlocks the door to more complex mathematical concepts but also has practical applications in everyday life. So, the next time you come across a worksheet on comparing mixed numbers, remember that you’re not just learning math, you’re learning a life skill.
How to Compare Two Mixed Numbers
Step 1) Compare the Whole Number Part
The first step to compare two mixed numbers is to look at the whole number part of each. For instance, if you have 3 1/2 and 2 3/4, the whole numbers are 3 and 2 respectively. Since 3 is greater than 2, we can say that 3 1/2 is greater than 2 3/4, without needing to compare the fractional parts.
Step 2) Compare the Fractional Part if Whole Numbers are Equal
What if the whole numbers are the same? In that case, you need to compare the fractional parts. If you have 3 1/2 and 3 3/4, the whole numbers are both 3. So, we look at the fractions, 1/2 and 3/4. Here, 3/4 is greater than 1/2, so 3 3/4 is greater than 3 1/2.
Step 3) Make Denominators the Same, if Necessary
But how do you compare fractions if they don’t have the same denominator? You have to find a common denominator. For example, let’s compare 2 1/2 and 2 2/3. The whole numbers are equal, so we look at the fractions. But the denominators, 2 and 3, are not the same.
A common denominator for 2 and 3 is 6. We convert each fraction to an equivalent fraction with a denominator of 6:
1/2 becomes 3/6 (multiply the numerator and denominator by 3)
2/3 becomes 4/6 (multiply the numerator and denominator by 2)
So, now you’re comparing 2 3/6 and 2 4/6. It’s clear that 4/6 is larger than 3/6, so 2 2/3 is larger than 2 1/2.
Step 4) Convert to Improper Fractions or Decimals (if needed)
Sometimes, converting the mixed numbers to improper fractions or decimals can make the comparison easier. This is especially true for complex problems or if the numbers are difficult to compare at a glance.
An improper fraction is a fraction in which the numerator is greater than or equal to the denominator. For example, to convert 2 1/2 to an improper fraction, you would multiply the whole number part by the denominator of the fraction, then add the numerator, resulting in 5/2.
To convert a mixed number to a decimal, you divide the numerator of the fraction by the denominator and add the whole number. For example, 2 1/2 would become 2.5 as a decimal.
These conversions can simplify the process of comparing mixed numbers, especially if you’re comfortable with fractions or decimals.