Common Denominators Worksheets
All About These 15 Worksheets
Mastering fractions is a crucial stepping stone in every student’s mathematical journey, and at the heart of that mastery is the ability to find and use common denominators. These Common Denominators worksheets are meticulously designed to guide students through this essential concept, building both their confidence and competence in working with fractions. By providing a structured yet flexible approach, these worksheets do more than just offer practice-they create opportunities for deeper understanding and meaningful learning.
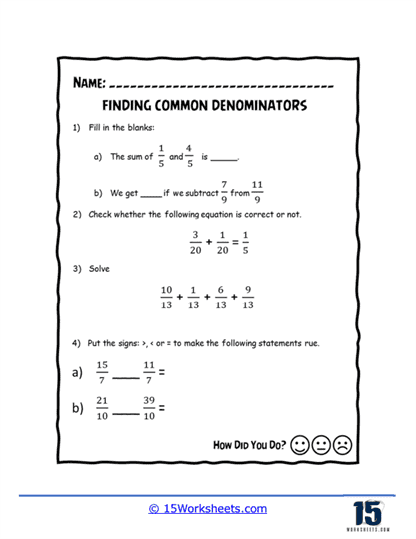
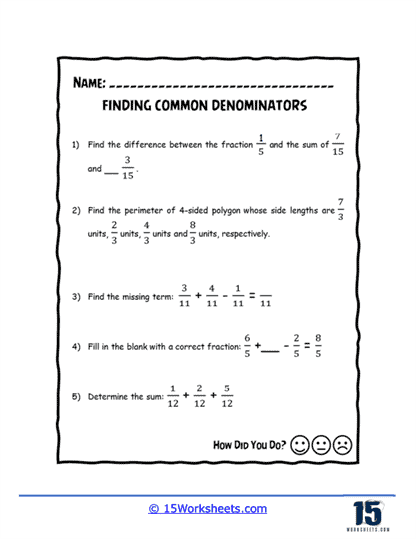
The importance of common denominators cannot be overstated. When working with fractions, whether for comparing, adding, or subtracting them, students must first align the denominators. Without this skill, they will struggle to grasp more complex mathematical operations later on. These worksheets offer a wide range of problems, each crafted to challenge students while ensuring that they progressively build their skills. The problems are designed with varying levels of difficulty to cater to students with different learning paces, ensuring that whether they are just beginning or seeking more advanced challenges, there’s always room for growth.
One of the standout features of these worksheets is the variety of problem types they include. Students aren’t just passively working through repetitive exercises-they are actively engaging with fractions in different contexts. Some problems ask students to find common denominators for pairs of fractions, while others require them to work with groups of fractions, converting them into equivalent fractions with a shared denominator. This diversity of problems helps students apply the concept in different scenarios, reinforcing their understanding through multiple angles. The worksheets encourage students to develop a flexible, adaptable mindset, a critical skill in math and beyond.
The expedition commences with “Finding Multiples” and “Matching Denominators,” where students play the role of numerical detectives, uncovering patterns and aligning denominators like seasoned matchmakers. “Least Common Denominator” and “Processing Denominators” elevate the challenge, prompting learners to delve deeper into the intricacies of fraction equivalence, all while maintaining a light-hearted tone that keeps the journey enjoyable.
As students progress, they encounter “Common Ground” and “Solid Base,” worksheets that metaphorically and literally lay the foundation for understanding shared denominators. “Walk Through Multiples” and “Here to There” guide learners through the maze of multiples, illustrating the pathways that lead different fractions to a common destination. These exercises not only reinforce mathematical concepts but also encourage students to appreciate the interconnectedness of numbers.
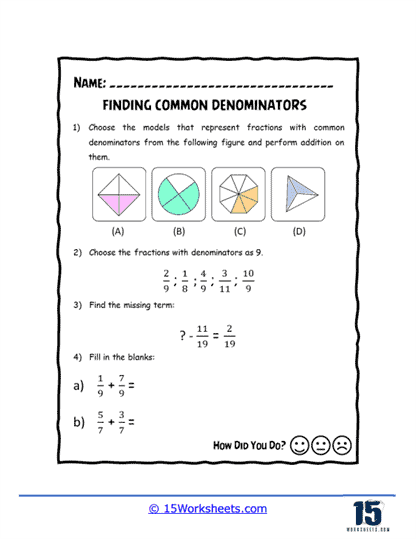
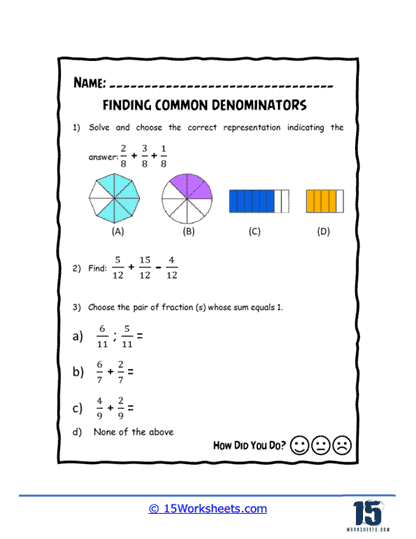
Venturing further, “Working With Larger Denominators” and “Unlike and Like Fractions” present scenarios that challenge students to apply their knowledge in more complex contexts. “Add and Subtract Unlikes” introduces operations with fractions that initially seem incompatible, teaching the art of reconciliation through common denominators. “Models and Fraction Identification” offers visual representations, aiding those who benefit from seeing fractions in a more tangible form.
The journey concludes with “Travelling Denominators,” “3 Questions About Denominators,” and “Denominator Sentences,” worksheets that encourage students to reflect on their learning, pose critical questions, and articulate their understanding in complete sentences. These final steps ensure that learners have not only practiced the mechanics of common denominators but have also internalized the concepts, ready to apply them in real-world situations.
For many, fractions can be a source of frustration or confusion, but with the structured support provided by these worksheets, students can overcome these challenges. Each time they solve a problem, find a common denominator, or convert fractions, they are building a stronger foundation in math. And this foundation is not just about passing the next test-it’s about equipping students with the skills they need for future math topics, like algebra and geometry, where fractions play a crucial role.
How to Find Common Denominators For Fractions
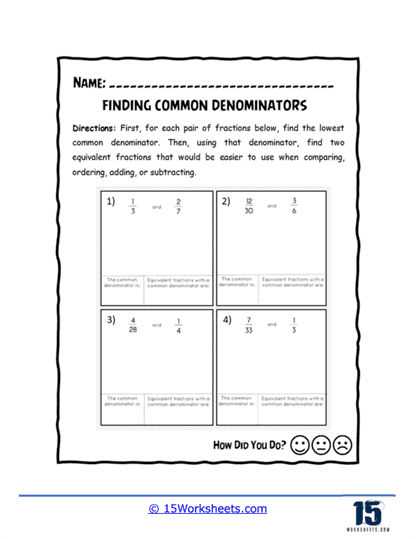
To find a common denominator for two or more fractions, follow these steps:
List the Multiples of Each Denominator
Write down the multiples of each denominator, starting with the denominator itself and increasing in value.
For example, if you have two fractions with denominators 3 and 5, list their multiples:
- Multiples of 3 – 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, …
- Multiples of 5 – 5, 10, 15, 20, 25, 30, 35, 40, 45, 50, …
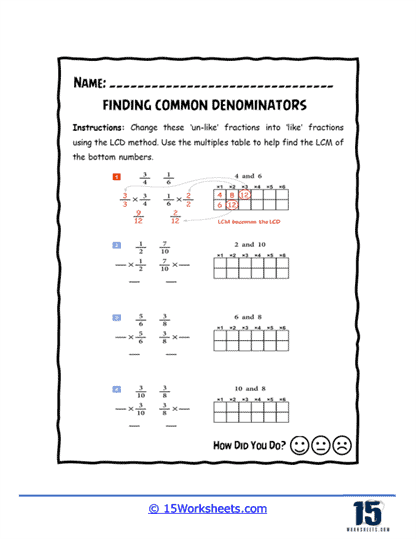
Identify the Least Common Multiple (LCM) of the Denominators
The LCM is the smallest number that is a multiple of each denominator. You can find the LCM by looking at the lists of multiples and finding the smallest number that appears in each list.
In our example, the least common multiple of 3 and 5 is 15 because it is the smallest number that appears in both lists of multiples.
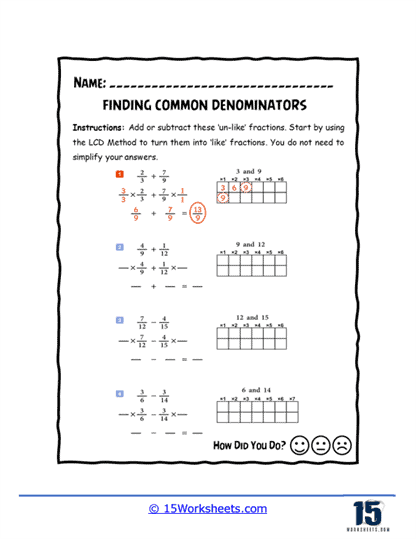
Convert Each Fraction to an Equivalent Fraction with the Common Denominator
To do this, divide the LCM by the original denominator, then multiply both the numerator and the denominator of the fraction by the result.
For example, let’s say you have the fractions 2/3 and 3/5. To convert them to equivalent fractions with a common denominator of 15:
For 2/3, divide 15 by 3 to get 5. Multiply both the numerator and denominator of 2/3 by 5 to get 10/15.
For 3/5, divide 15 by 5 to get 3. Multiply both the numerator and denominator of 3/5 by 3 to get 9/15.
Now, you have the equivalent fractions 10/15 and 9/15 with a common denominator of 15. You can use these fractions to perform arithmetic operations like addition, subtraction, multiplication, or division.
How to Find Common Denominators For Mixed Numbers
To find common denominators for mixed numbers, you first need to convert the mixed numbers into improper fractions. Then, follow the same steps as you would for finding common denominators for fractions. Here’s a step-by-step guide:
Convert Mixed Numbers to Improper Fractions
To do this, multiply the whole number by the denominator and add the result to the numerator. The result will be the new numerator, while the denominator remains the same.
For example, let’s say you have mixed numbers 2 1/4 and 1 2/3.
- To convert 2 1/4 to an improper fraction, multiply 2 by 4 and add 1: (2 * 4) + 1 = 9. The improper fraction is 9/4.
- To convert 1 2/3 to an improper fraction, multiply 1 by 3 and add 2: (1 * 3) + 2 = 5. The improper fraction is 5/3.
List the Multiples of Each Denominator
Write down the multiples of each denominator, starting with the denominator itself and increasing in value.
- Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40, …
- Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30, …
Identify the Least Common Multiple (LCM) of the Denominators
The LCM is the smallest number that is a multiple of each denominator. In our example, the least common multiple of 4 and 3 is 12.
Convert Each Improper Fraction to an Equivalent Fraction with the common Denominator
Divide the LCM by the original denominator, then multiply both the numerator and the denominator of the fraction by the result.
- For 9/4, divide 12 by 4 to get 3. Multiply both the numerator and denominator of 9/4 by 3 to get 27/12.
- For 5/3, divide 12 by 3 to get 4. Multiply both the numerator and denominator of 5/3 by 4 to get 20/12.
Now, you have the equivalent improper fractions 27/12 and 20/12 with a common denominator of 12. You can use these fractions to perform arithmetic operations like addition, subtraction, multiplication, or division. If necessary, convert the resulting improper fraction back to a mixed number.