Adding Mixed Numbers Worksheets

All About These 15 Worksheets

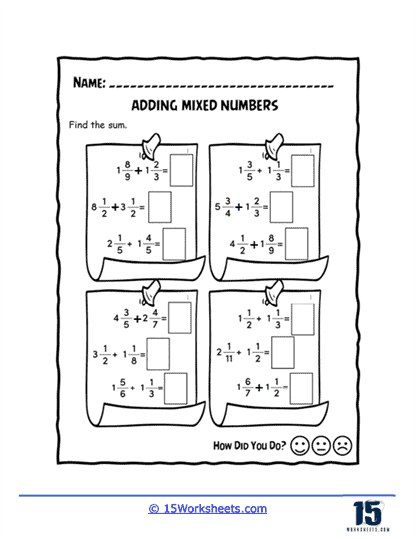
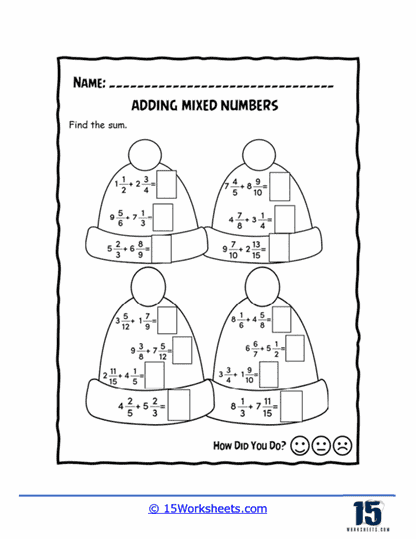
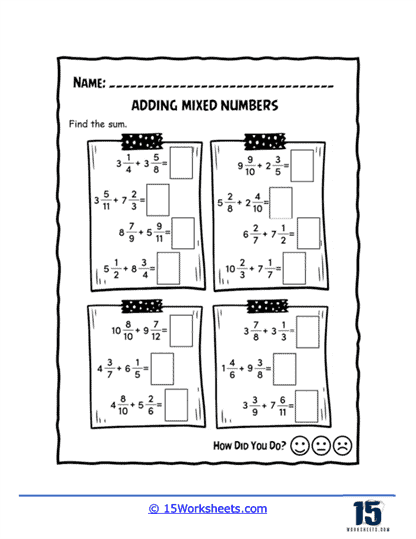
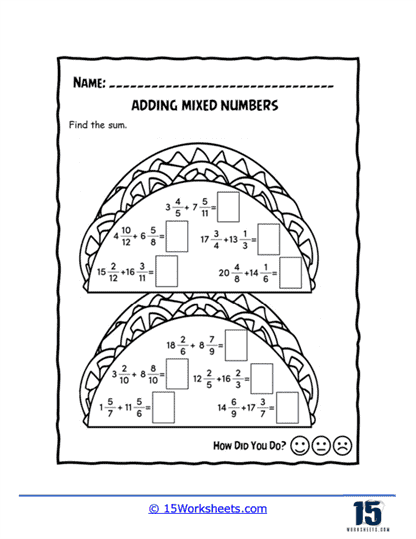
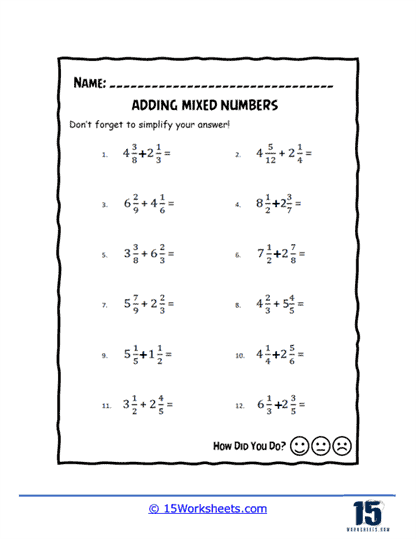
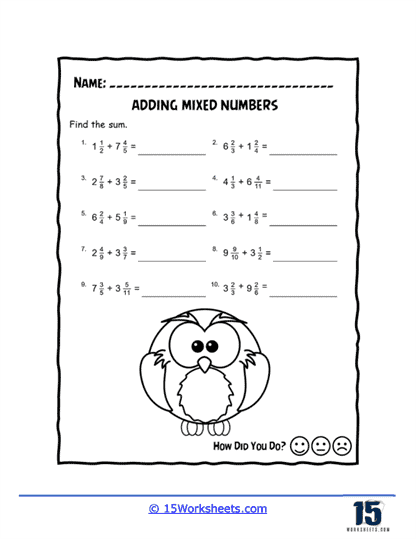
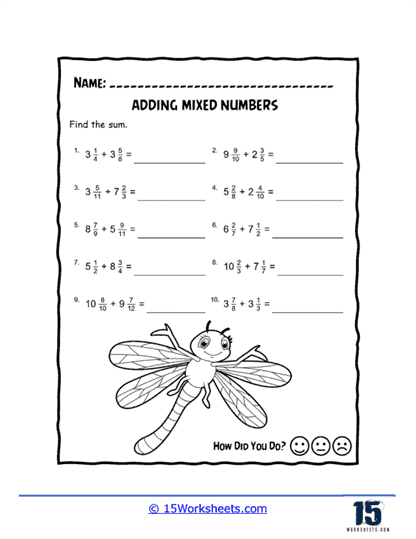
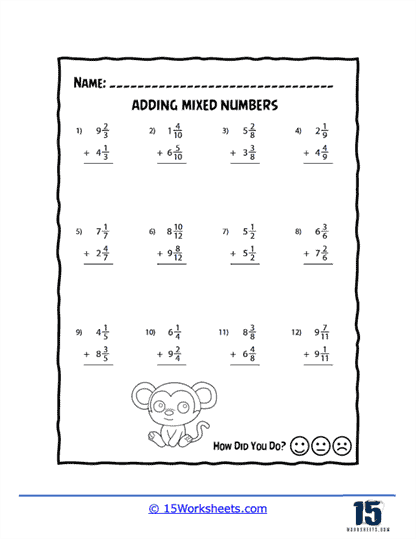
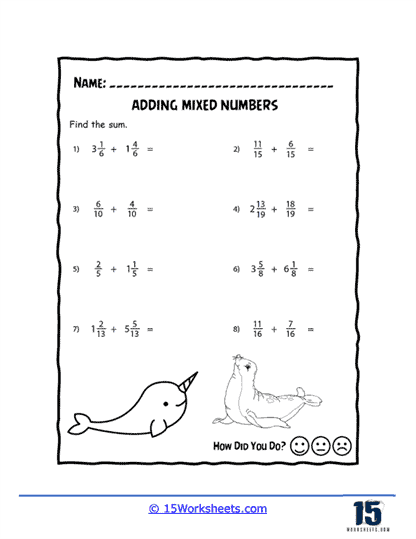
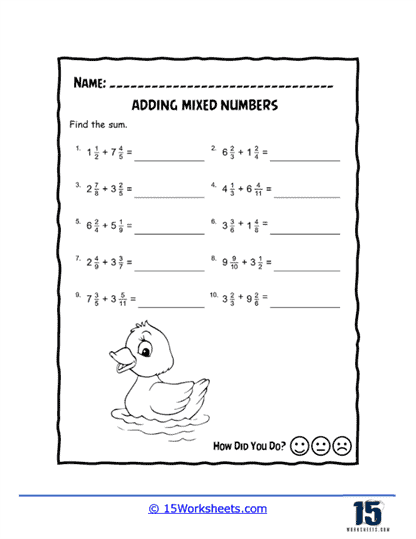
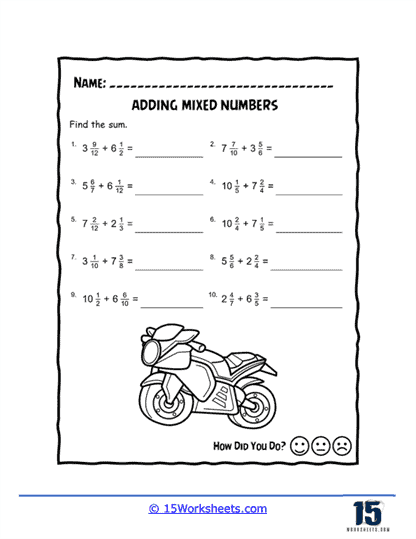
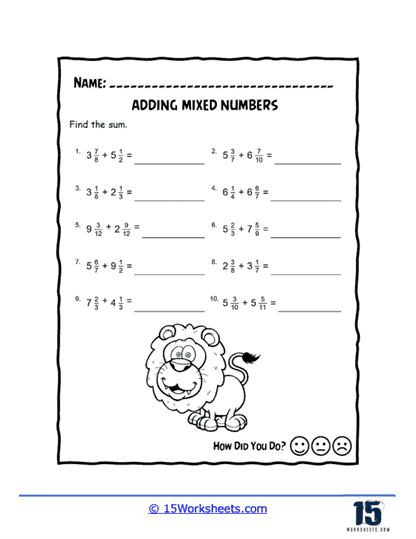
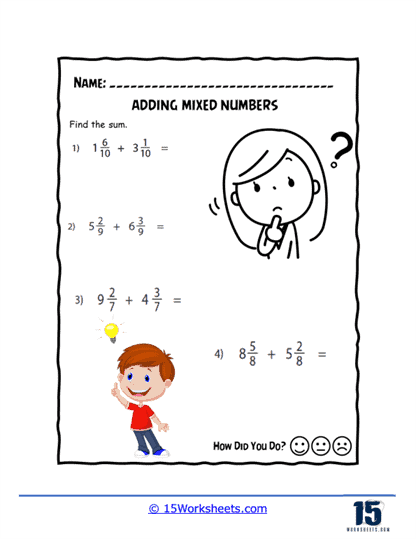
These Adding Mixed Numbers worksheets are designed to help students master the concept of adding mixed numbers. Mixed numbers are numbers that consist of a whole number and a fraction. The worksheets include a variety of creative and engaging exercises to help students build their skills and confidence in solving these types of math problems.

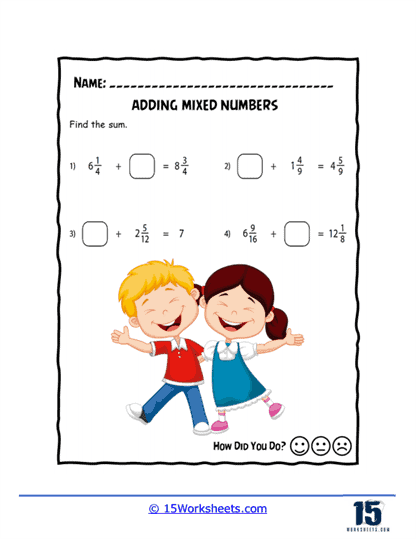
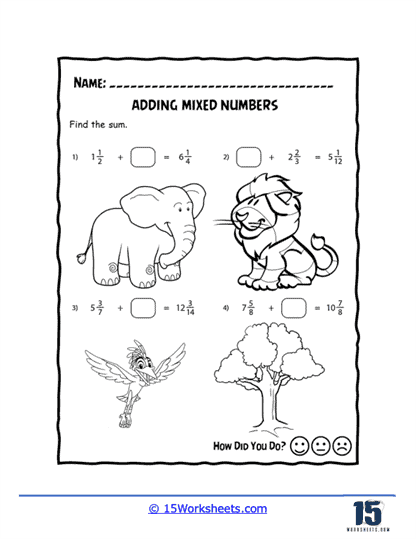
Each worksheet in the series includes a mix of problems with varying degrees of difficulty, designed to challenge and engage students at different skill levels. The problems require students to add mixed numbers with like and unlike denominators. Other worksheets in this series provide more challenge by asking students to find the missing addend instead of the sum in an equation involving mixed numbers.

This series is most suitable for students in grades 5 to 7. It can be used in the classroom as a supplement to the math curriculum or at home as a way for parents to help their children practice and reinforce their math skills.

Overall, these Adding Mixed Numbers worksheets provide a comprehensive and engaging way for students to develop their skills and confidence in adding mixed numbers. With these worksheets, students can build a solid foundation in math and develop the skills they need to succeed in higher-level math courses.

How to add mixed numbers

Here’s a step-by-step guide on how to add mixed numbers, along with an example:

Add 2 1/4 + 3 3/8

Step 1: Find a common denominator.

The denominators are 4 and 8. The lowest common multiple of 4 and 8 is 8, so we can use 8 as our common denominator.

Step 2: Convert mixed numbers to improper fractions.

2 1/4 can be converted to (2 x 4) + 1 = 9/4 3 3/8 can be converted to (3 x 8) + 3 = 27/8

Step 3: Add the fractions.

9/4 + 27/8 = (9 x 2)/(4 x 2) + 27/8 = 18/8 + 27/8 = 45/8

Step 4: Simplify the fraction (if necessary).

45/8 is already in its simplest form, so we don’t need to simplify it any further.

Step 5: Convert the improper fraction to a mixed number (if necessary).

To convert 45/8 to a mixed number, we divide the numerator (45) by the denominator (8). This gives us 5 with a remainder of 5. So the mixed number is 5 5/8.

Step 6: Check the answer.

We can check our answer by adding the mixed numbers again using a different method, such as converting both mixed numbers to improper fractions with a common denominator. If we get the same answer (5 5/8), we know our original answer is correct.

So the final answer is 5 5/8.