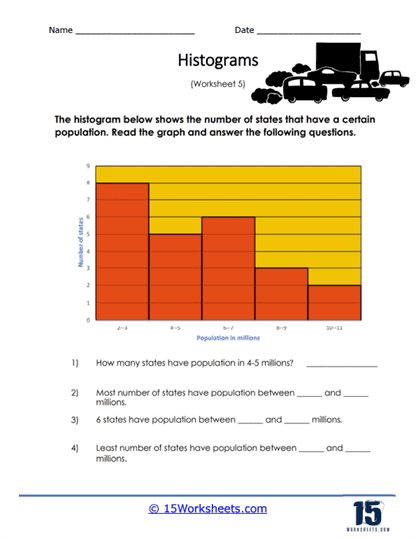
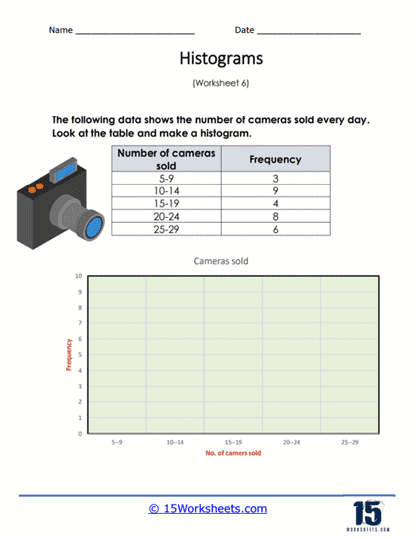
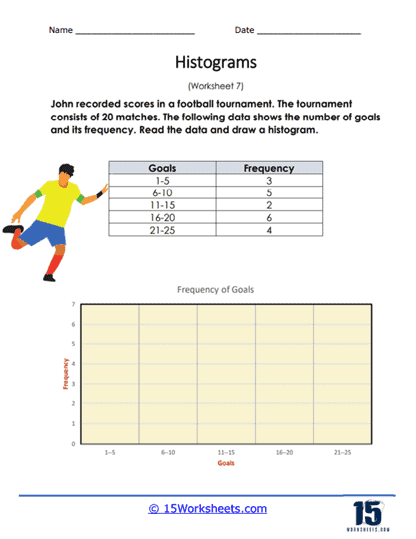
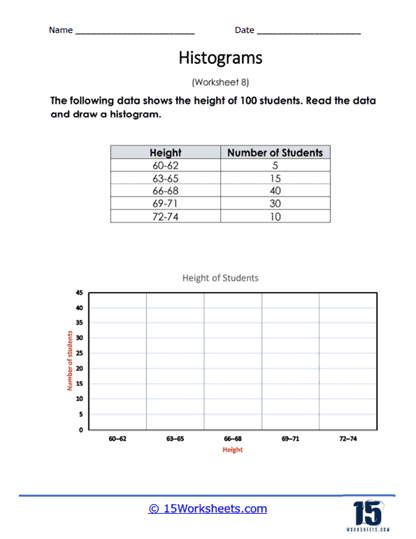
Histograms Worksheets

About These 15 Worksheets

Imagine a classroom filled with students, each with their own story of how many books they read in a month. Some are avid readers, tearing through novels, while others manage just one or two due to busy schedules. Now, what if you could take all that information and bring it to life through a visual tool that instantly reveals the reading habits of the entire class? This is where a histogram worksheet comes into play, transforming raw data into a clear, compelling picture that anyone can understand at a glance.

A histogram is more than just a graph; it’s a window into the underlying patterns of a dataset. Let’s say you’re interested in how many books your classmates read in a given month. A histogram worksheet will guide you step by step through the process of turning those numbers into a visual story. You’ll start with a simple set of data-perhaps a list of how many books each student read. This might seem like just a jumble of numbers at first, but a histogram will bring structure and meaning to the chaos.

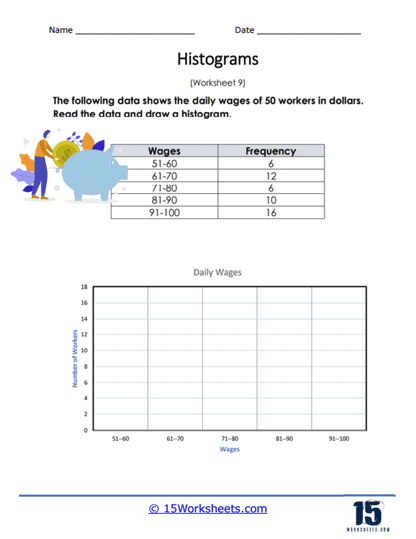
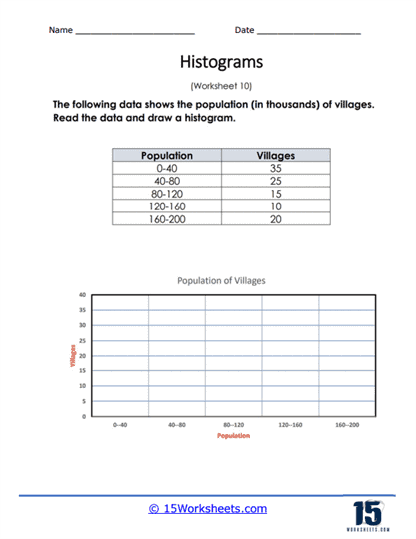
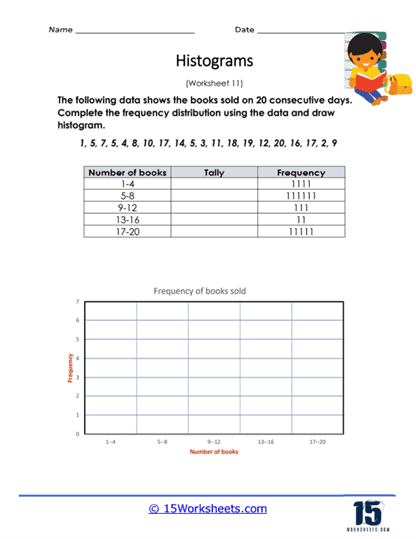
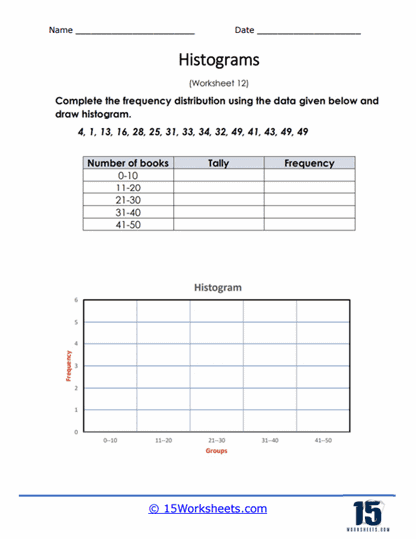
The first thing you’ll notice on a histogram worksheet is a table or a list of values. Each number represents a different student’s reading habits. But instead of just looking at individual numbers, which can be overwhelming, the worksheet will ask you to organize the data into intervals or “bins.” These bins might group the number of books read into ranges-such as 0-5 books, 6-10 books, and 11-15 books. This step is crucial because it simplifies the data, making it easier to spot trends and patterns.

Once the data is sorted into bins, the next step is setting up the axes of your histogram. Imagine drawing a graph with a vertical and a horizontal axis, much like the x and y axes in math class. But this time, the axes are labeled to reflect the data you’ve organized. For instance, the horizontal axis will show the bins you’ve created, like “0-5 books,” “6-10 books,” and so on. The vertical axis, on the other hand, will represent the frequency, or how many students fall into each bin.

Now comes the exciting part-actually building the histogram. You’ll look at your table of data and count how many students fit into each range. For example, you might find that three students read between 0 and 5 books, while five students read between 6 and 10 books. Each count becomes a bar on your histogram, and the height of the bar reflects how many students fall into that specific category. As you plot each bar, you’ll begin to see a clear picture of the data emerging, like pieces of a puzzle coming together.

The beauty of a histogram lies in its simplicity. Once all the bars are drawn, you can instantly see which range has the most students and which has the fewest. The taller the bar, the more students in that category. Maybe you’ll notice that most students read between 6 and 10 books, or perhaps the majority fall into the 0-5 range. Either way, the histogram makes it obvious at a glance-no complicated calculations needed. The visual nature of the histogram allows you to quickly understand the distribution of the data without sifting through rows of numbers.

But the value of a histogram worksheet doesn’t stop at creating the graph. It also challenges you to think critically about the data. After constructing the histogram, you might be asked questions like, “Which range has the most students?” or “Does the data suggest that more students are heavy readers or light readers?” These questions push you to not just look at the graph, but to interpret what it means. You’re not just plotting points-you’re learning to analyze and draw conclusions from the data in front of you.

This hands-on approach to data visualization is incredibly powerful. In a world where data is everywhere, the ability to organize and interpret information is a crucial skill. Histogram worksheets give you a foundation in these essential skills by showing you how to break down complex information and present it in a way that’s easy to understand. Whether you’re studying math, science, or even social studies, histograms are a versatile tool that can be applied to a wide range of topics.

For example, in science class, you might use a histogram to represent the distribution of temperatures over a week or the height of plants in an experiment. In social studies, histograms can help you analyze population data, economic trends, or voting patterns. The possibilities are endless because histograms are a universal tool for making sense of numbers, no matter the subject.

What Are Histograms?

Histograms are a type of graphical representation used to display the distribution of a continuous dataset by grouping the data into a series of intervals or bins. Each bin represents a range of values, and the height of each bar in the histogram corresponds to the frequency (number of occurrences) or density (percentage) of the data points that fall within that specific bin.

Histograms are particularly useful for understanding the shape and spread of a dataset, such as identifying patterns, trends, or outliers. They can provide insights into the central tendency (mean, median, mode), dispersion (range, variance, standard deviation), and the overall distribution (normal, skewed, bimodal) of the data.

For example, if you have a dataset of test scores for a large group of students, you can create a histogram to visualize the distribution of scores. The x-axis would represent score ranges (bins), and the y-axis would represent the frequency or density of students who achieved scores within each bin. By examining the shape of the histogram, you can gain insights into the overall performance of the students and identify any trends or outliers in the data.

A class of 12 students receive the following scores out a possible 100:

88, 67, 98, 75, 85, 62, 76, 80, 90, 76, 91, 83

We would create a histogram like this:

We can quickly understand the frequency distribution of the scores. We can understand that a slight majority of the students scored over 80%.

It’s important to note that the choice of bin size and the number of bins can significantly impact the appearance of a histogram. Smaller bins may reveal more details about the data distribution, while larger bins may provide a more general overview. However, using too few or too many bins can lead to a misleading representation of the data, so it’s crucial to choose an appropriate bin size and number to accurately visualize the dataset.

What Are Histograms Best Used For?

Histograms are a great way to show how a bunch of numbers are spread out. They look like bar graphs, but instead of comparing different things, they focus on how often certain numbers appear in a set of data. To make a histogram, the data is grouped into ranges, called “bins,” and the height of each bar shows how many numbers fall into each range. This helps you see patterns, like whether most of the numbers are close together or spread far apart.

One cool thing about histograms is that they make it easy to spot trends in the data. For example, if the bars form a nice hill shape, that usually means the data is “normal” or balanced. But if the bars are bunched up on one side, it means the data is uneven or “skewed.” This can tell you whether something unusual is happening with the numbers, like an unexpected outlier that stands out from the rest.

Histograms are also helpful for understanding how much the numbers in the data vary. If the bars are tall and close together, it means the numbers are pretty similar. But if the bars are spread out and shorter, it means the numbers are more different from each other. This is important when you want to see how consistent the data is, like when you’re checking test scores or measuring results in a science experiment.