Exponential Form Worksheets

About These 15 Worksheets

These worksheets provide students with exercises designed to enhance their understanding of exponents and their use in mathematical expressions. These worksheets are crafted to guide students through the many facets of working with powers, from basic introduction to more complex applications. A student’s journey into the world of exponents typically begins with understanding what an exponent is – a notation that represents the number of times a base number is multiplied by itself.

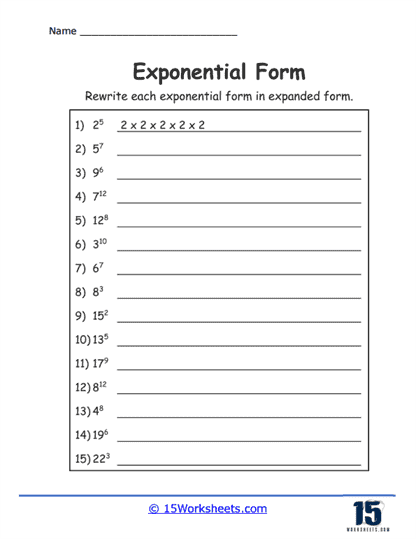
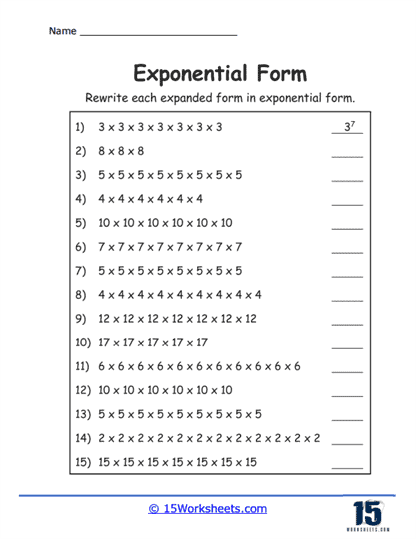
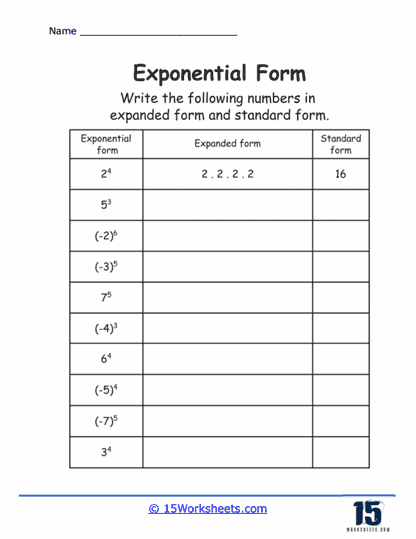
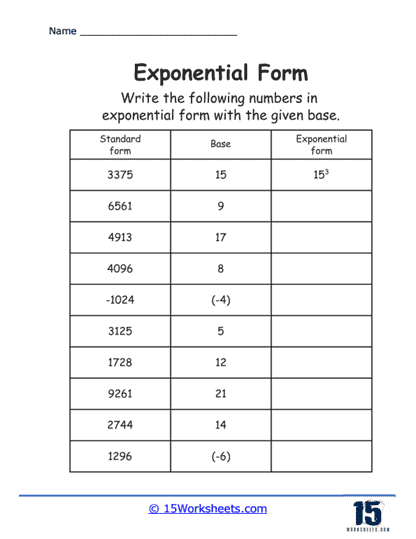
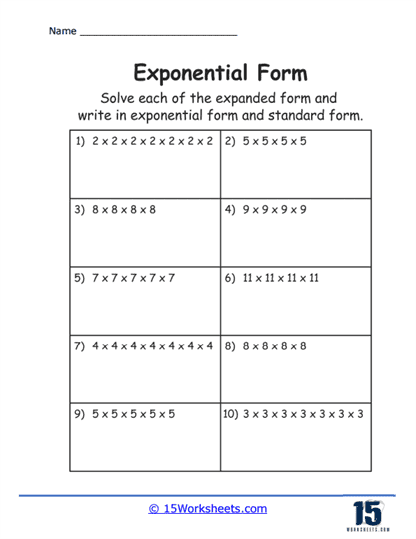
The various exercises found on these worksheets are strategically designed to cover a wide spectrum of concepts related to exponents. Students start with basic exponentiation tasks, which might involve writing out repeated multiplications to identify the base and the exponent. They might be asked to rewrite the expression 2 × 2 × 2 in exponential form as 2³. This initial step solidifies the relationship between exponentiation and multiplication.

Progressing in complexity, worksheets introduce the laws of exponents, such as the product rule, which states that when multiplying two powers with the same base, you add the exponents (x^a x x^b = x^{a + b}). Exercises will prompt students to practice this rule with numerical and algebraic expressions. For example, multiplying 3² by 3⁴ and simplifying it to 3⁶. Similarly, worksheets cover the power rule, where students learn to raise a power to another power by multiplying the exponents ((x^a)^b = x^ab), and the quotient rule, which teaches that dividing powers with the same base requires subtracting the exponents ((x^a / x^b = x^{a – b} ).

As students become comfortable with the basic rules, they encounter exercises that combine multiple laws of exponents in one expression, or that intermingle exponent rules with other operations, such as multiplication or division of terms that are not like bases. This requires a deep understanding of the order of operations and the ability to apply multiple exponent rules successively.

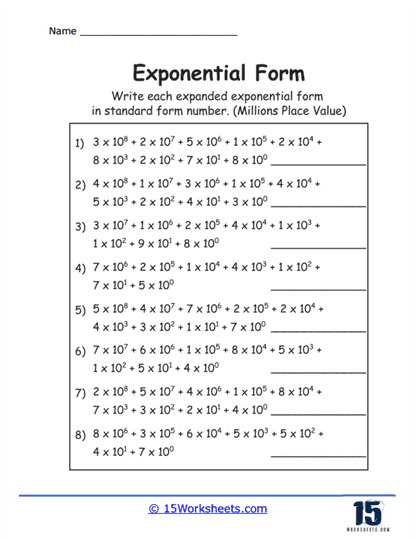
Another common type of exercise deals with scientific notation, a way of expressing very large or very small numbers using exponents. These worksheets teach students to convert standard decimal numbers into scientific notation and vice versa, which involves moving the decimal point to create a new base number between 1 and 10, and then counting the places moved as the power of 10. For example, converting the number 5,000 into scientific notation would yield 5 x 10³.

Higher-level worksheets might introduce the concepts of fractional exponents and their relation to roots, as well as exponential equations where the variable is in the exponent, leading to the use of logarithms for solving.

The Benefits Of These Worksheets

Practicing with these worksheets helps students in a multitude of ways. First and foremost, it solidifies their foundational knowledge of exponents, making abstract mathematical ideas concrete. Regular practice also enhances students’ numerical fluency and their ability to perform calculations involving powers more quickly and accurately.

Working with exponents through these worksheets encourages strategic thinking. Students develop problem-solving skills as they learn to recognize when and how to apply different exponent rules. It builds their confidence in manipulating expressions and equations involving exponents, which is crucial for higher-level math courses.

Practicing exponentiation also helps students develop an understanding of the hierarchy in mathematics, particularly the order of operations, as many exponentiation exercises require the simultaneous application of various mathematical principles. They learn that mathematics is not just about knowing the rules but also about understanding when and how to apply them efficiently.

These worksheets also prepare students for real-world applications. Mathematics is not just an academic subject but a tool used in science, engineering, finance, and data science. A solid grasp of exponents and their operations is vital for anyone pursuing a career in these fields.

What Is The Difference Between Standard Form and Exponential Form?

Standard form and exponential form are two ways of expressing numbers in mathematics, each with its own uses and characteristics. Understanding the difference between these two forms is crucial for performing various mathematical operations and applications.

Standard Form

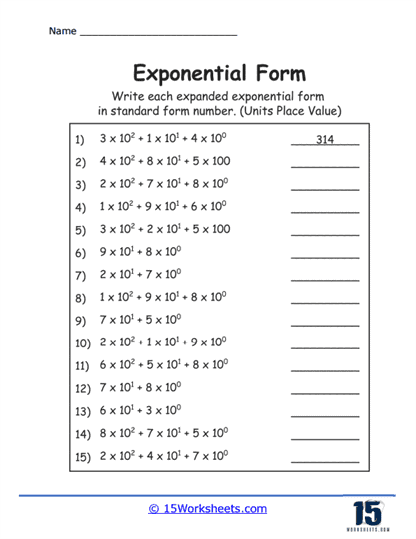
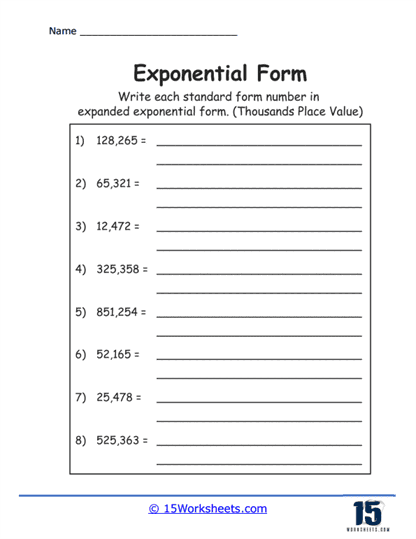
Standard form, also known as positional notation, is the typical way of writing numbers using digits and place value. In this system, the value of a digit is determined by its position in the number. For example, in the number 345, the 5 is in the ones place, the 4 is in the tens place, and the 3 is in the hundreds place. The number is understood as the sum of these values – 3 x 100 + 4 x 10 + 5 x 1. Standard form is how numbers are usually written and understood in daily life.

In a different context, particularly in the fields of science and engineering, standard form can also refer to scientific notation, which is used to write very large or very small numbers. This is done by expressing a number as a product of a coefficient (between 1 and 10) and a power of 10. For instance, the scientific standard form of 2,000 is 2 x 10³, and the standard form of 0.005 is 5 x 10^-3.

Exponential Form

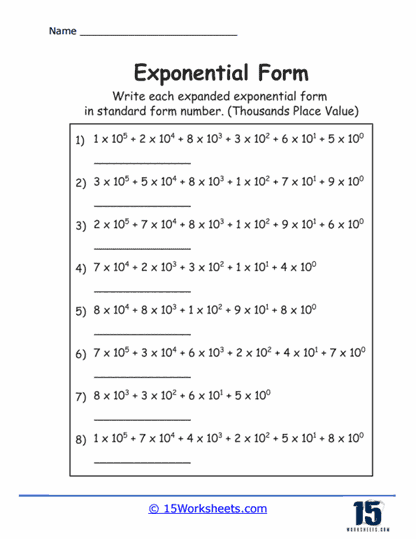
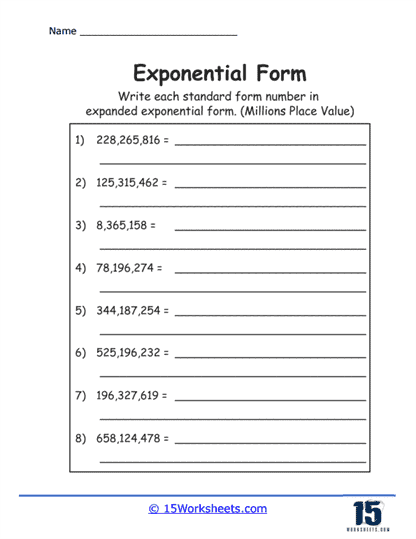
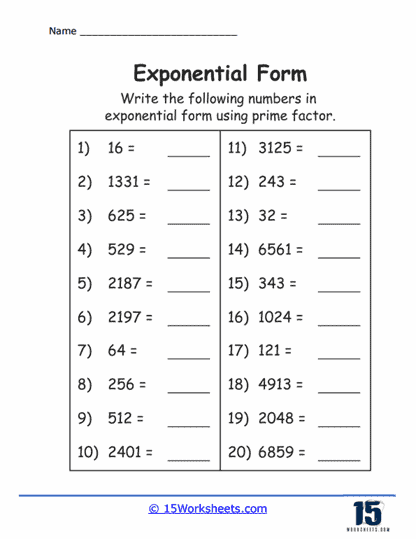
Exponential form, on the other hand, is a way of expressing numbers using exponents to signify repeated multiplication of a base number. An exponent shows how many times the base number is multiplied by itself. For instance, 2⁴ (read as “two to the fourth power” or “two raised to the power of four”) represents 2 x 2 x 2 x 2, which equals 16. In this expression, 2 is the base, and 4 is the exponent. Exponential form is especially useful for simplifying and working with very large numbers, very small numbers, and in algebraic expressions.

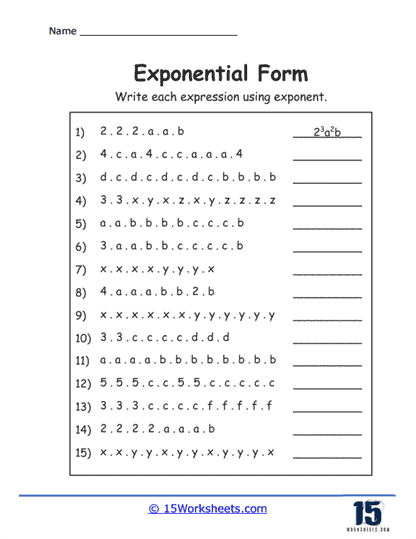
Exponential form can also be used to express the multiplication of variables. For example, y⁵ means y x y x y x y x y. When working with algebra, exponential form can make it easier to handle polynomials and manipulate expressions involving variables.

Comparison

The main difference between standard form and exponential form lies in their representation of numbers and their use. Standard form is a straightforward expression of the number as we regularly see and use it, showing the value of each digit based on its place value. Exponential form is more about expressing a number in terms of a base raised to a power, indicating how many times the base number is used as a factor in multiplication.

Here’s a practical comparison using the number 1,000:

In standard form, 1,000 is simply written as “1,000”, representing one thousand units.

In exponential form, 1,000 can be written as 10³, signifying that the number 10 is used as a factor three times (10 × 10 × 10).

Understanding these forms and the ability to convert between them is a key skill in mathematics. Standard form is often used for basic arithmetic and everyday counting, while exponential form is indispensable for higher mathematics, including algebra, calculus, and various scientific applications. They both reflect the inherent versatility and precision of the language of mathematics.

Real World Use Of Exponential Form

Exponential form is a powerful mathematical tool that finds its application in numerous fields beyond the classroom, helping to model phenomena where quantities grow or decay at rates proportional to their current value. Its ubiquity in the real world is undeniable, from the vast expanses of space to the minute intricacies of subatomic particles.

In the sciences, particularly physics and chemistry, exponential form is indispensable. Scientists use scientific notation, an application of exponential form, to handle the enormous range of measurements they work with. For example, the mass of an electron is a minuscule 9.11 x 10^-31 kilograms, while the distance to the nearest stars is a whopping 9.461 x 10¹⁵ meters.

Exponential decay, another application, is used to describe the rate at which radioactive substances lose their activity, with their activity halving over fixed periods known as half-lives. In biology, exponential growth equations model how populations increase under ideal conditions, which is particularly relevant in understanding ecosystems and managing conservation efforts.

The financial sector relies heavily on exponential form to calculate the growth of investments. Compound interest, a concept vital to savings and investment strategies, is calculated using an exponential formula. This ensures that the growth of an investment is proportional to the current amount, leading to the investment’s value compounding over time. In these formulas, the variables represent the principal amount, the rate of interest, the number of times the interest is compounded per year, and the time for which the investment is made. As a result, exponential form becomes a core part of retirement planning, as it helps predict how much one’s investments might be worth in the future.

In computer science, exponential form is seen in the quantification of data storage. The binary system, fundamental to all computing processes, scales in powers of two, with terms like kilobytes (2¹⁰ bytes), megabytes (2²⁰ bytes), and beyond describing exponentially larger amounts of data. The complexity of algorithms is also often described in exponential terms, particularly when analyzing their efficiency and scalability.

Demography and epidemiology are two fields where exponential equations are particularly useful. Population studies use them to estimate growth under specific conditions, which is crucial for urban planning and resource allocation. Similarly, the spread of infectious diseases can follow an exponential pattern, especially in the early stages of an outbreak. This understanding is essential for the formulation of public health policies and intervention strategies.

The world of physics offers yet more examples. The behavior of particles at the quantum level is often described using wave functions that are expressed in exponential form. These wave functions provide probabilities for the position of particles, a core principle of quantum mechanics. Furthermore, atmospheric pressure decreases exponentially with altitude, a relationship that is critical for understanding weather patterns and the environment.

In acoustics and the study of sound, our perception of pitch is logarithmic; hence musical scales and frequencies are calculated using powers, showcasing the exponential nature of sound perception. Musicians and sound engineers often manipulate these exponential relationships to create harmonies and effects that resonate with the human auditory system.

Exponential forms are foundational to our modern existence, touching on so many aspects of daily life. Whether it’s in the saving and accumulation of personal wealth, the functionality and operation of our digital devices, the understanding of natural processes, or even the enjoyment of music, exponential forms allow us to quantify, understand, and predict the behavior of systems both vast and microscopic. Their use in real-world applications underlines the profound importance of mathematical concepts and their far-reaching impact.