Exponent Multiplication Worksheets
About These 15 Worksheets
These worksheets will help students master the concept of exponentiation, which involves raising numbers to certain powers. These worksheets cover a range of exercises, each focusing on different aspects of working with exponents, especially their multiplication. Practicing with these worksheets not only solidifies a student’s understanding of the basic principles of exponents but also enhances their ability to manipulate and calculate powers in various mathematical contexts.
The core concept behind exponentiation is to repeat the multiplication of a number by itself a specified number of times. For example, 34 means multiplying 3 by itself 4 times (3 x 3 x 3 x 3), which equals 81. Exponents are a foundational concept in mathematics, underpinning more complex topics such as algebra, calculus, and beyond.
Through a variety of exercises, from basic multiplication to more complex applications, these worksheets equip students with the skills necessary to navigate both academic and real-world mathematical challenges. Regular practice not only bolsters students’ numerical and algebraic proficiency but also cultivates a deeper understanding of how exponentiation functions within a broader mathematical context.
Exponent multiplication worksheets typically encompass a variety of exercises designed to cater to different learning stages and abilities. These exercises include:
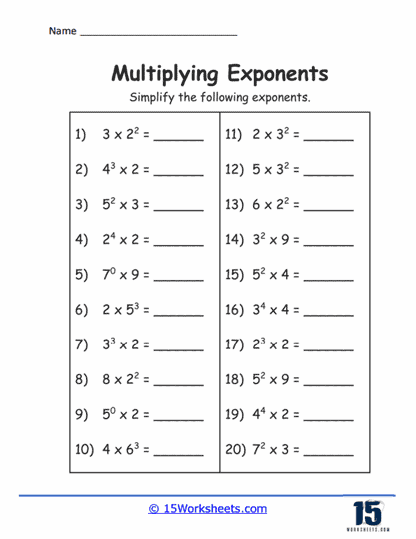
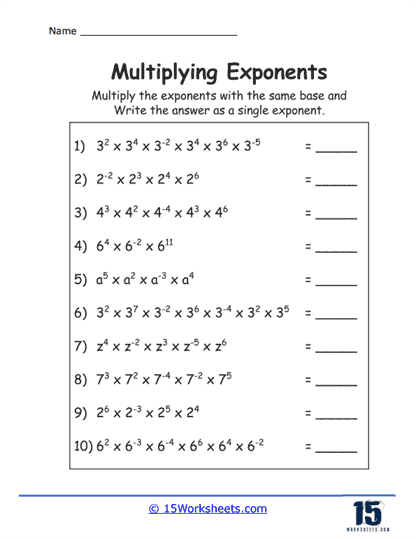
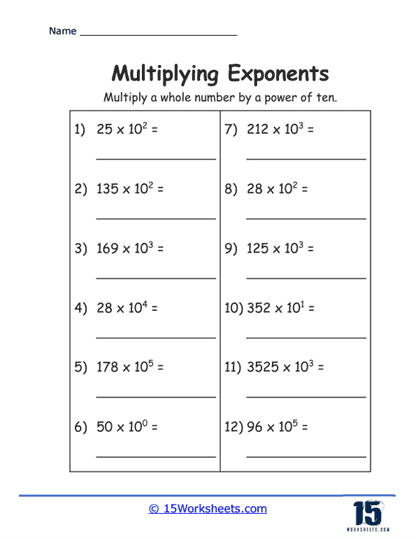
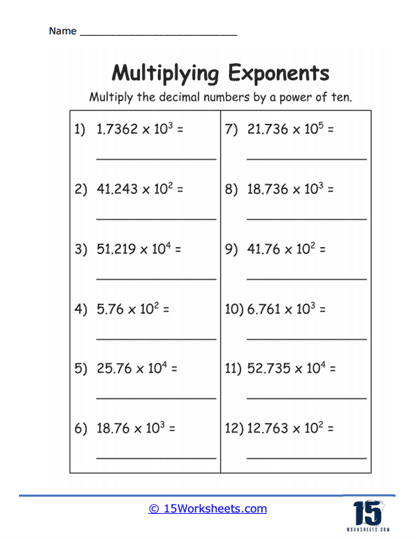
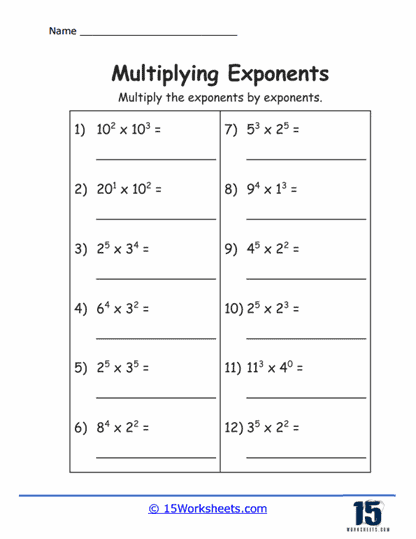
Basic Exponent Multiplication – This type of exercise introduces students to the fundamental concept of multiplying exponents with the same base. Students learn the rule that when multiplying exponents with the same base, you keep the base and add the exponents. For example, xa x xb = xa + b.
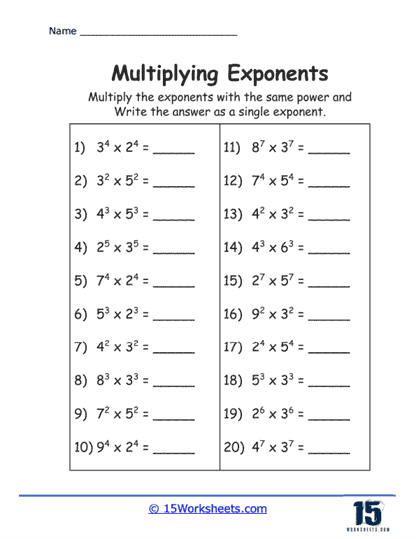
Multiplying Exponents with Different Bases – These exercises challenge students to multiply powers with different bases, requiring a solid understanding of multiplication tables and the distributive property of multiplication over exponentiation.
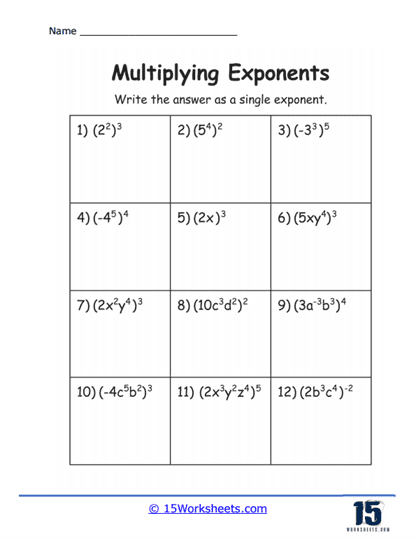
Power of a Power – Exercises that involve taking a power of a power (e.g., (xa)b) teach students to multiply the exponents. This is crucial for understanding how exponentiation scales when nested within itself.
Power of a Product – Students encounter problems where a product is raised to a power (e.g., (xy)a). They practice applying the exponent to each factor within the parentheses, which reinforces the distributive property of exponents over multiplication.
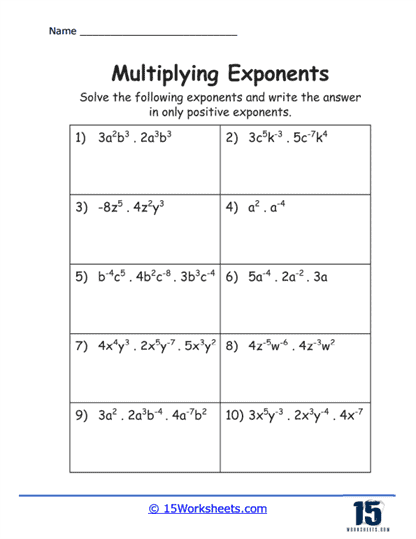
Negative and Zero Exponents – These exercises introduce the concepts of negative and zero exponents, showing students that a negative exponent indicates division (or reciprocal) and that any non-zero number raised to the power of zero equals one. For instance, x-a = 1/xa and x0 = 1.
Fractional Exponents – Exercises on fractional exponents help students grasp how these represent roots (e.g., x1/2 is the square root of x). They learn to apply rules of exponents even when the exponents are not whole numbers.
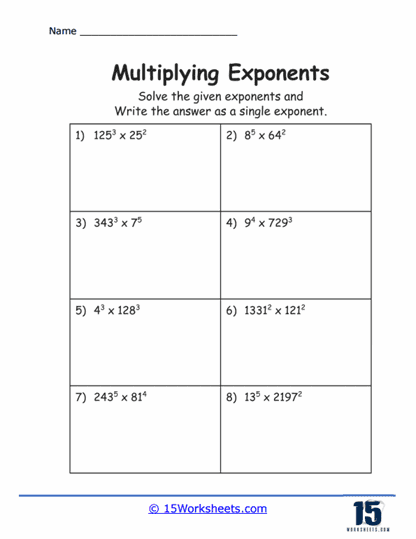
Mixed Operations – These more advanced exercises combine multiplication, division, and sometimes addition and subtraction of exponents. They are designed to reinforce all the learned rules and encourage students to strategize their approach to solving problems. Incorporating exponents into word problems helps students apply their mathematical knowledge to real-world scenarios. These problems often involve exponential growth or decay, requiring students to set up and solve equations that model situations like population growth, investment growth, or radioactive decay.
The Benefits of These Worksheets
Practicing with exponent multiplication worksheets significantly benefits students by:
Enhancing Numerical Fluency – Regular practice with exponents helps students become more fluent in performing calculations involving powers, an essential skill in many areas of mathematics and science.
Developing Strategic Thinking – As students work through various types of exponent exercises, they learn to recognize patterns and develop strategies for simplifying expressions, fostering critical thinking skills.
Building Algebraic Foundations – Mastery of exponents lays the groundwork for understanding more complex algebraic concepts, such as polynomials and logarithms. It is a stepping stone toward high school and college-level mathematics.
Improving Problem-Solving Skills – The diverse types of problems found in exponent multiplication worksheets teach students to approach mathematical challenges from different angles, enhancing their problem-solving abilities.
Preparing for Real-World Applications – Understanding exponents is crucial for fields that rely on mathematical modeling, such as physics, engineering, and finance. These worksheets prepare students for future studies and careers in these areas.
How Do You Multiply Values With Exponents?
Multiplying values with exponents, a fundamental operation in algebra, follows specific rules designed to simplify and solve expressions involving powers efficiently. Understanding these rules is essential for navigating more complex mathematical concepts. There are two primary scenarios to consider when multiplying exponents – when they have the same bases and when they have different bases.
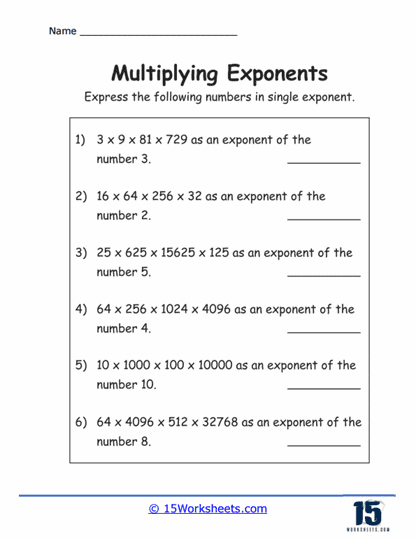
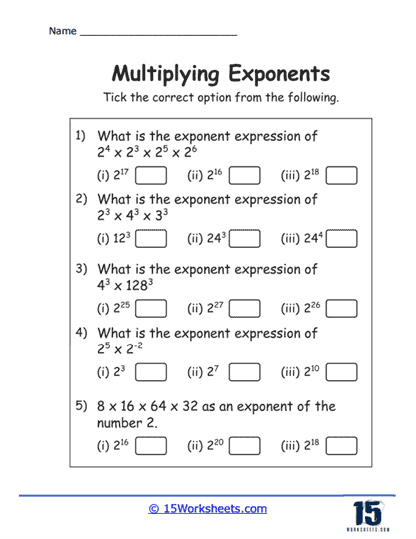
Multiplying Exponents with the Same Bases
When you multiply exponents with the same base, you keep the base and add the exponents. This rule stems from the definition of exponentiation, which involves repeated multiplication of the base by itself. The rule can be expressed algebraically as:
xa x xb = xa + b
Example
Let’s multiply 32 by 33.
Here, both terms have the same base (3), and their exponents are 2 and 3, respectively.
According to the rule, we add the exponents while keeping the base the same:
32 x 33 = 32 + 3 = 35
To check our work, we can calculate each side of the equation:
The left side is 32 x 33 = 9 x 27 = 243
The right side, 35, means multiplying 3 by itself 5 times, which is also 3 x 3 x 3 x 3 x 3 = 243.
Both sides equal 243, confirming the rule.
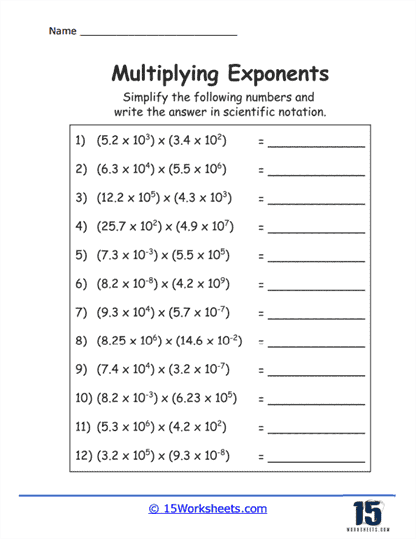
Multiplying Exponents with Different Bases
When multiplying exponents with different bases but the same exponent, you can multiply the bases first and then apply the exponent to the result. This scenario occurs less frequently in its pure form but is equally important. The rule for this situation can be represented as:
am x bm = (ab)m
However, when the bases and the exponents are different, there’s no simplified rule that combines them into one exponentiation operation. Instead, you would typically handle each exponentiation separately or apply other algebraic properties if the situation allows.
Example 1 (Same Exponent, Different Bases) – 23 x 53
Since the exponent is the same (3) for both terms, we multiply the bases (2 and 5) first and then apply the exponent:
23 x 53 = (2 x 5)3 = 103 = 1000
Example 2 (Different Bases and Exponents) – 23 x 52
Here, you have different bases (2 and 5) and different exponents (3 and 2), so you calculate each part separately:
23 = 8
52 = 25
Then, multiply the results – 8 x 25 = 200
In this case, since the bases and exponents are different, we don’t combine them into a single exponentiation expression but rather work through each exponentiation individually.
The rules for multiplying exponents streamline the process of working with powers and are foundational for higher-level math. By understanding and applying these rules, you can solve a wide range of problems more efficiently and accurately. Whether dealing with the same bases or different ones, these principles enable a deeper comprehension of the structure and behavior of exponential expressions.