Braces, Brackets, and Parentheses Worksheets
All About These 15 Worksheets
Braces, brackets, and parentheses are indispensable elements of mathematical notation, each with its unique role in organizing, structuring, and simplifying complex expressions, equations, and functions. Parentheses, for instance, are often used to denote priority in operations, ensuring clarity in the order in which calculations should be performed. Brackets can signify a secondary level of grouping or are often employed in matrix notation, while braces are frequently associated with sets, denoting collections of elements in algebra and beyond. These symbols not only provide clarity but also eliminate ambiguities that could lead to errors, making them fundamental tools in both simple arithmetic and advanced mathematical reasoning.
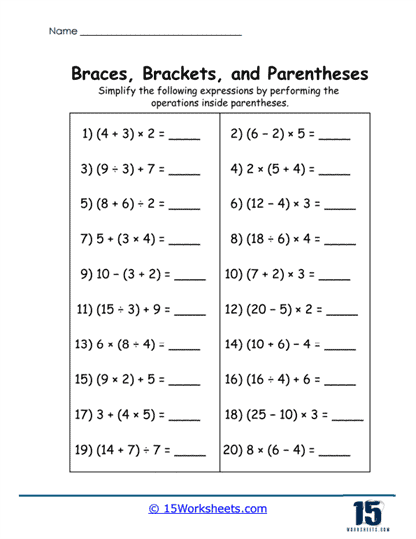
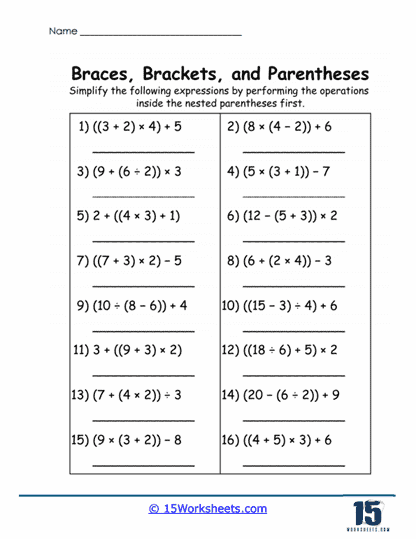
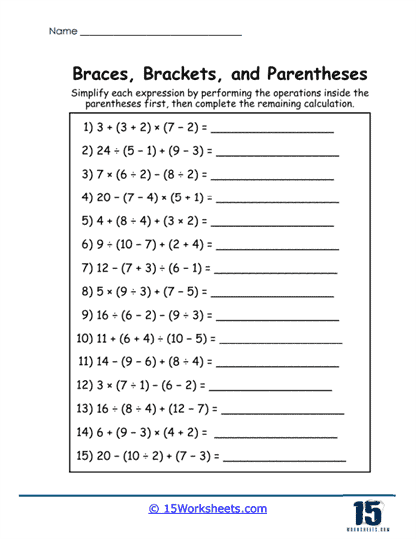
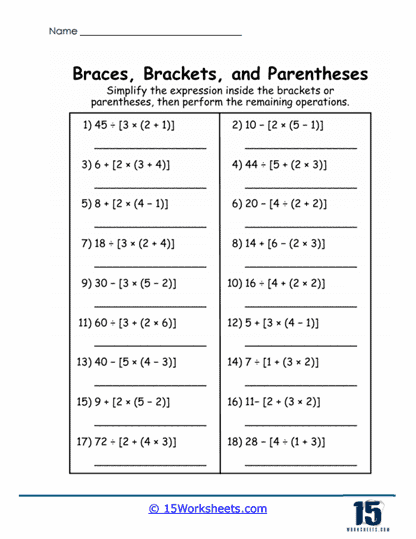
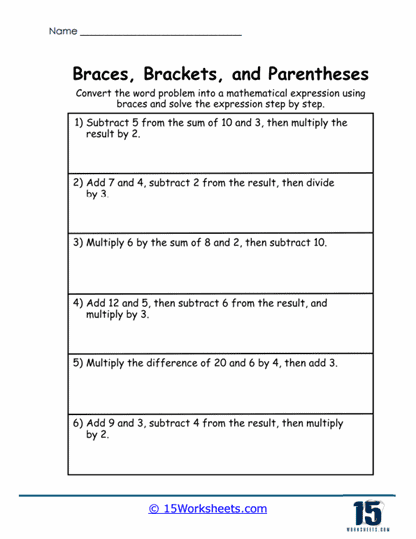
These worksheets are focused on the use of these symbols and shows students how to use them correctly. For younger students, these worksheets introduce basic concepts such as grouping numbers in addition or multiplication problems, helping them understand the importance of the order of operations. As students progress, these exercises become more complex, requiring them to manage nested symbols, such as parentheses within brackets or braces. At more advanced levels, worksheets may cover their use in algebraic functions, solving inequalities, or working with matrices and piecewise functions. By offering structured practice, these materials ensure that students gain both confidence and competence in using these notational tools.
The importance of mastering these symbols extends beyond individual exercises, as they are foundational to mathematical communication and problem-solving. By engaging with worksheets that systematically build their skills, students learn to interpret and construct mathematical statements with precision. This ability is crucial not only for success in mathematics but also for applications in science, engineering, and computer programming, where such notation is frequently employed. In essence, these worksheets bridge the gap between theoretical understanding and practical application, equipping students with the skills necessary for tackling increasingly sophisticated mathematical challenges.
The Purpose of Braces, Brackets, and Parentheses
In mathematics, braces {}, brackets [], and parentheses () are collectively referred to as grouping symbols, playing a critical role in structuring mathematical expressions and ensuring clarity in calculations. These symbols help define the order of operations, thereby preventing ambiguity and errors in computation. By organizing terms, they guide mathematicians and students alike in interpreting complex expressions and prioritizing operations appropriately. Their utility spans various fields of mathematics, from basic arithmetic to advanced calculus and algebra, making them indispensable tools for learners and practitioners.
Grouping symbols not only facilitate understanding but also serve as a foundation for effective problem-solving. By mastering their use, students develop essential skills for approaching equations and functions with precision and confidence. Each symbol has a distinct role, yet their interplay is often crucial, especially in nested or layered expressions. Understanding the purpose and function of these symbols is a fundamental step in building mathematical literacy and problem-solving capabilities.
Parentheses ()
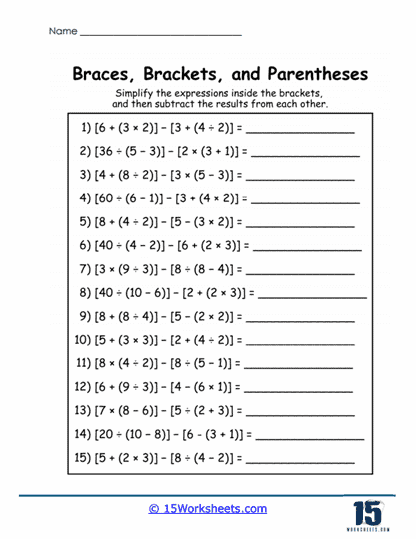
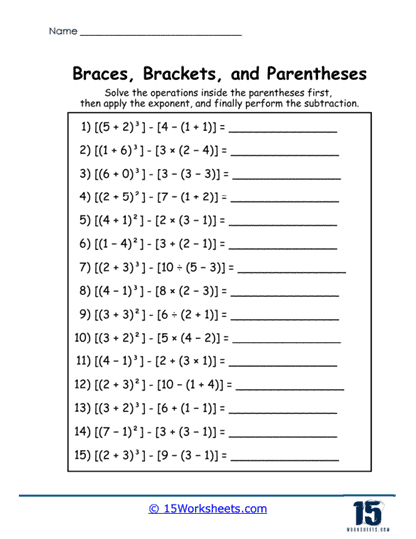
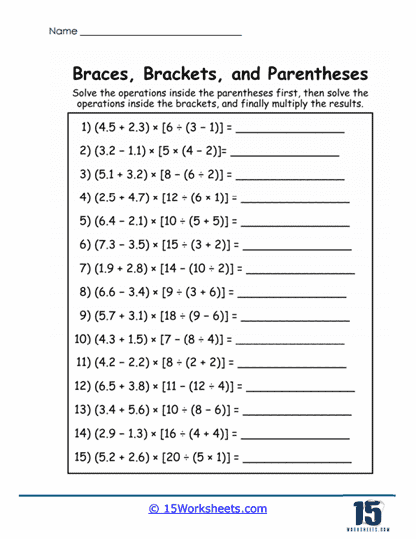
Parentheses are the most frequently used grouping symbols in mathematics and are foundational in defining the order of operations. According to the PEMDAS/BODMAS rule, operations inside parentheses take precedence over others, ensuring clarity and consistency in calculations. For instance, in the expression 2 x (3 + 4), the parentheses dictate that 3 + 4 must be calculated first, resulting in 2 x 7, or 14. This prioritization eliminates ambiguity and ensures accuracy, particularly in multi-step problems.
Parentheses are also widely used in algebra, where they help group terms in equations and functions. For example, in simplifying expressions like 3(x + 2), parentheses emphasize that the distributive property should be applied, yielding 3x + 6. Beyond arithmetic and algebra, parentheses are pivotal in higher mathematics, such as calculus, where they are employed to denote intervals (e.g., (a, b) for open intervals) or functions (e.g., f(x)). Their consistent and versatile application makes them a cornerstone of mathematical notation.
Brackets []
Brackets provide an additional layer of grouping and are commonly used within parentheses to handle nested operations. They help organize expressions with multiple levels of complexity, ensuring that calculations are executed in the correct sequence. For example, in 2 x [(3 + 4) – 5], the brackets indicate that the subtraction [(3 + 4) – 5] should occur after evaluating the parentheses but before multiplying by 2. Without such structure, interpreting the expression could lead to errors or confusion.
In more advanced contexts, brackets are essential for matrix operations, where they encapsulate rows and columns of numbers. For instance, a matrix such as [1 2; 3 4] uses brackets to define its boundaries and distinguish it from scalar values or other expressions. In algebra and calculus, brackets are also used to denote closed intervals, such as [a, b], where both endpoints are included. This multifunctional utility underscores the importance of brackets in both elementary and sophisticated mathematical problems.
Braces {}
Braces are less commonly encountered in basic arithmetic but are crucial in specific mathematical contexts, particularly in representing sets and organizing higher-order groupings. In set theory, braces are used to denote collections of distinct elements, such as {1, 2, 3}, which represents a set containing the numbers 1, 2, and 3. This application is fundamental in areas like probability, statistics, and discrete mathematics, where sets form the basis for further analysis.
In addition to sets, braces play a role in advanced calculations that require multiple levels of grouping. For instance, in {[3 x (2 + 1)] + 4}, the braces organize the entire expression, encompassing both brackets and parentheses to clarify the intended order of operations. Braces are also encountered in programming and computer science, where they are used in defining blocks of code or specifying function domains. Despite their less frequent use in basic mathematics, braces are indispensable in higher-level problem-solving and notation.
Importance of Grouping Symbols in Mathematics
Grouping symbols, such as braces, brackets, and parentheses, are fundamental tools in mathematics. They not only aid in solving complex problems but also serve as a cornerstone for understanding higher-level math concepts. Worksheets dedicated to these symbols provide invaluable benefits, fostering skills that students will carry into various academic and professional disciplines.
Promoting Logical Thinking
The use of grouping symbols inherently teaches students to approach mathematical problems methodically. These symbols guide learners to follow the correct order of operations, ensuring that calculations are carried out systematically. This process cultivates logical thinking as students must carefully analyze and sequence each step to arrive at the correct solution. Worksheets focusing on grouping symbols reinforce this structured approach, enabling students to break down problems into manageable parts and enhancing their problem-solving capabilities.
Preventing Ambiguity
One of the key roles of grouping symbols is to eliminate ambiguity in mathematical expressions. By explicitly indicating which operations should be performed first, these symbols provide clarity and precision. For instance, they help students avoid common mistakes, such as performing multiplication before resolving terms inside parentheses. Worksheets dedicated to this topic give students ample practice in interpreting and applying these conventions, reducing errors and boosting their confidence in handling complex expressions.
Building a Strong Foundation
The ability to correctly use grouping symbols is a foundational skill in mathematics that students rely on throughout their education. Whether solving algebraic equations or working with functions, the proper application of braces, brackets, and parentheses is essential. Introducing these concepts through worksheets at an early stage builds a strong base for future learning. By mastering these basics, students gain the confidence to tackle more intricate problems and develop resilience when faced with challenging mathematical tasks.
Real-World Applications
Grouping symbols are not confined to academic exercises; they have practical applications in various real-world contexts. Fields such as science, engineering, finance, and computer programming frequently employ these symbols to organize and prioritize operations. Worksheets that emphasize grouping symbols help students understand their relevance beyond the classroom, illustrating how they are used to solve real-life problems. This connection to practical applications motivates students and provides a broader perspective on the importance of mathematical accuracy and structure.