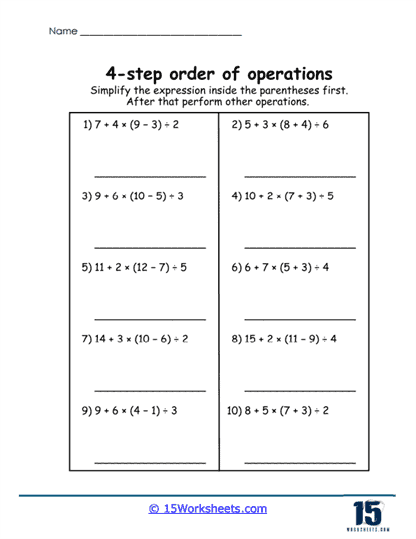
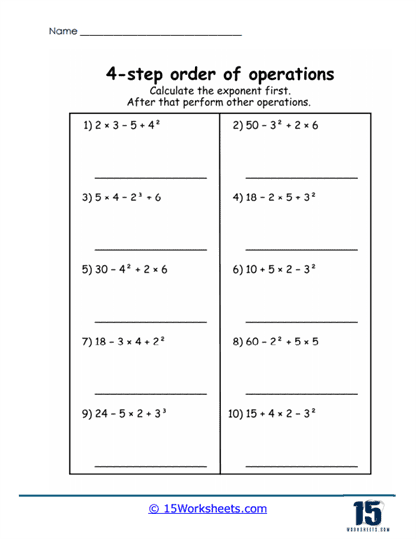
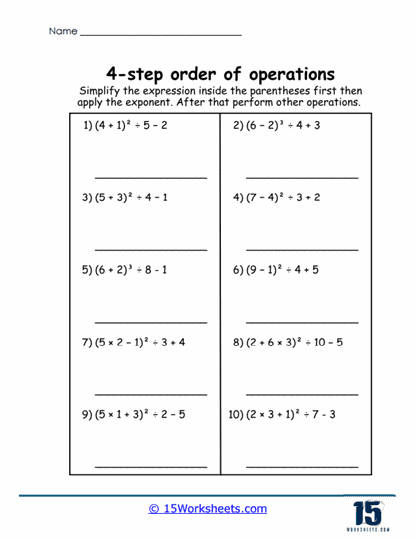
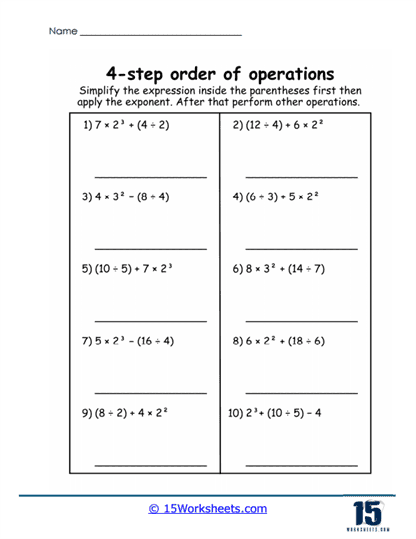
Four Step Order of Operations Worksheets
All About These 15 Worksheets
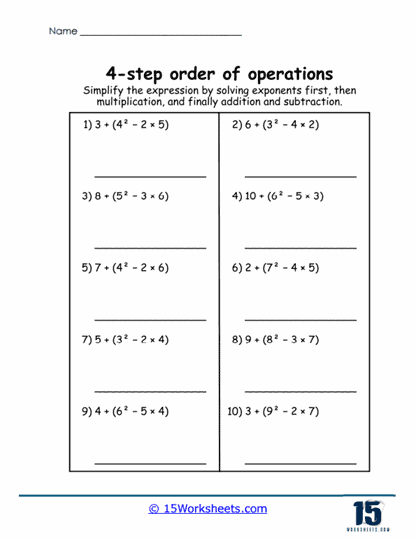
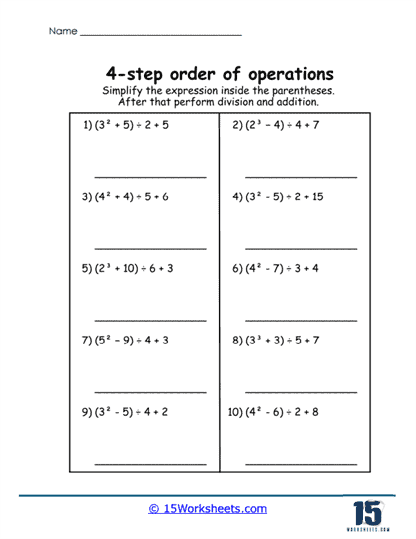
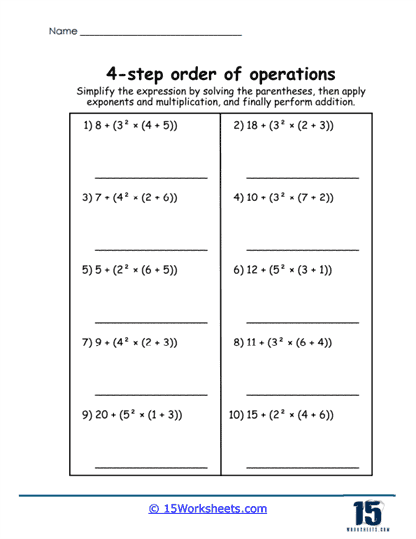
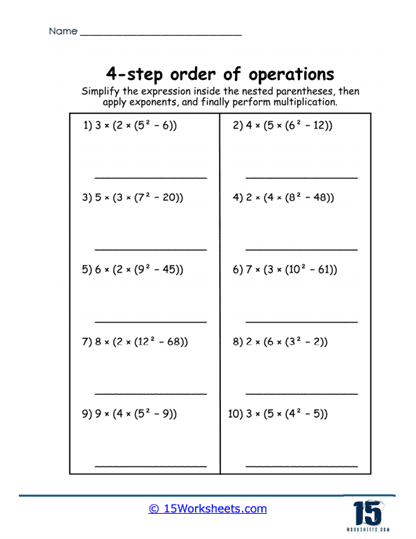
These worksheets are designed to teach and reinforce the foundational principles of the order of operations in mathematics. These worksheets are structured to help students systematically approach multi-step problems involving various mathematical operations. By following a prescribed sequence of operations, students learn to solve complex expressions accurately and consistently.
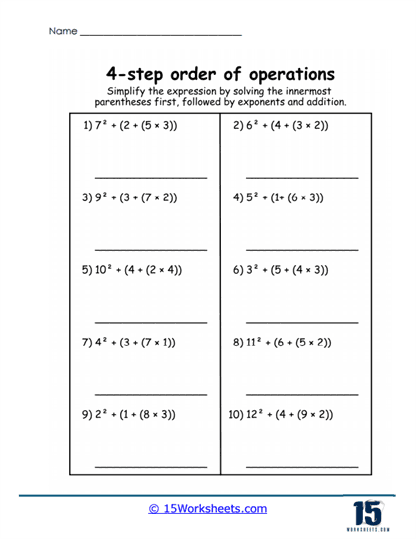
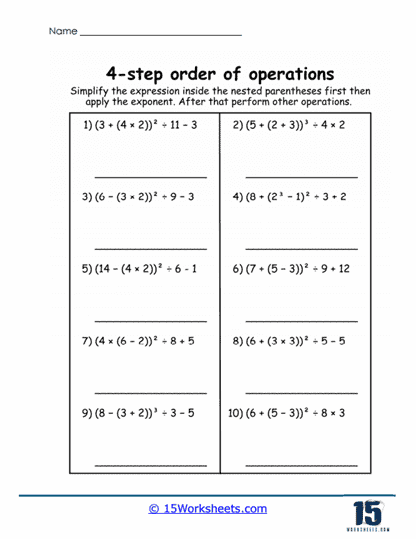
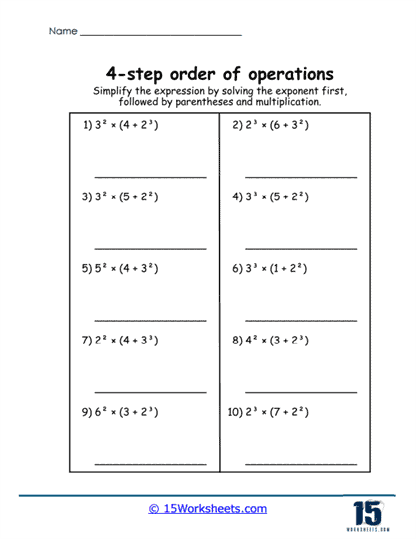
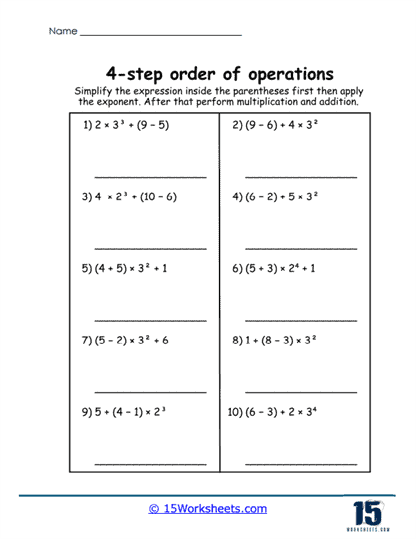
These worksheets emphasize the application of the PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) hierarchy, guiding students to follow the correct sequence when performing operations. Each problem is structured to incorporate different combinations of these operations, ensuring a comprehensive understanding of the order of operations. This focused approach not only reinforces the importance of following a systematic method but also helps students build confidence in tackling multi-step problems. By progressively increasing in difficulty, these worksheets cater to students at various skill levels, making them a versatile resource in math education.
Example Problem
A typical problem from a Four Step Order of Operations worksheet might look like this:
6 + (3 x 22) – 8 ÷ 2
To solve this, students would follow these steps:
1. Solve the exponent: 22 = 4
The first step is to identify and simplify the exponent in the problem. In this case, 22 (two squared) is equal to 4. This step reinforces the concept of exponents and ensures students address this higher-order operation before moving on to simpler ones.
2. Perform multiplication inside the parentheses: 3 x 4 = 12.
After simplifying the exponent, the focus shifts to the multiplication within the parentheses. Students multiply 3 by 4 to get 12. This demonstrates the importance of resolving operations inside parentheses completely before dealing with the rest of the expression.
3. Perform division: 8 ÷ 2 = 4.
Once the parentheses are resolved, students handle any division in the expression. Here, 8 divided by 2 equals 4. This step highlights the rule that multiplication and division should be performed from left to right as they appear in the expression.
4. Perform addition and subtraction from left to right: 6 + 12 – 4 = 14.
Finally, students perform addition and subtraction in the order they appear. Adding 6 and 12 results in 18, and subtracting 4 from 18 gives a final answer of 14. This step emphasizes the importance of working sequentially and ensures students don’t skip or reorder steps.
Why These Worksheets Are Important
By tackling problems like the one above, students gain a thorough understanding of how to approach multi-step mathematical expressions. They learn to recognize which operations take priority and how to resolve each step methodically. This procedural clarity not only helps in solving problems accurately but also builds a strong foundation for tackling more complex algebraic expressions in the future. Furthermore, the progressive difficulty of the worksheets ensures that students are continually challenged, encouraging growth and deeper comprehension over time.
1. Building Confidence in Math
Successfully solving four-step order of operations problems instills a sense of accomplishment, which is critical for building a student’s confidence in math. Each solved problem provides tangible evidence of their growing skills, which encourages them to tackle increasingly challenging questions. This confidence often has a ripple effect, motivating students to engage more actively in math lessons and other STEM-related activities. As they master the systematic process required by these worksheets, they feel a sense of control over their learning, reducing anxiety around math. Furthermore, consistent success reinforces a positive attitude toward mathematics, fostering resilience and a willingness to persevere even when faced with complex problems.
2. Preparing for Advanced Mathematics
The order of operations forms the foundation for nearly every branch of advanced mathematics. Without a strong grasp of these rules, students struggle to understand algebra, calculus, and beyond, where expressions become more complex and nuanced. Four-step worksheets help students practice breaking down problems systematically, a skill essential for solving equations, working with functions, and performing matrix operations in advanced courses. These worksheets also introduce students to concepts like exponents and grouping, which are critical for understanding polynomial expressions and graphing. Additionally, this preparation ensures students are better equipped to succeed on standardized tests, where mastery of the order of operations is often assumed.
3. Encouraging Discipline and Methodical Thinking
Four-step worksheets require students to approach problems in a disciplined and methodical way, fostering habits that extend far beyond mathematics. The structured nature of these exercises teaches students to think sequentially, ensuring they address each step of a problem before moving on to the next. This approach minimizes errors and instills a sense of order, which is invaluable in fields like engineering, coding, and scientific research. Moreover, this discipline helps students develop patience and attention to detail, qualities essential for long-term academic and professional success. By consistently working through these problems, students learn to trust the process of problem-solving, which builds their ability to handle even more complex tasks in the future.
4. Standardizing Mathematical Understanding
Adhering to the universal conventions of PEMDAS ensures that students’ solutions align with global mathematical standards. This consistency is vital for clear communication, especially in collaborative environments where multiple people may need to interpret the same expression. Standardization eliminates ambiguity, enabling students to present their work confidently and accurately in both academic and real-world settings. Moreover, understanding and applying these conventions prepares students for careers in fields like data analysis, finance, and technology, where precision in mathematical communication is critical. By mastering these worksheets, students internalize a shared language of mathematics, making it easier to transition into higher education and professional disciplines.
Types of Problems
These worksheets vary in complexity and are tailored to different grade levels and student proficiencies. Below are some examples of the types of problems typically included:
1. Basic Arithmetic Problems
Example: 4 + 3 x 5 – 6 ÷ 2
Focus: Reinforcing the sequence of basic operations without parentheses or exponents.
2. Problems with Parentheses
Example: (7 – 2) x (3 + 4) ÷ 5
Focus: Teaching students to prioritize parentheses before moving to other operations.
3. Problems with Exponents
Example: 32 + (6 ÷ 2) – 4
Focus: Introducing powers and roots into the sequence.
4. Mixed Problems with Decimals and Fractions
Example: (1.5 + 0.5) x (3/4 ÷ 1/2)
Focus: Challenging students to apply the order of operations with fractions and decimals.
5. Multi-Level Problems
Example: [(8 ÷ 4) + 23] x (7 – 5)
Focus: Combining multiple operations, parentheses, and exponents to create more challenging problems.