Order of Operations Worksheets
About These 15 Worksheets
These worksheets will teach, reinforce, and assess students’ understanding of the sequence in which mathematical operations should be performed. These worksheets are typically used in classrooms, homeschool settings, or for independent practice to ensure students develop a solid grasp of this fundamental mathematical concept. Understanding the order of operations is crucial for solving complex mathematical problems accurately and consistently.
These worksheets are meticulously designed to help students grasp the rules encapsulated in the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), which dictates the order of solving mathematical expressions. By providing structured practice opportunities, these worksheets not only introduce the concept but also reinforce it through repetitive problem-solving, allowing students to internalize the process and apply it with confidence across various mathematical scenarios.
Order of Operations worksheets are instrumental in bridging the gap between theoretical understanding and practical application. Teachers often use them as a supplement to lectures, enabling students to apply the PEMDAS rule to progressively challenging problems. In homeschool settings, these worksheets provide a systematic way for parents to guide their children through a structured learning process. For independent learners, they serve as a self-assessment tool, enabling them to identify areas of strength and those requiring further focus. By working through diverse examples, students develop not only accuracy in computation but also a deeper understanding of mathematical principles, preparing them for more advanced problem-solving.
Mathematics often involves multiple operations within a single expression, such as addition, subtraction, multiplication, division, and grouping symbols (e.g., parentheses). The order in which these operations are performed affects the outcome. For example:
2 + 3 x 4 is ambiguous unless a standardized order is followed.
Using the correct order of operations, the answer is 2 + (3 x 4) = 2 + 12 = 14.
If performed left-to-right without following proper rules, the result would be incorrect: (2 + 3) x 4 = 5 x 4 = 20.
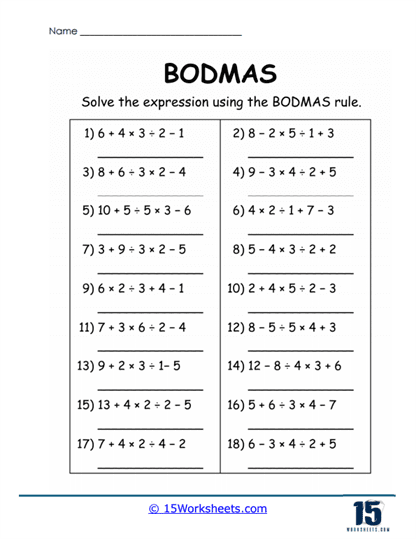
The established rules for the order of operations are commonly remembered using the acronym PEMDAS, which stands for:
Parentheses – Solve expressions within parentheses or other grouping symbols first.
Exponents – Next, evaluate exponents (e.g., powers and roots).
Multiplication and Division – Perform these operations from left to right.
Addition and Subtraction – Finally, perform these operations from left to right.
Challenges and Common Mistakes
The order of operations is a foundational concept in mathematics, but it presents numerous challenges for students. These difficulties stem from a variety of factors, including cognitive overload, lack of foundational understanding, and simple carelessness. Mastering this concept requires consistent practice and structured guidance, which worksheets are uniquely equipped to provide. Let’s explore the common challenges students face and how worksheets can effectively address these issues.
Forgetting or Misapplying the Rules
One of the most common challenges students face is forgetting the order of operations rules, often abbreviated as PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction). When confronted with problems involving multiple operations, students may either forget the hierarchy or apply it inconsistently. For instance, some might mistakenly prioritize addition over multiplication simply because it appears first in the problem. This confusion is exacerbated when students encounter more complex problems involving nested operations. Worksheets play a critical role here, offering repeated practice that reinforces the proper order. By presenting problems of increasing difficulty, worksheets help students internalize the rules and apply them accurately over time.
Misinterpreting Grouping Symbols
Grouping symbols such as parentheses, brackets, and braces often trip up students, particularly when these symbols are nested. Many students struggle to understand how to approach problems where operations are embedded within layers of grouping symbols. Without a clear understanding, they may either skip operations or misinterpret the boundaries of these symbols. Worksheets are invaluable for clarifying these concepts. Well-designed worksheets include problems with detailed examples and step-by-step solutions, demonstrating how to correctly interpret and solve problems with grouping symbols. Additionally, these worksheets often highlight common pitfalls, enabling students to anticipate and avoid errors.
Overlooking Exponents
Exponents introduce another layer of complexity to the order of operations, especially for beginners. Students often overlook exponents entirely or attempt to resolve them out of sequence, disrupting the hierarchy of operations. This mistake frequently occurs because exponents are less familiar than basic addition or multiplication. Worksheets address this issue by dedicating specific sections to exponent rules and their application. These targeted exercises help students build confidence and competence with exponents before incorporating them into more comprehensive order of operations problems. With consistent practice, students develop a stronger grasp of this crucial component.
Rushing Through Problems
A tendency to rush through problems is another pervasive challenge, particularly among students who feel pressured to complete assignments quickly. This haste often leads to careless mistakes, such as performing operations in the wrong order or skipping steps entirely. Rushing can also result in misreading the problem, leading to fundamentally incorrect answers. Worksheets help combat this tendency by encouraging a deliberate approach to problem-solving. Timed worksheets, for example, create a structured environment where students must balance speed with accuracy. Additionally, worksheets that break problems into smaller, more manageable parts teach students to work methodically and carefully.
A Look At The Different Category of Worksheets
These activities involve solving problems with two operations, such as addition and multiplication, emphasizing the importance of following a specific sequence. They keep tasks simple and engaging, making them perfect for developing foundational problem-solving skills. Students build confidence while mastering basic concepts.
Three Step Order of Operations
With three operations in each problem, these exercises encourage learners to handle more complexity while reinforcing logical thinking. They bridge the gap between simple and advanced equations, promoting a deeper understanding of the order of operations. These tasks sharpen problem-solving abilities and enhance precision.
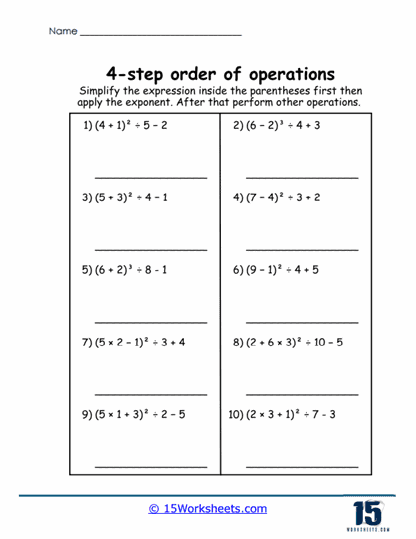
By incorporating four different operations in each problem, these challenges help students tackle multi-step equations with confidence. They encourage a systematic approach and logical reasoning to solve problems effectively. This format prepares learners for advanced math concepts and applications.
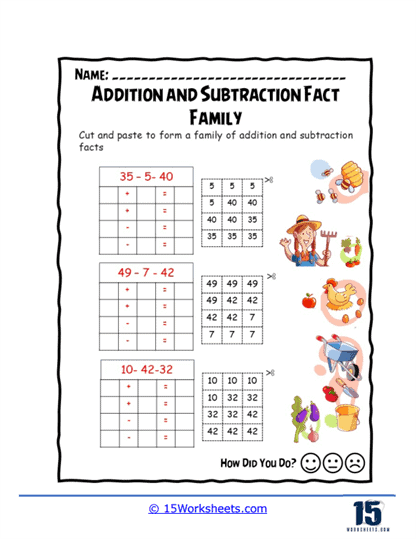
Add and Subtract Fact Families
These activities use sets of numbers to explore the relationship between two basic arithmetic operations. They emphasize how addition and subtraction connect, building a strong foundation for computational fluency. Learners gain a better understanding of number patterns through hands-on practice.
Focused on the hierarchy of mathematical operations, these tasks teach learners how to approach problems with multiple operations systematically. They ensure students grasp the importance of brackets and orders while solving equations accurately. This format prepares learners for more complex calculations.
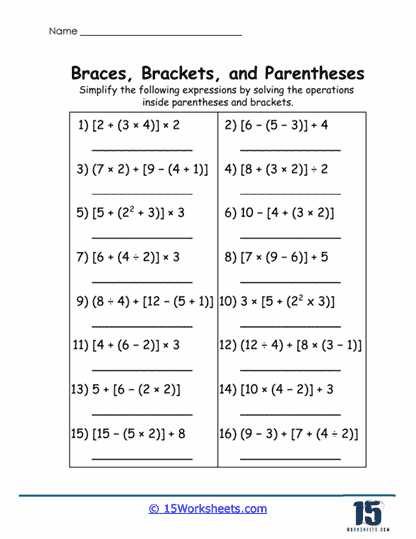
Braces, Brackets, and Parentheses
These exercises require students to navigate equations with nested grouping symbols, promoting precision and logical sequencing. By prioritizing operations correctly, learners strengthen their problem-solving skills. These tasks are ideal for advancing toward algebra and higher-level math.
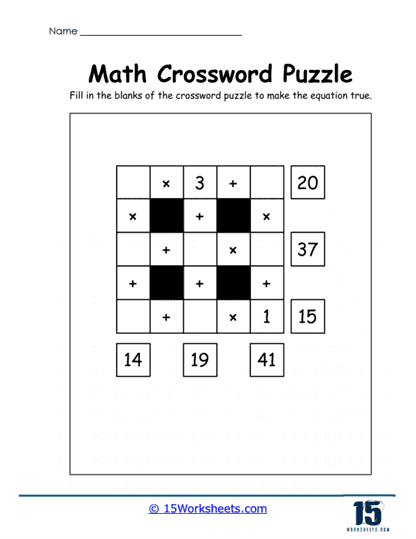
Combining problem-solving with a fun format, these puzzles challenge learners to fill in grids by solving math problems. They engage students while reinforcing skills in a creative and interactive way. This format is perfect for combining learning with entertainment.
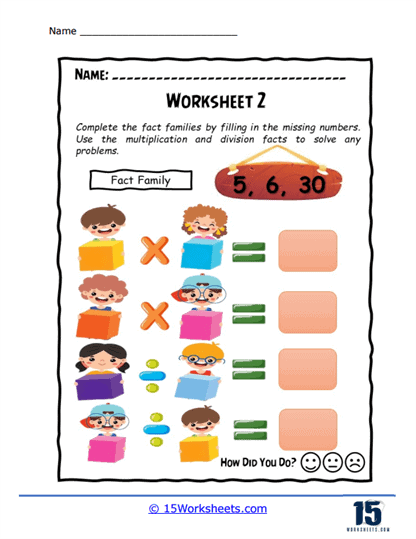
Multiplication and Division Fact Families
These tasks highlight the connections between multiplication and division, simplifying the learning process by emphasizing patterns. They help students see relationships within sets of numbers, fostering a deeper understanding of arithmetic operations. This approach builds essential math fluency.
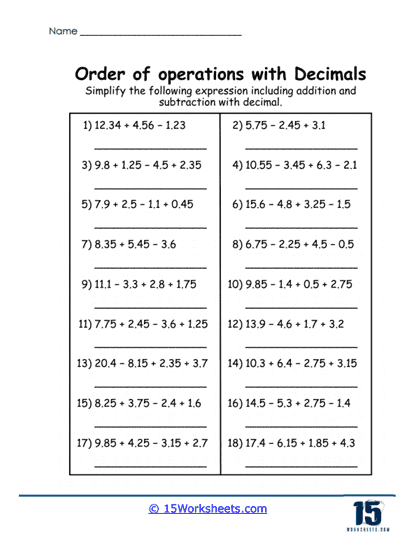
Order of Operations with Decimals
These problems incorporate decimals into multi-step equations, encouraging learners to apply the correct sequence while handling precise calculations. They build confidence in working with decimals and applying them to real-world contexts. Students strengthen both computation skills and practical problem-solving.
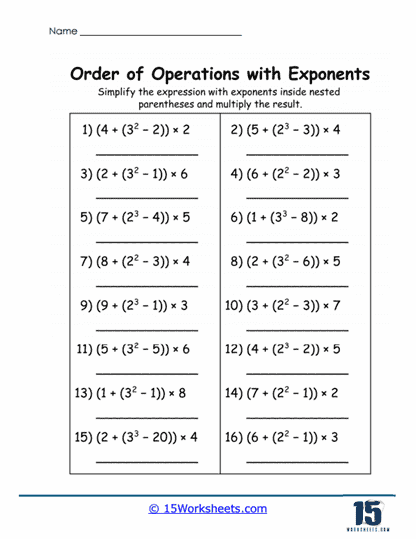
Order of Operations with Exponents
These challenges incorporate powers alongside other operations, teaching learners how to approach calculations with exponents carefully. They emphasize attention to detail and prepare students for advanced mathematical topics. This approach builds a strong foundation for algebraic thinking.
Order of Operations with Fractions
These problems combine fraction arithmetic with multi-step sequences, helping students practice accurate computation while following specific rules. They develop proficiency in handling fractions within complex equations. These activities support the growth of both conceptual understanding and arithmetic skills.
These exercises focus on the structured approach to solving equations by following the correct sequence of operations. They clarify the use of parentheses, exponents, and other operations, ensuring students avoid common errors. This method lays the groundwork for logical reasoning and problem-solving efficiency.