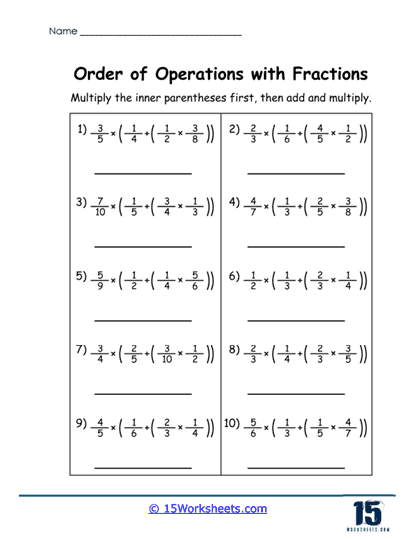
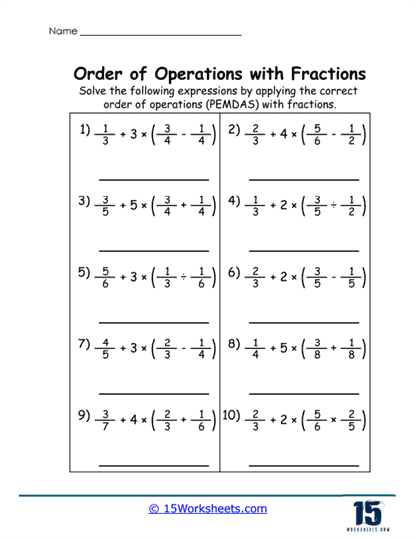
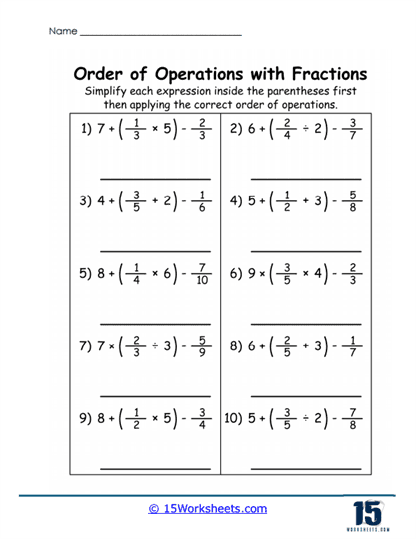
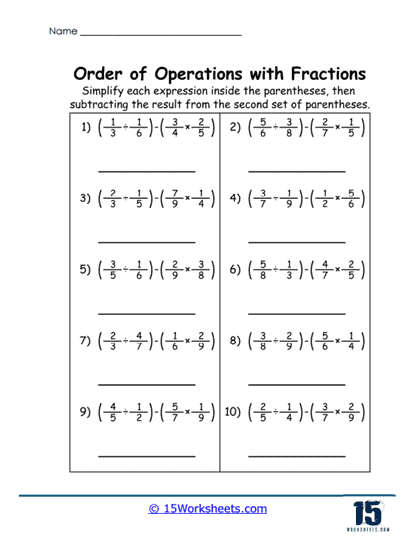
Order of Operations with Fractions Worksheets
All About These 15 Worksheets
They are crafted to challenge and deepen students’ understanding of the rules governing arithmetic operations and the hierarchy in which these operations must be performed. At their core, these worksheets emphasize the importance of the PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) rule while layering in the complexities of fractions. Fractions, unlike whole numbers, require specific operations that depend on common denominators, reciprocal relationships, and other nuances that demand a higher level of analytical thinking. As such, these worksheets not only teach mathematical procedures but also encourage students to think critically about how numbers and operations interact.
The significance of these worksheets lies in their ability to prepare students for more advanced mathematical concepts. Mastering the order of operations is foundational for algebra, geometry, and beyond. Introducing fractions into the mix amplifies this preparation, as fractions are pervasive in various branches of mathematics and real-life applications, such as measuring, budgeting, and data interpretation. Furthermore, these worksheets help students develop precision and attention to detail. A small misstep, such as performing addition before multiplication or incorrectly simplifying fractions, can lead to incorrect results. By repeatedly practicing with these worksheets, students build the habit of approaching problems systematically, an invaluable skill in both academic and real-world problem-solving scenarios.
However, fractions often become a stumbling block for students, leading to confusion and frustration. Many learners struggle to remember and apply the specific rules for operations with fractions, such as finding a common denominator for addition and subtraction or multiplying by the reciprocal when dividing. This challenge is compounded when fractions are nested within multi-step problems requiring adherence to the order of operations. For example, students might incorrectly simplify a fraction too early in the process or mismanage the placement of parentheses. These difficulties highlight the importance of a strong foundational understanding of both fractions and the order of operations before attempting more complex problems.
To overcome these challenges, educators can employ various strategies to make these concepts more accessible. Visual aids, such as fraction bars and number lines, can help students conceptualize the relationships between fractions and whole numbers. Step-by-step examples that break problems into manageable chunks can clarify how to approach complex worksheets systematically. Additionally, incorporating real-world scenarios, like cooking recipes or dividing objects, can make fractions and the order of operations more relatable and engaging. Regular practice combined with immediate feedback also ensures that students learn from their mistakes and refine their problem-solving techniques. Ultimately, order of operations worksheets with fractions are more than just exercises—they are valuable tools for fostering mathematical confidence, precision, and critical thinking.
Why Are These Worksheets Important?
These worksheets serve several purposes in mathematical education, making them a critical tool for developing foundational skills and preparing students for advanced mathematical challenges.
Reinforcing the Order of Operations
The worksheets help students internalize the PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) rule, which is a cornerstone of arithmetic and algebra. By practicing with these worksheets, students learn to approach equations methodically, avoiding common errors caused by neglecting the proper sequence. This skill is especially crucial as mathematical problems become more complex in higher grades. It not only enhances computational accuracy but also builds confidence in handling multi-step equations. Furthermore, consistent practice with order of operations fosters a deeper understanding of how mathematical expressions are structured and evaluated.
Developing Fraction Fluency
Including fractions in the worksheets ensures that students get ample practice with fundamental arithmetic operations such as addition, subtraction, multiplication, and division. These exercises expose learners to realistic scenarios, helping them see the practical importance of fractions in everyday life and advanced math. Regular engagement with fraction problems strengthens numerical sense and reinforces the relationships between whole numbers and parts of a whole. This fluency is essential for solving problems in a variety of subjects, including science, engineering, and finance. Ultimately, fraction practice helps students overcome one of the most common hurdles in mathematics by making complex calculations more approachable.
Problem-Solving and Logical Thinking
The complexity of combining fractions with order-of-operations rules challenges students to engage in critical thinking and logical reasoning. They must analyze problems step by step, ensuring each operation follows the correct sequence while maintaining accuracy with fractions. This process sharpens their ability to solve multi-layered problems, an essential skill in academics and real-world scenarios. These worksheets encourage perseverance as students work through challenging tasks, which in turn fosters resilience and adaptive thinking. Over time, students develop a systematic approach to problem-solving, which is transferable to other areas of learning and decision-making.
Preparation for Advanced Topics
Mastery of these worksheets lays a strong foundation for success in algebra, calculus, and other advanced mathematical subjects. Topics such as rational expressions, differential equations, and real-world applications often involve fractional quantities and require a solid grasp of the order of operations. By excelling in these preparatory exercises, students are better equipped to tackle more abstract and theoretical concepts in higher education. Furthermore, these skills are invaluable for real-life problem-solving, where fractions and order-of-operations thinking frequently arise, such as in cooking, budgeting, and data analysis. Preparing with these worksheets ensures students not only meet academic expectations but also build confidence in applying math in practical settings.
Common Areas of Confusion
Although the concept of order of operations is straightforward, the introduction of fractions adds layers of complexity that can confuse students. Let us explore these areas of confusion in detail.
Misunderstanding PEMDAS with Fractions
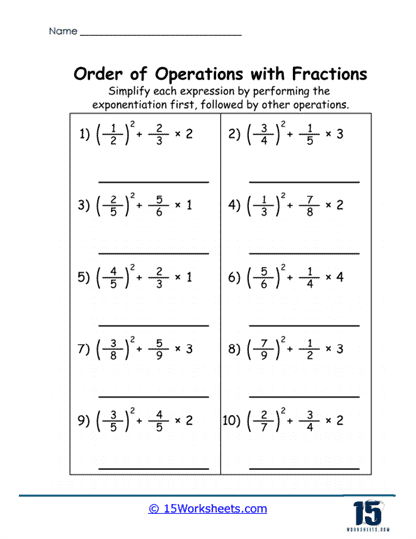
Students often find it difficult to correctly apply the PEMDAS rule (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) when fractions are involved. They may treat operations as sequential from left to right, ignoring the importance of parentheses or exponents in guiding the order of calculations. Furthermore, they might misinterpret the relationship between multiplication and division versus addition and subtraction, leading to improper prioritization of operations. This confusion becomes more pronounced when fractions make expressions appear more complicated, even if the same PEMDAS principles apply. Overcoming this challenge requires students to slow down, carefully identify each operation, and follow the hierarchy with precision, ensuring no steps are skipped.
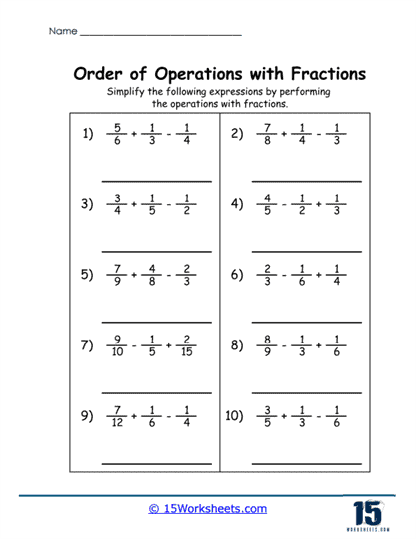
Struggling with Fraction Operations
Many students struggle with the basic arithmetic of fractions, which compounds their difficulties in solving expressions that involve them. For example, they may forget to find a common denominator when adding or subtracting fractions, leading to incorrect results. When multiplying fractions, some students incorrectly multiply only the numerators or only the denominators, failing to account for both parts. Division introduces its own challenge, as students may forget the reciprocal rule or apply it incorrectly. Addressing these gaps requires focused practice on fraction operations, ensuring that students develop fluency and confidence before tackling more complex expressions.
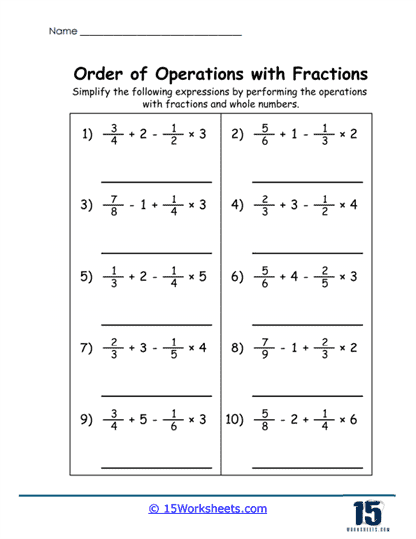
Difficulty with Mixed Numbers
Mixed numbers add another layer of difficulty, as students often mishandle their conversion to improper fractions. Instead of converting, some students attempt to perform operations directly on the whole number and fractional parts separately, which can lead to inconsistent results. For example, multiplying the whole number portion of a mixed number separately from its fractional portion creates errors that accumulate through the calculation. Additionally, students may forget to reconvert improper fractions back to mixed numbers in their final answers, leaving their work incomplete or incorrect. Teaching students to approach mixed numbers systematically, starting with conversion to improper fractions, helps to streamline the process and reduce errors.
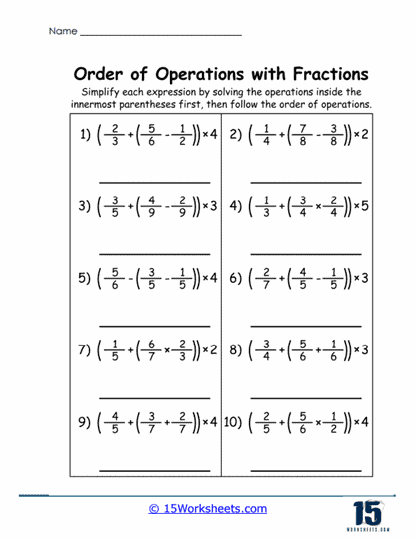
Parentheses and Nested Operations
Expressions with nested parentheses challenge students’ ability to prioritize operations correctly within layers of grouping symbols. The multiple steps involved can be overwhelming, leading students to skip necessary simplifications or perform operations out of order. For example, when parentheses are combined with fractions, students might divide prematurely or neglect simplifying within the innermost parentheses first. This creates confusion and incorrect final answers. Encouraging students to work from the innermost parentheses outward and double-check each step can help them handle nested operations with greater accuracy.
Overcomplicating Simple Expressions
Fractions often make expressions appear more complex than they are, leading students to overthink and complicate simple calculations. For instance, when dividing one fraction by another, some students mistakenly multiply by the given fraction rather than its reciprocal. Similarly, they might add unnecessary steps, introducing errors into otherwise straightforward operations. This tendency to overanalyze stems from a lack of confidence in handling fractions. Simplifying instructions and reinforcing the basics can help students approach these problems with greater clarity and efficiency.
Simplification Errors
After performing operations, students frequently make mistakes in simplifying their answers, particularly when dealing with improper fractions or reducing to lowest terms. They might leave improper fractions unsimplified or simplify incorrectly, such as by reducing only the numerator or denominator. Additionally, students sometimes over-simplify, resulting in an answer that no longer reflects the original fraction’s value. These errors not only impact accuracy but also hinder students’ understanding of how fractions represent quantities. Consistent practice in simplifying fractions, paired with clear feedback, helps students build this critical skill and avoid these common pitfalls.