PEMDAS Worksheets

All About These 15 Worksheets

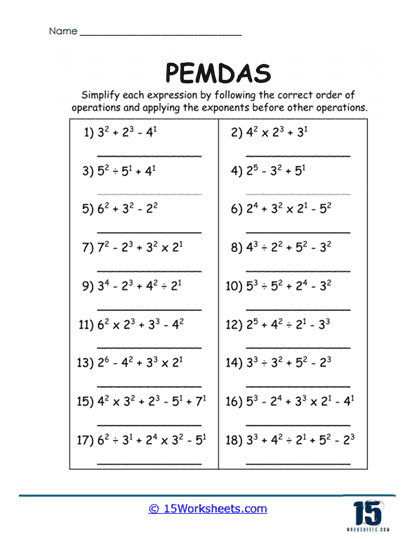
PEMDAS is an acronym representing the mathematical order of operations: Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction. This rule ensures a standardized approach to solving mathematical expressions, providing clarity and consistency in interpretation. By following PEMDAS, students and professionals alike can avoid confusion and arrive at correct results, even for complex equations. It serves as a critical foundation in mathematics, offering a universally accepted sequence that dictates how operations should be performed in multi-step calculations.

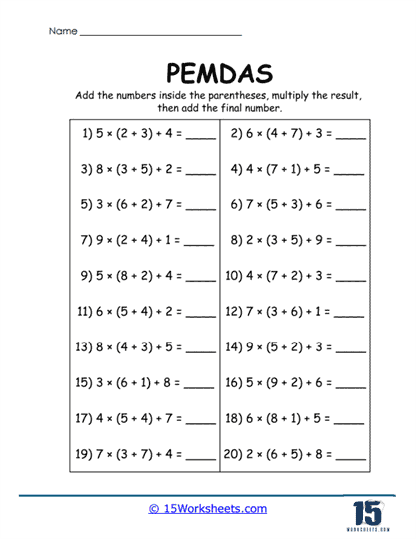
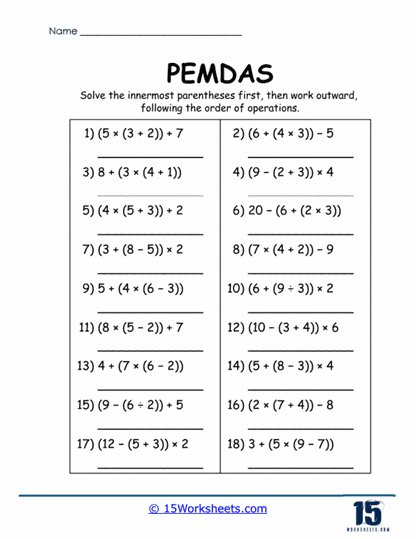
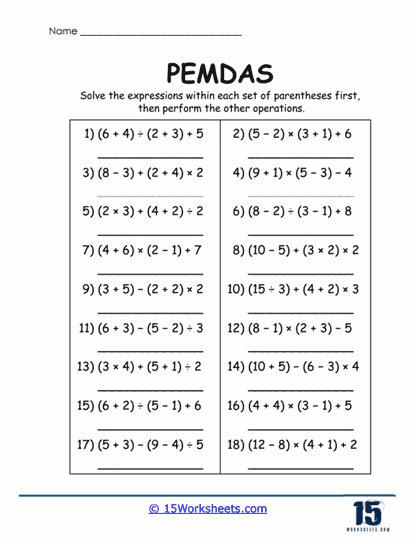
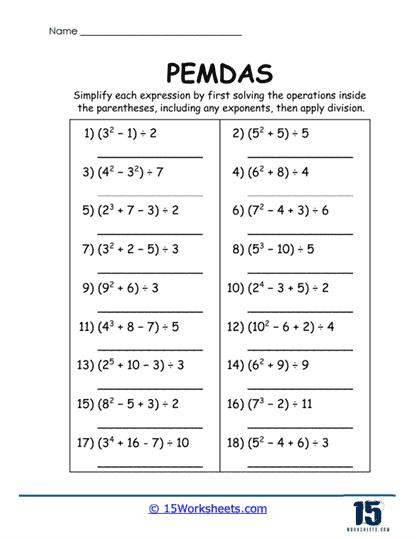
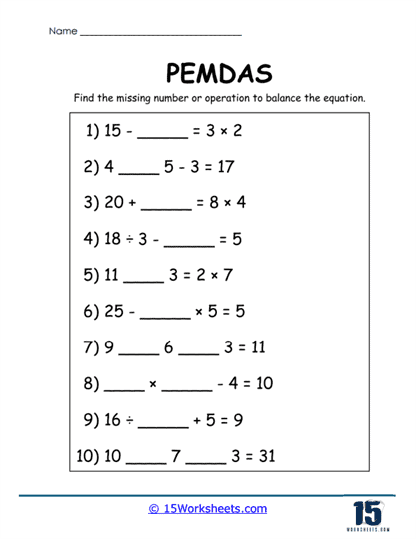
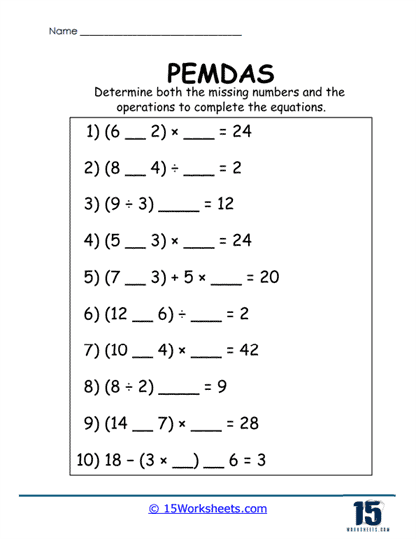
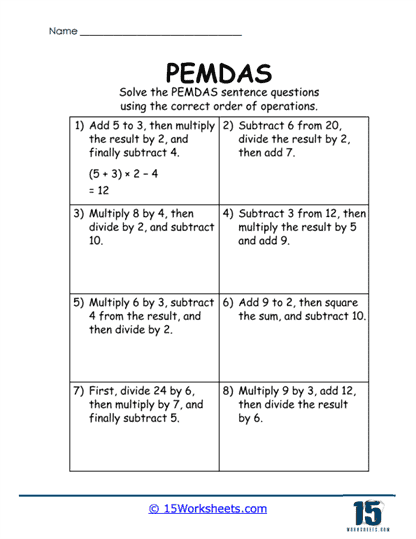
To help learners grasp the concept and application of PEMDAS, educators commonly use specialized worksheets. These PEMDAS worksheets are designed as educational tools that introduce, reinforce, and assess a student’s understanding of the rule. They typically feature problems that range from simple expressions, requiring basic application of the sequence, to more complex scenarios where students must carefully analyze and apply each step of the process. These exercises play a crucial role in developing problem-solving skills, encouraging logical thinking, and building confidence in handling mathematical operations systematically.

The versatility of PEMDAS worksheets makes them suitable for learners at various skill levels. Beginners might encounter straightforward tasks that focus on a single operation within parentheses or simple exponentiation, while advanced students tackle intricate problems involving nested parentheses and multiple steps. By practicing with these worksheets, students not only solidify their understanding of the order of operations but also improve their ability to approach math problems methodically. This foundational skill becomes invaluable as they progress in mathematics and encounter more challenging concepts that rely on the accurate application of PEMDAS.

PEMDAS is an essential concept because it prevents ambiguity in mathematical computations. For example, the expression 8 + 2 x 5 can yield different results depending on how it’s solved:

If solved left-to-right: (8 + 2) x 5 = 50

If solved using PEMDAS: 8 + (2 x 5) = 18

The correct answer, based on PEMDAS, is 18. This example highlights the importance of a consistent method for evaluating expressions.

The Purpose of PEMDAS Worksheets

Introducing the Rule

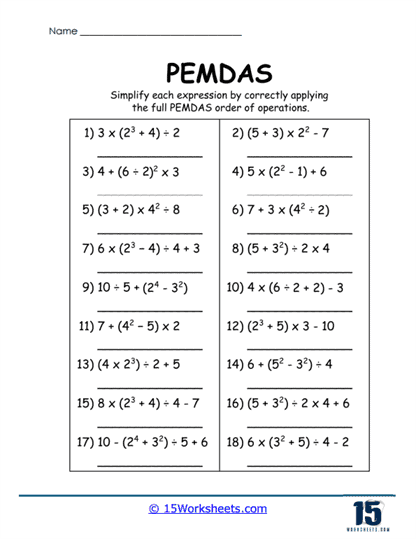
PEMDAS worksheets are instrumental in introducing students to the order of operations, providing a systematic framework for solving mathematical expressions. These worksheets typically start with simple problems, allowing students to grasp the sequence of operations: Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right). This structured introduction ensures that students understand not only the rule itself but also its importance in maintaining consistency and accuracy in math. By starting with manageable exercises, students can focus on learning the concept without being overwhelmed by overly complex equations.

As students progress, the worksheets gradually increase in complexity, reinforcing the importance of mastering each step of the process. Visual aids, such as flowcharts or mnemonic devices, often accompany these exercises to help students internalize the rule. Teachers can also use these worksheets to demonstrate how following the order of operations leads to correct and consistent results, providing examples of how errors occur when the sequence is ignored. This foundational phase is crucial in preparing students for more advanced math topics that rely on the principles of PEMDAS.

Practicing Skills

Once the basics are introduced, PEMDAS worksheets offer extensive practice opportunities that help students refine their skills. Regular practice enables students to recognize patterns, identify the correct order of steps, and apply the rule with greater ease. Worksheets often include a variety of problem types, such as numeric expressions, word problems, and equations with nested parentheses, to ensure that students can apply PEMDAS in diverse contexts. This variety keeps students engaged and ensures that their understanding extends beyond rote memorization.

Consistent practice allows students to spot and correct their own mistakes, fostering a sense of self-reliance. As they work through problems, they develop strategies to approach complex expressions systematically, improving their speed and accuracy over time. This phase of skill-building is essential for bridging the gap between learning the rule and applying it confidently in real-world math scenarios or standardized tests.

Assessing Understanding

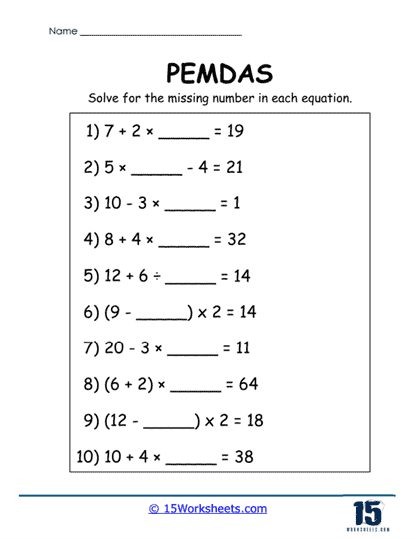
Teachers utilize PEMDAS worksheets as a diagnostic tool to assess students’ understanding and pinpoint areas where they may struggle. These worksheets serve as an objective measure of whether students can apply the rule consistently and accurately in a variety of problems. By reviewing students’ work, teachers can identify common mistakes, such as misapplying operations or overlooking parentheses, and address these gaps through targeted instruction.

The worksheets often include progressively challenging problems to test deeper levels of understanding. For example, while simpler problems assess basic comprehension, more complex problems evaluate students’ ability to apply the rule in multi-step or abstract contexts. This layered approach helps teachers differentiate instruction, offering advanced challenges for proficient students while providing additional support to those who need reinforcement.

Common Challenges Students Face with PEMDAS

Misunderstanding Parentheses

Students often misinterpret how to handle operations inside parentheses, believing that simply enclosing numbers and operations within parentheses dictates that every operation must be completed first without regard to the standard order of operations. For example, when solving (3 + 4 x 2), a common error is to prioritize addition over multiplication because the parentheses appear to “override” PEMDAS. This misstep can lead students to calculate (3 + 4) x 2 = 14, rather than the correct solution of 3 + (4 x 2) = 11. The confusion arises because students mistakenly treat parentheses as exempt from the internal application of PEMDAS.

The root of this misunderstanding often lies in insufficient practice with complex parenthetical expressions. Many students fail to realize that parentheses are merely a grouping mechanism that still requires respect for the operational hierarchy inside them. To address this, educators should create exercises that alternate between simple and complex parenthetical problems. For instance, worksheets should present problems like (2 + 3) alongside (2 + 3 x 4) to help students grasp that PEMDAS governs the operations within parentheses.

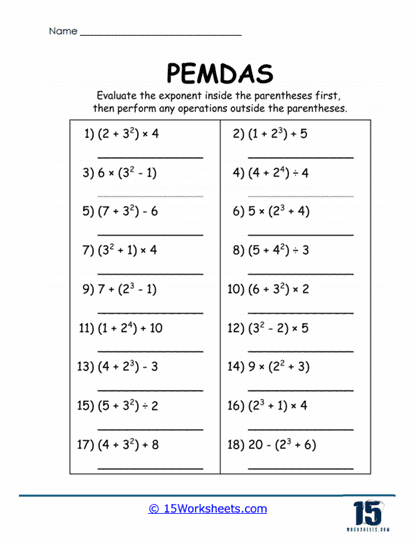
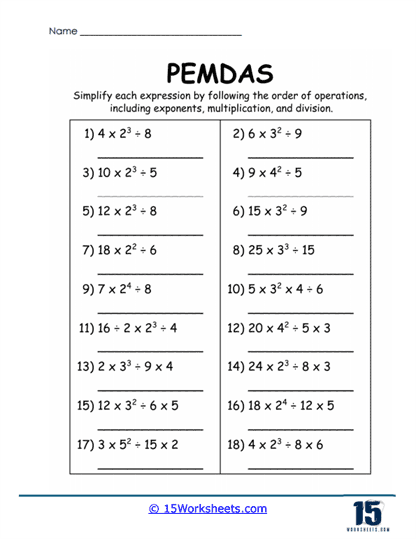
Exponents Confusion

Exponents frequently trip students up because they are less intuitive than basic arithmetic operations. Students may treat exponents as simple multipliers rather than recognizing them as repeated multiplication. For example, given 2³ x 4, a student might mistakenly compute (2 x 3) x 4 = 24 instead of the correct (2 x 2 x 2) x 4 = 32. This fundamental misunderstanding often stems from a lack of early exposure to the concept of powers and their role in the order of operations.

To combat this confusion, teachers should isolate exponentiation in exercises before integrating it into more complex equations. By offering straightforward exponent problems such as 3² and 5³, followed by progressively harder multi-step problems, students can build confidence. Visual aids, like diagrams or repeated multiplication breakdowns, can further demystify the concept and reinforce its unique role in PEMDAS.

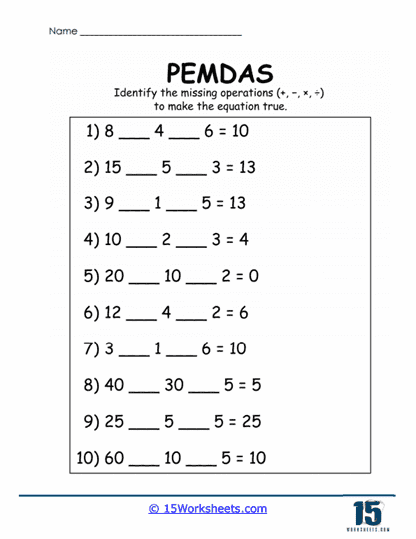
Multiplication and Division Order

Another frequent challenge is the misinterpretation of the PEMDAS acronym as strictly sequential, leading students to assume that multiplication always precedes division. For instance, in 8 ÷ 4 x 2, some students calculate 8 ÷ (4 x 2) = 1, incorrectly prioritizing multiplication because it appears earlier in the acronym. The correct approach, however, is to solve from left to right: (8 ÷ 4) x 2 = 4.

This error arises because students often overgeneralize the hierarchy implied by PEMDAS. Educators should clarify that multiplication and division share the same priority and must be addressed sequentially from left to right when both operations appear in a single expression. Worksheets with paired examples, such as 8 ÷ 4 x 2 and 8 x 4 ÷ 2, can highlight this nuance and help solidify the correct interpretation.

Addition and Subtraction Order

Addition and subtraction can confuse students who believe addition must always precede subtraction. In 10 – 5 + 2, some students incorrectly compute 10 – (5 + 2) = 3 instead of following the left-to-right rule: (10 – 5) + 2 = 7. This error mirrors the confusion seen with multiplication and division, stemming from the sequential misinterpretation of PEMDAS.

To address this, teachers should emphasize the shared priority of addition and subtraction while reiterating the importance of solving from left to right. Practice problems that mix these operations in various sequences, such as 7 + 3 – 2 and 10 – 4 + 6, can reinforce this principle. Using number lines or visual demonstrations can also make the left-to-right rule more intuitive for visual learners.

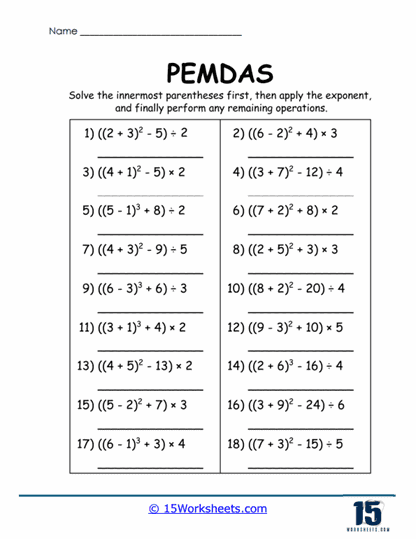
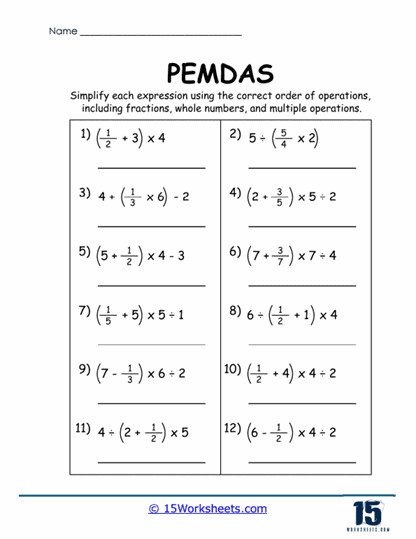
Combining All Steps

Multi-step problems pose a significant challenge as students must juggle multiple operations and keep track of intermediate steps. For example, in (3 + 2²) x (6 ÷ 3), it is common for students to skip steps or misapply the order of operations. They might calculate (3 + 4) x 6 ÷ 3 = 42, ignoring the necessity of solving (3 + 4) x 2 = 14 in proper sequence.

The difficulty here lies in the cognitive load required to manage numerous steps and rules simultaneously. To help students, teachers should introduce problems incrementally, encouraging them to solve in clearly defined stages. For example, breaking the problem into sub-parts, such as solving 2² first and isolating 6 ÷ 36 ÷ 3, allows students to focus on one operation at a time before combining results. Annotated solutions and guided practice are invaluable for building proficiency.