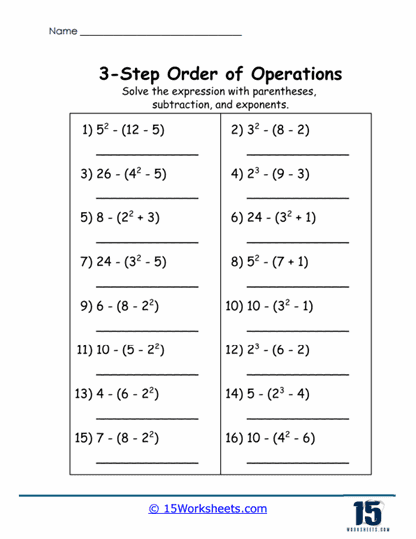
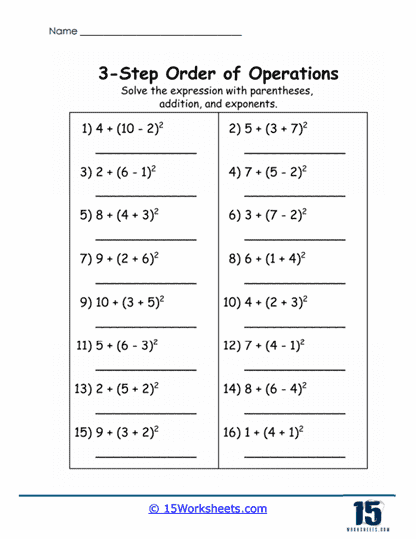
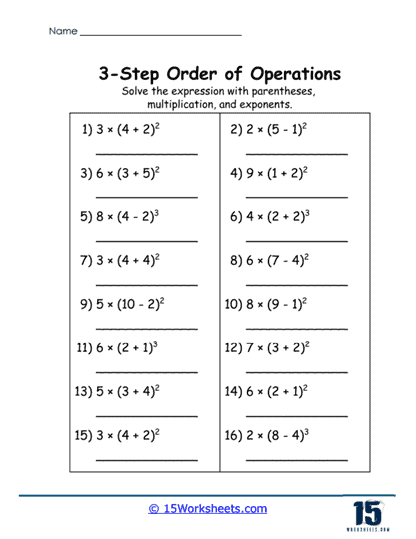
Three Step Order of Operations Worksheets

All About These 15 Worksheets

Three-step order of operations worksheets are a type of educational resource designed to help students practice and master the mathematical concept of performing operations in the correct sequence. These worksheets introduce problems that involve three distinct mathematical operations – addition, subtraction, multiplication, division, exponents, and sometimes parentheses – requiring students to apply the foundational rules of the order of operations systematically.

What Is the Order of Operations?

The order of operations is a set of rules that dictate the sequence in which mathematical operations should be performed to ensure consistency and accuracy in calculations. This sequence is commonly remembered by the acronym PEMDAS:

P: Parentheses (solve expressions inside parentheses first)

E: Exponents (evaluate powers or roots)

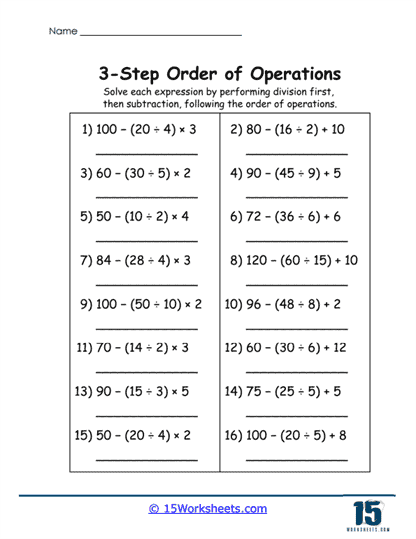
MD: Multiplication and Division (from left to right)

AS: Addition and Subtraction (from left to right)

The goal of order of operations worksheets is to help students practice applying these rules correctly, which is crucial as mathematical expressions grow more complex.

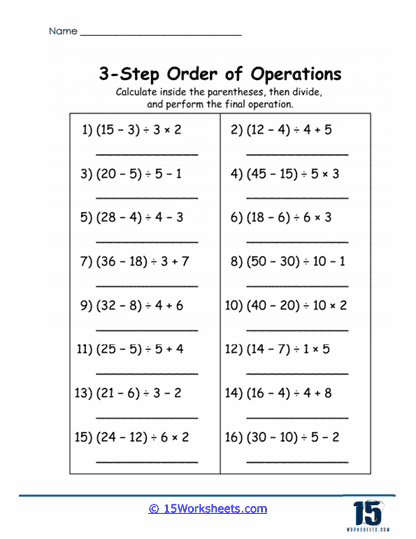
Structure of These Worksheets

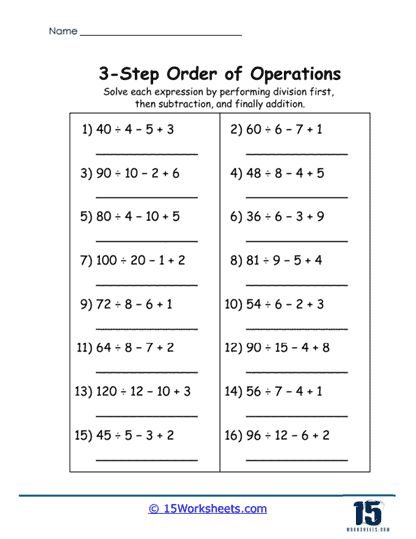
Three-step order of operations worksheets are designed to help students methodically solve problems by following a sequence of three mathematical operations. Each problem requires students to apply the correct order of operations, ensuring they understand the hierarchy of mathematical rules. The structure is incremental, starting with simpler problems and advancing to more complex equations as students build confidence and skill. This progression not only reinforces fundamental math principles but also encourages problem-solving discipline. By addressing these problems step by step, students develop a solid foundation for handling more advanced mathematical challenges in the future.

Simpler Problems

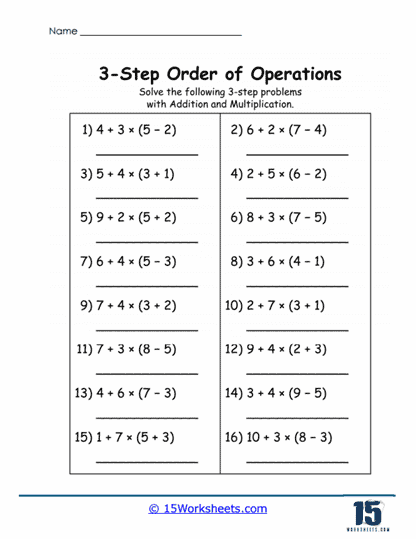
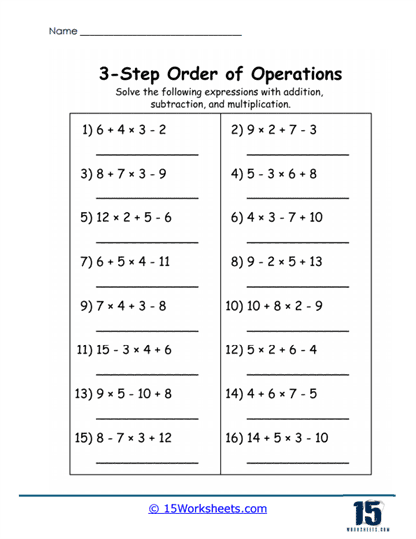
A typical example of a simpler problem might look like this: 8 + 6 x 3.

In such problems, students are required to apply the rule that multiplication takes precedence over addition.

This ensures that they calculate 6 x 3=18 before adding 8 + 18=26, rather than simply working from left to right. These problems help reinforce the critical concept that operations do not occur in the order they are written but must follow established mathematical conventions. By repeatedly practicing these simpler problems, students build a reliable understanding of prioritizing operations. The simplicity of these exercises ensures they are accessible to beginners, laying the groundwork for tackling more complex problems later.

Intermediate Problems

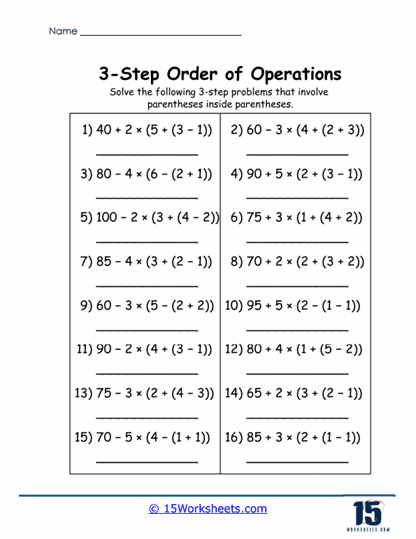
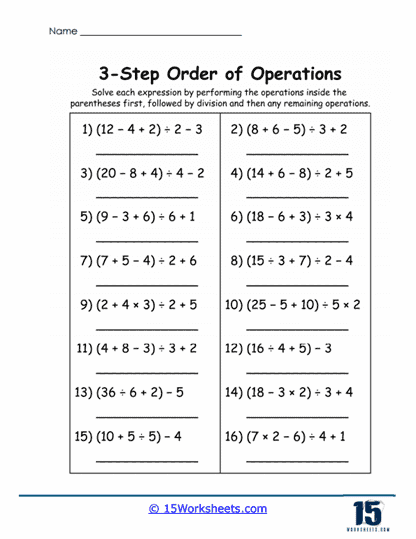
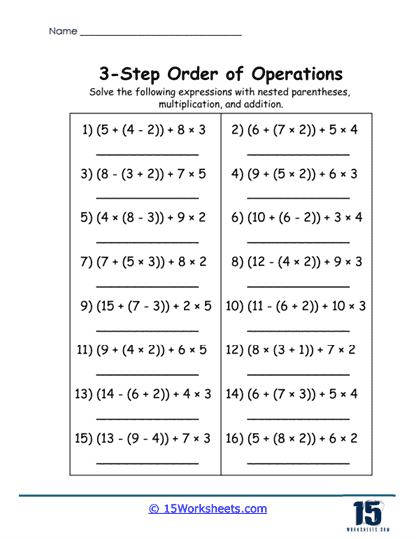
As students progress, they encounter intermediate problems such as (5 + 2) x 4 – 3.

These problems introduce parentheses, requiring students to first resolve the operation inside the parentheses: 5 + 2 = 7. Next, they move to multiplication, calculating 7 x 4 = 28, and finally subtract 3 to arrive at 28 – 3 = 25. This layer of complexity challenges students to manage multiple rules simultaneously, enhancing their ability to prioritize operations correctly. Intermediate problems are crucial for teaching students to slow down and evaluate the problem piece by piece. Such exercises build mental flexibility, as students learn to handle additional steps without becoming overwhelmed.

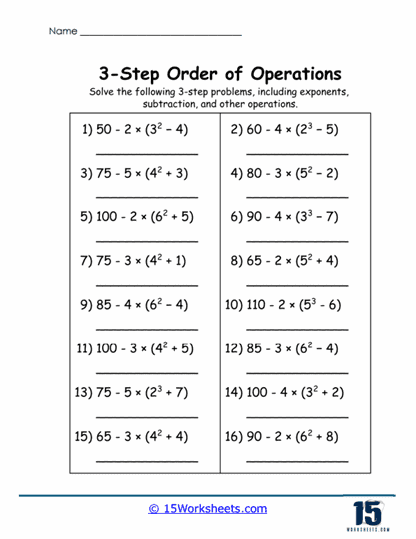
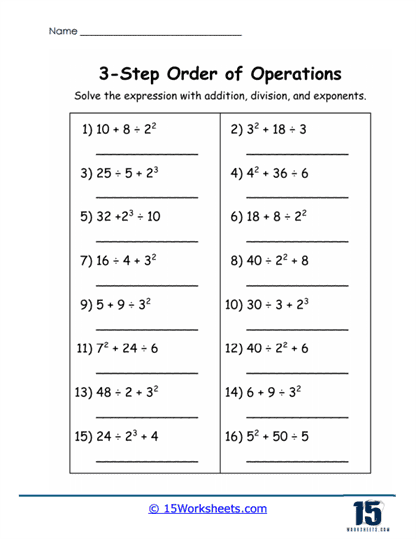
Advanced Problems with Exponents

For advanced learners, problems may involve exponents, adding another layer of complexity, as seen in an example like (3² + 2) ÷ 5 x 4.

To solve this, students must first handle the exponent 3² = 9, then proceed to resolve the parentheses by adding 9 + 2 = 11. Next, they divide 11 ÷ 5 = 2.2 and multiply the result by 4 to arrive at 2.2 x 4 = 8.8. These advanced problems require a deeper understanding of the order of operations, as well as precision in handling decimals and fractions. By incorporating exponents, these problems challenge students to integrate all their prior knowledge while introducing new concepts. Practicing such equations helps prepare students for even more complex math tasks in higher-level courses.

Three-Step Label and Incremental Learning

The “three-step” label signifies that these problems require exactly three distinct operations to solve, offering a clear structure for students to follow. This incremental approach is deliberate, allowing students to master basic rules before advancing to problems with additional layers of difficulty. The gradual increase in complexity ensures students do not feel overwhelmed while providing opportunities to build confidence with each step. This structured methodology helps to establish strong problem-solving habits, fostering both accuracy and efficiency in mathematical thinking. As students progress through the worksheets, they not only improve their computational skills but also develop a deeper appreciation for the logic and structure inherent in mathematics.

How They Can Develop Math Skills

The skills developed through these worksheets extend beyond basic arithmetic. They form the foundation for solving more complex mathematical equations and enhance problem-solving abilities in real-world contexts. This is not just about memorizing formulas or steps but about cultivating a deeper understanding and confidence in mathematical reasoning. These worksheets serve as tools that bridge foundational knowledge with advanced problem-solving capabilities, creating a continuum of learning. Let’s explore these benefits in greater depth:

1. Reinforcing the Basics of Arithmetic

Before students can confidently approach multi-step problems, they must master fundamental operations like addition, subtraction, multiplication, and division. These operations are the building blocks upon which all of mathematics is based, making their mastery crucial. Consistent practice with these worksheets ensures that students not only know how to perform these operations but also understand their logic and relationships. This fluency in basic computations allows students to approach more complex problems with greater ease, as they no longer struggle with simple mistakes. Over time, these exercises build confidence, enabling students to transition seamlessly into more challenging mathematical tasks.

2. Developing Logical Thinking

The order of operations teaches students to approach problems systematically. It requires them to follow a specific sequence, instilling a sense of discipline and structure in their problem-solving process. This logical progression is not just important in mathematics but is a transferable skill applicable to other disciplines such as computer science, engineering, and even daily decision-making. For instance, writing a computer program or designing a circuit both rely on step-by-step logical reasoning. Understanding how different operations interact and affect outcomes helps students anticipate results and avoid errors, laying the groundwork for success in technical and analytical fields.

3. Building Precision and Accuracy

Missteps in applying the order of operations can lead to incorrect results, which can be frustrating and demotivating for students. Through regular practice, these worksheets teach students to pay attention to details, such as the correct placement of parentheses or the sequence of multiplication and addition. This emphasis on precision helps students develop habits of double-checking their work, a skill that is invaluable in mathematics and beyond. In fields like engineering or medicine, where small errors can have significant consequences, the ability to perform tasks with precision and accuracy is essential. Students who learn these skills early are better prepared for success in rigorous academic and professional settings.

4. Encouraging Analytical Skills

By breaking down problems into smaller steps, students learn to analyze expressions and make decisions about what to solve first. This approach encourages them to think critically about the problem at hand rather than simply applying rote memorization. Analyzing a problem involves identifying patterns, understanding relationships, and predicting outcomes – all of which are key components of analytical thinking. This mindset is crucial not only in academics but also in real-world scenarios, such as budgeting, troubleshooting technical issues, or planning projects. Students who regularly practice this method become adept at breaking down complex tasks, leading to better decision-making and problem-solving abilities.

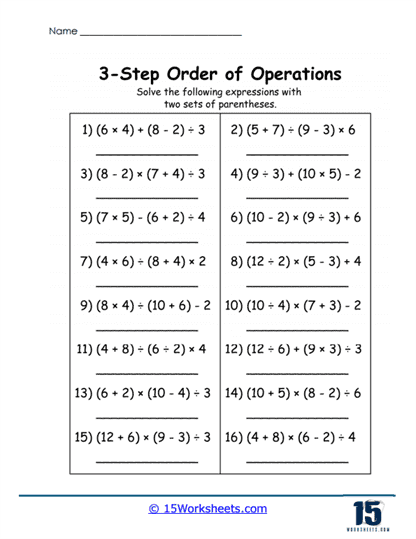
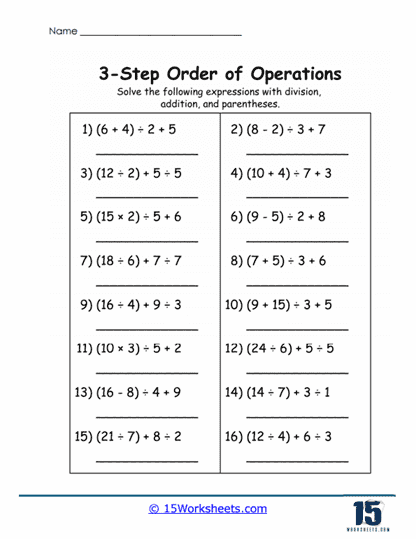
5. Introducing Parentheses and Grouping

The inclusion of parentheses in three-step problems introduces the concept of grouping, a foundational idea in algebra. Parentheses show students how to prioritize parts of an expression, teaching them to focus on one segment of a problem at a time. This skill becomes increasingly important as they progress to topics like solving equations, graphing, and working with functions. By understanding how grouping affects calculations, students can approach algebraic expressions with confidence and clarity. Moreover, this concept has real-world applications, such as understanding formulas in physics or organizing tasks in a project, making it a versatile and practical skill.

6. Preparing for Higher-Level Math

Topics like algebra, calculus, and statistics require a firm grasp of the order of operations. Mastering three-step problems lays the groundwork for understanding these advanced concepts. In algebra, simplifying expressions and solving equations both depend on a clear understanding of operational hierarchy. In calculus, evaluating functions, derivatives, and integrals often involves multi-step processes that require precision and accuracy. Similarly, in statistics, calculating probabilities, averages, or variances involves following specific sequences of operations. The foundational skills honed through these worksheets give students the confidence and competence needed to excel in these areas, ensuring that they are well-prepared for academic and professional challenges in mathematics and related fields.