Two Step Order of Operations Worksheets
All About These 15 Worksheets
Mathematics is a cornerstone of education, providing students with essential skills for problem-solving, logical reasoning, and decision-making. Two-step order of operations worksheets are a highly effective tool in this journey, offering structured, engaging, and accessible exercises tailored to strengthen foundational arithmetic abilities. These worksheets emphasize solving equations involving two operations, such as addition and multiplication or subtraction and division, ensuring students gain a clear understanding of the correct sequence of mathematical operations.
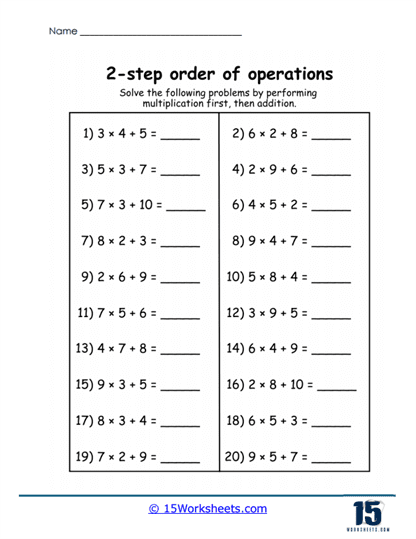
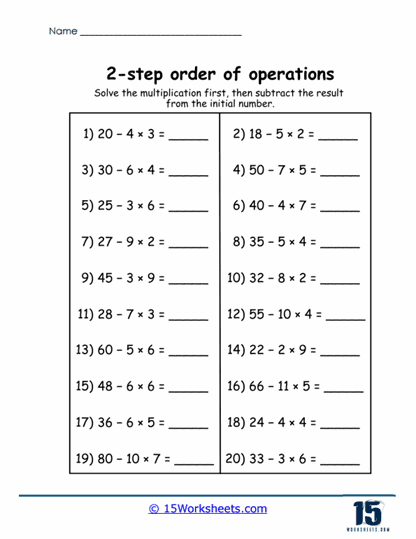
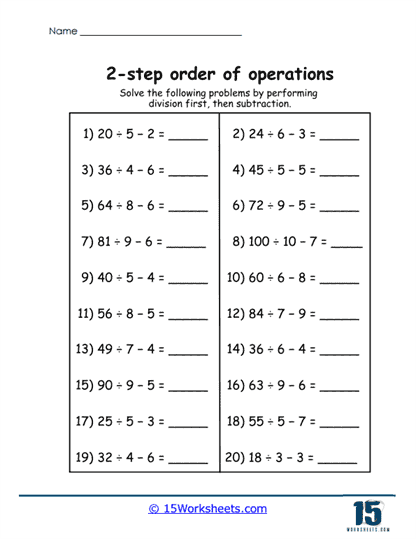
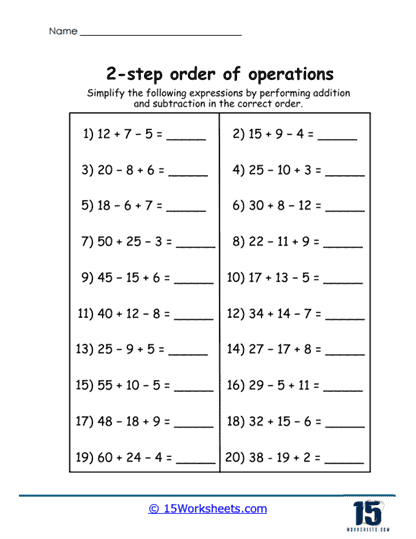
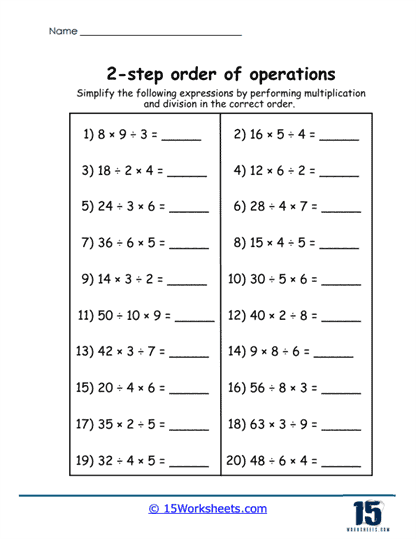
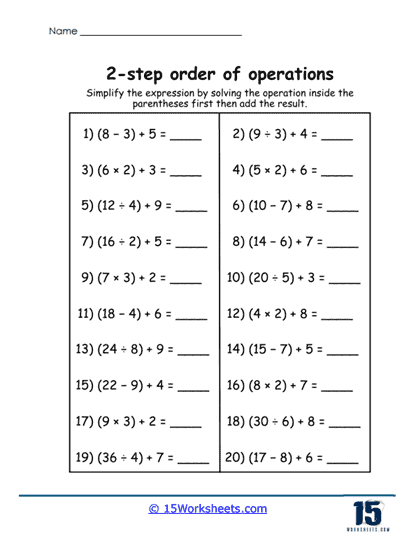
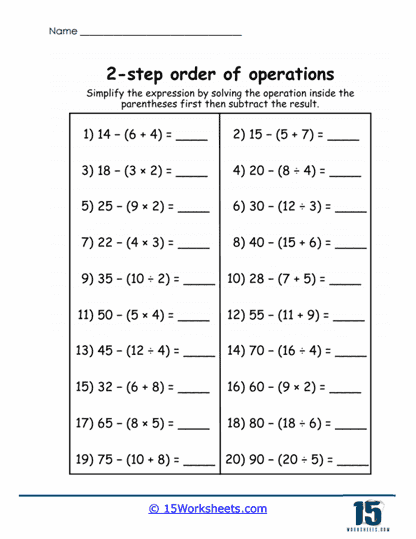
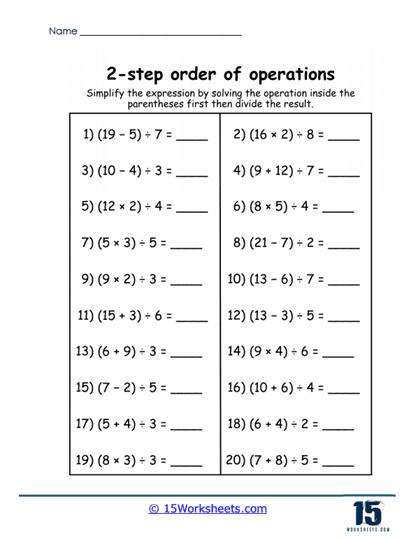
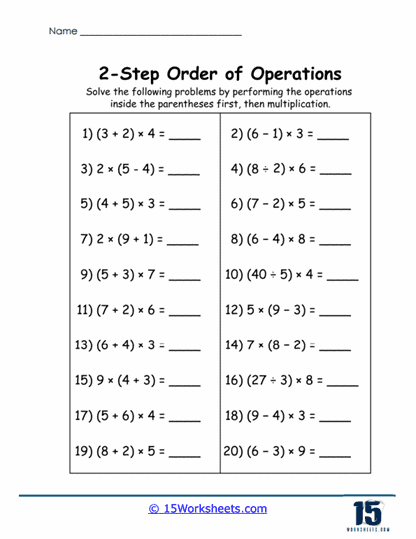
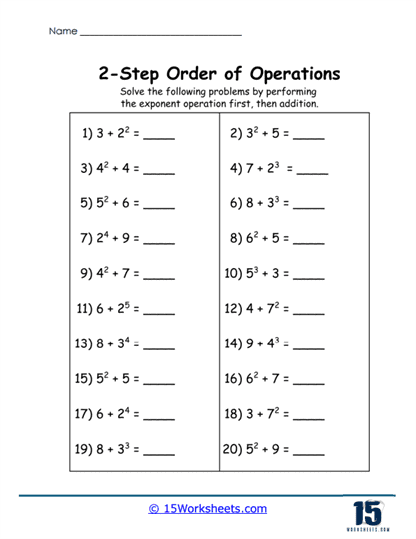
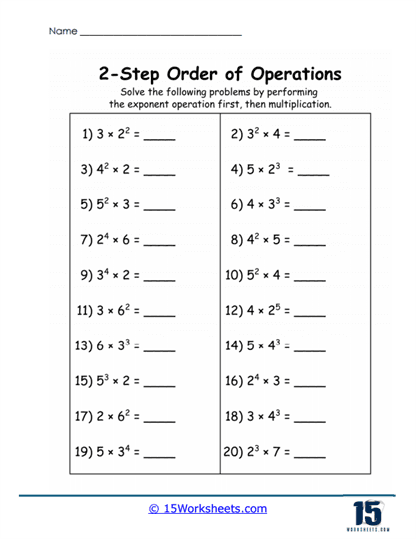
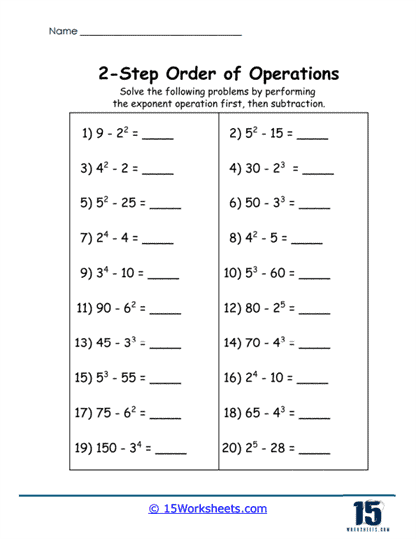
At their core, these worksheets are designed to help students practice the proper order of operations in arithmetic problems. Following the widely accepted PEMDAS/BODMAS rule – Parentheses/Brackets, Exponents/Orders, Multiplication and Division (from left to right), Addition and Subtraction (from left to right) – students learn to handle problems with multiple operations systematically. These worksheets focus on two-step problems, striking the right balance between simplicity and challenge to build confidence and mastery. For example, students may encounter problems such as 5 x 3 + 25 x 3 + 2 or 18 ÷ 6 – 118 ÷ 6 – 1, requiring them to apply multiplication or division before addition or subtraction.
Structure and Format of the Worksheets
The structure and format of these worksheets are meticulously crafted to address the needs of a wide range of learners. By combining various elements such as problem types, levels of difficulty, step-by-step problem-solving guidance, and diverse presentations, they ensure that students are engaged and learning effectively. Here’s an expanded breakdown of each key feature:
Variety in Problem Types
A key feature of these worksheets is the inclusion of a diverse range of problem types, from straightforward numerical expressions to immersive word problems. The numerical expressions serve as a foundation, helping students focus on mastering basic mathematical operations such as addition, subtraction, multiplication, and division without the distraction of extra context. Word problems, on the other hand, introduce complexity by requiring learners to analyze a scenario, extract relevant data, and apply their mathematical knowledge to solve real-world problems. This variety not only caters to different learning preferences but also ensures that students develop both procedural fluency and conceptual understanding. Furthermore, word problems enhance critical thinking by simulating scenarios students might encounter outside the classroom, making math more relatable and practical. The mix of problem types allows educators to assess multiple aspects of a student’s skill set, from basic computation to higher-order thinking.
Progressive Difficulty Levels
The worksheets are designed to accommodate learners at varying levels of proficiency by incorporating a progressive system of difficulty. Beginner-level worksheets introduce simple, straightforward problems with clear instructions, allowing students to build confidence in their foundational skills. As students advance, they encounter intermediate problems that might include slightly larger numbers, multiple steps, or additional elements like fractions or decimals. Advanced worksheets go further by incorporating abstract problem wording, multi-step operations, or tasks that require higher-order reasoning. This progression ensures that students remain challenged without feeling overwhelmed, fostering a sense of accomplishment as they advance. By gradually increasing the complexity, the worksheets support differentiated learning, enabling educators to provide targeted instruction based on individual needs. The structure also encourages self-paced learning, as students can work through problems at a level that matches their readiness.
Step-by-Step Problem Sets
To guide students in effective problem-solving, the worksheets feature ample space and a step-by-step structure for tackling problems. Each problem is designed to encourage logical sequencing, helping students understand the importance of solving operations in the correct order, such as following the principles of PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction). By laying out problems in this format, students are less likely to skip steps or arrive at incorrect answers due to oversight. The inclusion of space for annotations allows learners to break down complex problems into manageable components, reinforcing the process of working through solutions systematically. This approach not only helps students internalize mathematical procedures but also builds habits of organized thinking and precision. Additionally, it provides educators with insight into a student’s thought process, making it easier to identify and address misconceptions.
Varied Presentation
Recognizing that monotony can hinder engagement, these worksheets incorporate a variety of presentation styles. Problems are delivered in formats such as grids, tables, diagrams, and standalone equations, catering to different visual preferences and learning styles. For example, tables and grids can help students organize data and see patterns, while standalone equations challenge them to apply skills in a direct and focused manner. Visual learners, in particular, benefit from diagrams or graphic representations that make abstract concepts more tangible. The varied formats keep the material fresh and engaging, reducing the likelihood of students becoming bored or disinterested. Moreover, the diversity in presentation mimics real-world applications of math, where problems do not always appear in uniform formats. This adaptability prepares students to approach a range of scenarios with confidence and versatility.
A Step-by-Step Example
To illustrate the utility of these worksheets, consider a sample problem:
Example Problem: Solve 8 + 4 x 28 + 4 x 2.
Step 1: Apply the multiplication operation first.
According to the order of operations, multiplication precedes addition.
Compute 4 x 24 x 2, which equals 88.
Step 2: Add the result of the multiplication to 8.
Compute 8 + 88 + 8, which equals 1616.
Conclusion: The solution to 8 + 4 x 28 + 4 x 2 is 1616.
By presenting problems in this step-by-step format, the worksheets help students clearly understand the rationale behind each operation. This clarity is especially beneficial for learners who may struggle with remembering or applying the rules of operation sequences.
The impact of consistent practice with two-step order of operations worksheets goes beyond immediate skill acquisition. Over time, students develop a more intuitive understanding of how mathematical operations interact, equipping them for more advanced studies in algebra, geometry, and beyond. The logical reasoning skills honed through these exercises also translate into other disciplines, including science and computer programming.
By using these worksheets regularly, students cultivate a problem-solving mindset, gaining not only mathematical knowledge but also the confidence to approach challenges methodically. These lifelong skills ensure they are well-prepared for academic success and practical problem-solving in everyday life.