Order of Operations with Exponents Worksheets

All About These 15 Worksheets

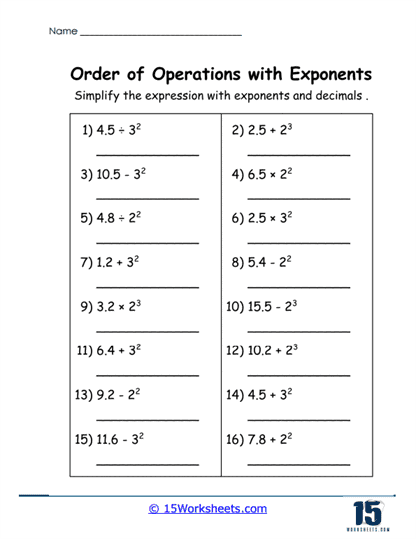
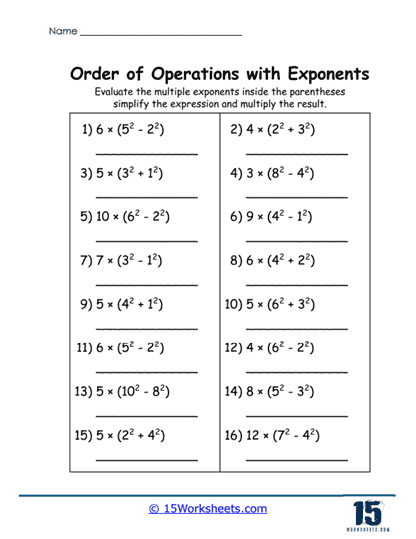
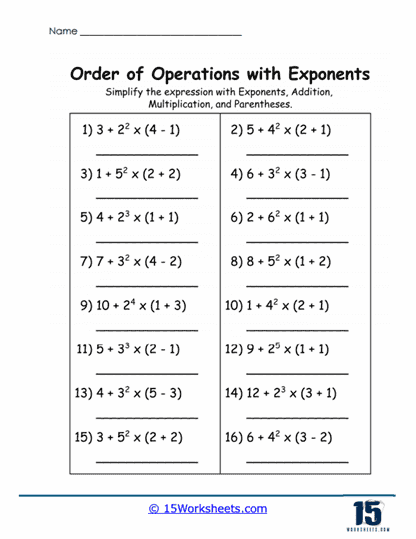
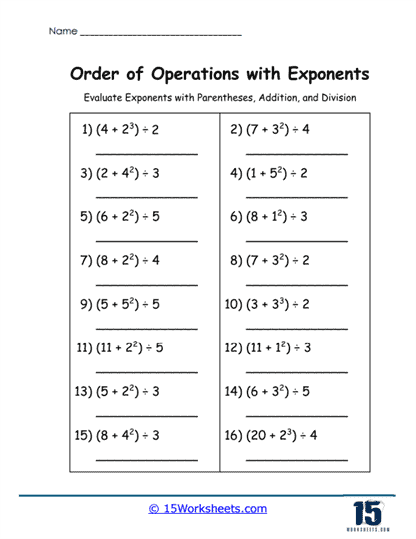
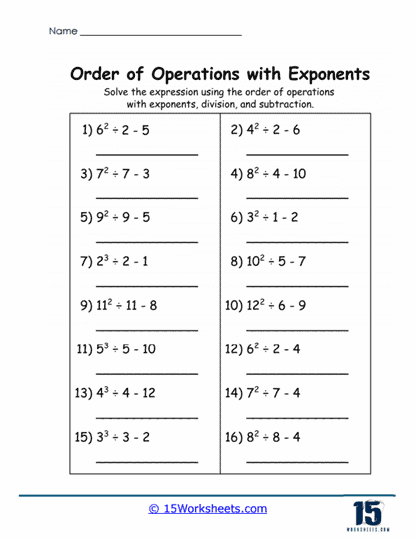
These worksheets were designed to help students understand the structured sequence of operations required to solve mathematical expressions involving exponents. These worksheets emphasize the significance of following the correct sequence, commonly known as PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), to arrive at accurate solutions. By adhering to the proper sequence, students gain confidence in handling increasingly complex mathematical problems. These exercises serve as an essential foundation for mastering algebra and other advanced mathematical topics.

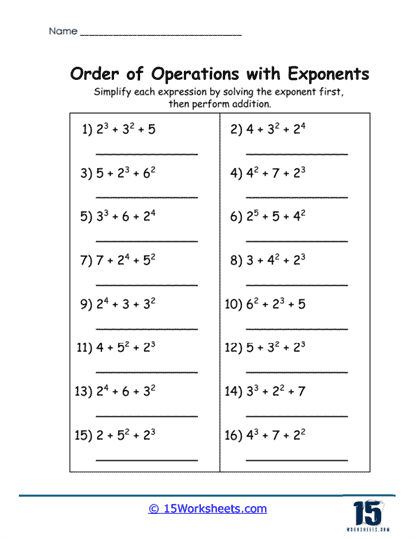
The primary goal of these worksheets is to teach students how to correctly interpret and evaluate exponents within a broader expression. Exponents indicate repeated multiplication, and understanding this concept is key to solving problems involving powers. Worksheets often provide visual aids, step-by-step examples, and targeted practice to solidify this understanding. By focusing on exponents in isolation and then integrating them with other operations, students can gradually build their skills.

These worksheets cater to a wide range of learning levels. From introducing basic exponents to exploring multi-step problems that combine them with various operations, they allow students to progress at their own pace. This progression ensures a thorough understanding of the topic and prepares students for more advanced mathematical challenges.

The Role of Exponents in the Order of Operations

Exponents, which signify repeated multiplication, play a fundamental role in maintaining the structure and consistency of mathematical problem-solving. Positioned immediately after parentheses in the PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction) hierarchy, they serve as a cornerstone for establishing the correct sequence of calculations. Their placement ensures that operations involving powers are completed before the more straightforward processes of multiplication, division, addition, or subtraction, thereby minimizing ambiguity and errors.

This specific placement in the order of operations is not arbitrary but is designed to uphold logical clarity in mathematical expressions. Without this standardized sequence, the interpretation of expressions could vary, leading to conflicting results. For example, an expression like 3 + 2² x 4 would yield different outcomes depending on whether the exponent was resolved first or after the multiplication. Adhering to the PEMDAS framework ensures uniformity, making exponents a crucial element in preserving mathematical coherence.

How Exponents Influence the Order of Operations

Priority After Parentheses

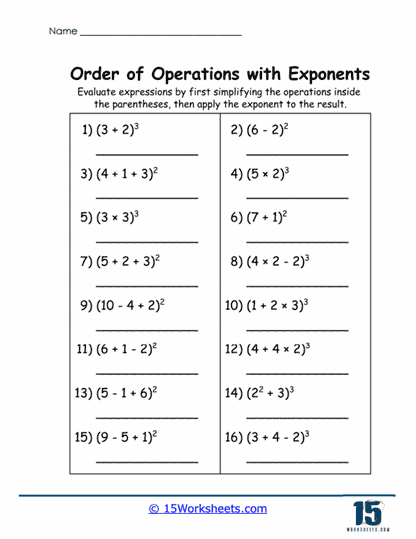
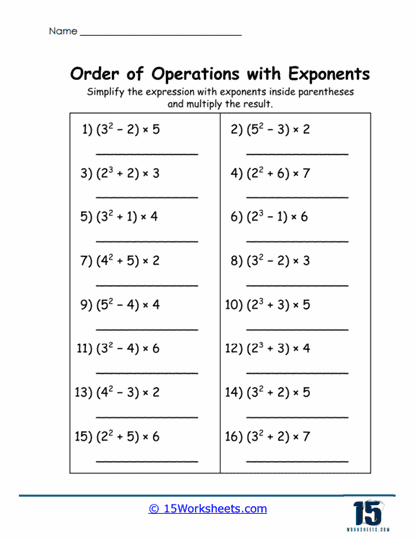
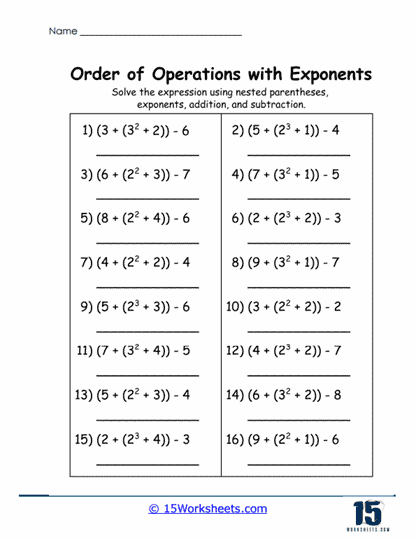
When expressions involve multiple operations, exponents are evaluated immediately after resolving parentheses or other grouping symbols. This priority ensures that complex expressions retain their intended meaning. Consider the example (3 + 2)². Here, the addition inside the parentheses is resolved first to simplify the expression to 5², and then the exponentiation is performed to yield 25. Without following this sequence, the integrity of the operation could be compromised, leading to incorrect outcomes.

This rule emphasizes that grouping symbols take precedence, but exponents come next, establishing their dominant role in structuring calculations. By resolving parentheses first, we simplify expressions to manageable forms before applying the exponential operation. This layered approach ensures that more intricate expressions, such as (4 + 1)³ – 2², can be systematically simplified without confusion.

Interaction with Multiplication and Division

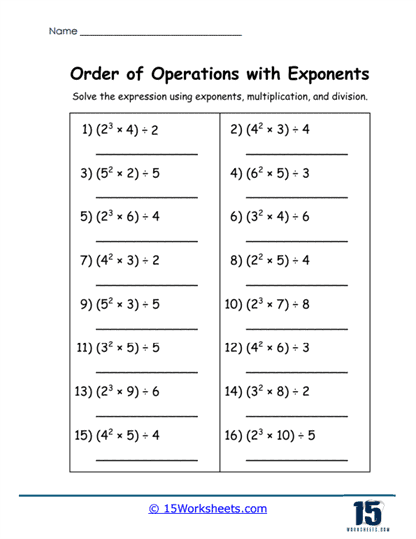
Exponents must always be resolved before addressing multiplication or division unless parentheses dictate otherwise. This prioritization avoids misunderstandings and ensures accuracy in computation. For instance, in 2 x 3², the exponentiation 3² = 9 is resolved first, resulting in 2 x 9 = 18. Attempting to multiply first, however, would incorrectly treat the expression as (2 x 3)², yielding a wrong answer of 36.

This clear distinction between exponents and other operations highlights their intermediate priority in the hierarchy. While multiplication and division are fundamental, they do not supersede the rules governing exponents. This logical structuring allows mathematicians and students to approach calculations systematically, building confidence in their problem-solving methods.

Impact on Addition and Subtraction

Similarly, exponents always precede addition and subtraction unless explicitly overridden by grouping symbols. This ensures that powers are calculated first, preserving the intended complexity of the expression. Take 3 + 2³, for instance. Here, 2³ is resolved to 8, and then added to 3, yielding 11. If addition were performed first, the calculation would mistakenly result in 5³ = 125, a vastly different and incorrect result.

By prioritizing exponents, the mathematical order remains consistent and logical. This rule also prevents potential confusion when dealing with combined operations, such as 7 – 3² + 5, where exponents are calculated first, followed by the left-to-right resolution of subtraction and addition.

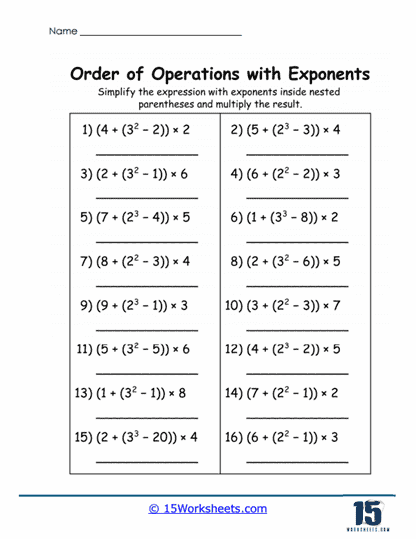
Nesting Exponents

When exponents are nested or combined with additional operations, they are resolved from the innermost level outward, ensuring a step-by-step approach to simplify expressions. For example, in (2²)³, the inner exponent 2² = 4 is resolved first, resulting in 4³ = 64. This methodical process ensures accuracy, even in complex scenarios.

Nesting rules also apply to expressions involving additional operations, such as ((3 + 1)²)³. Here, the parentheses are resolved first, yielding (4²)³. Then, following the nesting rule, 2³ is calculated to give 4⁸, an extensive computation emphasizing the importance of proper sequencing. By following these rules, even intricate calculations can be approached with clarity and confidence.

Common Mistakes and Misconceptions

Skipping Steps

One common error is skipping steps in the order of operations, particularly ignoring exponents and jumping directly to addition or multiplication. For instance, when solving 2 + 3², some students might incorrectly add 2 + 3 first, yielding 5² = 25 instead of the correct result, 2 + 9 = 11. This tendency often stems from a misunderstanding of the hierarchy of operations or a rushed approach to problem-solving, where the significance of exponents is underestimated.

This mistake not only leads to incorrect answers but also hinders a deeper understanding of mathematical structure. By skipping the evaluation of exponents, students miss the opportunity to engage with the rules that govern complex expressions. Addressing this issue requires emphasizing the importance of following the sequence systematically and practicing problems that isolate each operation step to reinforce clarity and accuracy.

Misinterpreting PEMDAS

Another common misconception is interpreting PEMDAS as requiring a strict left-to-right evaluation for all operations, leading to errors in calculation. For example, in the expression 4 + 2 x 3², students might process the operations in sequence as 4 + 2= 6 and 6 x 3² = 54. However, following the correct order of operations, exponents are addressed first (3² = 9), then multiplication (2 x 9 = 18), and finally addition (4 + 18 = 22).

This confusion arises from an oversimplified interpretation of PEMDAS, where students incorrectly apply left-to-right processing uniformly instead of recognizing the hierarchy within the acronym. To counter this, educators should provide explicit examples showing how the order is prioritized, using visual aids or mnemonic enhancements to reinforce that multiplication and division, as well as addition and subtraction, are resolved only after higher-priority operations like parentheses and exponents.

Confusion with Negative Bases

Negative bases introduce another layer of complexity that often confounds students. Consider the distinction between (-3)² and – 3². The expression (-3)² treats the negative as part of the base, resulting in (-3) x (-3) = 9. In contrast, -3² follows the order of operations, where the exponent is applied first to 3, yielding 3² = 9, and the negative is then applied, giving -9.

This confusion stems from not understanding how parentheses dictate grouping and the order of operations. Without parentheses, the exponentiation takes precedence over the negative sign, often leading to misinterpretation. To address this, students need practice in distinguishing between scenarios where parentheses are present and absent. Visual demonstrations and real-world analogies can help clarify these differences, ensuring they comprehend how grouping symbols affect the calculation’s outcome.