Complementary and Supplementary Angles Worksheets
About These 15 Worksheets
These worksheets will help students understand and practice calculating the measures of angles that sum to specific values. Complementary angles are two angles whose measures add up to 90 degrees, while supplementary angles are two angles whose measures add up to 180 degrees. These worksheets typically contain a variety of exercises that require students to identify, calculate, and compare these types of angles. By engaging with these worksheets, students develop a deeper understanding of geometric relationships and improve their mathematical problem-solving skills.
Math Skills Explored
The primary math skills explored in complementary and supplementary angles worksheets include angle identification, angle calculation, and the application of geometric principles. Students learn to recognize the characteristics of complementary and supplementary angles and apply their knowledge to solve various problems. These worksheets also reinforce students’ understanding of the relationship between angles and their measures. Additionally, students practice arithmetic skills as they calculate the measures of unknown angles, enhancing their overall mathematical proficiency.
Types of Exercises
Complementary and supplementary angles worksheets offer a variety of exercises and practice problems to cater to different learning styles and skill levels. Below are the different types of exercises commonly found in these worksheets:
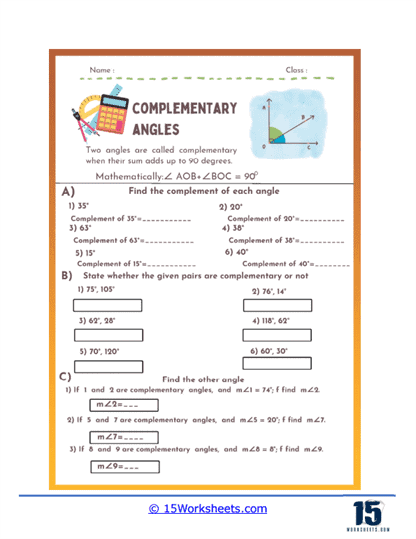
Finding Complements and Supplements – In this type of exercise, students are given a specific angle measure and are asked to find its complement and supplement. For example, if the given angle is 30 degrees, students must calculate that the complement is 60 degrees (since 30 + 60 = 90) and the supplement is 150 degrees (since 30 + 150 = 180). This exercise helps students practice basic arithmetic and reinforces their understanding of the definitions of complementary and supplementary angles.
Identifying Pairs – Students are presented with pairs of angles and must determine whether they are complementary, supplementary, or neither. This exercise requires students to add the measures of the given angles and compare the sum to 90 and 180 degrees. For example, if the given pair is 45 degrees and 45 degrees, students will identify them as complementary because their sum is 90 degrees. This type of problem helps students practice their addition skills and reinforces their understanding of angle relationships.
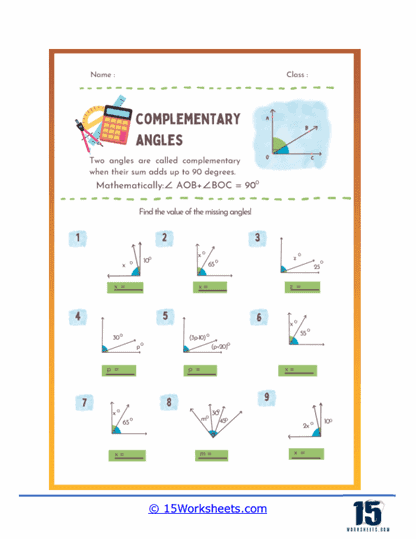
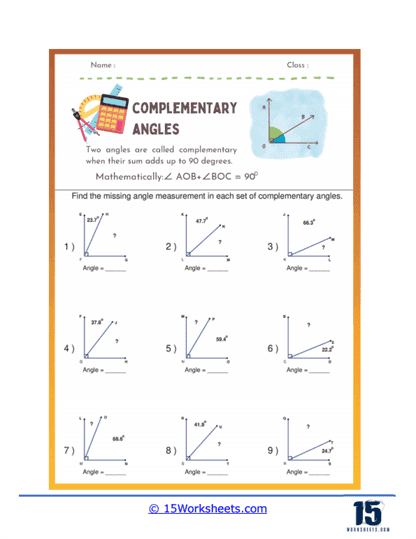
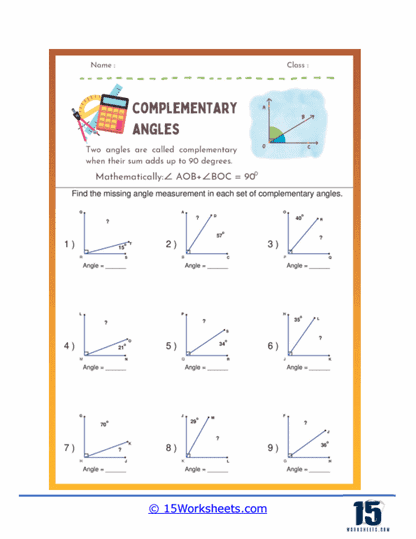
Solving for Unknown Angles – These problems present students with one angle measure in a complementary or supplementary pair and require them to find the measure of the missing angle. For example, if students know that one angle is 65 degrees and it is complementary to another angle, they must calculate that the missing angle is 25 degrees (since 65 + 25 = 90). This exercise helps students practice subtraction and enhances their problem-solving abilities.
Word Problems – Complementary and supplementary angles worksheets often include word problems that require students to apply their knowledge of angle relationships to real-world scenarios. For example, a problem might describe the angles of a ramp or the angles formed by the hands of a clock, and students must determine whether the angles are complementary or supplementary. These problems help students see the practical applications of their mathematical knowledge and develop critical thinking skills.
Benefits of These Worksheets
Complementary and supplementary angles worksheets offer numerous benefits for students, enhancing their understanding of geometry and building essential mathematical skills. Firstly, these worksheets help build a strong foundation in geometry by developing essential skills in angle measurement and relationships. Understanding complementary and supplementary angles is a fundamental concept in geometry that serves as a building block for more advanced mathematical studies. By mastering these basic angle relationships, students are better prepared to tackle complex geometric problems and proofs, fostering a deeper comprehension of the subject.
Enhancing Problem-Solving Abilities
These worksheets significantly enhance students’ problem-solving abilities. By working through various exercises and practice problems, students develop critical thinking skills and learn to approach problems systematically. This structured approach to problem-solving is valuable not only in mathematics but also in other academic subjects and everyday life. As students encounter different types of problems involving complementary and supplementary angles, they learn to apply logical reasoning and develop strategies to find solutions efficiently. This skill set is essential for academic success and can be transferred to various real-world scenarios, improving overall cognitive abilities.
Practical Application of Arithmetic Skills
Complementary and supplementary angles worksheets provide a practical application of arithmetic skills, reinforcing basic mathematical operations. Students practice addition and subtraction as they calculate the measures of complementary and supplementary angles, helping to solidify their arithmetic skills and enhance their overall mathematical proficiency. This reinforcement is crucial for building confidence in handling numbers and performing calculations accurately. By integrating arithmetic practice into geometric exercises, these worksheets offer a comprehensive learning experience that strengthens multiple areas of mathematical understanding.
Engaging Learning Experience
Complementary and supplementary angles worksheets provide an engaging and interactive learning experience for students. The variety of exercises and practice problems keeps students motivated and interested in the subject matter. The hands-on approach helps make abstract geometric concepts more concrete and understandable, facilitating better retention and comprehension. Interactive elements such as puzzles, real-life scenarios, and practical applications make learning enjoyable and relevant. This engagement is essential for maintaining students’ interest in geometry and encouraging a positive attitude towards learning mathematics.
Visual Learning Aids
These worksheets often include visual aids, such as diagrams and illustrations, to help students better understand the concepts. Visual aids are particularly helpful for visual learners and can make the learning process more enjoyable. Diagrams showing how complementary and supplementary angles interact in different shapes and real-world contexts help students visualize the relationships and internalize the concepts more effectively. By catering to different learning styles, these worksheets ensure that all students have the opportunity to grasp the material and succeed in their studies.
Versatility in Teaching
Teachers can use complementary and supplementary angles worksheets in various ways, including individual practice, group activities, homework assignments, and assessments. This versatility makes them a valuable resource in the classroom, allowing educators to adapt their teaching strategies to meet the needs of their students. Worksheets can serve as a quick assessment tool to gauge students’ understanding, as a collaborative group activity to encourage peer learning, or as homework to reinforce classroom instruction. This flexibility ensures comprehensive instruction and provides multiple opportunities for students to practice and master the concepts.
Progressive Difficulty
The progressive nature of the exercises ensures that students can build their skills gradually. Starting with basic identification tasks, such as recognizing and labeling complementary and supplementary angles, and moving to more complex problems, like solving for unknown angles in geometric figures, helps ensure a thorough understanding of the topic. This gradual increase in difficulty allows students to build confidence as they master each level before moving on to more challenging tasks. By scaffolding the learning process, these worksheets support continuous improvement and mastery of geometric concepts.
Practical Applications
The skills developed through complementary and supplementary angles worksheets have numerous practical applications. In fields such as engineering, architecture, and design, understanding angle relationships is crucial for creating accurate and functional structures. For example, architects use their knowledge of angles to design buildings with precise angles and measurements. Engineers apply these principles to ensure that components fit together correctly in machinery and structures, maintaining both functionality and safety. Understanding complementary and supplementary angles is essential for solving real-world problems, such as determining unknown angles in various shapes and structures, which is a vital skill in many technical professions.
Real-World Applications
Complementary and supplementary angles play crucial roles in various real-world applications, extending beyond the classroom to numerous practical scenarios. Understanding these angles equips students with the skills needed to navigate and solve real-life problems efficiently. For example, knowing that the angles in a triangle add up to 180 degrees helps in determining unknown angles in various shapes and structures. Similarly, understanding the relationship between the angles formed by the hands of a clock can help in time-telling and scheduling. By recognizing and applying the principles of complementary and supplementary angles, students can gain insights into various fields such as architecture, engineering, interior design, sports, and even everyday tasks.
Sports and Everyday Tasks
In sports, particularly those involving aiming and trajectory, complementary and supplementary angles are frequently used. For instance, in basketball, players use complementary angles when aiming their shots. The angle of the shot and the backboard must complement each other to ensure the ball goes into the hoop. In baseball, the trajectory of a pitch and the angle at which the bat strikes the ball are essential for successful hits. Coaches and athletes analyze these angles to improve performance and strategy. Understanding the angles involved can enhance an athlete’s ability to execute precise movements and achieve better results in their sport.
Even in everyday tasks, people encounter and utilize complementary and supplementary angles. For example, when hanging a picture frame on a wall, ensuring that the frame is level involves understanding and applying complementary angles. Similarly, when cutting a piece of wood or fabric at a specific angle, knowing how to calculate supplementary angles ensures that the pieces fit together correctly. These skills are also useful in DIY projects and home improvement tasks, where precise measurements and angles are crucial for successful outcomes. Understanding these angle relationships helps individuals perform tasks accurately and efficiently, demonstrating the practical value of geometry in daily life.