Adjacent Angles Worksheets
About These 15 Worksheets
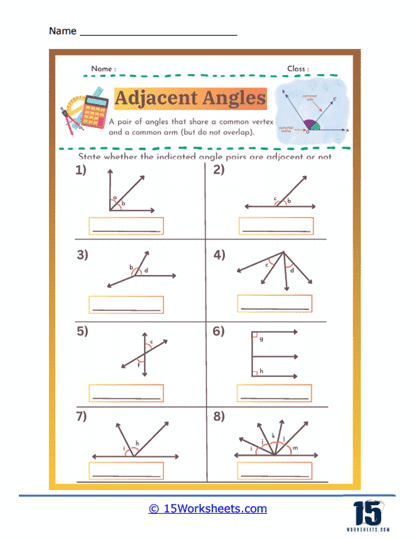
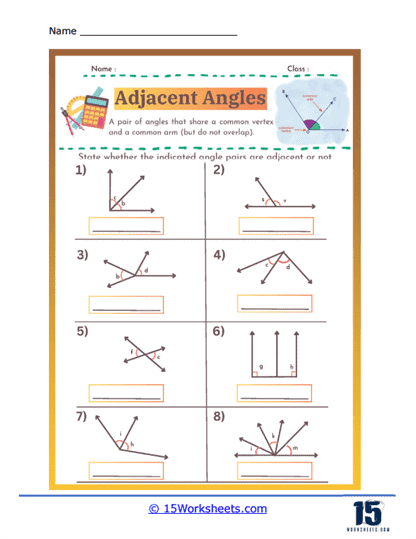
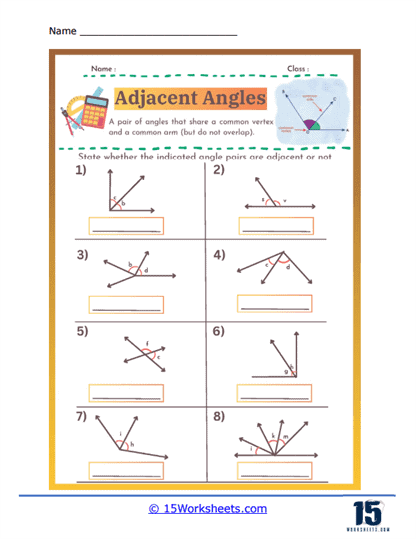
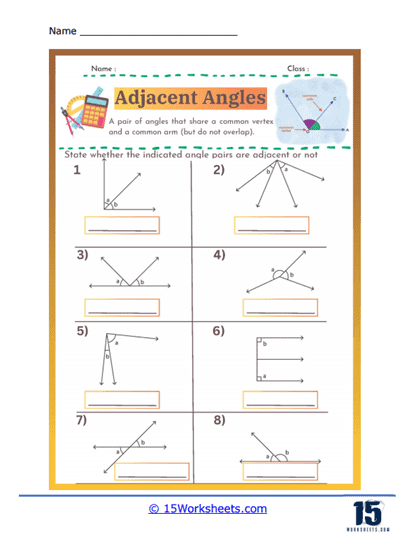
These worksheets will help students understand and identify pairs of angles that share a common vertex and a common side but do not overlap. These worksheets provide structured exercises that guide students through the fundamental concepts of adjacent angles, reinforcing their geometric reasoning and spatial awareness. They typically include a variety of problems, from basic identification tasks to more complex exercises that require analytical thinking and application of geometric principles.
What Are Adjacent Angles?
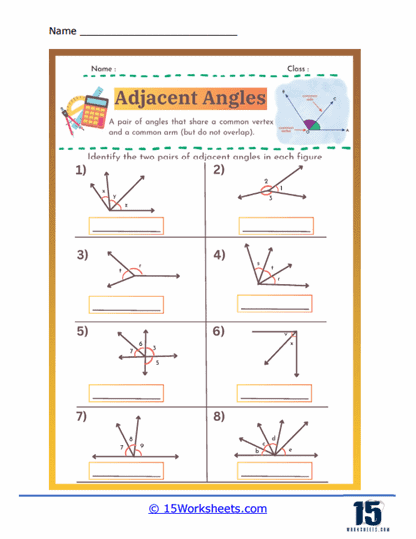
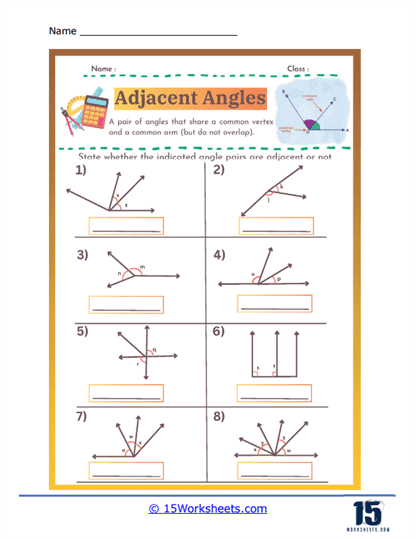
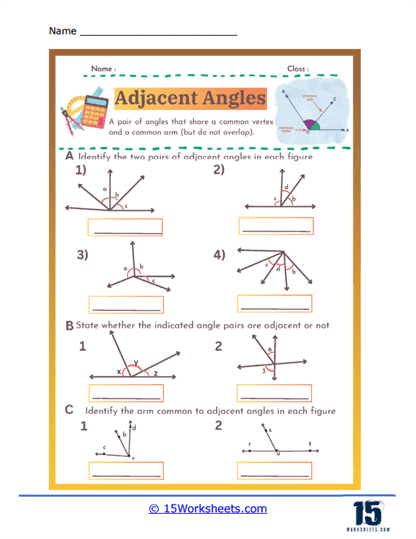
Adjacent angles are pairs of angles that share a common vertex and a common side but do not overlap. These angles are positioned next to each other on a plane, creating a straight line with their non-common sides extending in opposite directions. The sum of adjacent angles that form a linear pair is always 180 degrees, making them supplementary. This property is useful in solving geometric problems and proving theorems related to angles and parallel lines. Understanding adjacent angles is essential for accurately constructing and analyzing geometric shapes. They are widely used in various fields, such as engineering, architecture, and design, where precise angle measurements are necessary. Recognizing adjacent angles aids in developing spatial awareness and problem-solving skills in geometry.
The Math Skills Explored
The primary math skill explored in Adjacent Angles worksheets is the identification and classification of adjacent angles. Students learn to recognize pairs of angles that share a vertex and a side, distinguishing them from other types of angle pairs. This skill is foundational in geometry, as understanding the relationships between angles is crucial for more advanced topics such as the properties of polygons, congruence, and similarity.
In addition to identifying adjacent angles, these worksheets often incorporate other related skills, including:
Angle Measurement – Students practice measuring angles using a protractor, enhancing their precision and accuracy in geometric calculations.
Angle Addition Postulate – Understanding that the sum of adjacent angles forms a larger angle helps students grasp how individual angles contribute to a composite figure.
Complementary and Supplementary Angles – These concepts are often intertwined with adjacent angles, allowing students to explore how angles can be classified based on their sums.
Problem-Solving and Analytical Thinking – Many worksheets include problems that require students to apply their knowledge of adjacent angles to solve puzzles and real-world scenarios, promoting critical thinking.
Types of Exercises
Adjacent Angles worksheets feature a wide range of exercises designed to cater to different learning stages and abilities. Here are some common types of problems you might find:
Identification Exercises – These exercises are fundamental and typically the starting point in Adjacent Angles worksheets. Students are presented with various geometric figures, and their task is to identify and mark pairs of adjacent angles. For instance, a figure might show several intersecting lines, and students need to circle or label all pairs of adjacent angles. This type of exercise reinforces the basic definition and visual identification of adjacent angles.
True or False Questions – True or False questions are straightforward yet effective in assessing students’ understanding. For example, a worksheet might present several angle pairs and ask whether each pair is adjacent. These questions prompt students to apply their knowledge and make quick judgments, reinforcing their conceptual understanding.
Matching Problems – In matching problems, students are given two columns – one with geometric figures and another with descriptions or properties of angles. Their task is to match each figure with the correct description. This type of problem enhances students’ ability to connect visual representations with theoretical concepts, solidifying their understanding of adjacent angles and related properties.
Fill-in-the-Blank – Fill-in-the-blank exercises require students to complete sentences or equations related to adjacent angles. For example, a worksheet might present an incomplete statement like “The angles ___ and ___ are adjacent because they share a common ___.” These exercises test students’ recall and understanding of geometric terminology and relationships.
Angle Measurement – Worksheets often include problems that involve measuring angles with a protractor. Students might be asked to measure given angles and determine if they are adjacent. This practice hones their precision in using geometric tools and reinforces the concept of adjacent angles through hands-on activities.
Angle Addition Problems – Angle addition problems involve scenarios where students must use the Angle Addition Postulate to find unknown angles. For instance, a worksheet might present two adjacent angles and provide the measure of one, asking students to calculate the measure of the second angle. These problems develop students’ ability to work with angle sums and understand the additive nature of adjacent angles.
Real-World Scenarios – Applying geometric concepts to real-world scenarios is a crucial aspect of learning. Adjacent Angles worksheets often include problems that relate to everyday situations. For example, students might be asked to find adjacent angles in architectural designs, road intersections, or various objects. These scenarios make learning more relevant and demonstrate the practical applications of geometry.
Higher-level worksheets include complex problem-solving challenges that require a deeper understanding of adjacent angles and related concepts. These might involve multi-step problems where students must identify adjacent angles, measure them, and apply their knowledge to find missing values or solve geometric puzzles. Such challenges promote critical thinking and analytical skills, preparing students for advanced mathematical studies.
Benefits of These Worksheets
Using Adjacent Angles worksheets offers several educational benefits that go beyond simple practice and repetition. These worksheets serve as valuable tools in enhancing various aspects of students’ mathematical abilities and cognitive skills. Here are some of the key advantages:
Reinforces Foundational Geometry Concepts
Adjacent Angles worksheets provide a structured approach to learning fundamental geometry concepts. By concentrating on adjacent angles, students build a strong foundational knowledge that is crucial for understanding more complex geometric relationships and properties. These worksheets help students grasp the basic principles of angles, their measurement, and their interaction within geometric figures. This foundational knowledge is essential as students progress to more advanced topics in geometry, such as theorems involving parallel lines and polygons.
Enhances Spatial Reasoning
Identifying and working with adjacent angles requires students to visualize geometric shapes and comprehend spatial relationships. This practice significantly enhances their spatial reasoning abilities, a critical skill not only in mathematics but also in fields such as engineering, architecture, and computer graphics. By regularly engaging with problems that involve visualizing and manipulating geometric figures, students develop the ability to think in three dimensions and understand how different shapes interact in space.
Promotes Precision and Accuracy
Measuring angles and working with geometric figures demand precision and accuracy. Adjacent Angles worksheets provide ample opportunities for students to practice using protractors and other geometric tools. This repeated practice helps students develop a high level of precision and accuracy, which are crucial skills for success in various academic and professional fields. By mastering the use of these tools, students learn to approach problems methodically and with attention to detail.
Encourages Critical Thinking
Problem-solving exercises and real-world scenarios included in Adjacent Angles worksheets encourage students to think critically and apply their knowledge in various contexts. These exercises promote analytical thinking and problem-solving abilities, skills that are invaluable in both academic and real-world settings. By facing challenging problems that require them to apply geometric principles, students learn to approach complex situations logically and creatively.
Provides Hands-On Learning
Many Adjacent Angles worksheets incorporate hands-on activities, such as measuring angles and constructing geometric figures. These activities actively engage students in the learning process, making geometry more interactive and enjoyable. Hands-on learning helps students to better retain information and understand abstract concepts by applying them in tangible ways. This engagement fosters a deeper appreciation for the subject and enhances overall learning outcomes.
Demonstrates Real-World Applications
Adjacent Angles worksheets often include real-world examples that show the practical applications of geometric concepts. For instance, consider a scenario where a student is asked to design a simple garden layout with several pathways intersecting at different angles. Using their knowledge of adjacent angles, the student can accurately measure and label the angles at each intersection. This ensures that the pathways meet at precise angles, creating a well-organized and visually appealing garden layout.
In this real-world example, the student applies their understanding of adjacent angles to a practical task, utilizing tools like a protractor to ensure accuracy. This exercise highlights the relevance of geometric concepts in everyday life and underscores the importance of learning about adjacent angles. It demonstrates how geometric principles are not just theoretical but have tangible applications that can enhance the quality of our surroundings and contribute to various professional fields.
Real-World Applications of Adjacent Angles
The concept of adjacent angles is not just confined to the pages of mathematics textbooks; it finds practical applications in numerous real-world situations. Understanding and using adjacent angles is fundamental in various fields, from construction and design to technology and everyday problem-solving.
Architecture and Construction
In architecture and construction, adjacent angles are crucial in designing and constructing buildings and other structures. Architects and engineers must accurately measure and use adjacent angles to ensure that the corners and joints of a structure are precise and secure. For example, when designing floor plans, the angles between walls must be measured and constructed accurately to ensure that rooms are perfectly shaped and the overall structure is stable. This precision is essential for the safety and aesthetic quality of buildings.
Interior Design
Interior designers frequently use the concept of adjacent angles when arranging furniture and other elements within a space. For instance, when placing furniture in a room, designers must consider the angles at which pieces intersect to maximize space usage and create visually appealing arrangements. This involves measuring the angles between walls, furniture, and other objects to ensure a harmonious and functional layout. Accurate angle measurements help designers create spaces that are both practical and aesthetically pleasing.
Engineering and Manufacturing
In engineering and manufacturing, adjacent angles play a vital role in the design and assembly of various components. Mechanical engineers, for example, must understand how different parts of a machine fit together at precise angles to ensure smooth operation and efficiency. In the automotive industry, the alignment of parts like the steering mechanism or suspension system often relies on accurate measurements of adjacent angles. This precision ensures that the machinery operates correctly and efficiently, reducing wear and tear and improving overall performance.
Carpentry and Woodworking
Carpenters and woodworkers regularly use adjacent angles when cutting and joining pieces of wood. When creating furniture, cabinets, or other wooden structures, they must measure and cut wood at specific angles to ensure that pieces fit together seamlessly. For instance, when constructing a picture frame, the angles between the sides must be precisely measured and cut to form perfect corners. Mastery of adjacent angles allows woodworkers to produce high-quality, durable pieces with tight, precise joints.
Urban Planning and Landscaping
Urban planners and landscape designers use adjacent angles to design efficient and visually pleasing layouts for public spaces, parks, and gardens. When planning pathways, roads, and intersections, they must consider the angles at which these elements meet to ensure smooth traffic flow and aesthetic appeal. For example, the layout of a garden with intersecting pathways requires careful measurement of adjacent angles to create a harmonious and functional design. Accurate angle measurements help planners and designers create spaces that are safe, accessible, and enjoyable for the public.
Technology and Computer Graphics
In technology and computer graphics, adjacent angles are used in the creation of digital images, animations, and models. Graphic designers and animators rely on geometric principles, including adjacent angles, to create realistic and proportionate visuals. For example, when designing a 3D model, understanding how adjacent angles affect the shape and appearance of the object is crucial for creating lifelike representations. This knowledge is also essential in programming computer algorithms that generate images and animations.
Everyday Problem-Solving
Even in everyday problem-solving, understanding adjacent angles can be beneficial. For instance, when hanging a picture on a wall, measuring the angles between the picture, the wall, and surrounding objects ensures that the picture is level and positioned correctly. Similarly, when arranging furniture or planning a home renovation, knowing how to measure and use adjacent angles can help create functional and aesthetically pleasing spaces. This practical application of adjacent angles demonstrates their relevance in day-to-day activities.