Angle Bisectors Worksheets
About These 15 Worksheets
These worksheets will help students understand and apply the concept of bisecting angles. These worksheets are an essential part of the geometry curriculum and serve as a hands-on method for students to learn how to divide angles into two equal parts. By engaging with these worksheets, students develop a deeper understanding of geometric principles and improve their spatial reasoning skills. Angle bisectors worksheets typically include a variety of problems that range from basic constructions to more complex applications involving different geometric shapes and real-world contexts.
These worksheets usually begin with simple exercises where students are asked to draw the bisector of a given angle using a protractor and a ruler. As students progress, the problems become more challenging, requiring them to apply their understanding of angle bisectors to solve problems within triangles, quadrilaterals, and other polygons. Some worksheets may also integrate real-life scenarios where angle bisectors are used, helping students see the practical applications of what they are learning.
What Are Angle Bisectors?
An angle bisector is a line or ray that divides an angle into two equal smaller angles, each having the same measure. It originates from the angle’s vertex and cuts through the interior, ensuring the two resulting angles are congruent. The concept is crucial in geometry for constructing precise angles and triangles. When applied to a triangle, the angle bisectors of all three interior angles meet at a single point known as the incenter. This incenter is equidistant from all sides of the triangle, making it the center of the triangle’s inscribed circle. Angle bisectors are used in various geometric proofs and constructions, highlighting their importance in understanding and manipulating geometric shapes. Their properties aid in solving complex problems and play a significant role in the study of symmetry and congruence in geometry.
Math Skills Explored
Angle bisectors worksheets explore several fundamental math skills that are crucial for students’ overall understanding of geometry and their ability to solve complex mathematical problems. These skills include:
Constructing Angle Bisectors – Students learn how to use geometric tools such as protractors and compasses to construct angle bisectors accurately. This skill is foundational in geometry and helps students understand the precision required in mathematical constructions.
Understanding Angle Properties – By working with angle bisectors, students gain a deeper understanding of the properties of angles, including the concept that a bisector divides an angle into two equal parts. This knowledge is essential for solving various geometric problems.
Symmetry and Congruence – Angle bisectors help students understand the concepts of symmetry and congruence. When an angle is bisected, the two resulting angles are congruent, reinforcing the idea of equal measures and balance within geometric figures.
Problem-Solving and Analytical Thinking – These worksheets require students to apply their knowledge of angle bisectors to solve problems. This process enhances their problem-solving and analytical thinking skills, as they must determine the steps needed to construct bisectors and solve related problems accurately.
Spatial Reasoning – Working with angle bisectors helps students improve their spatial reasoning skills. They learn to visualize geometric figures, understand their properties, and manipulate them mentally, which is essential for success in higher-level mathematics and related fields.
Types of Exercises
Angle bisectors worksheets feature a wide range of exercises and practice problems designed to reinforce students’ understanding of the concept and help them master the related skills. Some common types of exercises include:
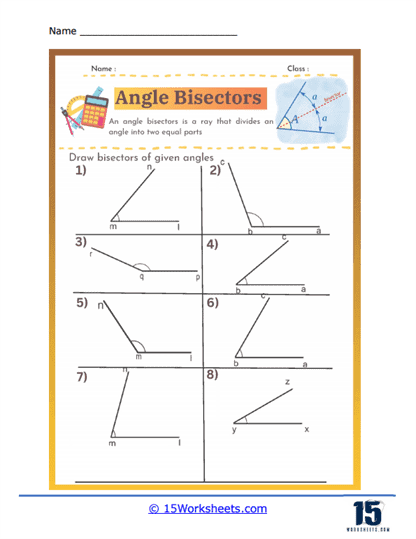
Basic Construction Exercises – These exercises introduce students to the concept of angle bisectors. Students are given various angles and are asked to construct the bisector for each angle using a protractor and a ruler. This helps them understand the basic idea of dividing an angle into two equal parts.
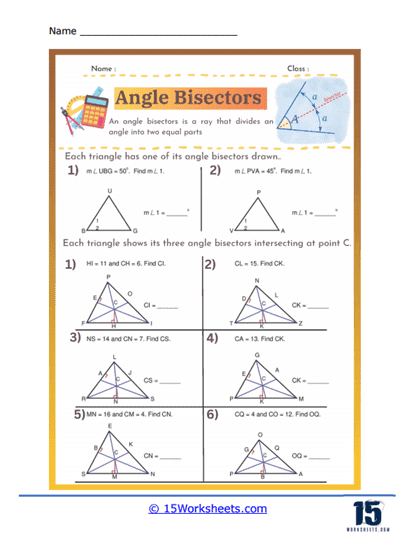
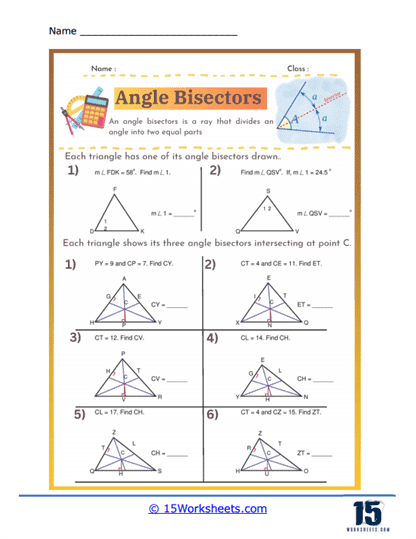
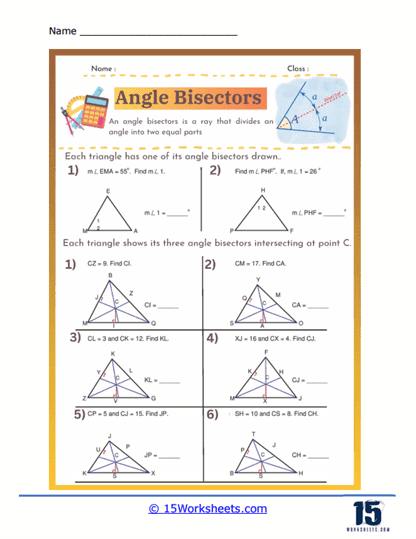
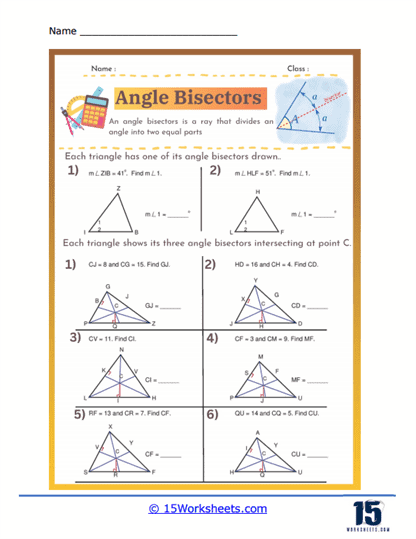
Angle Bisectors in Triangles – These problems require students to find and construct the angle bisectors within different types of triangles. Students might be asked to draw all three angle bisectors of a triangle and identify the point where they intersect, known as the incenter, which is equidistant from all sides of the triangle.
Angle Bisectors in Quadrilaterals and Polygons – As students progress, they encounter problems involving angle bisectors in more complex shapes, such as quadrilaterals and other polygons. These exercises help students understand how angle bisectors function in different geometric contexts and reinforce their understanding of geometric properties.
Real-Life Application Problems – Some worksheets include problems that involve real-life scenarios where angle bisectors are used. For example, students might be asked to determine the optimal path for a robot to take by bisecting the angles formed by obstacles. These problems help students see the practical applications of angle bisectors and understand their importance in various fields.
Interactive and Collaborative Exercises – Some worksheets incorporate interactive or collaborative exercises where students work in pairs or groups to construct angle bisectors and solve related problems. This type of activity fosters teamwork and communication skills while reinforcing geometric concepts.
Integration with Other Geometric Concepts – Angle bisectors worksheets often integrate other geometric concepts such as perpendicular bisectors, medians, and altitudes. This helps students see the connections between different geometric principles and understand how they work together to solve complex problems.
Benefits of These Worksheets
Angle bisectors worksheets offer numerous benefits for students, teachers, and the overall learning process. These worksheets not only enhance geometric understanding but also build practical skills and foster critical thinking. Here are the expanded benefits of incorporating angle bisectors worksheets into educational settings:
Enhanced Understanding of Geometry
Angle bisectors worksheets provide a structured and systematic approach for students to learn about angle bisectors and their properties. By working through a variety of problems, students gain a deeper understanding of geometric concepts and improve their ability to apply these concepts to solve problems. These worksheets help students grasp the significance of angle bisectors in dividing angles into two equal parts, which is foundational for understanding more complex geometric relationships.
Development of Practical Skills
Constructing angle bisectors requires the use of geometric tools such as protractors and compasses. By practicing these constructions, students develop practical skills that are essential for success in geometry and other related fields. The hands-on experience with these tools enhances students’ manual dexterity and precision, preparing them for tasks that require meticulous attention to detail in both academic and professional contexts.
Improved Problem-Solving Abilities
Angle bisectors worksheets challenge students to think critically and solve problems, thereby enhancing their problem-solving abilities. This process helps students develop analytical thinking skills needed to tackle complex mathematical problems. By encountering various scenarios where angle bisectors must be applied, students learn to approach problems methodically and think logically to find solutions.
Reinforcement of Key Concepts
These worksheets reinforce key geometric concepts such as symmetry, congruence, and the properties of angles. By repeatedly applying these concepts in different contexts, students solidify their understanding and improve their ability to recall and use these principles when needed. Consistent practice with angle bisectors helps students internalize these fundamental ideas, making them more adept at recognizing and applying geometric principles in various situations.
Preparation for Advanced Mathematics
Understanding and working with angle bisectors is foundational for success in higher-level mathematics. By mastering these skills, students are better prepared for more advanced topics such as trigonometry, calculus, and various branches of engineering and physics. A strong grasp of angle bisectors equips students with the necessary tools to excel in more complex mathematical disciplines, providing a solid foundation for future academic and professional pursuits.
Application to Real-World Problems
Many angle bisectors worksheets include problems that involve real-world applications. This helps students see the relevance of what they are learning and understand how geometric principles are used in everyday life and various professions. For example, architects and engineers use angle bisectors to ensure that structures are symmetrical and stable. Real-world examples in worksheets demonstrate the practical utility of geometric concepts, making learning more meaningful and engaging for students.
Engagement and Motivation
These worksheets often include a variety of problem types and interactive exercises that keep students engaged and motivated. By providing a range of challenges, they cater to different learning styles and help students stay interested in the subject. Engaging activities and real-world applications make learning about angle bisectors enjoyable and relevant, encouraging students to actively participate and persist in their studies.
Real-World Applications of Angle Bisectors
Angle bisectors, which divide an angle into two equal parts, have numerous practical applications in the real world. Understanding and using angle bisectors is essential in fields such as architecture, engineering, design, navigation, and various branches of science. Here are some examples of how angle bisectors are applied in real-world scenarios:
Architecture and Engineering
Architects and engineers use angle bisectors to ensure that structures are symmetrical and stable. When designing a building, the precise bisecting of angles helps in creating equal and balanced sections, which is crucial for both aesthetics and structural integrity. In civil engineering projects, such as constructing roads and bridges, angle bisectors are used to design accurate and safe pathways and structures. These precise measurements are essential for maintaining the safety and functionality of infrastructure.
Everyday Activities
Students might come across angle bisectors in various everyday activities that require precision and symmetry. For example, when cutting a piece of cake or pizza into equal slices, using the concept of angle bisectors ensures that each piece is the same size. This practical application helps students understand the importance of equal division in real life. Similarly, in art projects, creating symmetrical shapes and patterns requires an understanding of angle bisectors, enhancing students’ creativity and spatial awareness.
Sports and Strategy
The skills related to understanding and using angle bisectors are foundational for developing spatial awareness and geometric reasoning. These skills help students visualize and manipulate shapes and angles, which is essential for problem-solving in various contexts. In sports, understanding angles and their bisectors can improve strategies and performance. For instance, a soccer player might use the concept of angle bisectors to determine the best angle for taking a shot on goal, ensuring that it is well-aimed and difficult for the goalkeeper to reach. This strategic use of angles can make a significant difference in the outcome of a game.