Least Common Multiples Worksheets
About These 15 Worksheets
This collection of Least Common Multiple (LCM) worksheets serves as a powerful tool for building foundational mathematical skills, targeting both conceptual understanding and practical applications. Each worksheet is designed to engage students with a range of activities that progressively develop their proficiency in identifying, calculating, and applying the least common multiple in various mathematical contexts.
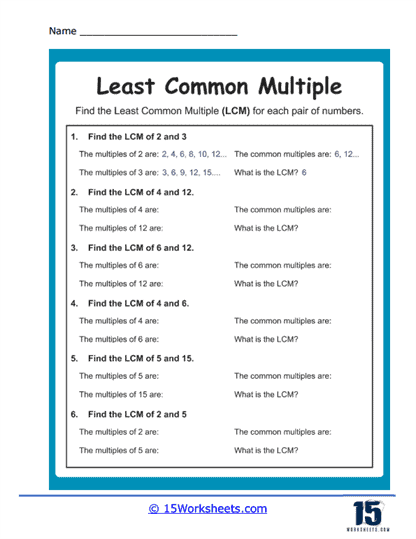
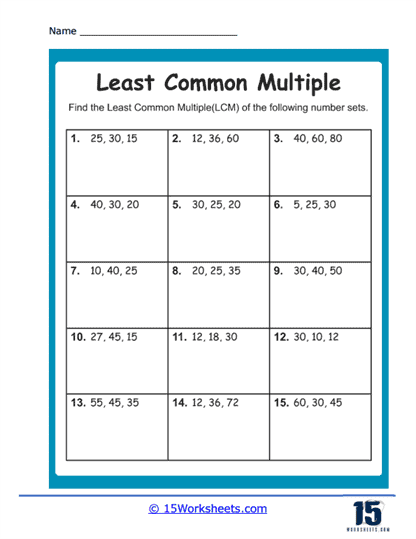
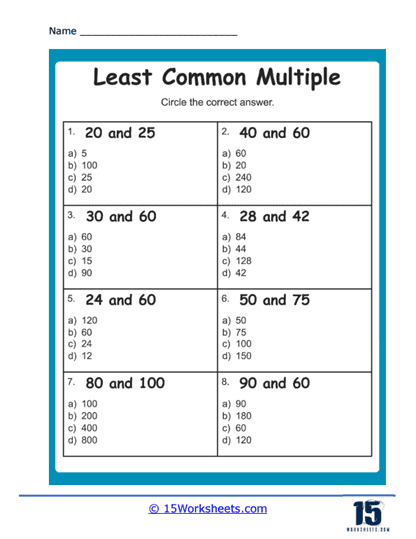
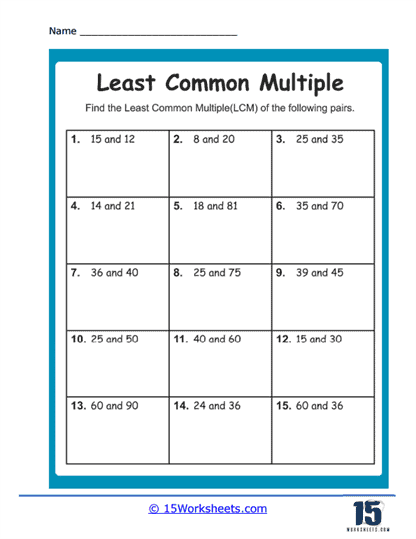
At its core, the collection emphasizes mastery of basic LCM computation, which is an essential building block for more advanced mathematical operations such as working with fractions, ratios, and solving real-world problems. The exercises in the worksheets encourage students to find the LCM of two or more numbers, a skill that goes beyond mere rote memorization and pushes students to recognize patterns and relationships between numbers. The act of finding common multiples reinforces the idea that numbers are part of a larger numerical system, fostering number sense and a deeper understanding of arithmetic.
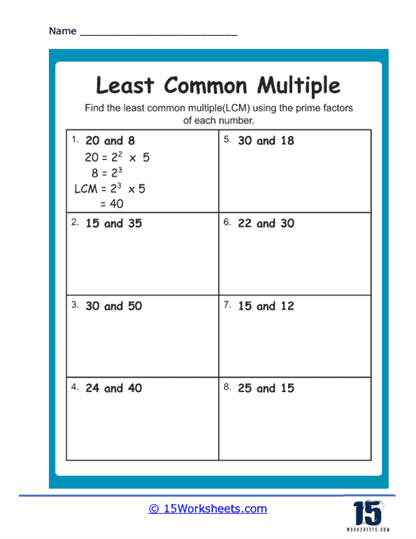
Another critical component of this collection is the focus on prime factorization as a method for determining the LCM. Worksheets that guide students through the process of breaking numbers down into their prime factors help develop a more nuanced understanding of the structure of numbers. This skill is indispensable not only for finding the LCM but also for strengthening students’ knowledge of factors and multiples, both of which are fundamental to higher-order mathematical topics like algebra and number theory. Prime factorization exercises also bridge the gap between simple multiplication and more complex concepts like the greatest common divisor (GCD), making this collection an excellent preparatory resource for a variety of mathematical challenges.
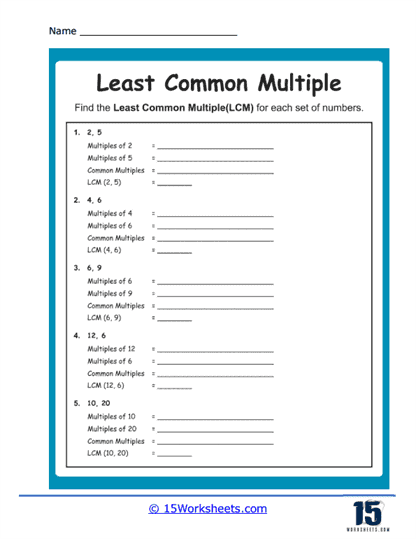
The collection also shines in its ability to foster visualization and comparison of multiples. Many worksheets prompt students to list out multiples of numbers and compare them to identify the least common multiple. This not only reinforces computational fluency but also encourages students to develop strategic thinking. By repeatedly engaging with numerical patterns, students become more adept at recognizing similarities and differences between numbers, which is a crucial skill for higher mathematics and real-life problem solving.
To complement these exercises, the inclusion of step-by-step guided examples is invaluable, especially for students who may struggle with understanding abstract concepts. These guided sections break down the process of finding the LCM into digestible steps, helping students build confidence as they follow a logical progression of thought. This explicit instruction not only reinforces procedural fluency but also nurtures critical thinking and attention to detail-skills that are transferable across all areas of learning.
As LCM is critical for adding and subtracting fractions with unlike denominators, worksheets that integrate LCM into fraction operations would be an ideal next step. This would reinforce the practical utility of LCM while simultaneously improving students’ proficiency with fractions. Exercises that require students to use the LCM to compare and order fractions would give students yet another application of the concept. By finding common denominators through LCM, students can develop a clearer understanding of fraction relationships.
One of the standout features of this collection is its incorporation of word problems and real-life applications, which extend students’ understanding of LCM beyond the confines of the classroom. These problems challenge students to apply their LCM knowledge to practical situations, such as organizing schedules, planning events, or calculating rates. By connecting mathematical concepts to real-world contexts, students begin to see the relevance and utility of the skills they are learning, which can enhance motivation and retention.
How to Find the Least Common Multiple
The Least Common Multiple (LCM) is the smallest number that is a multiple of two or more numbers. It’s like finding the next common point where two or more numbers “meet” when you list out their multiples.
Here’s a little metaphor: Imagine you and a friend are running around a track. You’re both running at different speeds, but eventually, you will both end up at the same spot again. That spot is like the LCM!
Let’s break down the process in an engaging way, with an easy step-by-step approach!
Method: Prime Factorization
A classic method to find the LCM is Prime Factorization. In this method, you break each number down into its prime factors and use the highest powers of all primes involved.
Step-by-Step Example #1: Let’s find the LCM of 12 and 15.
12 = 22 x 3
15 = 3 x 5
List all prime factors: The prime factors are 2, 3, and 5.
Take the highest powers:
For 2, the highest power is 22 (from 12).
For 3, the highest power is 31 (appears in both, but the highest is still just 3).
For 5, the highest power is 51 (from 15).
Multiply these together:
LCM = 22 x 3 x 5 = 4 x 3 x 5 = 60.
So, the LCM of 12 and 15 is 60.
This means that 60 is the smallest number both 12 and 15 divide into evenly.
Step-by-Step Example #2: Now, let’s try to find the LCM with three numbers: 8, 9, and 12.
8 = 23
9 = 32
12 = 22 x 3
List all prime factors: The prime factors are 2 and 3.
Take the highest powers:
For 2, the highest power is 23 (from 8).
For 3, the highest power is 32 (from 9).
Multiply these together:
LCM = 23 x 32 = 8 x 9 = 72.
So, the LCM of 8, 9, and 12 is 72.
This means that 72 is the smallest number that all three numbers (8, 9, and 12) divide into evenly.
The Real World Applications of the Least Common Multiple (LCM)
The concept of Least Common Multiple (LCM) plays a significant role in everyday situations, particularly in scheduling, time management, and planning. One of the most common real-world applications is in coordinating events or activities that occur at regular intervals. For example, if two buses arrive at different intervals, say one every 12 minutes and another every 20 minutes, the LCM of 12 and 20 tells us when both buses will arrive at the station simultaneously. In this case, the LCM is 60, meaning both buses will arrive together every 60 minutes. This concept helps in organizing schedules in a way that minimizes conflicts or inefficiencies.
LCM is also useful in tasks that involve repeating actions over time, such as maintenance or restocking in a business. For instance, if a factory needs to service a machine every 6 weeks and restock supplies every 9 weeks, finding the LCM of 6 and 9 will help in determining when both tasks can be performed simultaneously, optimizing time and resources. The LCM ensures that operations are streamlined by identifying the earliest time both events coincide, which in this case would be every 18 weeks. This is particularly beneficial in fields where coordination is key to reducing downtime and ensuring efficient use of resources.
In financial contexts, LCM can be applied to problems involving periodic payments or billing cycles. For example, if someone has two different loan payment schedules, one requiring payment every 15 days and another every 20 days, calculating the LCM helps in determining when both payments will fall on the same day, making it easier to plan for those financial obligations. This helps individuals manage their cash flow better, ensuring they are prepared for overlapping financial commitments without surprises.
The concept of LCM is also critical in computing, especially in managing data transmission and digital signals. Devices that operate on different frequencies or cycles need synchronization to function efficiently together. For example, if two devices transmit data at intervals of 8 milliseconds and 12 milliseconds, the LCM helps determine the optimal point where they can synchronize without collision, improving data flow and reducing errors. The concept of LCM, therefore, provides solutions for managing periodic events, optimizing schedules, and coordinating actions in a wide variety of real-world scenarios.