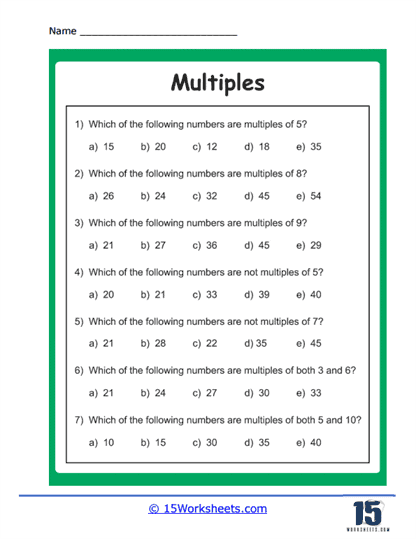
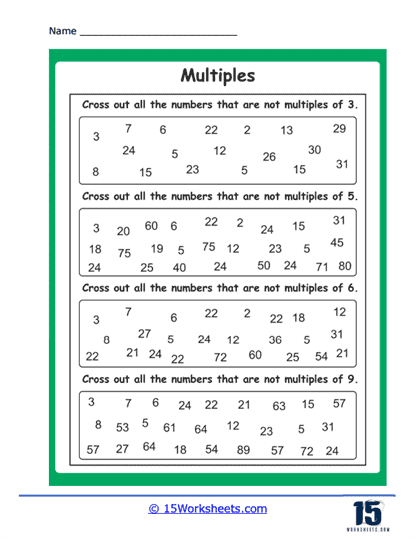
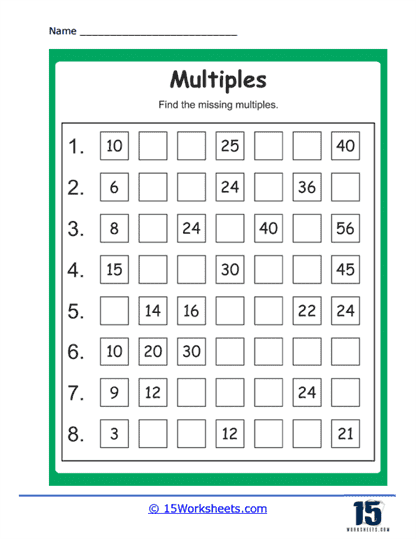
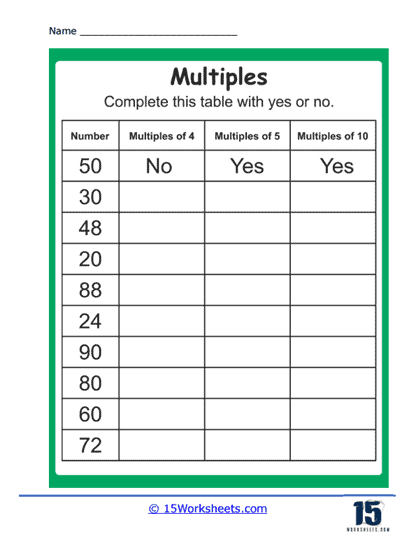
Multiples Worksheets

About These 15 Worksheets

Multiples can feel a little slippery at first-like numbers that just keep stretching out forever. This set of worksheets takes that big idea and breaks it into bite-sized, approachable activities. Kids get to hunt for multiples, fill in missing ones, and even color their way through number patterns, which makes the practice way more fun than just rote memorization.

Each worksheet zooms in on a slightly different angle-finding common multiples, spotting even ones, or testing with yes/no questions-so students build a flexible understanding. Instead of just memorizing lists, they’re learning to think about how multiples work in different situations. That means more chances for kids to see the “big picture” of multiplication.

And the best part? These activities aren’t just drills; they’re like mini-games and puzzles that encourage kids to explore. Along the way, learners build confidence, sharpen their multiplication recall, and get ready for more advanced ideas like least common multiples and fractions. It’s math practice that feels both useful and engaging.

Have a Look Inside Each Worksheet

Multiples Between
This worksheet asks students to find the multiples that fall between two numbers. It’s like filling in the “in-between” steps on a math number line. The activity encourages careful counting and attention to sequence. It helps learners strengthen their understanding of multiples by seeing how they fit inside ranges.

First 5 Multiples
Students will list the first five multiples of a given number. It’s a simple, confidence-boosting activity that gets them warmed up on the basics. Like collecting the first five “fans” of a number, it’s straightforward and easy to follow. It’s a solid foundation exercise for multiplication fluency.

Even Multiples
Here, learners focus only on multiples that are even. It’s like shining a spotlight on one side of the number world. Students practice filtering results and recognizing patterns within multiples. It reinforces multiplication and division skills while narrowing their focus.

Groups of Numbers
Students group sets of numbers by their multiples in this worksheet. It’s like sorting socks into matching pairs, but with math. The activity strengthens organizational thinking and classification. It helps kids see the bigger picture of how multiples connect different numbers.

Three Common Multiples
This worksheet challenges learners to find three multiples that numbers share. It’s a “find what they have in common” kind of puzzle. Students build logical thinking by comparing and cross-checking lists. It reinforces how multiples overlap and prepares them for LCM work.

Missing Multiples
Students fill in the blanks with the missing multiples. It’s a math version of a fill-in-the-gaps game. The task keeps them alert and strengthens sequential number skills. It makes them more confident in recalling and predicting multiplication patterns.

Color Grids
Here, multiples are highlighted or colored within a grid. It’s a hands-on visual activity that feels more like art class than math. Kids enjoy the puzzle-like challenge of spotting patterns. It reinforces recognition of multiples in a creative, visual way.

Visual Multiples
This worksheet presents multiples in diagrams or pictures. Students “see” the multiples instead of just listing them. It adds another layer of learning by connecting visuals to numeric patterns. Perfect for visual learners who need more than plain numbers.

Multiple Choice
Students pick the correct multiples from a set of options. It’s a quick-check quiz style that builds confidence. Learners practice discrimination-spotting correct answers among distractors. It’s an easy way to reinforce skills while keeping things light.

Cross Out All
Kids must cross out numbers that aren’t multiples in a group. It’s like playing detective, eliminating the imposters. The task sharpens critical thinking and accuracy. It reinforces what a true multiple looks like.

Rows of Multiples
This worksheet organizes multiples into rows for easy scanning. It feels a little like filling a multiplication table. Students practice systematic listing and pattern recognition. It strengthens fluency by reinforcing structure in multiples.

Yes or No
Students decide if a number is or isn’t a multiple of another. It’s like giving each number a ticket: in or out of the multiples club. This builds fast recognition and recall. It deepens understanding by making learners justify their choices.

Multiple Matrix
Learners work with a grid or matrix to spot multiples. It’s like solving a math crossword puzzle. This helps with cross-referencing and strengthens mental math agility. The activity is both logical and a bit playful.

1st Five
Similar to “First 5 Multiples,” this worksheet asks students to identify the first few multiples of numbers. It’s a basic but essential exercise. The repetition helps cement multiplication facts. It’s ideal for daily practice and skill building.

In the Times Table Worksheet
This activity ties multiples directly to times tables practice. It’s a way of showing students that multiples and times tables are really two sides of the same coin. It makes connections crystal clear and strengthens recall. Perfect for bridging multiplication drills with multiples work.

What is a Multiple?

In mathematics, a multiple of a number is the product obtained when that number is multiplied by an integer. Simply put, if you have a number
a and you multiply it by any integer, the result is a multiple of a. For example, the multiples of 3 are found by multiplying 3 by different integers, such as 3 x 1 = 3, 3 x 2 = 6, 3 x 3 = 9, and so on. Multiples can be positive, negative, or zero depending on the integer used in the multiplication. It’s important to note that every number is a multiple of itself because multiplying a number by 1 yields the number itself.

Characteristics of Multiples

One key feature of multiples is that they continue infinitely. This is because there are an infinite number of integers to multiply by. For any number a, there is no limit to how large or small its multiples can be. Moreover, all multiples of a given number are evenly divisible by that number. For example, any multiple of 5 is divisible by 5 without leaving a remainder. This property helps in solving problems involving divisibility, common multiples, and greatest common divisors.

Example 1: Multiples of 4

Let’s take 4 as an example. The multiples of 4 are obtained by multiplying 4 by 1, 2, 3, 4, etc. So, the multiples of 4 are:

4 x 1 = 4

4 x 2 = 8

4 x 3 = 12

4 x 4 = 16

4 x 5 = 20

These multiples go on indefinitely. Each of these numbers can be divided by 4, confirming that they are indeed multiples. Additionally, if we consider negative integers, the multiples of 4 can also include negative numbers like 4 x -1 = -4, 4 x -2 =-8 and so on.

Example 2: Multiples of 7

Let’s consider the number 7. To find its multiples, we multiply 7 by positive and negative integers. The first few multiples of 7 are:7 x 1=7,7 x 2=14,7 x 3=21,7 x 4=28,7 x 5=35

As with 4, the multiples of 7 extend infinitely in both positive and negative directions. For instance, 7 x -1 = -7 and 7 x -2 = -14. Again, each of these multiples is divisible by 7.

Common Multiples

Sometimes, we are interested in the multiples that two or more numbers share. These are called common multiples. For instance, 4 and 6 have common multiples because some numbers, like 12 and 24, are multiples of both. Finding the least common multiple (LCM) of two numbers can help solve problems related to synchronization or matching quantities, like when two events need to occur at the same time in periodic intervals.