Greatest Common Factors Worksheets
About These 15 Worksheets
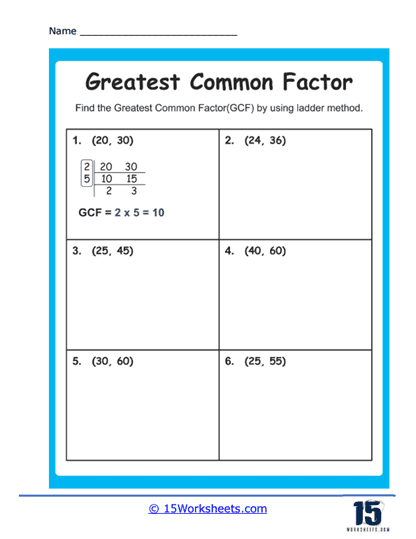
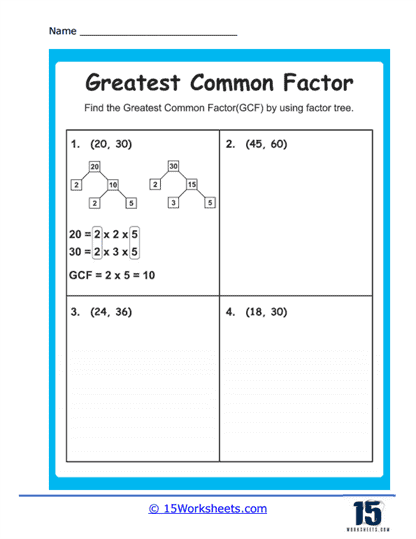
At the heart of many mathematical problems lies the need to find commonality between numbers. The Greatest Common Factor serves this exact purpose. Picture two numbers in your mind, perhaps 12 and 16. Now, if we were to take a deep dive into both these numbers, we’d be looking for numbers that can cleanly divide both 12 and 16. In this instance, those numbers turn out to be 1, 2, and 4. The largest of these divisors is 4, which signifies that the GCF of 12 and 16 is 4. Simple, right?
However, as is often the case with math, simple ideas lay the groundwork for deeper, more complex problems. This foundational knowledge is essential, and mastering it will make future problems less daunting.
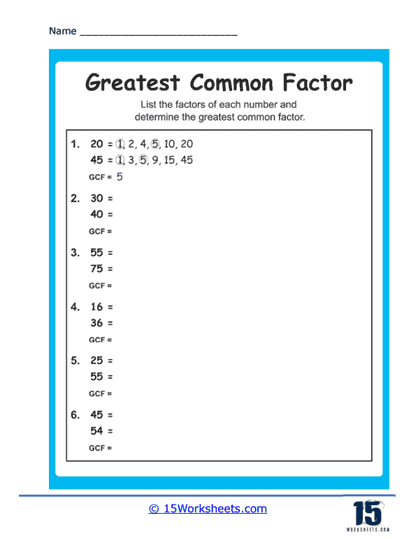
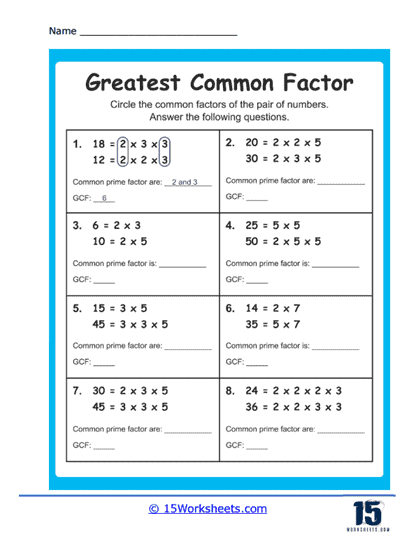
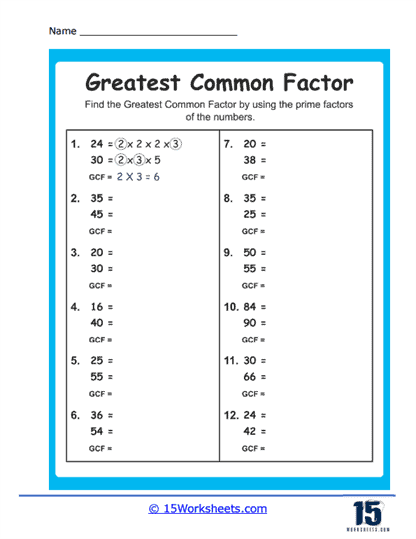
Imagine a sheet filled to the brim with math exercises. Each exercise presents a puzzle, beckoning you to unlock its mysteries. The primary goal? Sharpening your skill in locating the GCF amidst a set of numbers. These worksheets can take multiple forms, but the essence remains the same – equipping you with the expertise to effortlessly determine the GCF.
The Types Of Problems On These Worksheets
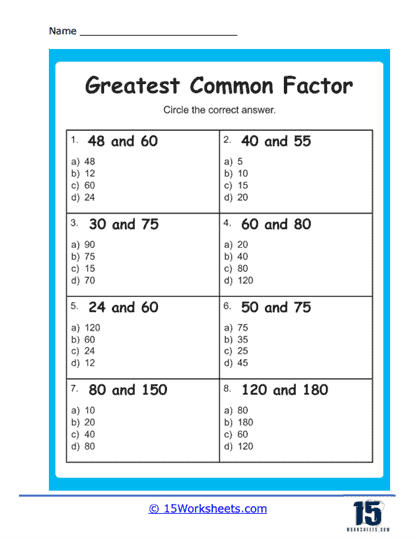
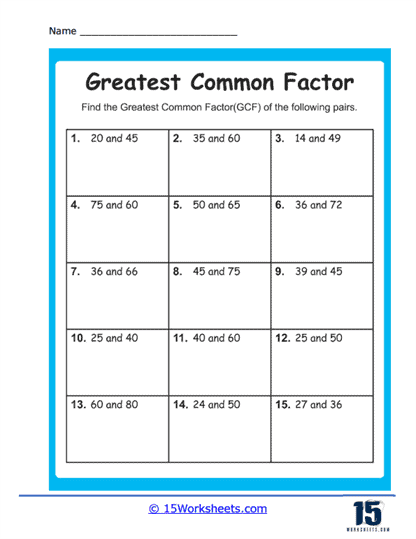
Basic GCF Problems – These are your initial stepping stones. They might look something like this – “Find the GCF of 14 and 28.” It’s direct, and it serves to cement your foundational knowledge.
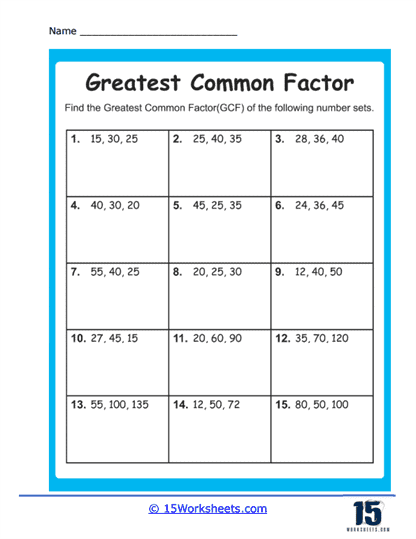
Multiple Numbers – Moving up the complexity ladder, you might be presented with not two, but perhaps three or even more numbers. A typical question could be – “Determine the GCF of 15, 30, and 45.” This nudges you to think a bit harder and find the common factor linking all these numbers.
Word Problems – Suddenly, numbers transform into stories, each narrating a tale or painting a scenario. A fun example might read – “Amy has 16 apples. She’s throwing a party for 8 of her friends and wants each of them to have an equal share of apples, without cutting any apple. How many apples will each friend get?” Such problems merge real-life situations with mathematical concepts, making them both challenging and relatable.
GCF with Variables – As you gain proficiency, you’ll start seeing letters dancing amidst the numbers. These letters, often x, y, or z, are termed as variables. A typical problem could be – “Determine the GCF of 6x and 9x.” Here, the GCF isn’t just about numbers but involves the mysterious variables too.
Factoring Expressions – To further enhance your skills, some worksheets present algebraic expressions and challenge you to tease out the GCF. For instance, a question might prompt you to “Factor out the GCF from the expression 12x^2 + 16x.”
The Benefits of These Worksheets
It’s one thing to learn a concept; it’s another to master it. Mastery comes with consistent practice and a determination to understand not just the ‘how’, but also the ‘why’. The GCF worksheets serve this exact purpose.
With each problem you solve, you’re not just getting an answer. You’re evolving your problem-solving acumen, learning how to tackle challenges systematically. As you ascend to higher mathematical echelons, topics like factoring polynomials will cross your path. With a robust understanding of GCF, these advanced topics will seem less intimidating.
Over time, as you consistently engage with these worksheets, two things happen. Firstly, the time you take to solve problems diminishes. You become faster. Secondly, you start making fewer mistakes. Your accuracy soars. But beyond these tangible benefits, there’s a subtle yet profound transformation. Your confidence in your mathematical abilities burgeons. Each solved problem, each correct answer, adds a brick to the edifice of your self-belief.
How Does This Skill Help Your Everyday?
Sharing Equitably – Have you ever found yourself amidst a conundrum, wondering how to equitably share a batch of cookies with your friends? The GCF elegantly solves this dilemma, ensuring everyone gets an equal share without breaking any cookie.
Organizing Events – If you’ve ever hosted a party, you’ll appreciate the challenges in arranging seating. The GCF can aid in perfecting such arrangements, ensuring every table has an equal number of chairs.
Culinary Adventures – Cooking and baking, at their core, are a blend of art and science. When adjusting recipes for different serving sizes, the GCF becomes an invaluable tool, ensuring the ingredients scale proportionately.
Crafting Wonders – Engaging in arts and crafts projects often requires patterns. If you’re designing with beads, the GCF can guide you in choosing the perfect number to maintain your pattern.
Eco-Conscious Decisions – As we navigate a world grappling with climate change, recycling and waste management become pivotal. Products often come in bundles. The GCF can guide us in understanding how many items can be optimally recycled or repurposed, reducing waste.