List All Factors Worksheets
About These 15 Worksheets
When we embark on our mathematical journey, we encounter a landscape rich with concepts that challenge and shape the way we think about numbers and their relationships. Among these foundational concepts, one that frequently captures attention is the idea of “factors.” Although the term may seem straightforward at first glance, the underlying concept carries significant weight in our broader understanding of mathematics. Factors are not just numbers on a page; they represent the building blocks of numerical relationships, and recognizing them can unlock a deeper comprehension of how numbers interact with one another.
But what exactly are factors? To put it simply, a factor of a number is a whole number that divides that number evenly, leaving no remainder. For example, if we consider the number 4, its factors are 1, 2, and 4, because these are the numbers that divide 4 without leaving anything behind. While this definition is simple, it holds profound implications for students as they dive deeper into the world of mathematics. From basic arithmetic to advanced algebra, factors lay the groundwork for a range of mathematical concepts that will appear again and again.
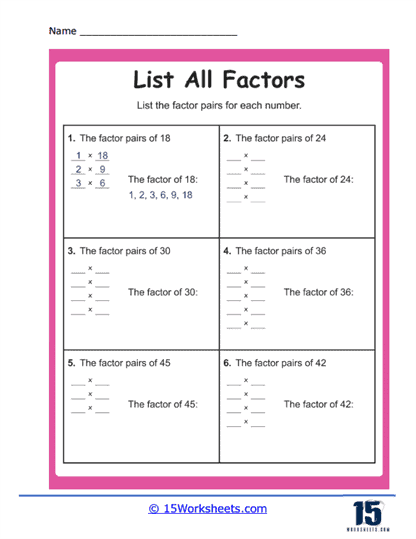
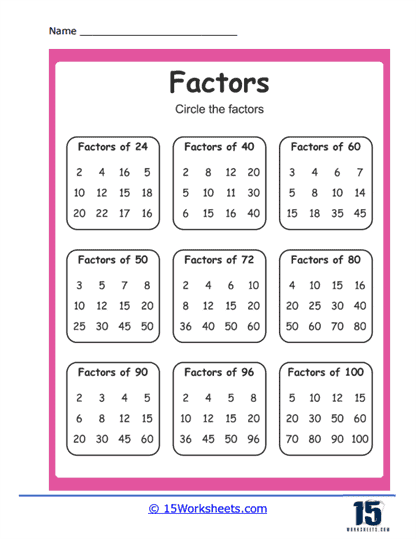
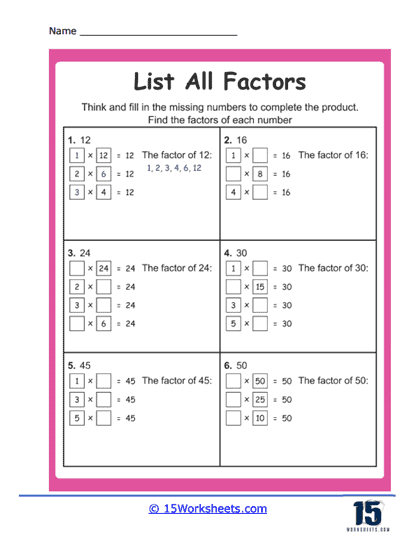
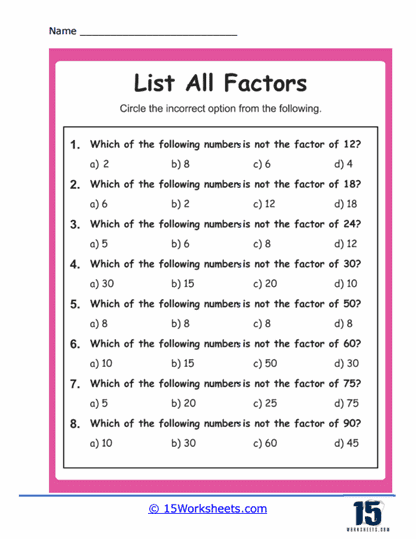
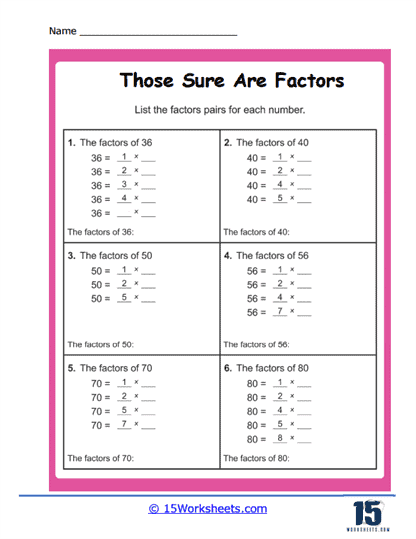
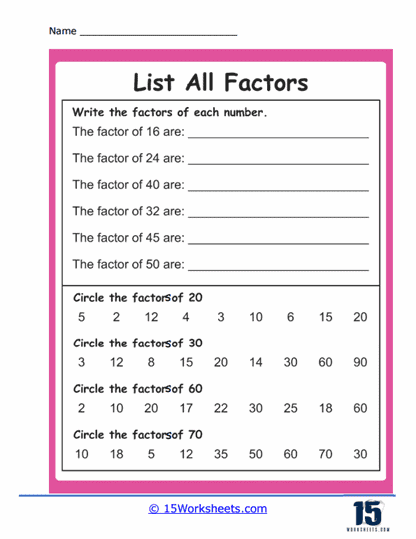
At the heart of understanding factors lies the ability to recognize and identify them through practice, and this is where the “List All Factors Worksheets” come into play. These worksheets are much more than a mere list of exercises; they are carefully crafted tools designed to help students develop a keen eye for spotting factors and understanding their significance. By repeatedly engaging with these worksheets, students learn to unravel the mystery of numbers and discover the hidden patterns that define their structure.
To truly appreciate the importance of factors, it helps to see how they manifest across different types of numbers. Some numbers, like prime numbers, have only two factors: 1 and the number itself. These numbers, such as 5 or 11, stand apart because they are indivisible by anything other than 1 and their own value. On the other hand, composite numbers, like 12, have multiple factors. For instance, 12 can be evenly divided by 1, 2, 3, 4, 6, and 12. Identifying these factors might seem like a routine task at first, but it requires a level of precision that comes with practice. Recognizing the factors of any number becomes a skill-a mathematical instinct that grows sharper with time and experience.
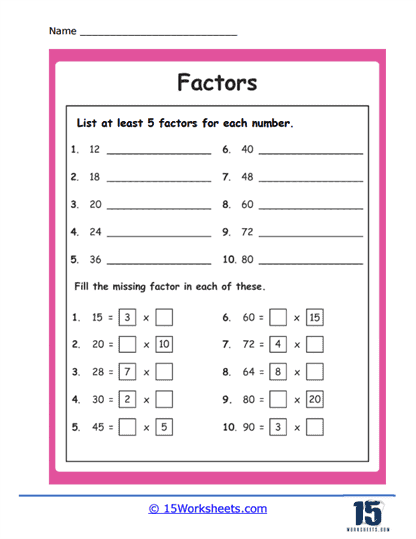
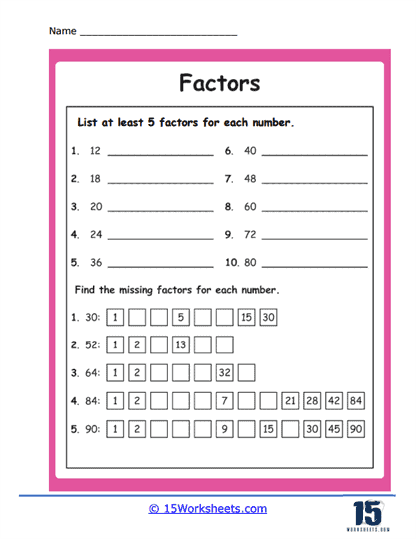
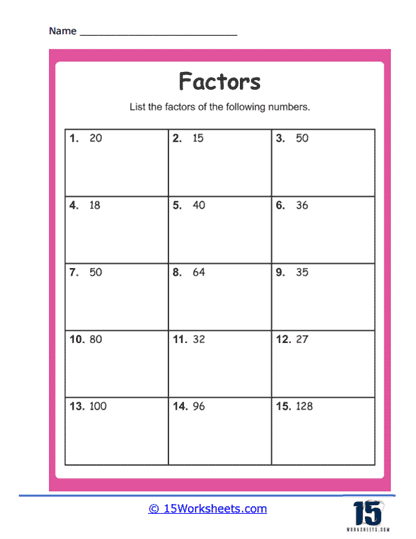
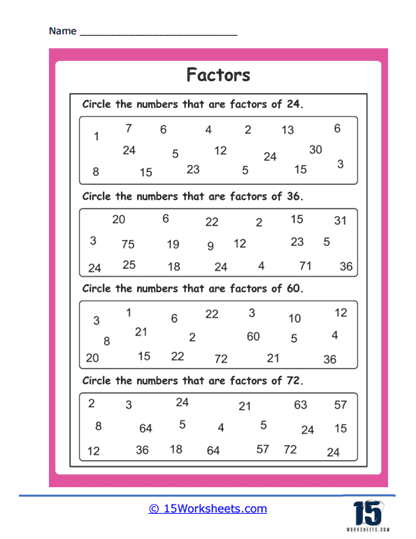
The exercises begin with simple tasks: listing the factors of small numbers like 10 or 12. Although these tasks may seem basic, they play a crucial role in building a solid foundation. Each time a student lists the factors of a number, they reinforce their ability to deconstruct a number into its essential components. Through repetition, the student becomes quicker, more confident, and more capable of tackling increasingly complex problems.
As the worksheets progress, they introduce larger numbers, such as 100 or 150, which challenge students to think more strategically. No longer can students rely on simple division by memory; they must employ reasoning and pattern recognition to find all the factors. This shift from smaller to larger numbers encourages students to think critically, fostering a deeper level of engagement and analytical thinking. This kind of exercise is not just about memorizing answers; it’s about developing a mathematical mindset that allows students to break down complex problems into manageable steps.
But the value of these worksheets doesn’t stop with simple number listings. As students advance, they encounter word problems that weave real-world scenarios into the concept of factors. Imagine a gardener who has 24 plants and wants to arrange them into equal rows. The problem now becomes a practical application of factors: how many different ways can the gardener organize the plants? This type of exercise not only reinforces the concept of factors but also illustrates how mathematics influences everyday decisions. In this way, factors move beyond abstraction and become tangible tools for solving real-life challenges.
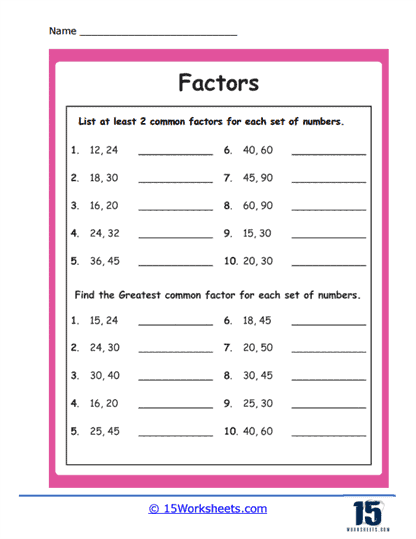
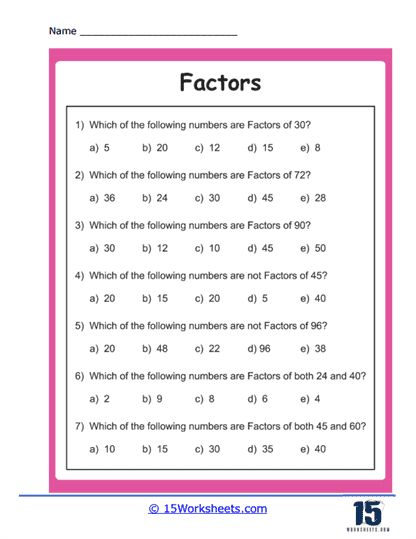
These worksheets will introduce the idea of “common factors,” a stepping stone to more advanced mathematical topics. In exercises focused on common factors, students may be presented with two or more numbers and asked to identify the factors they share. For example, the numbers 12 and 18 share the factors 1, 2, 3, and 6. This exercise builds the foundation for understanding the greatest common factor (GCF), a critical concept in algebra and number theory. As students master the ability to identify common factors, they also prepare themselves for more complex topics like factoring polynomials and solving equations.
These worksheets provide students with repeated exposure to factors, allowing them to internalize the process of identifying factors for any given number. However, beyond the simple act of recognition, these worksheets foster the development of analytical thinking. Every number becomes a puzzle waiting to be solved, and with each solution, students refine their approach to problem-solving. They learn to think methodically, to test different possibilities, and to arrive at a solution through logical reasoning.
Why Is This Skill Important?
The concept of factors may seem like a simple and abstract mathematical idea at first glance, but its significance stretches far beyond the pages of textbooks. In fact, the knowledge gained through exercises related to factors is a foundational building block for many areas of mathematics and real-world applications. As students progress in their academic journey, they will encounter complex topics such as fractions, algebra, and number theory-subjects where a deep understanding of factors plays a crucial role in their success. But the relevance of factors doesn’t stop at solving equations; it’s a versatile tool that empowers individuals to think critically and make efficient decisions in their everyday lives.
Consider the seemingly mundane task of simplifying fractions. While it may appear to be a basic skill, the underlying process of identifying common factors between numbers is a powerful application of number theory. Take the fraction 12/16 as an example. By recognizing that both the numerator and denominator share the factor 4, students can simplify the fraction to 3/4. This seemingly simple action requires a clear understanding of how numbers are constructed and how they can be broken down. The skill of simplifying fractions becomes invaluable as students move on to more advanced areas of math, such as solving complex equations or working with algebraic expressions. Factoring, in particular, becomes essential when tackling quadratic equations. Without a firm grasp of how factors work, solving these types of problems becomes an uphill battle. A solid foundation in factors, therefore, ensures that students are well-equipped to handle these challenges with confidence.
However, the importance of factors goes far beyond the classroom. In daily life, we encounter situations that rely on our ability to work with numbers, even if we don’t immediately recognize it. For instance, when following a recipe, adjustments to serving sizes require an understanding of how quantities relate to each other. Doubling a recipe or halving it is, in essence, working with factors to maintain the correct proportions of ingredients. Without this knowledge, one might inadvertently throw off the balance, leading to under-seasoned or overly spiced dishes. Factors provide the mathematical backbone that ensures consistency, whether in the kitchen or in any other scenario that requires precise scaling.
Let’s take a closer look at another everyday application: managing time and resources. Imagine you’re organizing an event and need to figure out how to arrange chairs in neat rows or how to allocate a fixed budget across multiple categories. The ability to list the factors of a number is essential in these situations. For instance, if you have 36 chairs and want to arrange them evenly in rows, knowing that the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36 gives you a variety of ways to set up the seating arrangement. You could opt for 6 rows of 6, 4 rows of 9, or even 3 rows of 12-each configuration providing a different layout that suits your space and aesthetic needs. This skill isn’t just limited to event planning; it’s equally useful in financial planning, where breaking down a budget into manageable portions requires an understanding of factors to ensure that each category is evenly funded.
The ability to list and work with factors also enhances problem-solving in a variety of contexts. Consider the challenge of finding the least common multiple (LCM) between two numbers. Whether you’re trying to coordinate schedules, align cycles in a process, or manage events that repeat at different intervals, the LCM becomes a valuable tool. For example, if two buses arrive at different intervals-one every 15 minutes and the other every 20 minutes-understanding the factors of these numbers helps you figure out when both buses will arrive simultaneously. The least common multiple of 15 and 20 is 60, so you know that every 60 minutes, both buses will be at the station at the same time. This skill is crucial in time management, helping you plan around recurring events and avoid conflicts.
In the world of design and construction, factors play a critical role in maximizing efficiency. Picture a scenario where you are tiling a floor that covers 48 square feet. You want to use tiles that fit perfectly into the space without the need for cutting. By listing the factors of 48-1, 2, 3, 4, 6, 8, 12, 16, 24, and 48-you can quickly identify which tile sizes will allow for a seamless installation. Whether you choose 12-inch or 16-inch tiles, you can be confident that the entire space will be covered efficiently, saving both time and materials. This same principle applies to many aspects of construction, from dividing materials evenly to determining the dimensions of a project. Factors provide the mathematical clarity needed to make decisions that are both practical and economical.
Even in the realm of recreation, factors make an appearance. Board games and strategy games often require players to group, divide, or organize objects in a way that maximizes efficiency or score points. Whether the task is dividing resources among players or arranging pieces on a board, the underlying math often involves factors. Players may not realize it, but their understanding of how to break down numbers is helping them strategize, outsmart opponents, and win the game. This seemingly simple mathematical concept becomes a powerful tool for critical thinking and strategic planning in both playful and serious scenarios.
Perhaps one of the most impactful real-world applications of factors lies in the area of financial planning. Managing money-whether through budgeting, saving, or paying off debts-often involves breaking down large sums into smaller, more manageable portions. For instance, if you are hosting an event with a budget of $1,000 and need to divide that amount across categories such as food, decorations, and entertainment, knowing the factors of 1,000 helps ensure that each portion is allocated evenly. Similarly, if you are trying to pay off a loan in equal installments, an understanding of factors allows you to break the total amount into equal payments that fit your financial situation. This practical use of factors can make the difference between a well-organized financial plan and one that quickly spirals out of control.