Prime and Composite Numbers Worksheets
About These 15 Worksheets
This collection of worksheets will help students understand the fundamental concepts of number classification in mathematics. These worksheets present exercises that focus on identifying, differentiating, and analyzing prime and composite numbers. Prime numbers are defined as numbers greater than 1 that have no divisors other than 1 and themselves. Composite numbers, on the other hand, are numbers greater than 1 that have more than two divisors. These worksheets provide structured practice for students to recognize these categories of numbers, develop number sense, and enhance their problem-solving skills.
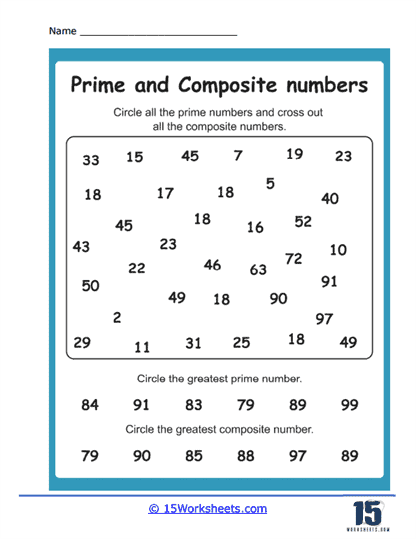
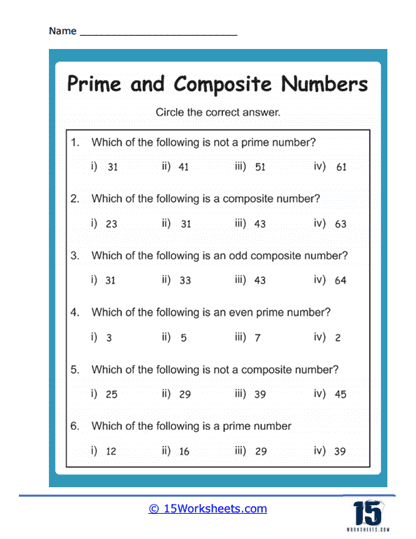
These worksheets include tasks such as circling prime or composite numbers in a set of given numbers, sorting numbers into prime and composite categories, and completing tables that require identifying factors of given numbers. Other tasks require students to write whether a number is prime or composite, based on their understanding of factors, or fill in blanks between numbers with appropriate composite numbers. There are also exercises where students follow a path of prime numbers in a grid to reach a goal, helping them reinforce their understanding in an engaging manner.
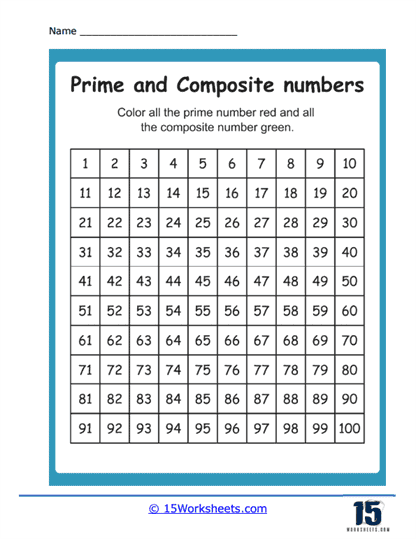
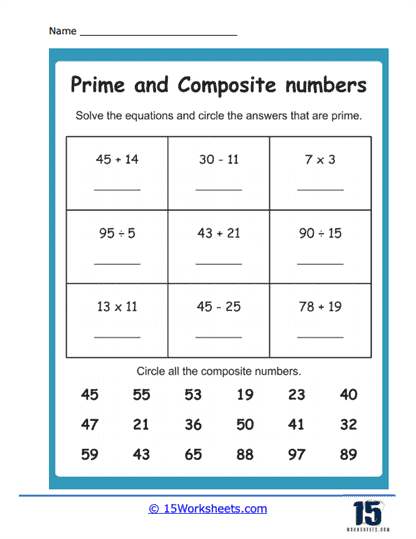
The collection of sheets incorporates visually interactive activities, such as coloring tasks, where students color prime numbers one color and composite numbers another. Such visual differentiation helps students better grasp the concept through color association. Some tasks also ask students to add prime and composite numbers to find the total, further promoting the application of number theory in basic arithmetic. These varied exercises are designed to engage different learning styles and ensure that students not only memorize but also apply their knowledge of prime and composite numbers in multiple contexts.
One of the key benefits of prime and composite numbers worksheets is that they simplify abstract concepts into practical, hands-on activities. By engaging with various problems, students can see real examples of prime and composite numbers, which aids in the retention of definitions. These worksheets may include exercises like listing numbers within a given range and determining whether each number is prime or composite, or decomposing composite numbers into their prime factors. The repetitive nature of such tasks reinforces the students’ ability to recall the properties of prime and composite numbers quickly and accurately.
The series often features worksheets that provide step-by-step problems that lead students to the discovery of patterns in number theory. For example, students might be guided through a sequence of prime factorizations, which helps them understand how every composite number can be broken down into a unique set of prime numbers. These exercises build a strong foundation for more advanced mathematical topics like factors, multiples, and eventually concepts such as the greatest common divisor (GCD) and least common multiple (LCM). Worksheets often include problems that encourage students to apply these concepts in different contexts, deepening their understanding.
In addition to developing basic arithmetic skills, these worksheets help foster logical reasoning and critical thinking. When students determine whether a number is prime or composite, they are required to check divisibility and think analytically about factors. This process improves their ability to approach problems methodically, breaking down complex questions into manageable parts. As students progress, worksheets may introduce higher-level questions, such as identifying prime numbers within larger sets or exploring the relationship between prime numbers and number patterns. These challenges promote deeper exploration and stimulate curiosity in mathematical inquiry.
Teachers can use these resources to gauge students’ understanding of number theory, provide additional practice, or introduce new concepts related to factors and multiples. Since the worksheets can be tailored to different levels of difficulty, they cater to a wide range of learners, from beginners just learning about number classifications to more advanced students exploring prime factorization and its applications. As a result, prime and composite numbers worksheets support diverse learning styles and help students build confidence in their math skills.
What are Prime Numbers?
Imagine walking into a toy store and seeing shelves filled with colorful building blocks. Some of these blocks can only be arranged in a single, unique way-just one on top of the other. They are simple, unbreakable structures that have only one method of construction. This is a perfect metaphor for prime numbers, the foundational units in the world of mathematics that are unique and indivisible in more than one way.
Prime numbers are special, almost magical in their simplicity. These are numbers greater than 1 that can only be divided by 1 and themselves, with no other numbers working as dividers to give a whole, complete result. They can be thought of as the “atomic” numbers in math, much like the atoms in chemistry that form the building blocks of more complex molecules. Just as atoms cannot be broken down into smaller particles that retain the same properties, prime numbers cannot be broken down into smaller factors beyond 1 and the number itself.
Let’s explore some prime numbers. The number 2, for example, stands as the smallest and only even prime number. No other even number can be prime because all even numbers after 2 can be divided by 2. This makes 2 particularly special-it’s a unique case where the rules of even numbers don’t disrupt its prime nature. Similarly, numbers like 3, 5, and 7 follow suit. These numbers can’t be neatly broken into smaller parts. If you try to divide 5 by anything other than 1 or 5, you’ll end up with decimals or fractions, incomplete pieces that don’t fit the whole.
Think of trying to divide 7 by 2 or 3. You can do it, sure, but the result won’t be a nice, clean number. It will be messy-3.5 or 2.3333 repeating-which means 7 resists being split evenly by anything other than 1 or 7. This characteristic of prime numbers makes them essential building blocks in the grand architecture of math. They are the first layer of structure that everything else rests upon.
What are Composite Numbers?
Now, on the other hand, there are composite numbers. If prime numbers are like those rigid blocks that only stack one way, composite numbers are like a versatile set of building blocks that can be arranged in multiple configurations. You don’t just have to stack them one on top of another; you can build rows and columns, giving you more flexibility and options for construction. This flexibility reflects the nature of composite numbers, which can be divided not just by 1 and themselves, but by additional numbers as well.
Composite numbers offer more options. Take the number 4, for instance. Unlike a prime number, which can only be neatly divided by 1 and itself, the number 4 can be divided by 2 in addition to 1 and 4. This means 4 is not rigid like a prime number; it can be broken down into smaller whole-number components. Imagine trying to build a structure using blocks representing the number 4. You could stack two blocks next to each other to represent 2 x 2, or you could view the whole structure as four individual blocks, or even one block with the number 4 etched on it. These multiple ways of dividing 4 reflect its composite nature.
The number 6 is another clear example of a composite number. You can divide 6 by 1 and 6, but you can also divide it evenly by 2 and 3. In essence, 6 can be expressed as 2 x 3, meaning it’s a combination of these two prime numbers. Composite numbers, in fact, are often made by multiplying prime numbers together. This process of multiplying primes to form composites is why primes are considered the essential building blocks of all numbers.
Let’s take 12 as an example to see how many different ways you can divide it. You can divide 12 by 1, 2, 3, 4, 6, and 12, which gives us many options. One interesting thing about composite numbers like 12 is that they can be broken down into smaller primes. In this case, 12 is 2 x 2 x 3, a combination of the primes 2 and 3. This breakdown shows how composite numbers are constructed from primes, making primes the fundamental units that compose the world of whole numbers.
The Importance of Prime and Composite Numbers
Understanding the distinction between prime and composite numbers is essential not just for basic math, but for more complex fields like cryptography, computer science, and number theory. Prime numbers have a critical role in encryption algorithms that secure online data. The difficulty of factoring large composite numbers into their prime components is what keeps your private information safe during internet transactions.
In this way, prime numbers act like tiny, unbreakable keys, while composite numbers are complex locks that can be opened only if you understand how they’re constructed. Think of an enormous number like 91,427. It might appear prime at first glance, but with some work, it can be revealed as the product of smaller primes: 97 x 943. Understanding these building blocks helps unravel the mysteries of larger, more complicated numbers.
The relationship between prime and composite numbers is similar to the relationship between raw materials and finished products. A prime number is raw, unprocessed, and indivisible, whereas a composite number is more like a crafted item made from combining multiple prime materials. Each has its place in the mathematical universe, with primes serving as the bedrock upon which more complex structures are built.
Types of Exercises on the Worksheets
Circle the Prime Numbers – In this type of exercise, you’ll see a list of numbers. Your task is to circle or color all the prime numbers.
Example: Numbers – 2, 4, 5, 6, 9
You would circle – 2 and 5 because they’re prime!
Underline the Composite Numbers – Just like the previous exercise, but this time, you underline or highlight the composite numbers.
Example: Numbers – 3, 7, 8, 10, 11
You would underline – 8 and 10.
Matching Pairs – This exercise will have two columns. One with numbers and another with the words ‘prime’ and ‘composite’. You draw a line connecting each number to the right word.
Example: Column A – 2, 9, 12, Column B – Prime, Composite
You would connect 2 to ‘Prime’, 9 and 12 to ‘Composite’.
Factoring Fun– In this game, you’ll list all the numbers that can multiply together to give the number in question. This helps to see if a number is prime or composite.
Example: Number – 6
Factors – 1, 2, 3, 6
Color the Grid – You’ll get a grid filled with numbers. Using different colors, you color in the prime numbers with one color and the composite numbers with another.
Fill in the Blanks – This exercise has sentences with blanks. Fill them in using the correct number type (prime or composite).
Example: 10 is a ________ number.
Answer – Composite