Absolute Value Worksheets
About These 15 Worksheets
These worksheets help students explore the concept of absolute value. This is a mathematical function that measures the distance between a number and zero on the number line. It is denoted by two vertical bars surrounding a number or expression, like |x|. For a real number x, the absolute value of x is defined as follows:
If x is positive or zero, then |x| = x.
If x is negative, then |x| = -x.
In other words, the absolute value of a positive number is itself, and the absolute value of a negative number is its negation (making it positive).
You went 5 steps to the left, and your friend went 5 steps to the right. When asked how far each of you went from home, the answer would be 5 steps, even if the direction was different. This concept of “how far” from a starting point, without considering the direction, is what we call the absolute value in math.
Absolute value is a way to always think of a number in positive terms, no matter if it started out as negative or positive. In math, we represent the absolute value of a number by putting vertical bars around it. For example, the absolute value of -7 is written as |-7| and is equal to 7. Similarly, the absolute value of 7 is |7| which is still 7.
Different Types of Exercises on These Worksheets
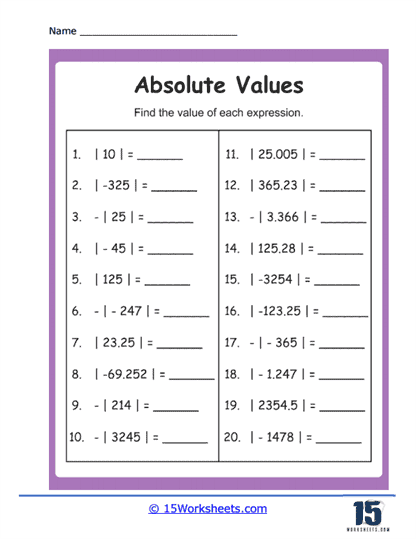
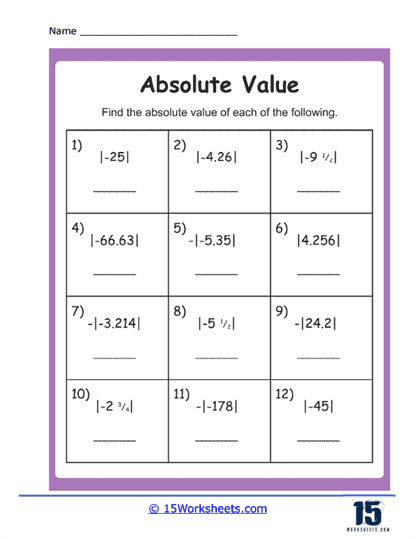
Identification of Value – Here, you’ll be given numbers and you’ll have to write their absolute value. For instance, if you see -8, you’ll write its absolute value as 8.
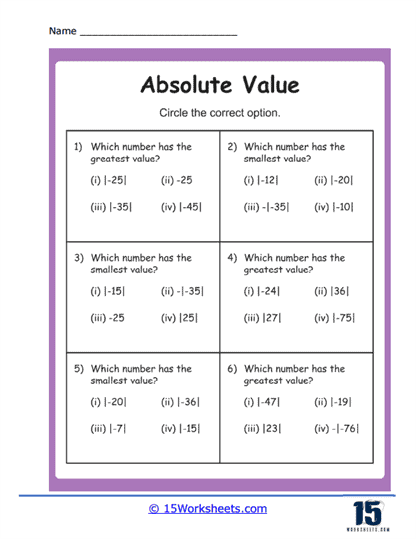
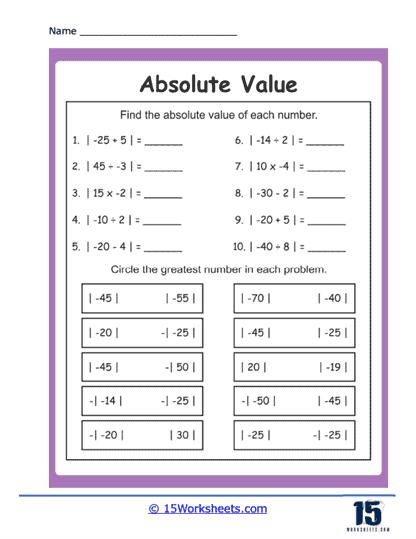
Comparing Values – For this type, you might see questions like, “Which has a greater absolute value – -12 or 9?” Here, |-12| is 12 and |9| is 9, so -12 has the greater absolute value.
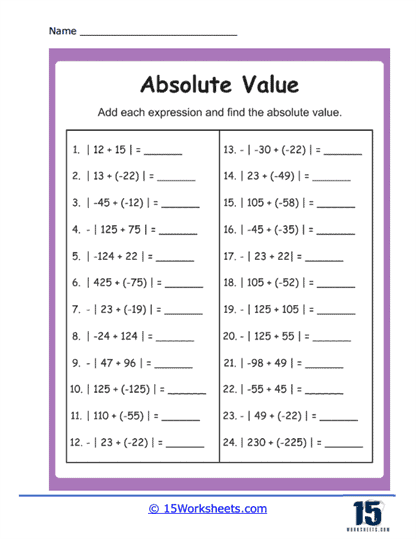
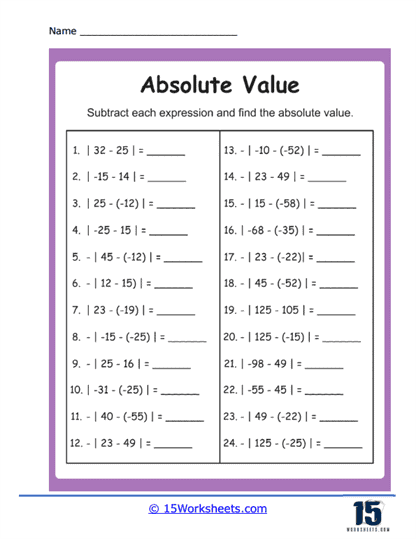
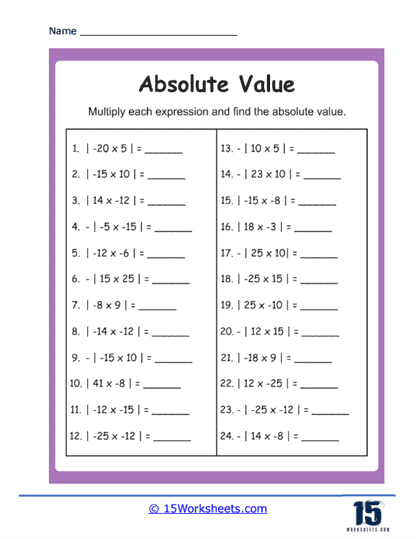
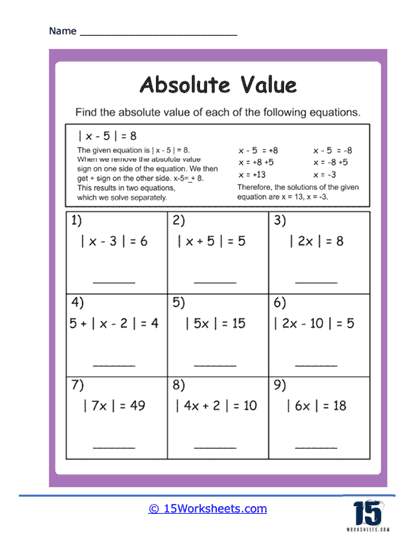
Operations with Absolute Values – These exercises challenge you to add, subtract, multiply, or divide using absolute values. For example, |-3| + |5| = 3 + 5 = 8.
Where Would You Use This Skill in Everyday Life?
Money – Let’s say your allowance went down by $10 this month and your sibling’s allowance went up by $10. When discussing the change in allowance (without worrying about up or down), both of you had an absolute value change of $10.
Temperature – If it was 5 degrees below freezing yesterday and 3 degrees above freezing today, the absolute value would tell you how cold or warm it was without considering below or above freezing.
Distance – If you walk 3 miles north and your friend walks 3 miles south, you both have walked an absolute distance of 3 miles.
Elevations – If you’re comparing depths of places below sea level to heights of mountains above sea level, the absolute value can tell you about the magnitude or size of these places without worrying about the direction (up or down).
The Fundamental Properties of Absolute Value
1. Non-negativity – For any real number x, |x|≥ 0
This means the absolute value of any number is always non-negative. Specifically, |0| = 0
2. Positive Definiteness – |x| = 0 if and only if x = 0
The absolute value of a number is zero if and only if that number is zero.
3. Symmetry – |-x| = |x|
The absolute value of a number and its negative are the same. This is because both numbers are the same distance from zero on the number line.
4. Triangle Inequality – For any real numbers x and y, |x + y| ≤ |x| + |y|
This property is crucial in various mathematical proofs and is reminiscent of the idea that the shortest distance between two points is a straight line.
5. Multiplicative Property – |xy| = |x| x |y|
The absolute value of a product is the product of the absolute values.
6. Quotient Property (for y ≠ 0)
|x/y| = |x| / |y|
The absolute value of a quotient is the quotient of the absolute values, provided the denominator is not zero.
7. Squares and Roots – |x|2 , √x2 = |x|
The square of the absolute value of a number is the same as the square of the number itself. And conversely, the square root of the square of a number is its absolute value.
Examples of Absolute Value Problems
1. Identification
Find the absolute value of the following numbers
a) -15
b) 10
c) -23
Answer:
a) |-15| = 15
b) |10| = 10
c) |-23| = 23
2. Operations with Absolute Values
Evaluate the expression:
a) |-7| + |4|
b) |-8| – |3|
c) |-5| x |2|
Answer:
a) |-7| + |4| = 7 + 4 = 11
b) |-8| – |3| = 8 – 3 = 5
c) |-5| x |2| = 5 x 2 = 10
3. A Word Problem with Absolute Value
Alex deposited $50 into his bank account on Monday, but on Tuesday, he withdrew $75. By using absolute values, calculate the total change in money Alex experienced over the two days.
Answer:
Change on Monday = |$50| = $50
Change on Tuesday = |-$75| = $75
Total change = $50 + $75 = $125