Degrees, Minutes, and Seconds Worksheets
About These 15 Worksheets
These worksheets will help students understand the finer details of angle measurement. These worksheets introduce and reinforce the concept of breaking down degrees into smaller units, namely minutes and seconds, which are crucial for accurate angle measurement in various mathematical and practical applications. These units are particularly useful in fields such as navigation, astronomy, and even in some areas of engineering where precision is paramount. By engaging with these worksheets, students develop the skills necessary to convert between these units and decimal degrees, which is an essential trigonometric competency.
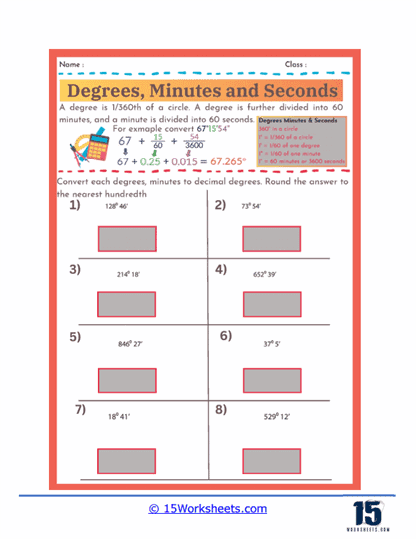
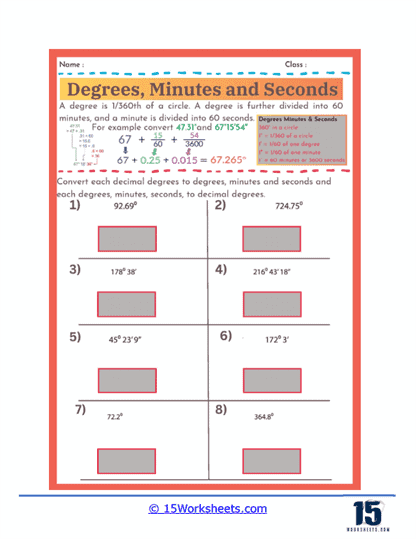
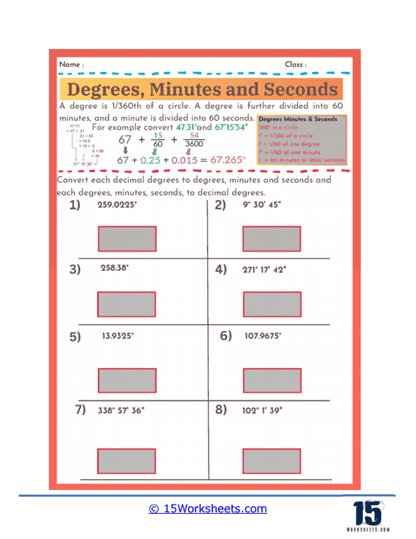
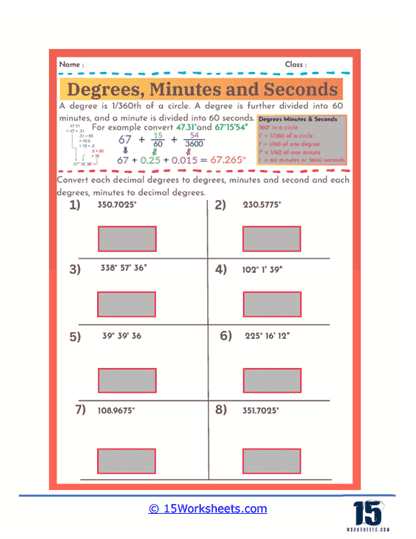
The concept of degrees, minutes, and seconds is based on the fact that a degree is a measure of 1/360th of a circle. To further refine this measurement, each degree is divided into 60 minutes, and each minute is subdivided into 60 seconds. This subdivision allows for very precise measurement of angles, which is particularly important in fields where exactitude is critical. The worksheets typically include problems that require students to convert angles given in decimal degrees into degrees, minutes, and seconds, and vice versa. This dual conversion practice helps students to see the relationship between these units and understand how to switch between them depending on the context.
In one type of worksheet, students might be asked to convert a series of angles given in decimal degrees into degrees, minutes, and seconds. For example, an angle might be given as 123.456 degrees, and students would need to express this as 123 degrees, 27 minutes, and 21.6 seconds. This type of problem helps students practice breaking down the decimal part of the degree into the smaller units, reinforcing their understanding of how these units relate to one another. This exercise not only sharpens their mathematical skills but also prepares them for more advanced trigonometric calculations where such conversions are necessary.
Another common type of worksheet flips this process around, asking students to convert angles from degrees, minutes, and seconds into decimal degrees. For example, students might be given an angle of 45 degrees, 30 minutes, and 15 seconds and be asked to convert this into a decimal, such as 45.5042 degrees. This type of conversion requires students to understand the proportional relationship between the degrees, minutes, and seconds and apply this understanding to calculate the equivalent decimal value. This exercise is critical for students who will go on to work in fields where decimal degree measurements are standard, such as in GPS technology and various scientific disciplines.
A particularly engaging aspect of these worksheets is how they incorporate real-world applications of these conversions. For instance, students might encounter problems that simulate navigation or astronomy scenarios where precise angle measurements are crucial. By framing the exercises in this way, the worksheets make the math feel more relevant and applicable, which can help motivate students to engage more deeply with the material. Real-world context not only enhances understanding but also shows students the practical importance of what they are learning.
Some worksheets will include mixed practice, where students must switch back and forth between converting from decimal degrees to degrees, minutes, and seconds, and vice versa. This mixed approach ensures that students are not simply memorizing procedures but are actually internalizing the relationships between these units. Such practice helps to build fluency in these conversions, ensuring that students can perform them quickly and accurately, which is important in both academic and professional settings.
Another skill that these worksheets help develop is attention to detail. Since converting between decimal degrees and degrees, minutes, and seconds involves handling small units and precise calculations, students must be meticulous in their work. This precision is a critical skill not only in trigonometry but in all areas of mathematics and science where exact measurements are required. The worksheets also typically ask students to round their answers to the nearest second or the nearest hundredth of a degree, further reinforcing the need for precision.
The worksheets also help students develop a deeper understanding of the concept of angular measurement. By working with degrees, minutes, and seconds, students gain a more intuitive grasp of what an angle represents and how it can be measured in different ways. This understanding is foundational for more advanced topics in trigonometry, such as working with radian measures or solving trigonometric equations. The ability to visualize and manipulate angles is crucial for success in these areas, and these worksheets provide the practice needed to build that capability.
In addition to the mathematical skills, these worksheets also help students develop problem-solving strategies. Converting between different units of measurement requires students to think critically about the relationships between those units and to apply their knowledge in a systematic way. This type of thinking is essential for solving complex problems in trigonometry and beyond. By practicing these conversions, students are also learning how to break down a larger problem into smaller, more manageable steps, which is a valuable skill in any area of study.
This set of worksheets often incorporate opportunities for students to check their work and correct mistakes. For example, after converting an angle from decimal degrees to degrees, minutes, and seconds, students might be asked to convert it back to decimal degrees to ensure their conversion was accurate. This process of checking and revising reinforces the learning and helps students to develop a more thorough understanding of the material. It also teaches the importance of accuracy and verification in mathematical work, which are critical habits for success in any quantitative field.
Why Are These Units of Measure Significant in Trigonometry?
Degrees, minutes, and seconds (DMS) are important in trigonometry because they help us measure angles very precisely. Imagine cutting a pizza into 360 slices; each slice is one degree. But sometimes, we need to be even more precise, so each degree can be divided into 60 smaller parts called minutes, and each minute can be split into 60 even tinier parts called seconds. This way of measuring angles is crucial because it allows us to be very exact when we need to calculate things in trigonometry.
In the real world, DMS is used in many interesting ways. For example, sailors and pilots use it to navigate, helping them find their way across the ocean or sky by pinpointing exact locations. Surveyors, who measure land, also use DMS to make sure their measurements are precise. Astronomers use this system to track stars and planets in the sky, ensuring they know exactly where these celestial objects are at any given time. Engineers and architects rely on DMS to design buildings and structures, making sure everything fits perfectly. So, whether it’s finding your way, measuring land, or designing a building, degrees, minutes, and seconds play a big role in making sure everything is accurate and works well.