Converting Degrees and Radians Worksheets
About These 15 Worksheets
Converting between degrees and radians is a fundamental concept in trigonometry, and worksheets that focus on this topic play a crucial role in helping students grasp this conversion process. These worksheets are designed to reinforce the understanding of how angles can be measured in different units, and they provide the practice needed to master the conversion between degrees and radians. Let’s explore what these worksheets typically include and the math skills they help to develop.
Understanding the Basics of Conversion
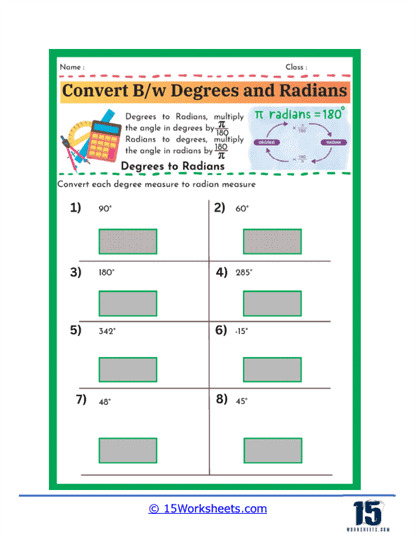
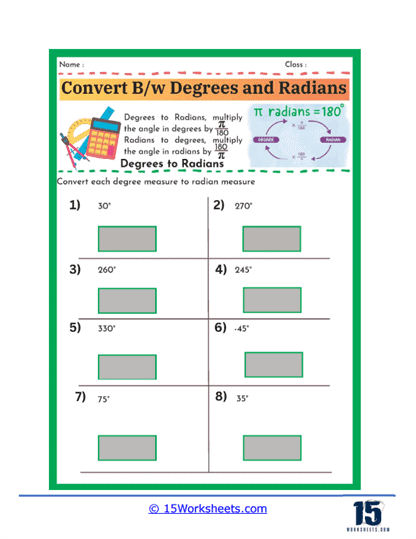
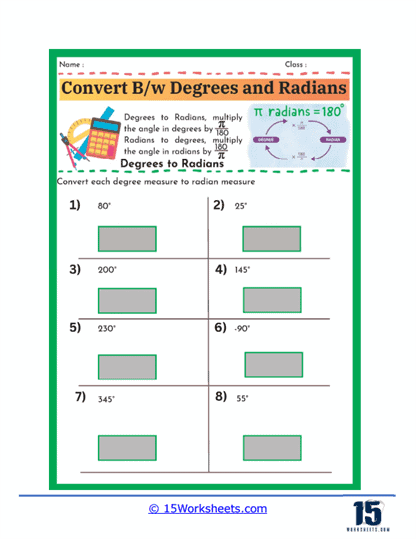
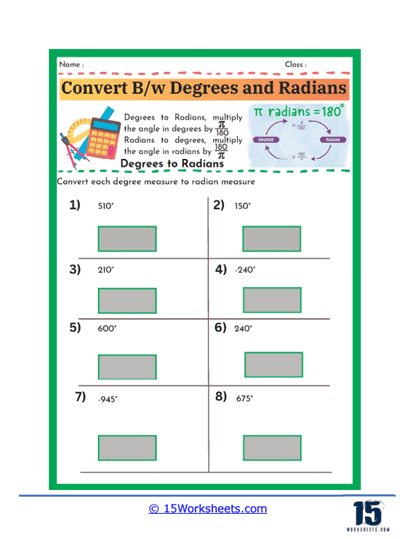
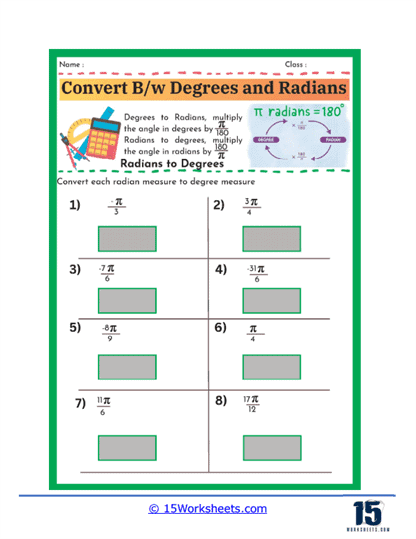
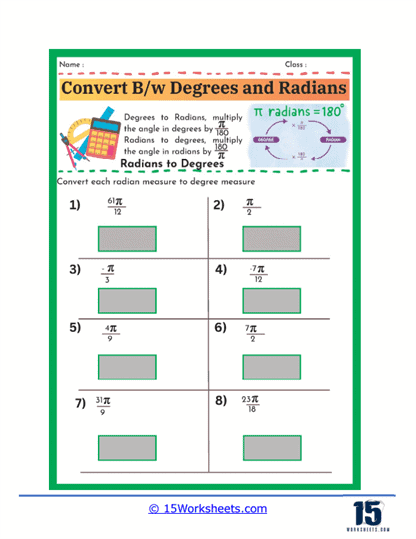
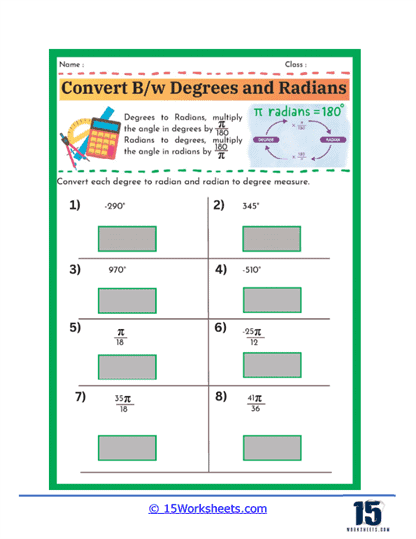
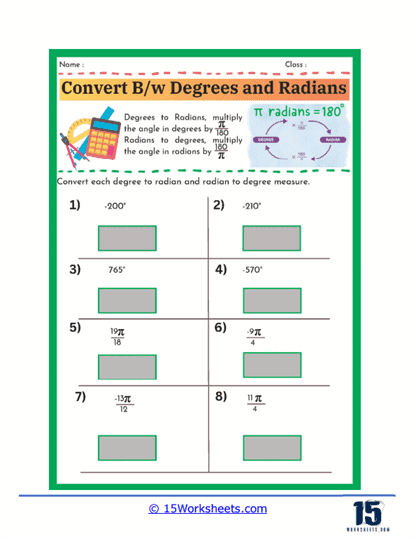
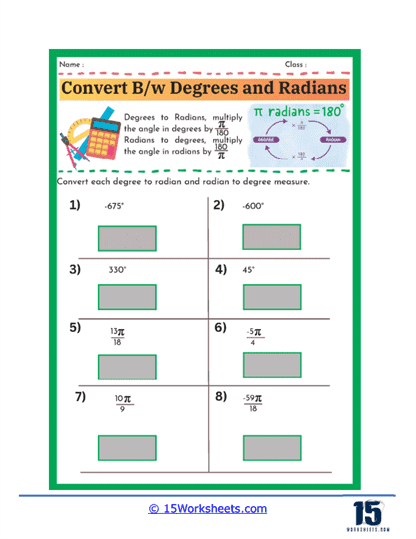
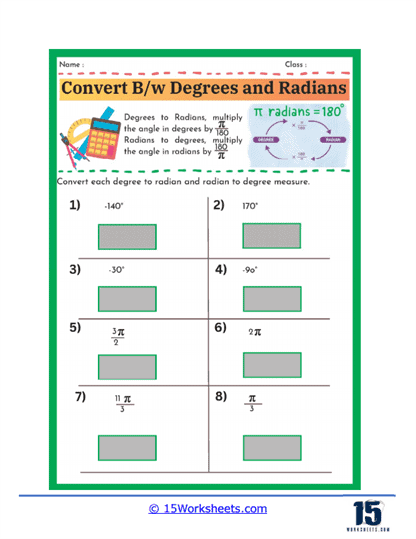
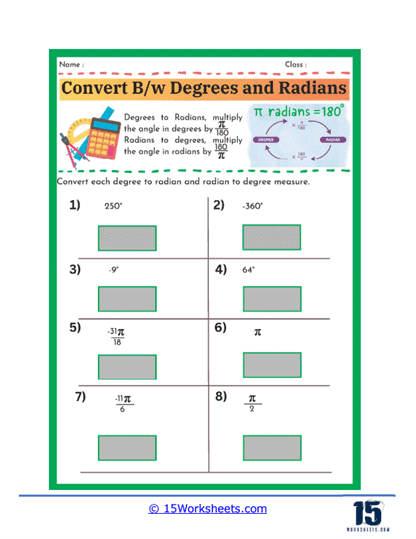
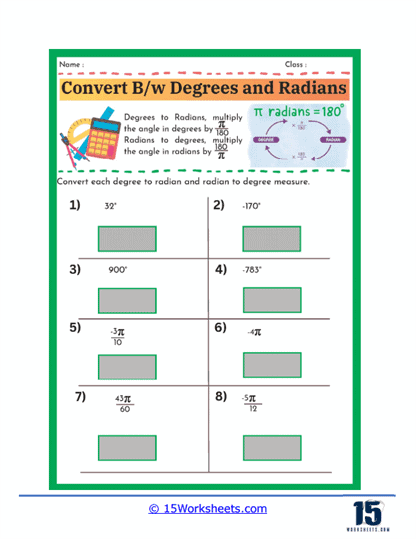
At the heart of converting between degrees and radians is the basic understanding that 180 degrees is equivalent to π radians. This equivalence serves as the foundation for all conversion exercises. Students need to internalize this relationship, as it allows them to transition seamlessly between the two units. Worksheets often begin by presenting this fundamental concept and then gradually guide students through the process of applying it.
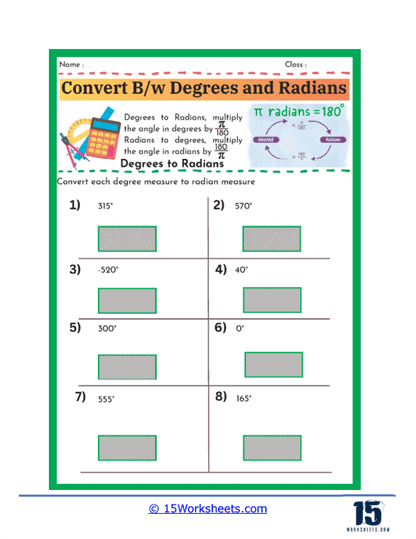
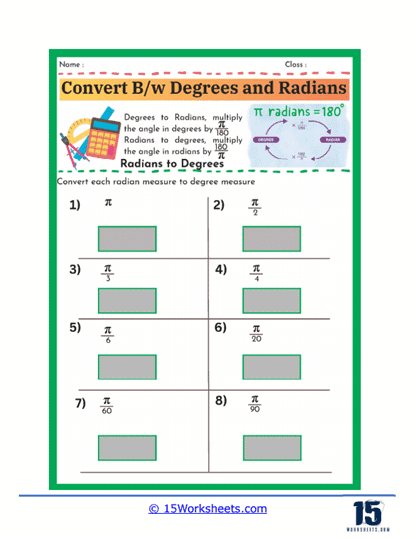
In these exercises, students might be asked to multiply or divide by π/180 or 180/π, depending on the direction of the conversion. For example, to convert degrees to radians, students multiply the number of degrees by π/180. Conversely, to convert radians to degrees, they multiply the radian measure by 180/π. These operations might seem straightforward, but consistent practice is necessary to ensure that students can perform them quickly and accurately.
Variety in Problem Types
These particular worksheets will help you when converting between degrees and radians they include a variety of problem types to cater to different learning stages. Early in the learning process, students may encounter problems that require direct conversion of whole numbers. For instance, they might be asked to convert 90 degrees into radians or π/2 radians into degrees. These problems reinforce the basic conversion formula and help students build confidence in their calculations.
As students become more comfortable with these conversions, worksheets might introduce more complex problems. These can include converting fractional degrees or radians, such as converting 32 degrees into radians or 3π/4 radians into degrees. These problems require students to pay closer attention to detail and manage fractions, reinforcing their understanding of both trigonometry and basic arithmetic.
Mixed Practice and Application
A crucial aspect of these worksheets is the inclusion of mixed practice problems, where students are required to convert both to and from degrees and radians within the same set of problems. This helps prevent students from simply memorizing procedures without truly understanding the relationships between the units. Mixed practice ensures that students develop a deeper conceptual understanding, as they must decide on the correct operation to perform for each problem.
Some worksheets also incorporate word problems or real-life scenarios where students must apply their conversion skills. For example, they might be asked to determine the radian measure of an angle that is critical in designing a ramp or calculate the degree measure of an angle in a navigational problem. These types of problems help students see the relevance of what they are learning, making the math more engaging and practical.
Advanced Exercises
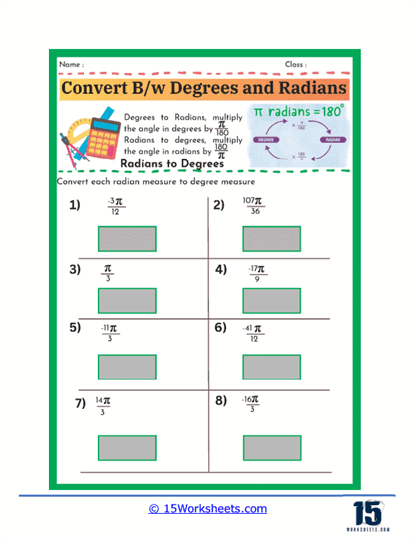
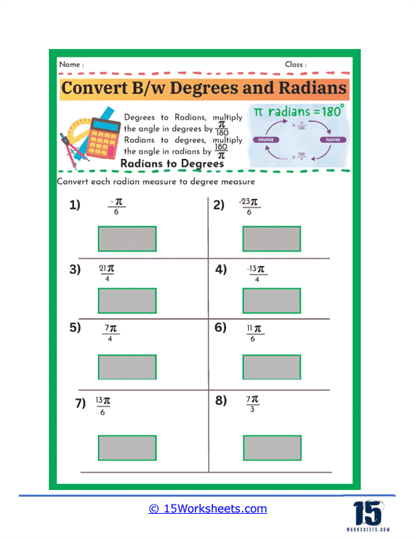
For students who have mastered the basic conversions, more advanced worksheets are available that challenge them further. These worksheets might include problems that involve negative angles or angles greater than 360 degrees (or 2π radians). Such problems require students to think critically about the cyclical nature of angles and how to handle conversions in less straightforward scenarios.
Additionally, some advanced worksheets might involve working with angles in different quadrants or require the use of inverse trigonometric functions to find angle measures in different units. These exercises are particularly useful for students who are preparing for higher-level mathematics courses, as they simulate the types of problems encountered in calculus and beyond.
Skills Development
The consistent practice provided by these worksheets helps students develop a range of mathematical skills. First and foremost, they enhance students’ arithmetic abilities, particularly in handling fractions and performing multiplication and division with π. These skills are not only crucial for trigonometry but also for other areas of mathematics.
These worksheets help students develop a strong sense of mathematical fluency, which is the ability to perform calculations accurately and efficiently. This fluency is vital for success in any STEM field, where quick and precise calculations are often required.
Another key skill that these worksheets help to build is problem-solving. By working through a variety of conversion problems, students learn to identify the appropriate method for each scenario and apply it correctly. This problem-solving ability is transferable to many other areas of mathematics and science.
Building Confidence
One of the most significant benefits of using these worksheets is the confidence they help build in students. As students progress through the exercises, they move from simple to more complex problems, allowing them to experience success at every stage. This gradual increase in difficulty ensures that students are not overwhelmed and can build their skills step by step.
By the time students complete these worksheets, they should feel confident in their ability to convert between degrees and radians without hesitation. This confidence is crucial as they move forward in their study of mathematics, as it lays the groundwork for tackling more complex trigonometric concepts.
In some cases, you will find very helpful visual aids, such as interactive unit circles, that help students see the relationships between degrees and radians in a more tangible way. By integrating technology, these worksheets can cater to different learning styles, making the learning process more engaging and effective.