Extend the Pattern Worksheets
About These 15 Worksheets
These worksheets were created to help primary level students understand and practice the concept of patterns. They come filled with a wide array of exercises aimed at enabling students to recognize, repeat, and extend patterns, which plays a crucial role in developing their mathematical proficiency.
The worksheets typically include activities involving different types of patterns – such as numerical patterns, shape patterns, color patterns, and so on. The child is usually presented with a sequence that follows a particular rule or pattern, and they are asked to extend or continue the pattern by figuring out what comes next.
For instance, a numerical pattern worksheet might present a series of numbers like 2, 4, 6, 8, and then ask the student what the next two numbers should be. Here, the child needs to recognize that the pattern involves adding two to the previous number, thus, the next two numbers would be 10 and 12.
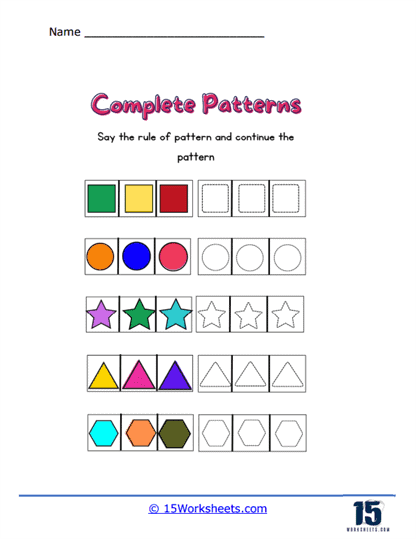
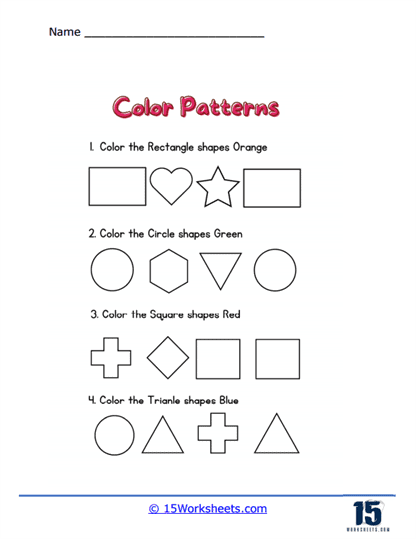
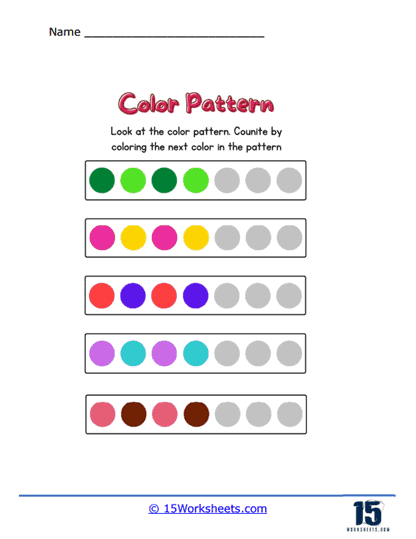
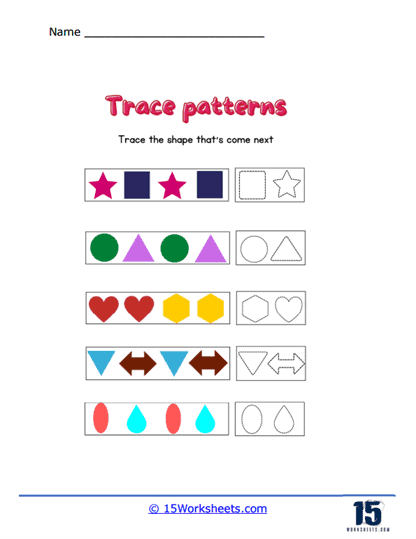
A shape or color pattern worksheet, on the other hand, may involve a sequence of shapes or colors (e.g., square, triangle, square, triangle) and the student would need to identify and continue the pattern.
These worksheets can be instrumental in improving math skills in several ways:
Foundation for Advanced Mathematics – Recognizing and extending patterns form the bedrock for understanding more complex mathematical concepts. From the number sequences that underpin arithmetic to the periodicity in trigonometry, patterns are integral to math. Hence, getting a strong handle on patterns in the early stages of learning math is beneficial.
Algebraic Thinking – Patterns play a key role in the development of algebraic thinking, which is the ability to explore and understand mathematical relationships. By identifying the rule that governs a pattern, children are effectively beginning to understand the essence of algebra – the use of symbols to represent relationships.
Problem Solving – Pattern recognition forms a crucial part of problem-solving. Many problems, even outside the sphere of math, can be resolved more easily by spotting a pattern. Hence, extending patterns enhances problem-solving skills.
Critical Thinking – Understanding and extending patterns require students to observe carefully, analyze the given sequence, identify the rule that governs the pattern, and apply that rule to extend the pattern. This process fosters critical thinking skills.
Observational Skills – Extend the Pattern Worksheets help enhance observational skills as students must scrutinize the pattern to understand the rule that drives it. These observational skills are essential, not only in math but in all areas of learning.
Attention to Detail – These worksheets cultivate an attention to detail in students. In order to successfully extend a pattern, children need to pay careful attention to each element of the pattern and the order in which they occur.
Predictive Abilities – Pattern recognition is a predictive skill, as the whole point of recognizing a pattern is to be able to predict what comes next. This skill has wide-ranging applications, from predicting the outcome of a math problem to forecasting real-life scenarios.
Cognitive Development – Extending patterns enhances cognitive abilities such as logical reasoning, sequential thinking, and spatial awareness. These cognitive skills play a critical role in overall academic performance and intellectual growth.
How Do You Plan to Extend a Pattern?
Extending a pattern, whether it’s numerical, visual, or auditory, involves a series of steps that require careful observation, identification, and application of the underlying rule. Here’s a step-by-step guide on how to plan to extend a pattern:
Observation – The first step in extending a pattern is to observe it closely. Look at the elements of the pattern, their sequence, and their relationship to each other. For example, if the pattern is numerical, observe the series of numbers carefully.
Identify the Type of Pattern – Determine what type of pattern it is. Patterns can be simple, such as an alternating color pattern, or complex, like a growing number sequence. They can be repeating or increasing, symmetrical or sequential. Understanding the type of pattern will give you a clue about how it’s formed.
Identify the Rule – Once you’ve identified the type of pattern, the next step is to figure out the rule that governs it. In a numerical pattern, this could involve addition, subtraction, multiplication, division, or more complex mathematical operations. In a shape pattern, it could be a rotation, reflection, or a sequence of shapes. The rule is the key that unlocks the pattern.
Apply the Rule – Once you’ve identified the rule, you can apply it to extend the pattern. Use the rule to determine what comes next. For example, if you’ve determined that a number pattern involves adding 2 each time, you can add 2 to the last number to find the next one.
Verify Your Work – After extending the pattern, check your work. Apply the rule again to ensure that your extension follows the same pattern. This step is important to confirm your understanding and accuracy.
Continue Extending – If needed, continue extending the pattern as far as required, each time applying the rule and verifying your work.
Reflect – Finally, reflect on the pattern and your understanding of it. Can you explain the rule in your own words? Can you create a similar pattern? This reflection can deepen your understanding and make it easier to recognize and extend similar patterns in the future.