Complete the Pattern Worksheets
About Our Complete the Pattern Worksheets
These worksheets typically consist of a series of visual or numerical patterns with missing elements that need to be filled in. The patterns can be based on shapes, colors, numbers, letters, or a combination of these.
The goal of these worksheets is for children to identify the underlying pattern and extend it by filling in the missing elements. By doing so, children learn to recognize and predict patterns, which is an important skill in various areas of mathematics, problem-solving, and critical thinking.
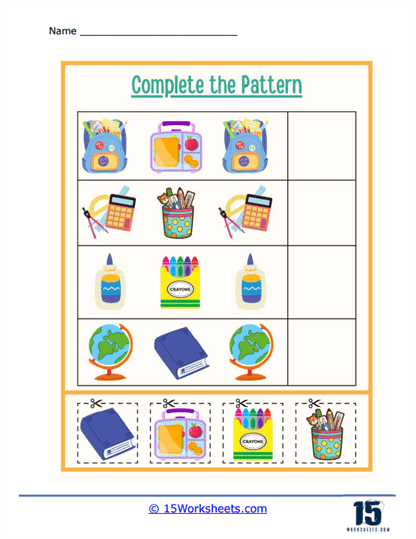
Take “Cut and Paste School Items“, for instance. Here, students navigate a thrilling paper trail of pencils, backpacks, and mystery erasers, all in a valiant effort to decide which school supply bravely goes where. It’s less about “what comes next” and more about “who dares take the final spot in this Office Depot saga.”
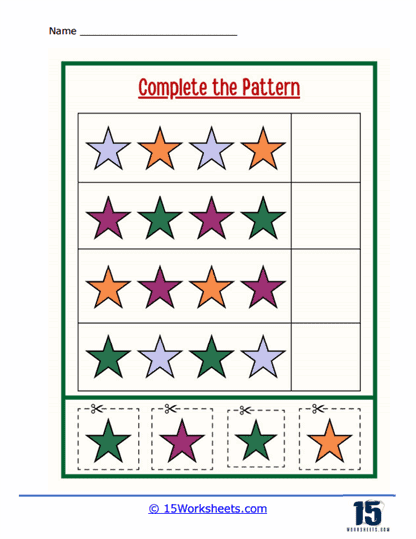
Seasonal chills sweep in with “Winter Items“, where mittens and scarves march in snug little sequences like fashion-forward snowmen with type-A personalities. Students must determine which cozy item best continues the wintery progression, lest the pattern be left shivering in incompletion. Meanwhile, “Finish the Star Pattern” is essentially patterning meets astronomy for preschoolers. Here, kids become cosmic cartographers, helping rebellious stars find their place in the twinkling order of the worksheet universe-no telescope required.
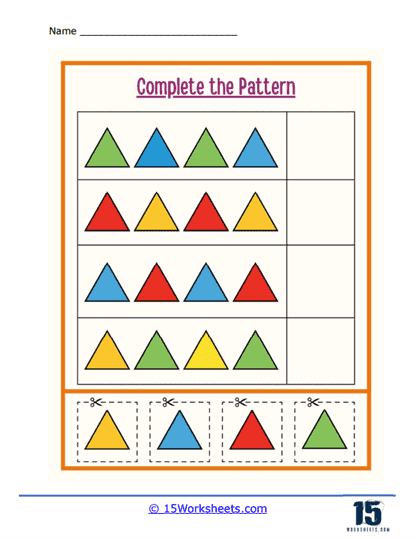
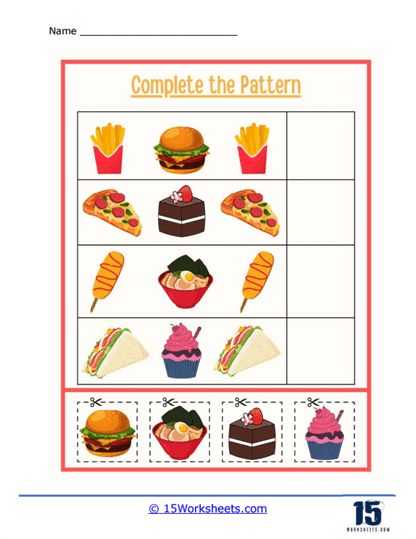
Things take a colorful turn in “Colored Triangles” and “Colorful Next Shapes“, which are like disco parties for polygons. These worksheets test a student’s color recognition and ability to not panic when faced with a purple triangle that should obviously come after a green square. Then comes “Junk Food Patterns“, the most deliciously confusing worksheet of all, where fries, donuts, and burgers dare you to figure out who’s next in line at the drive-thru of logic. Pair that with “Sweet to Eat Patterns“, and suddenly the classroom is a mathematical bakery-except you’re only allowed to look, not lick.
But the pattern path is not all treats and glitter. Worksheets like “Toy Pattern Completion“, “Mark the Next Shape“, and “What Goes Next” demand mental gymnastics. Students must channel their inner toy-box philosopher and decode the order of trains, blocks, or mysterious geometric nobles. “Complete the Shape Pattern” and “What Makes Shape Sense” practically require students to take an oath to Euclidean geometry. It’s shape identification with an existential twist: Does this rhombus truly belong, or is it merely a parallelogram with dreams?
Things wrap up (sometimes literally) with “Cut and Paste Shapes“, “What’s the Next Circle“, and “Hexagon Is Gone“. These are not just exercises-they are stories of loss and reunion. The missing circle must be found. The vanishing hexagon must be restored to its rightful tessellation. Every snip of the scissors and every dotted-line glue job is an act of heroic restoration. If you’re a student who completes all these worksheets, you don’t just master patterns-you become the pattern whisperer, fluent in the mysterious language of shapes and snacks.
The difficulty level of these worksheets can vary, starting from simple patterns with predictable repetitions to more complex sequences that require advanced logical reasoning. As children progress, they may encounter patterns with multiple variables or patterns that require understanding concepts such as symmetry or transformation.
Complete the pattern worksheets can be a fun and engaging way for children to enhance their cognitive abilities, improve their analytical thinking, and develop a solid foundation in mathematics.
In short, these worksheets aren’t just about learning sequences-they’re about becoming one with them. They’re an oddly philosophical, occasionally hilarious, and surprisingly profound adventure into the fabric of visual logic. Whether helping a lonely mitten find its pair or guiding a lost triangle home, kids sharpen their minds while giggling at how absurdly fun patterns can be. It’s the most epic journey a set of paper shapes and cartoon French fries can offer.
Why Are Understanding Patterns Important for Problem Solving?
Patterns provide a way to recognize relationships and connections between different elements or data points. By identifying patterns, we can extract meaningful information, uncover dependencies, and understand how various components relate to each other. This recognition of relationships is crucial in problem-solving as it helps us make logical connections and formulate effective strategies.
Patterns allow us to make predictions and extrapolate information beyond the given data points. By observing and understanding the pattern, we can anticipate what comes next or infer missing information. This ability to predict and extrapolate is valuable in problem-solving scenarios where we need to project outcomes, estimate trends, or fill in gaps to find solutions.
Patterns enable us to generalize information and abstract key principles. When we identify a pattern, we can often apply it to similar situations or use it as a basis for creating general rules or formulas. This capacity for generalization and abstraction is essential in problem-solving because it allows us to solve new problems by applying existing patterns or adapting them to fit new contexts.
Recognizing patterns can lead to more efficient problem-solving processes. By identifying repetitive elements or processes within a problem, we can develop systematic approaches, algorithms, or shortcuts. This can help streamline problem-solving and reduce the time and effort required to reach a solution.