Number Patterns Worksheets
About These 15 Worksheets
These worksheets will enhance a student’s ability to recognize, understand, and predict the sequence of numbers according to specific rules. These worksheets are fundamental tools in mathematics education, facilitating the development of numeracy, number sense, problem-solving abilities, and critical thinking skills. They come in various forms, each targeting different aspects of mathematical learning and cognitive development.
Through various exercises, they cultivate essential skills such as pattern recognition, numeracy, problem-solving, and critical thinking. Regular practice with these worksheets not only prepares students for more advanced mathematical concepts but also instills a sense of confidence and achievement. Engaging with number patterns in an interactive and thoughtful manner lays the groundwork for lifelong mathematical learning and application.
Types of Exercises
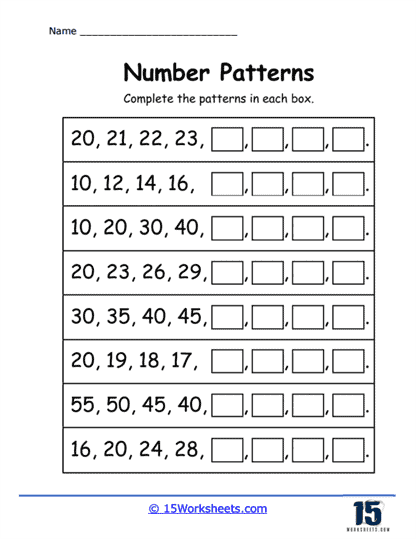
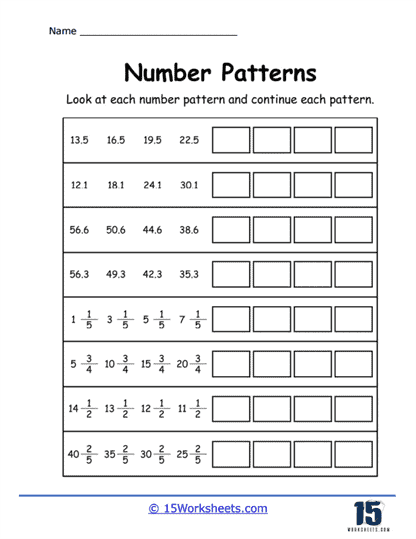
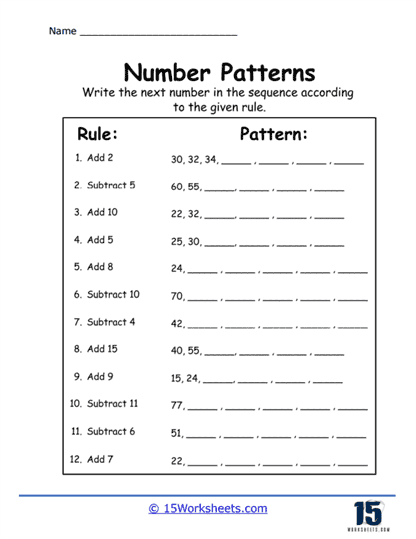
1. Simple Sequential Patterns – These exercises introduce students to the concept of sequences by asking them to identify the next number in a simple pattern, such as increasing by a constant amount (e.g., 2, 4, 6, 8…).
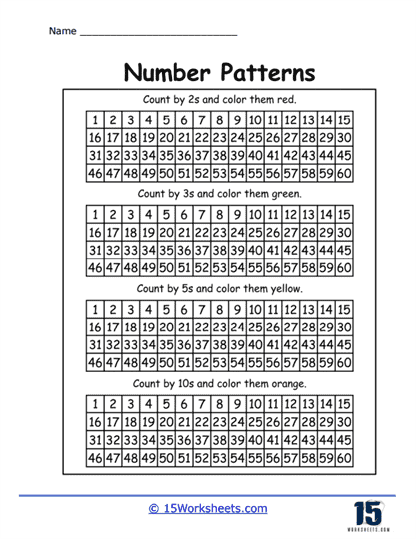
2. Skip Counting – Skip counting exercises involve counting forwards or backwards by numbers other than one, such as by twos, fives, tens, or even non-traditional numbers. This type of exercise helps students recognize patterns in multiplication tables and build a foundation for understanding multiplication and division.
3. Growing and Shrinking Patterns – These patterns require students to identify rules for sequences that increase or decrease by progressively larger or smaller amounts, respectively. This type of exercise challenges students to think about numbers in a more complex way, using addition and subtraction in varying increments.
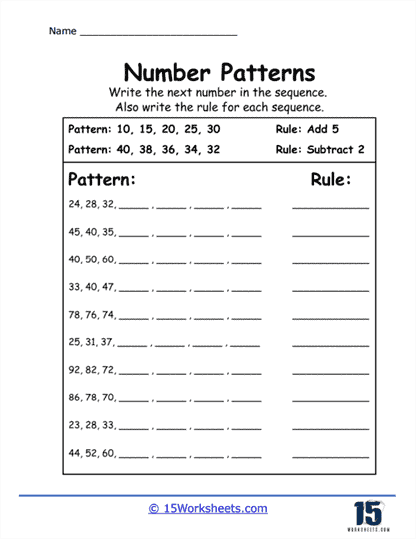
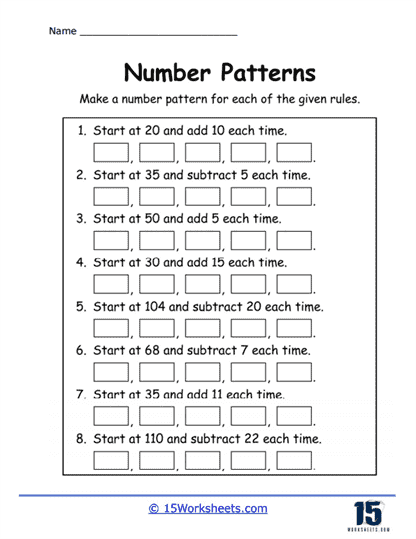
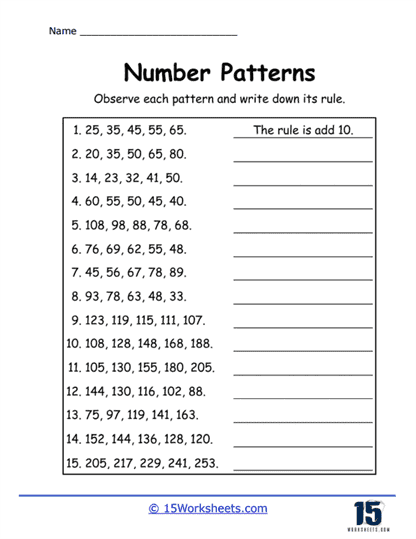
4. Arithmetic Sequences – These exercises involve sequences where each term is obtained by adding (or subtracting) a constant value, known as the common difference, to the previous term. Students must identify the common difference and use it to find missing numbers in the sequence.
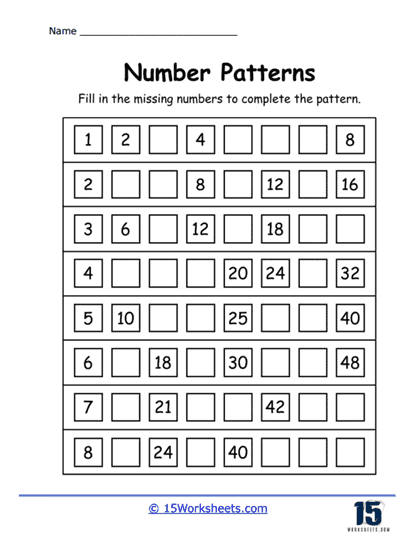
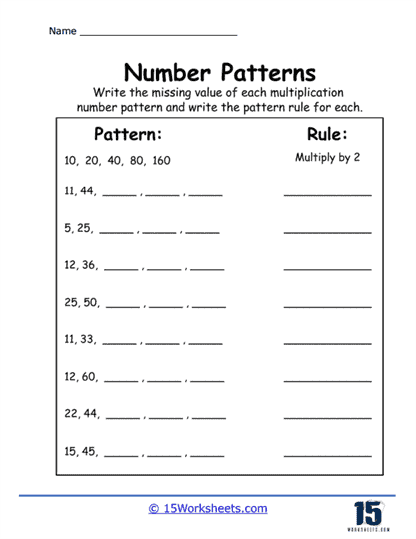
5. Geometric Sequences – In these sequences, each term is found by multiplying (or dividing) the previous term by a constant factor, known as the common ratio. Understanding geometric sequences lays the groundwork for exploring exponential growth and decay.
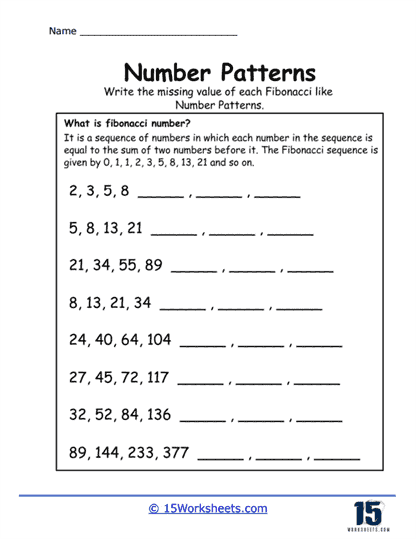
6. Fibonacci Sequence – This sequence is a special pattern where each number is the sum of the two preceding ones. Exercises based on the Fibonacci sequence help students recognize patterns that are not immediately obvious and develop their problem-solving skills.
7. Number Shape Patterns – These worksheets link numbers to geometric shapes, asking students to identify patterns based on the arrangement or number of shapes. This approach integrates spatial understanding with numerical patterns.
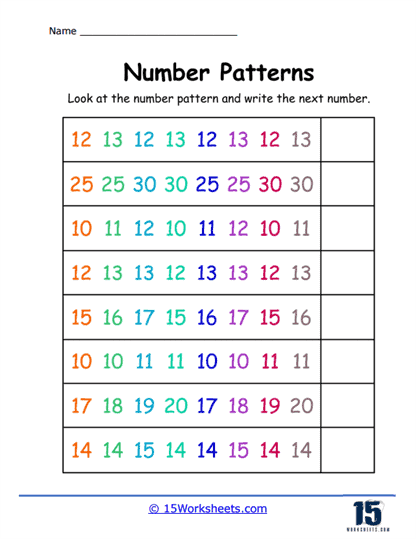
8. Repeating Patterns – Exercises with repeating patterns involve sequences that cycle through a set of numbers. Students must identify the cycle and predict which numbers will come next.
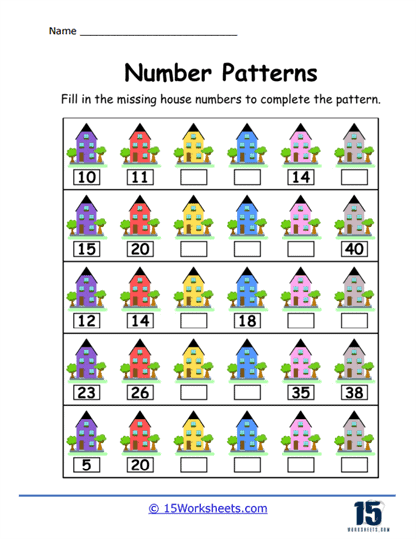
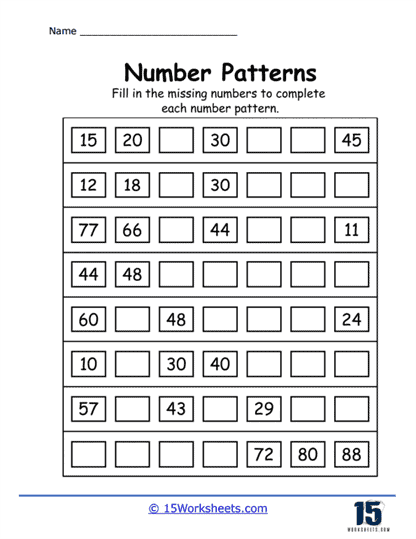
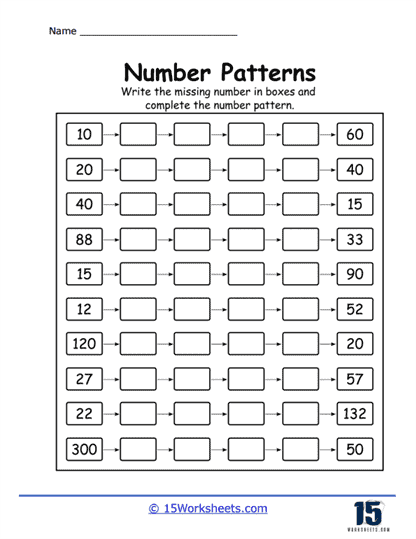
9. Missing Number Patterns – Students are given sequences with missing numbers and must determine the missing values based on the identified pattern. This type of exercise enhances problem-solving abilities and attention to detail.
The Benefits of These Worksheets
Enhances Pattern Recognition – Practicing with number patterns worksheets sharpens the ability to recognize and predict sequences, a skill crucial in mathematics and other disciplines. It helps students to see relationships and connections between numbers, fostering a deeper understanding of mathematical concepts.
Improves Numeracy and Number Sense – These worksheets help students develop a strong sense of numbers, their values, and their relationships. Students learn to manipulate numbers in various ways, enhancing their fluency with numbers and arithmetic operations.
Boosts Problem-Solving Skills – Number patterns worksheets present challenges that require students to apply logical steps to find solutions. This nurtures the ability to approach problems systematically, developing strategies that can be applied in diverse mathematical contexts and in real life.
Develops Critical Thinking – Identifying and predicting number patterns require analytical thinking, as students must evaluate information, make inferences, and justify their reasoning. This process encourages students to think critically and make decisions based on evidence. Working with number patterns helps students to reason mathematically, making connections between concepts and applying mathematical principles to solve problems. This foundation is essential for understanding more complex mathematical ideas.
Promotes Creativity – Creating their own number patterns or finding multiple solutions to pattern problems encourages creativity in mathematical thinking. Students learn that there can be more than one approach to solving a problem, fostering flexibility in thinking.
Builds Confidence – Mastery of number patterns can boost students’ confidence in their mathematical abilities. Success in these exercises reinforces their understanding and provides a sense of achievement that motivates further learning. Understanding number patterns is foundational to higher-level mathematics, including algebra, calculus, and beyond. These worksheets prepare students by developing the necessary skills to recognize and manipulate patterns, sequences, and relationships between numbers.