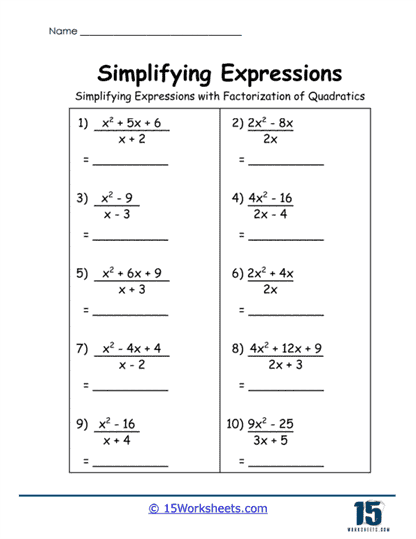Simplifying Expressions Worksheets
All About These 15 Worksheets
The collection of simplifying algebraic expressions Worksheets is a comprehensive and engaging resource designed to support students, teachers, and homeschoolers in mastering the key skill of simplifying algebraic expressions. These worksheets cover a wide array of algebraic concepts, from combining like terms and using the distributive property to handling fractions, decimals, and nested parentheses, offering a versatile learning tool for different levels of mathematical understanding. Each worksheet is carefully crafted to enhance problem-solving skills while reinforcing foundational algebraic principles, making it ideal for classroom use, homework, or independent study.
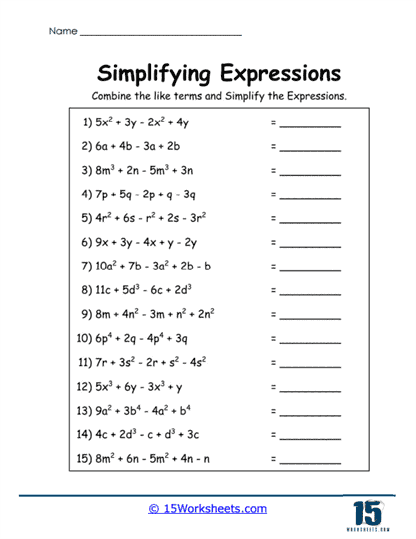
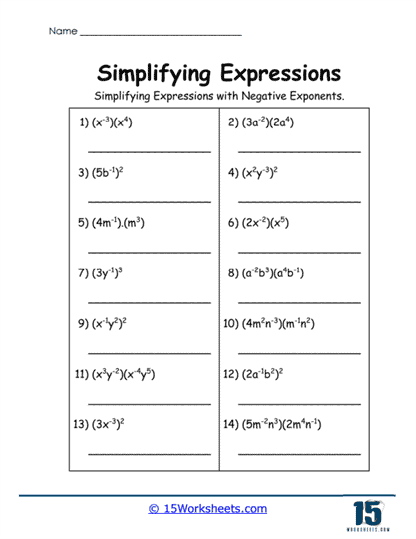
These worksheets encompass a wide range of algebraic activities, offering opportunities for students to practice different techniques and approaches to simplify expressions. Each worksheet addresses a specific skill or concept, ensuring targeted practice. From the basic combination of like terms to more complex activities involving variables, exponents, and decimals, the collection caters to a broad spectrum of learning levels.
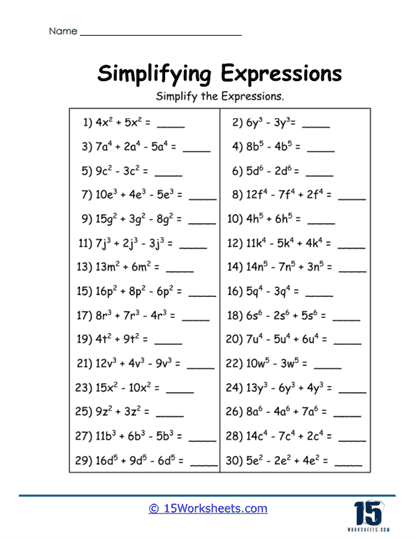
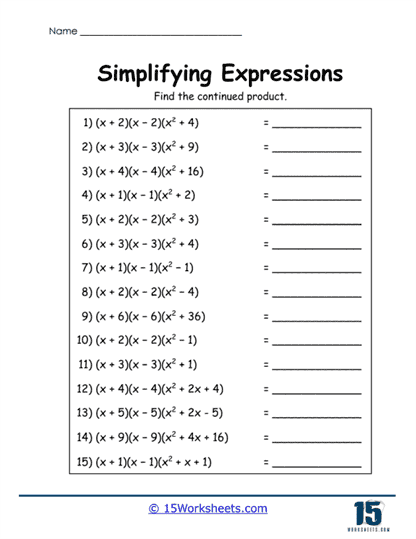
Combining Like Terms – Some worksheets in this collection focus on simplifying expressions by combining like terms. For example, expressions such as 4x2 + 5x2 or 7a2 + 2a2 provide students with the chance to work through problems where they must combine terms with the same variable and degree. This activity helps learners understand how to efficiently reduce expressions to their simplest forms and is foundational in algebra.
Simplifying Expressions Involving Subtraction and Negative Terms – Many of the worksheets introduce expressions that require handling subtraction and negative terms, such as 6y2 – 3y2 or 9c2 – 3c2. These problems enhance students’ ability to manage and simplify terms with negative signs, a critical skill in higher-level algebraic manipulation.
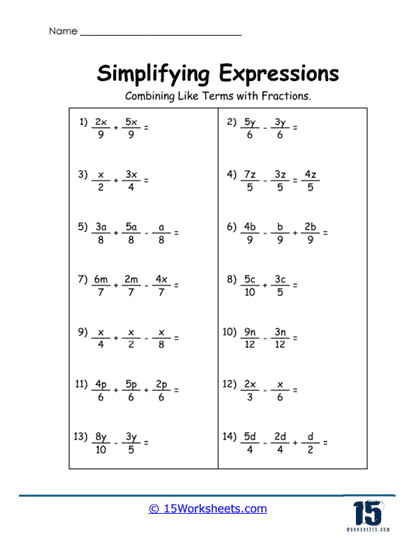
Fractions in Algebraic Expressions – For students needing to refine their skills in working with fractions, several worksheets include expressions with fractions. Problems like 2x/9 – 5x/9 or 7z/5 – 3z/5 allow learners to practice combining fractions with common denominators, further reinforcing their fraction arithmetic while applying it to algebraic contexts.
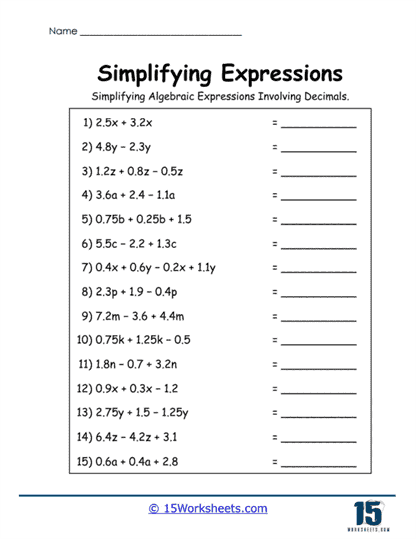
Simplifying Expressions with Decimals – Students will also find worksheets that incorporate decimals into algebraic expressions. These problems, such as 2.5x + 3.2x or 4.8y – 2.3y, encourage learners to work with both decimals and variables, helping them become more comfortable simplifying expressions that involve non-integer coefficients.
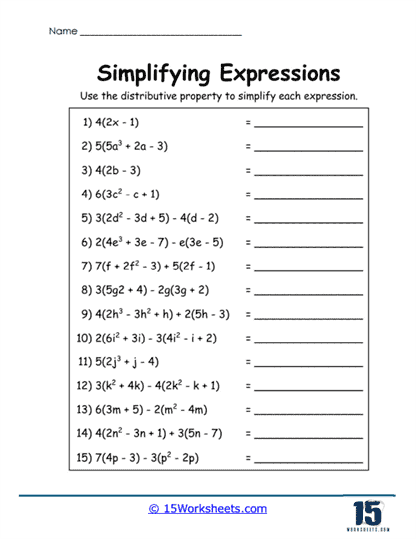
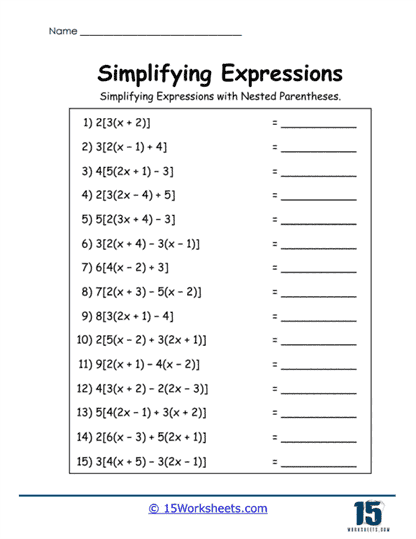
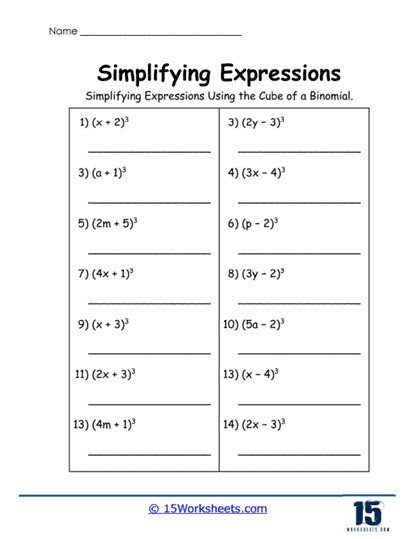
Handling Nested Parentheses – A more advanced set of worksheets focuses on simplifying expressions with nested parentheses. These worksheets challenge students to apply the distributive property effectively and work through complex structures, such as 3[2(x + 1) – 4]. Mastering this skill is essential for success in more advanced algebra and calculus.
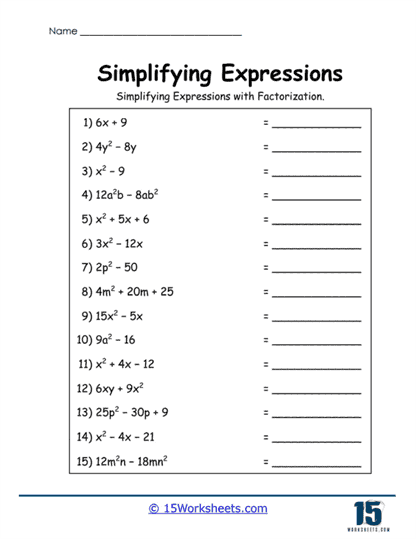
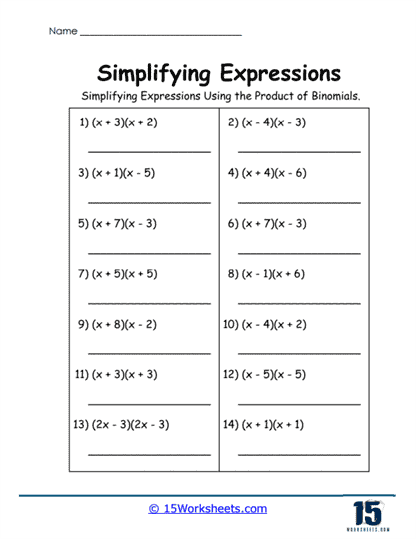
Factorization – Some worksheets help students practice simplifying expressions through factorization, such as reducing quadratic expressions like x2 + 5x + 6 or 9a2 – 16. Factorization is an essential algebraic skill, and these worksheets guide students through the process of identifying common factors and applying them to simplify expressions.
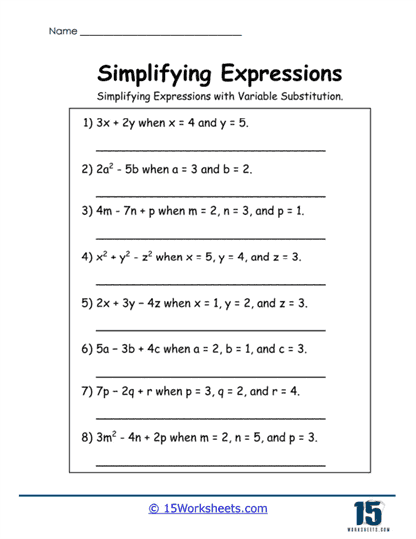
Variable Substitution – The collection also includes worksheets where students are asked to substitute specific values for variables and then simplify the resulting expressions. These problems, such as 3x + 2y when x = 4 and y = 5, encourage students to apply their simplification skills in practical scenarios, bridging the gap between abstract algebraic concepts and real-world applications.
The versatility of this collection makes it a valuable tool for both teachers in traditional classrooms and homeschool educators. Teachers can integrate these worksheets into daily lessons, assigning them as practice work, group activities, or homework. Homeschoolers will appreciate the self-contained nature of the worksheets, which are easy to follow and ideal for independent study.
All worksheets in this collection are conveniently available in PDF format, ensuring they are easy to view, download, and print. This makes the worksheets accessible for both teachers and students, whether they are in the classroom, at home, or on the go. The clear layout and design of the worksheets promote ease of use, enabling students to focus on the task at hand without unnecessary distractions.
Why We Simplify Expressions
Simplifying expressions is a fundamental concept in mathematics that involves reducing complex mathematical expressions to their simplest form. This process makes the expressions easier to understand, evaluate, and use. An expression is a combination of numbers, variables (like x or y), and mathematical operations such as addition, subtraction, multiplication, and division. Simplifying often involves combining like terms, performing arithmetic operations, and applying algebraic rules such as the distributive property or factoring. The goal is to rewrite the expression in a more manageable or concise form without changing its value or meaning.
At its core, simplifying expressions makes it easier to work with and solve equations. For example, consider the expression 2x + 4x – 3 + 5. This can be simplified by combining like terms (terms that involve the same variables). The result is 6x + 2, a simpler and more compact expression. By reducing the complexity of the expression, we make further mathematical operations, such as solving for x or plugging in values, much easier. Simplification also helps in identifying patterns or relationships within the expression that may not be immediately obvious in its original, more complex form.
In the real world, simplifying expressions is essential across various fields. One common application is in engineering, where professionals often deal with complex formulas and equations. For instance, electrical engineers work with circuits that involve multiple variables like voltage, current, and resistance. Simplifying these expressions helps them model and understand how different components interact and ensure their designs are functional and efficient. In physics, simplification is crucial when working with formulas like Newton’s second law of motion (F = ma). By simplifying expressions, physicists can focus on the key relationships and predict outcomes more easily.
Simplifying expressions also plays a critical role in computer science, particularly in algorithms and programming. When writing code, it’s important to reduce the complexity of calculations to ensure that programs run efficiently. Simplified expressions can lead to faster computations and less memory usage, which is especially important in fields like data science, where large datasets are processed. For example, optimizing algorithms that deal with large amounts of data often involves simplifying expressions and operations to improve speed and accuracy.
In finance, simplifying expressions helps with calculating interest, investments, and taxes. Financial models often include complex equations with multiple variables, and simplifying these expressions helps analysts and accountants make better decisions. For instance, compound interest formulas or profit and loss calculations can become overwhelming when dealing with large numbers, and simplifying expressions makes it easier to interpret financial data and trends.
The importance of simplifying expressions extends beyond specific technical fields; it is a critical skill in everyday life. Whether calculating discounts, understanding loan payments, or planning a budget, simplifying mathematical expressions helps individuals make sense of numbers and make informed decisions. For example, when comparing two different loan offers with varying interest rates and terms, simplifying the associated cost equations helps determine which offer is better in the long run.