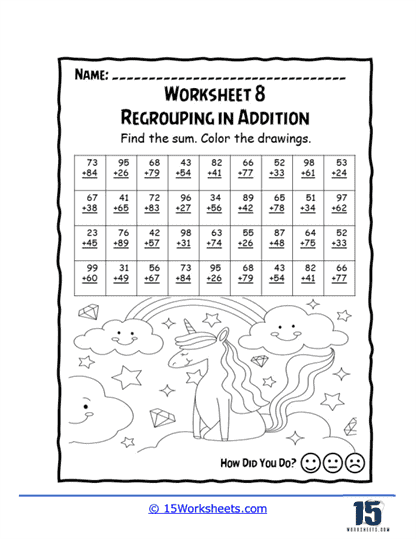
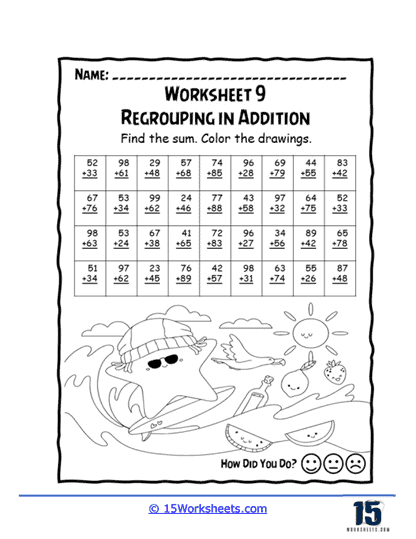
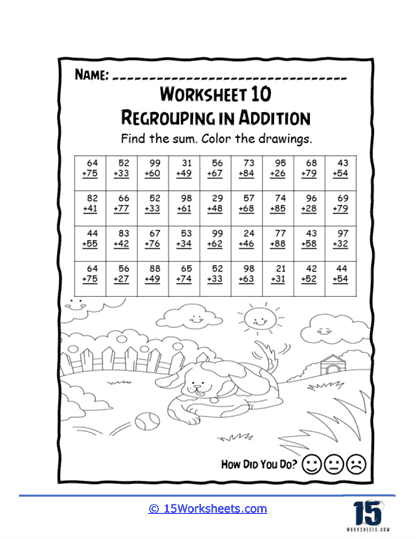
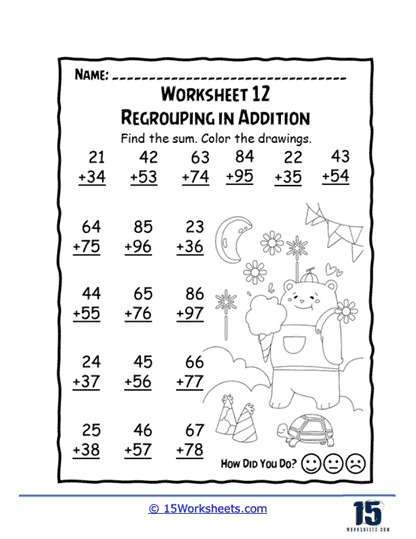
Regrouping in Addition Worksheets
About These 15 Worksheets
Regrouping in addition, often referred to as “carrying over,” is a fundamental mathematical technique used when the sum of two digits in a column exceeds nine, requiring the digit in the ones place to be carried over to the tens column. These worksheets present a variety of exercises that target different aspects of the regrouping process, ensuring that students grasp the concept thoroughly and can apply it confidently in their math operations. By practicing with these worksheets, students can significantly improve their understanding of basic math operations, enhance their problem-solving skills, and build a solid foundation for more advanced mathematical concepts.
Through a variety of exercises, these worksheets not only teach the specific technique of regrouping but also foster a deeper understanding of fundamental math concepts, problem-solving abilities, and cognitive skills. Regular practice with these worksheets can significantly improve students’ proficiency in basic math operations, setting a strong foundation for their future mathematical learning and academic success.
Types of Exercises
Single-digit Regrouping – The simplest form of regrouping exercises involves the addition of two single-digit numbers that result in a two-digit number. This introduces the concept of carrying over the extra value to the next column.
Double-digit Addition with Regrouping – These exercises involve adding two double-digit numbers where regrouping is necessary in the ones or tens place. It’s a step up in complexity, requiring students to understand how to carry over values across columns.
Triple-digit and Beyond – As students become more comfortable with the concept, worksheets include addition problems involving three-digit numbers or more, incorporating multiple instances of regrouping. This type of exercise challenges students to apply regrouping principles across various place values.
Mixed Problems (Regrouping and Non-regrouping) – Some worksheets mix problems that require regrouping with those that do not. This variation ensures that students understand when and why regrouping is necessary, improving their overall number sense and addition skills. Some worksheets challenge students to identify missing numbers in an addition equation that involves regrouping. This type of problem tests students’ understanding of the regrouping process and their ability to reverse-engineer addition problems.
Column Addition with Regrouping – Exercises where students practice adding numbers vertically in columns emphasize the structural aspect of regrouping. These problems often include multiple digits and require careful alignment of numbers by their place value.
The Benefits of These Worksheets
Practicing with regrouping in addition worksheets offers numerous benefits that extend beyond mastering the specific skill of regrouping:
Enhanced Number Sense – Regular practice with these worksheets helps students develop a deeper understanding of place value and the decimal system, foundational concepts in mathematics that are critical for understanding more complex operations and concepts. Through repeated practice, students become more accurate and faster at performing addition operations, including those that require regrouping. This efficiency is crucial for success in mathematics, where complex problems often involve multiple steps of calculation.
Strengthened Problem-solving Skills – The variety of exercises on these worksheets, especially word problems and puzzles, enhances students’ problem-solving abilities. They learn to approach problems methodically, breaking them down into simpler parts and applying their knowledge of regrouping where necessary. Mastery of regrouping through practice builds students’ confidence in their mathematical abilities. This confidence can lead to a more positive attitude towards math and a willingness to engage with more challenging concepts.
Preparation for Advanced Math Concepts – A solid grasp of addition with regrouping prepares students for advanced mathematical concepts that rely on this foundational skill, such as subtraction with borrowing, multiplication, and division of large numbers, and understanding fractions and decimals. The process of aligning numbers correctly, identifying when regrouping is necessary, and accurately carrying over values requires careful attention to detail. This skill is invaluable in mathematics and other academic disciplines. The mental process involved in addition with regrouping exercises critical thinking, memory, and cognitive flexibility, as students must remember and apply multiple steps and rules. These cognitive skills are essential for academic success across subjects.
What Is Regrouping in Addition?
Regrouping in addition is a mathematical technique used when adding two or more numbers together and the sum in any of the place values exceeds 9. This process involves carrying over the excess amount to the next higher place value to ensure that each digit in the answer correctly represents its place value. Regrouping is fundamental in understanding how to perform addition operations with multi-digit numbers effectively and accurately, laying the groundwork for more complex arithmetic operations. The concept is essential for developing a strong mathematical foundation, particularly in understanding place value and the base-ten number system.
When adding numbers, we typically start with the rightmost digits, which represent the units or ones place. If the sum of these digits is 10 or more, we need to regroup. To regroup, we keep the unit’s digit of the sum in the same column and carry over the digit(s) representing the tens (or higher place values) to the next left column, adding it to the sum of the digits in that column. This process may cascade, requiring regrouping at successive place values if those sums also exceed 9.
Regrouping ensures that each digit in the sum accurately represents its place value in the base-ten system. This process can be visualized through various methods, such as using physical counters (e.g., blocks or beads), pictorial representations (e.g., drawings of blocks), or abstract numerical methods (e.g., writing the numbers in columns and carrying over).
Example: Let’s add 47 and 58.
Step 1) Add the ones place digits. 7 (from 47) + 8 (from 58) = 15. Since 15 is more than 9, we need to regroup. We write the 5 in the ones place of the sum and carry the 1 (representing 10) over to the tens place.
47
+ 58
5
Step 2) Add the tens place digits along with the carried over 1. 4 (from 47) + 5 (from 58) + 1 (carried over) = 10. Again, since 10 is more than 9, we write the 0 in the tens place of the sum and carry over the 1 (representing 100) to the next higher place value, which, in this case, adds a new digit to the left of the sum.
47
+ 58
105
Final Sum: The result is 105.