Associative Property Worksheets
About These 15 Worksheets
These worksheets present a variety of exercises designed to elucidate the Associative Property’s concept, fostering critical thinking and problem-solving skills. By engaging with a diverse range of exercises, students not only grasp the essence of the Associative Property but also hone their proficiency in basic math operations.
These worksheets are meticulously crafted to cater to different skill levels, offering progressive challenges that scaffold students’ learning experiences. Through a combination of visual representations, numerical expressions, and word problems, students delve into the intricacies of the Associative Property, unraveling its significance in mathematical operations.
Through a diverse array of exercises encompassing numerical expressions, visual representations, word problems, and algebraic contexts, students engage in meaningful learning experiences that foster critical thinking, problem-solving proficiency, and algebraic fluency. By mastering the concept of the Associative Property, students lay a solid foundation for future mathematical endeavors, equipping them with essential skills for success in mathematics and beyond.
Types of Exercises
Numerical Expressions
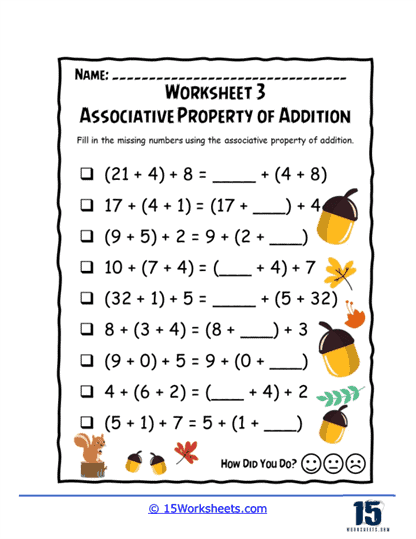
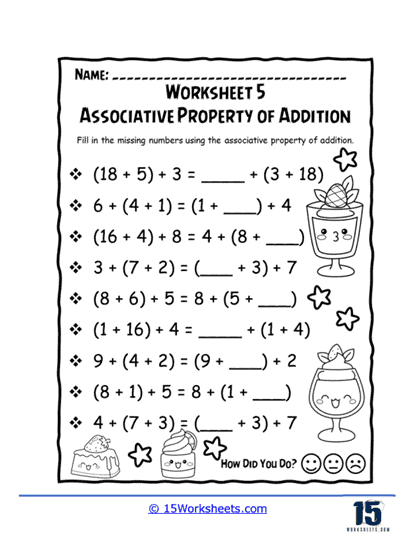
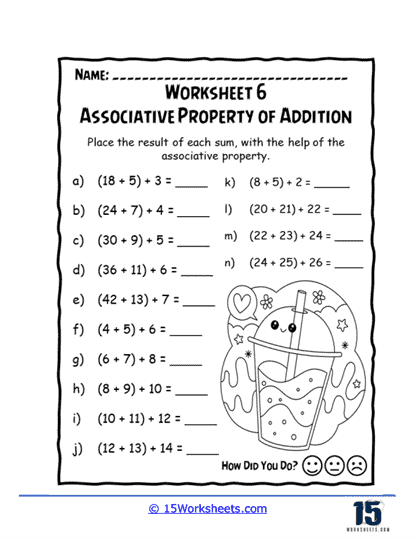
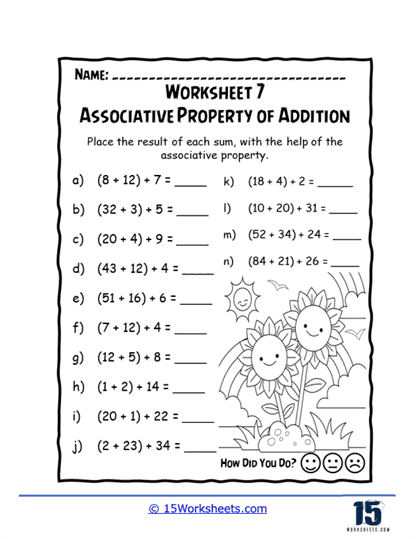
At the foundational level, Associative Property worksheets often feature exercises involving numerical expressions. Students are presented with addition expressions containing multiple terms, and they must apply the Associative Property to regroup the terms without altering the sum. For example – (3 + 5) + 2 = 3 + (5 + 2)
By rearranging the terms, students recognize that the sum remains constant regardless of the grouping.
Visual Representations
Visual representations play a pivotal role in reinforcing the concept of the Associative Property. Worksheets may include diagrams or illustrations depicting sets of objects or counters representing numerical values. Students are tasked with grouping the objects in different ways while maintaining the total count. This visual approach aids in developing students’ conceptual understanding and geometric reasoning skills.
Variable Expressions
Advanced Associative Property worksheets may incorporate variable expressions, allowing students to generalize the concept beyond specific numerical values. Students manipulate algebraic expressions containing variables to demonstrate the property’s applicability in algebraic contexts. For example – a + (b + c) = (a + b) + c
Students recognize that the Associative Property holds true for variables as well, regardless of their values.
Missing Number Problems
Another type of exercise found in Associative Property worksheets involves missing number problems. Students are presented with partially completed expressions or equations, and they must determine the missing number to maintain the validity of the property. For instance – 8 + (__ + 4) = (__ + 8) + 4
By identifying the missing numbers, students demonstrate their understanding of the property’s application in numerical expressions.
Proofs and Justifications
In more advanced worksheets, students may be challenged to provide proofs or justifications for the validity of the Associative Property. They are tasked with explaining why the property holds true and providing examples or counterexamples to support their reasoning. This higher-order thinking skill fosters a deeper conceptual understanding of mathematical principles.
The Benefits
Engaging with Associative Property of Addition Worksheets offers numerous benefits for students as they develop their mathematical reasoning and problem-solving skills:
Conceptual Understanding
Through a variety of exercises encompassing numerical expressions, visual representations, and word problems, students develop a profound conceptual understanding of the Associative Property. They grasp the fundamental principle that the grouping of numbers in addition operations does not alter the sum, regardless of the context.
These worksheets encourage students to think critically and analytically as they manipulate numerical expressions and solve problems. By exploring different ways of grouping numbers and evaluating their implications, students hone their deductive reasoning abilities and mathematical intuition.
Problem-Solving Proficiency
Integrating word problems into worksheets challenges students to apply the Associative Property in real-life scenarios, fostering problem-solving proficiency. Students learn to identify relevant information, formulate strategies, and communicate their solutions effectively, thus enhancing their overall mathematical literacy.
Exposing students to variable expressions within Associative Property worksheets cultivates algebraic fluency and abstraction skills. Students generalize the concept beyond specific numerical values, gaining insight into the algebraic structure underlying addition operations.
The skills acquired through practicing with Associative Property worksheets extend beyond addition operations, benefiting students across various mathematical domains. The ability to recognize patterns, manipulate expressions, and justify mathematical claims enhances students’ problem-solving capabilities and prepares them for advanced mathematical coursework.
What Is the Associative Property of Addition?
The Associative Property of Addition is a fundamental concept in mathematics that describes how the grouping of numbers in an addition operation does not affect the result. In simpler terms, it means that when adding three or more numbers together, the way they are grouped (parenthesized) does not change the sum. This property holds true regardless of the order in which the numbers are grouped.
Mathematically, the Associative Property of Addition can be expressed as follows:
For any three numbers a, b, and c, the sum of a and the sum of b and c are equal:
a + (b + c) = (a + b) + c
In this equation, a, b, and c can represent any real numbers, integers, fractions, or even variables.
To understand the Associative Property better, let’s explore it in detail with some examples:
Example 1 – 3 + (4 + 5) = (3 + 4) + 5
Here, we are adding the numbers 3, 4, and 5. According to the Associative Property, it doesn’t matter if we first add 4 and 5 together, or if we add 3 and 4 together. In either case, the final sum will be the same.
Example 2 – (2 + 7) + 6 = 2 + (7 + 6)
Again, in this example, the order of grouping the numbers changes, but the sum remains the same. This demonstrates the Associative Property in action.
Example 3 – (-5) + [(-8) + (-3)] = [(-5) + (-8)] + (-3)
Even when dealing with negative numbers, the Associative Property holds true. The grouping of negative numbers does not affect the final result of the addition operation.
Example 4 – x + (y + z) = (x + y) + z
In algebraic expressions, variables can also be used to represent numbers. The Associative Property applies to variables as well, meaning that regardless of how variables are grouped, the sum remains unchanged.
Understanding and applying the Associative Property is crucial in various areas of mathematics, including arithmetic, algebra, and even more advanced mathematical concepts. It allows mathematicians to simplify expressions, rearrange terms, and perform calculations more efficiently.
One practical application of the Associative Property is in mental math and problem-solving. By recognizing patterns and knowing that the grouping of numbers doesn’t affect the result, individuals can simplify complex addition operations and arrive at solutions more quickly.