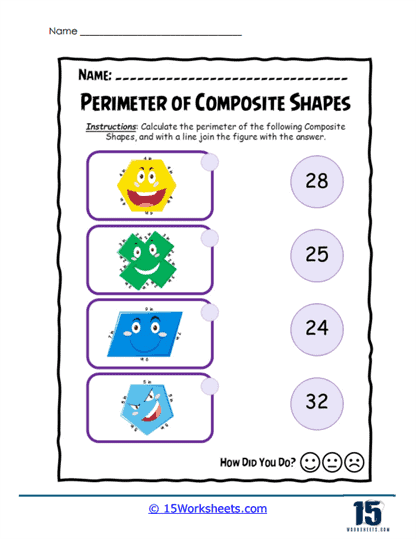
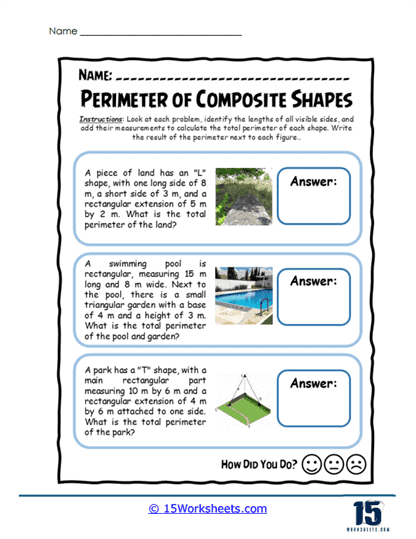
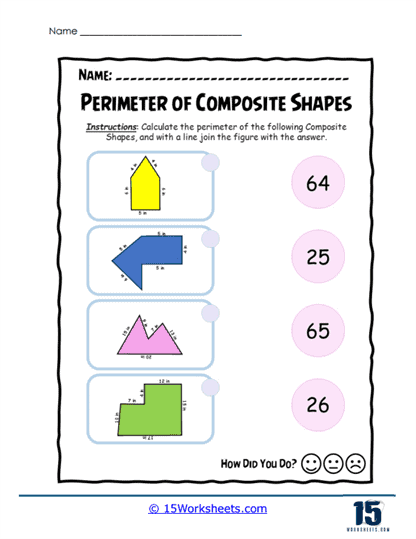
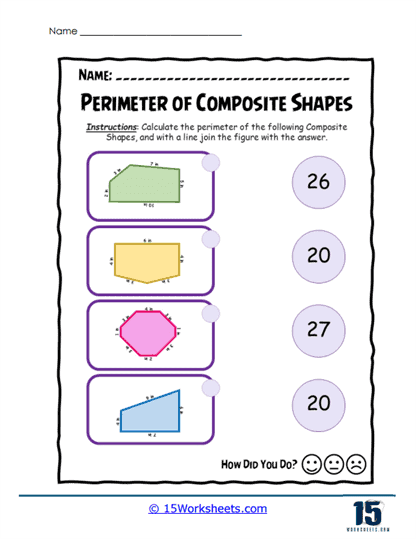
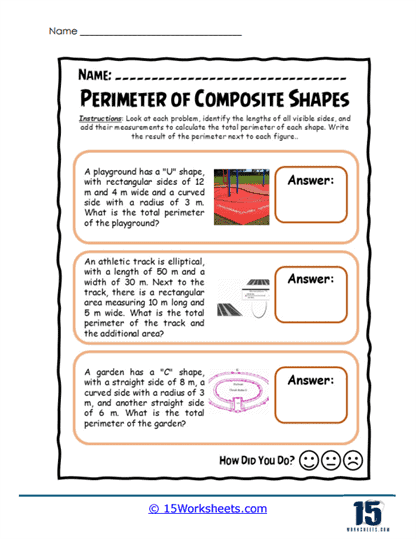
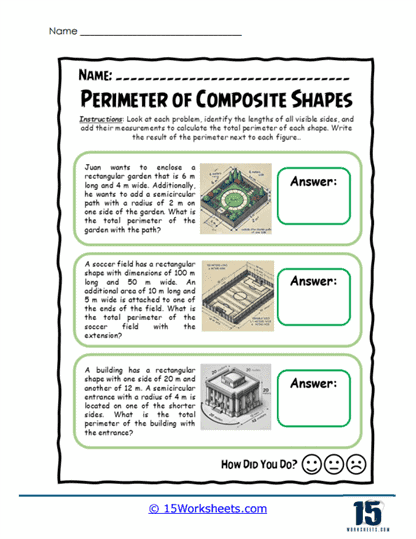
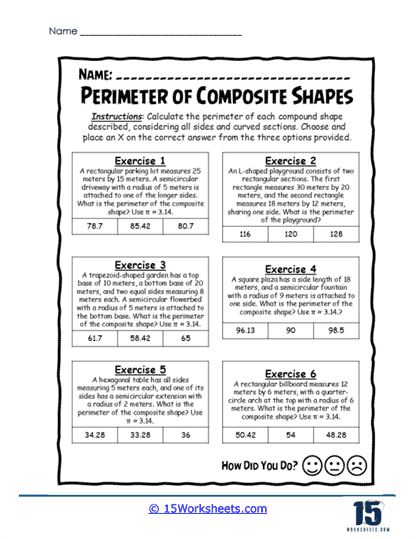
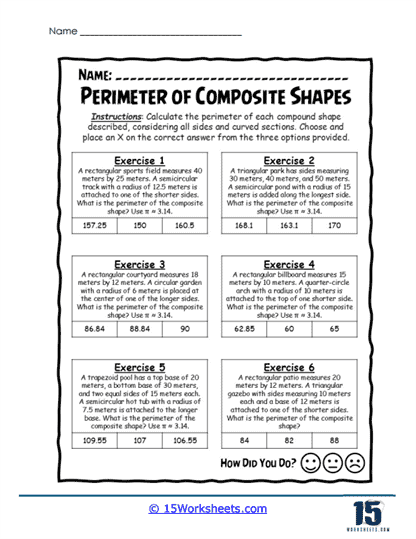
Composite Shapes Worksheets
About Our Perimeter of Composite Shapes Worksheets
Understanding the concept of perimeter is essential for students as they build a strong foundation in geometry, measurement, and spatial reasoning. A solid grasp of perimeter calculations helps learners develop critical thinking and problem-solving skills, which are applicable to both academic and real-world situations. The ability to calculate the perimeter of various shapes is not only a key math skill but also a stepping stone to more advanced topics, such as area, volume, and coordinate geometry. By practicing perimeter calculations, students enhance their mathematical fluency and confidence, enabling them to tackle more complex problems with ease.
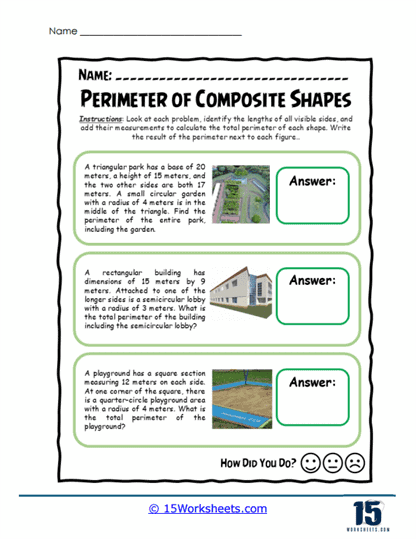
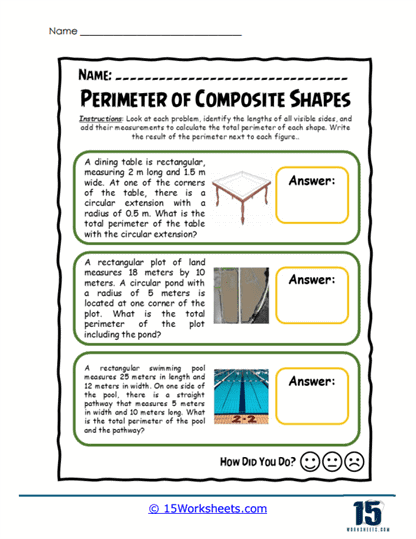
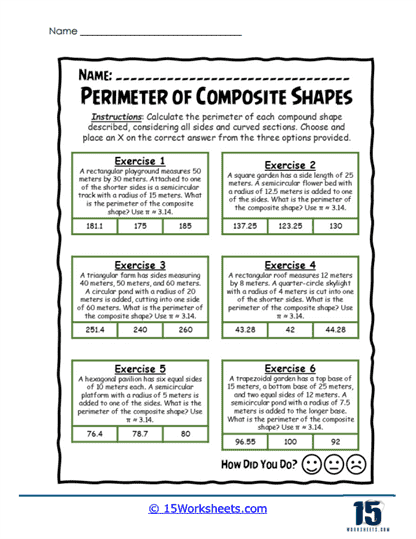
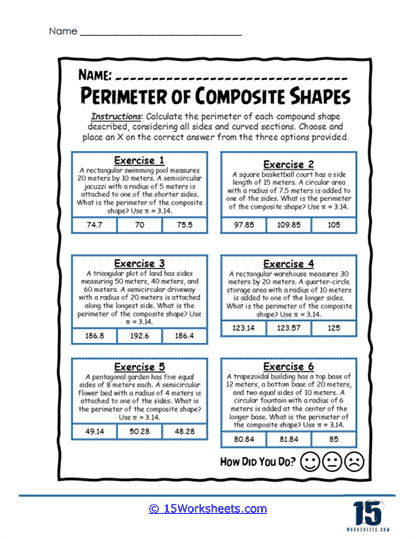
To support students in mastering this concept, the Perimeter of Composite Shapes Worksheets collection offers structured and engaging exercises. These worksheets are specifically designed to help students identify, decompose, and calculate the perimeter of irregular figures composed of multiple simple shapes. Instead of working with only basic squares and rectangles, learners will engage with composite figures that require them to think critically about how different segments connect and contribute to the total perimeter. By gradually increasing in difficulty, the worksheets cater to a wide range of learning levels, from elementary to middle school students.
One of the key features of this worksheet collection is the inclusion of various problem-solving strategies to approach perimeter calculations. Students will practice decomposing composite shapes into smaller, more manageable figures like rectangles, triangles, and circles. Additionally, they will apply addition and subtraction methods to determine missing side lengths, reinforcing their understanding of basic arithmetic within a geometric context. By incorporating multiple approaches, these worksheets provide a well-rounded learning experience that accommodates different problem-solving styles and abilities.
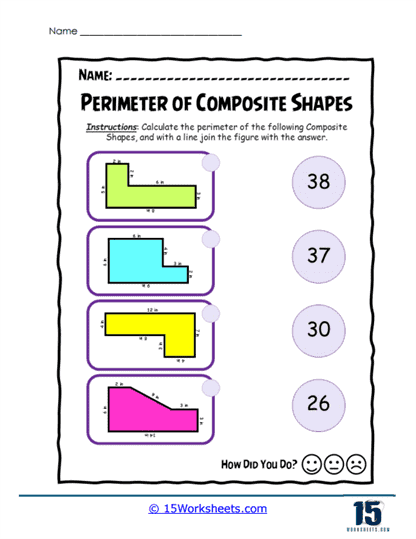
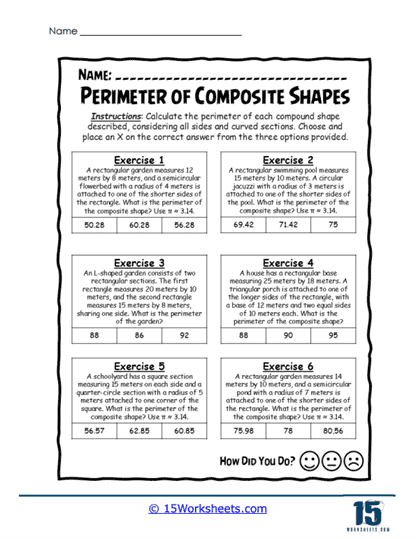
To enhance visualization and comprehension, some worksheets include grid-based exercises where students count unit lengths to estimate the perimeter of a shape. This method is particularly useful for learners who benefit from hands-on or visual learning experiences. By working with grids, students can see how individual line segments contribute to the overall perimeter, making the concept more intuitive. Furthermore, real-world word problems included in the collection encourage students to apply their knowledge in practical scenarios, such as fencing a yard or outlining a sports field, bridging the gap between theory and application.
What Are Composite Shapes?
Composite shapes are figures made up of two or more simple geometric shapes, such as rectangles, triangles, circles, or other polygons. Instead of having a single, uniform shape, composite shapes combine these basic forms into more complex figures. For example, an L-shaped figure can be seen as two rectangles joined together, while a house-like structure might consist of a rectangle and a triangle. Understanding composite shapes is essential in geometry because they appear frequently in real-world applications, from designing buildings and parks to solving engineering and construction problems.
Understanding this is an essential skill in geometry, as it requires students to break down complex figures into simpler, more manageable components. By identifying familiar shapes within a composite figure, students can apply appropriate mathematical formulas to determine key measurements such as area and perimeter. This process often involves not only recognizing how the smaller shapes fit together but also solving for missing dimensions, reinforcing their ability to think critically and systematically. Through practice, learners develop confidence in manipulating different geometric forms, making it easier to approach more advanced mathematical concepts.
How to Determine the Perimeter of a Composite Shapes
To determine the perimeter of a composite shape, start by identifying its outer boundary. A composite shape is made up of multiple basic geometric figures (such as rectangles, triangles, or semicircles) joined together. The perimeter is the total length of all the outer edges, so carefully trace the shape’s exterior and add up the lengths of all sides that form the boundary. If curved edges are present, use the appropriate formulas-such as the circumference formula for circles (C = 2πr) or arc length formulas-to determine their contribution to the perimeter.
It is crucial to exclude internal boundaries where shapes are joined, as these do not contribute to the outer perimeter. If some side lengths are missing, use geometric properties or given dimensions to determine them. For example, in a composite shape made of rectangles, opposite sides are often equal, allowing for easier calculations. For semicircles or quarter circles, calculate their curved perimeters separately and add them to the total. Once all the relevant edges are accounted for, sum them up to find the perimeter of the composite shape.
Beyond the mathematical calculations, working with composite shapes enhances students’ spatial reasoning and problem-solving abilities. Recognizing how various shapes combine to form a larger figure helps learners visualize geometric relationships and strengthens their ability to estimate measurements accurately. These skills are particularly valuable in real-world applications, where precise calculations are necessary for designing structures, planning layouts, and optimizing space. By engaging with composite shapes, students cultivate a deeper appreciation for geometry as more than just numbers and formulas-it becomes a practical tool for solving everyday problems.
Mastering the concepts of composite shapes also has practical implications outside the classroom. Whether determining the amount of flooring material needed for an irregularly shaped room or calculating the border length of a garden with multiple sections, these skills translate directly to real-life tasks. Architects, engineers, landscapers, and designers regularly rely on an understanding of composite shapes to complete projects efficiently and accurately. By honing their ability to deconstruct complex figures and apply geometric principles, students not only excel academically but also gain problem-solving strategies that serve them well in various professional and personal endeavors.
Composite shapes are not only important in mathematics but also play a crucial role in various real-world professions and daily tasks. Architects and engineers rely on composite shape calculations when designing buildings, bridges, and landscapes, ensuring that structures are both functional and aesthetically pleasing. Carpenters and interior designers use these concepts to measure materials accurately for flooring, furniture, and decorative installations. Even artists and game designers apply an understanding of composite shapes when creating intricate patterns, digital environments, and three-dimensional models. By studying composite shapes, students gain valuable skills that extend beyond the classroom, preparing them for practical applications in diverse fields and enhancing their ability to think critically about the space and structures around them.
In real-world scenarios, understanding how to find the perimeter of composite shapes is incredibly useful. From designing gardens, fencing properties, and measuring the borders of irregularly shaped rooms to estimating materials for construction and art projects, perimeter calculations are an essential skill. Students who grasp these concepts early will be better equipped to tackle practical applications in engineering, architecture, landscaping, and even everyday problem-solving tasks. These worksheets provide realistic problems that encourage learners to apply their mathematical knowledge beyond the classroom.