Adding Doubles Worksheets
About These 15 Worksheets
These worksheets are a fundamental part of early math education that significantly aid in developing students’ arithmetic skills. These worksheets focus on a specific type of addition problem-adding two identical numbers together, such as 2 + 2, 5 + 5, or 9 + 9. The concept is simple, yet the impact of mastering this skill is profound, providing a strong foundation for more complex mathematical operations. Adding Doubles worksheets are designed not only to help students memorize these particular sums but also to enhance their overall number sense, mental math abilities, and confidence in handling mathematical problems.
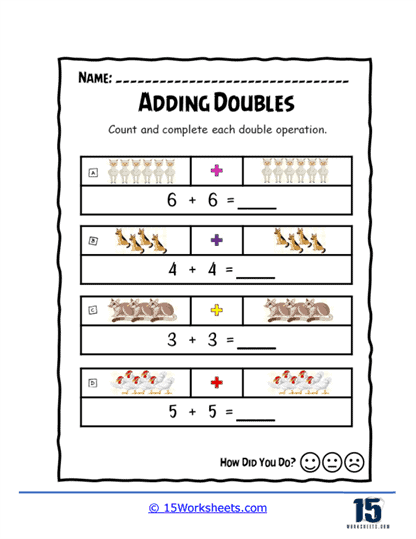
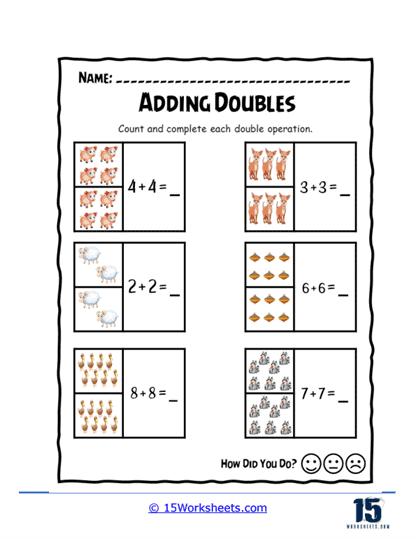
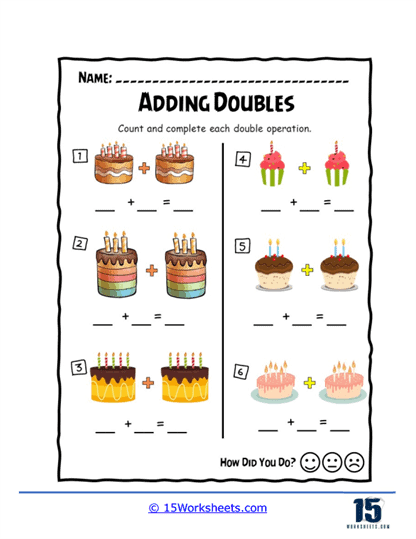
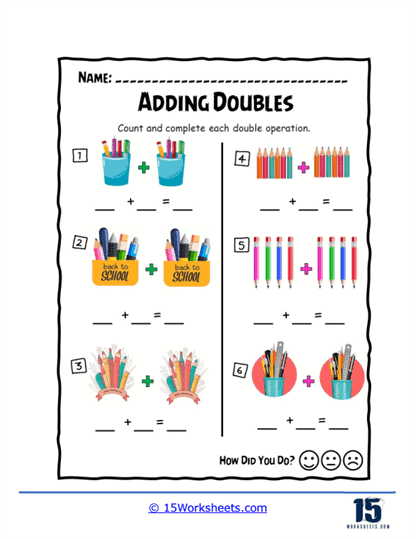
The most common element is a list of addition problems where the student is asked to add two of the same numbers. For example, the worksheet might present problems like 3 + 3, 6 + 6, or 7 + 7, requiring the student to solve each one. These problems are usually arranged in a straightforward format, allowing the student to focus solely on the arithmetic without distractions.
In addition to basic problems, many of the worksheets include visual aids such as pictures or diagrams. For instance, a problem might show two groups of four apples, helping the student visually understand that 4 + 4 equals 8. This use of imagery is especially beneficial for younger students who are just beginning to grasp the concept of addition, as it connects the abstract idea of numbers with tangible objects they can easily understand.
Another common feature of this set of worksheets is the use of number lines. A number line provides a visual representation of the addition process, allowing students to see how they move forward when they add two identical numbers. For example, to solve 5 + 5, a student can start at the number 5 on the number line and then make five jumps forward to reach 10. This helps reinforce the idea of addition as a process of counting forward and solidifies their understanding of how numbers relate to each other on a linear scale.
Some of the worksheets take the concept a step further by including word problems. These problems require students to apply their knowledge of doubles in a more practical context. For instance, a worksheet might pose a question like, “If you have 4 apples and your friend gives you 4 more, how many apples do you have in total?” This type of exercise not only reinforces the arithmetic involved in adding doubles but also helps students develop their problem-solving skills by translating a real-world scenario into a mathematical equation.
To add variety and keep students engaged, many of the worksheets also incorporate puzzles and games. For example, a worksheet might feature a matching activity where students must pair a sum with the correct addition problem. Another common game is a “fill in the blank” exercise, where part of the equation is missing, and the student must determine the correct number to complete the double. These types of activities make learning more interactive and fun, encouraging students to practice their math skills without feeling like they are simply doing repetitive exercises.
The Benefits of These Worksheets
At the most basic level, these worksheets help students memorize key addition facts, which is a crucial step in building math fluency. Knowing that 6 + 6 equals 12, for example, allows students to solve more complex problems more quickly and with greater confidence. This fluency frees up cognitive resources, enabling students to focus on higher-order problem-solving tasks rather than getting bogged down in basic calculations.
These types of worksheets play a significant role in developing a student’s number sense. Number sense refers to a person’s ability to understand, interpret, and work with numbers in a flexible and intuitive way. By regularly practicing adding doubles, students begin to see patterns in numbers and develop an intuitive grasp of how numbers work together. For example, when a student repeatedly works with problems like 4 + 4 or 8 + 8, they start to recognize that adding doubles always results in an even number. This recognition of patterns is a critical component of number sense and is foundational for more advanced math concepts such as multiplication and algebra.
Another important benefit of the worksheets is the enhancement of mental math skills. Mental math refers to the ability to perform arithmetic calculations without the use of paper, pencil, or calculators. Because the sums of doubles are relatively easy to memorize, students can quickly recall these sums when needed. This ability to quickly and accurately perform addition in their heads is a skill that will serve them well throughout their education and daily life. For example, if a student knows that 7 + 7 equals 14, they can easily use this knowledge to solve related problems, such as 7 + 8, by simply adding one more to 14.
Many students, especially in the early stages of their education, can feel overwhelmed by math. However, by mastering doubles, they gain a sense of achievement and self-assurance in their math abilities. This confidence can lead to a more positive attitude toward math in general, making them more willing to tackle new and challenging concepts. When students feel confident in their math skills, they are more likely to engage actively in lessons, participate in class discussions, and persist through difficult problems.
For students who are struggling, teachers can provide additional support through scaffolding techniques. Scaffolding might include breaking down the problems into smaller, more manageable steps, or providing extra visual aids to help students understand the concept. For example, a teacher might use manipulatives like counters or blocks to physically demonstrate the process of adding doubles. Alternatively, they might encourage students to draw pictures to represent the problem, which can help bridge the gap between concrete and abstract thinking.
For more advanced students, these worksheets can be expanded to include related concepts, such as near doubles. Near doubles involve adding numbers that are close to each other, such as 6 + 7 or 5 + 4. By using their knowledge of doubles, students can learn to quickly solve near doubles by adjusting the sum of the double slightly. For example, if a student knows that 6 + 6 equals 12, they can easily determine that 6 + 7 equals 13 by adding one more to 12. This extension of the concept helps deepen students’ understanding of addition and prepares them for more complex mathematical operations.