Percentages of Shapes Worksheets
About These 15 Worksheets
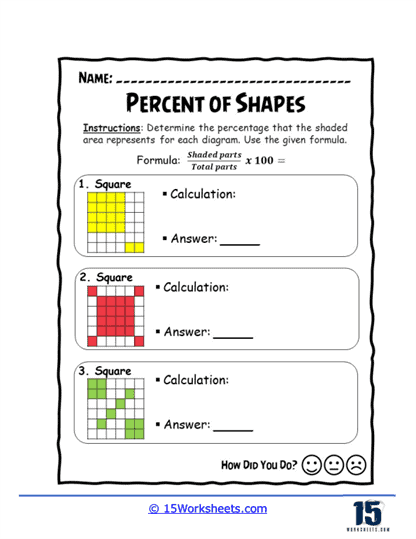
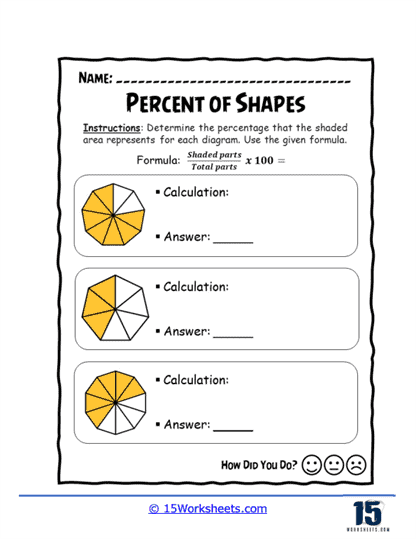
These worksheets will help students understand and practice calculating the percentage of an area that is shaded within a given shape. These worksheets use various geometric shapes, divided into smaller, equal parts, where some sections are shaded to represent a portion of the whole shape. The primary goal of these worksheets is to teach students how to calculate the percentage of the shaded area relative to the entire shape, using the formula: Percentage = (Shaded Parts / Total Parts) x 100.
Each worksheet features several different shapes, such as squares, rectangles, circles, triangles, and more complex polygons. The shapes are divided into equal parts, making it easier for students to count the total number of parts and the number of shaded parts. For example, a square might be divided into a grid of smaller squares, with some squares shaded in. Students are asked to calculate what percentage of the entire shape is shaded by counting the shaded squares and dividing that number by the total number of squares in the grid. This result is then multiplied by 100 to find the percentage.
The use of a variety of shapes and configurations in these worksheets helps reinforce the concept that percentages can apply to any scenario where a part of a whole is considered. For instance, a circle might be divided into equal wedges, with some wedges shaded. Students must calculate the percentage of the circle that is shaded by applying the same formula, demonstrating that the principle works regardless of the shape or configuration of the parts. This reinforces their understanding of percentages as a universal mathematical concept.
These worksheets also include more complex scenarios where shapes are divided into irregular sections, or where the shaded portions are not contiguous. In such cases, students must carefully count each shaded part and ensure that they accurately determine the total number of parts in the shape. This encourages precision and attention to detail, which are crucial skills in mathematics. By dealing with more complex shapes, students also learn to apply the basic principles of percentage calculation to less straightforward problems, thereby enhancing their problem-solving abilities.
One of the key skills taught through these worksheets is the ability to break down a problem into manageable steps. Students learn to approach each problem systematically: first, by identifying the total number of parts in the shape, then by counting the shaded parts, and finally by applying the formula to calculate the percentage. This step-by-step approach is fundamental to mastering more advanced mathematical concepts, where the ability to decompose a problem into smaller, more manageable tasks is often essential.
In addition to teaching percentage calculations, these worksheets also reinforce other important mathematical concepts, such as fractions and ratios. When students calculate the percentage of shaded areas, they are essentially working with fractions, as they determine what fraction of the whole shape is shaded. By multiplying this fraction by 100, they convert it into a percentage, which is a specific type of ratio that compares the shaded part to the whole, expressed as a part of 100. This reinforces their understanding of the relationship between fractions, ratios, and percentages, and helps them see how these concepts are interconnected.
These worksheets include problems that challenge students to think critically about the information presented. For example, a worksheet might ask students to determine how much more or less a shape is shaded compared to another shape. This requires students to not only calculate the percentage of shaded areas for each shape but also to compare these percentages and draw conclusions based on their findings. Such exercises promote higher-order thinking skills, as students must analyze, compare, and evaluate the information before arriving at a solution.
Another important aspect of these worksheets is that they help students develop spatial reasoning skills. As students work with different shapes and configurations, they become more familiar with geometric concepts and learn to visualize how parts of a shape relate to the whole. This is especially important when dealing with irregular shapes or when the shaded parts are not uniform, as students must mentally organize the parts to accurately calculate the percentage. Strengthening spatial reasoning is beneficial not only in mathematics but also in fields such as science, engineering, and technology, where the ability to visualize and manipulate shapes and spaces is often required.
These worksheets also provide an opportunity for students to practice their arithmetic skills, particularly multiplication and division, which are essential for calculating percentages. By repeatedly applying these operations in the context of real-world problems, students become more comfortable with the calculations and improve their overall arithmetic fluency. This fluency is critical for success in mathematics, as it allows students to focus on problem-solving rather than getting bogged down by basic calculations.
The visual nature of the problems makes them particularly effective for introducing these concepts, as students can see the relationships between the parts of a shape and the whole, rather than just dealing with abstract numbers. This concrete representation of mathematical concepts helps students grasp the ideas more quickly and deeply. Additionally, because the worksheets are structured in a way that gradually increases in difficulty, they can be used to differentiate instruction, with more advanced students tackling the more complex problems while others work on the basics.
For homework, these worksheets offer a way for students to reinforce what they have learned in class. By working through the problems independently, students can practice applying the concepts and skills they have been taught, which helps to solidify their understanding. The repetition and variation in the problems ensure that students get ample practice with different types of scenarios, which prepares them for more complex problems they may encounter in the future.