Percentage of Error Worksheets
About These 15 Worksheets
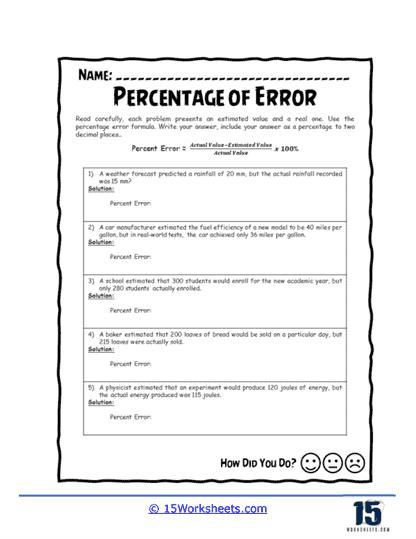
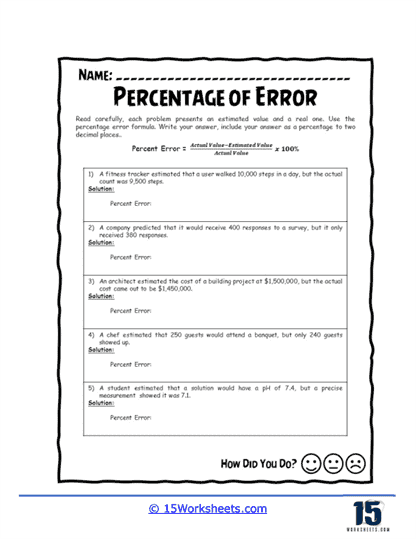
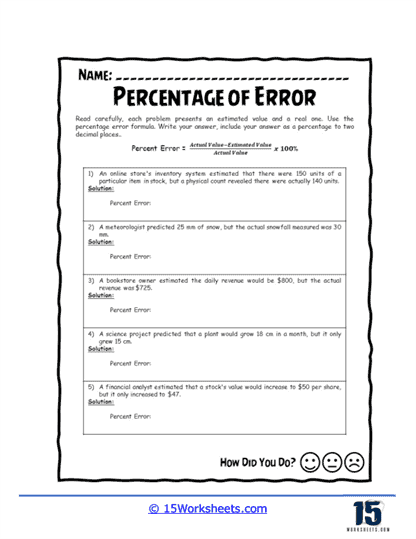
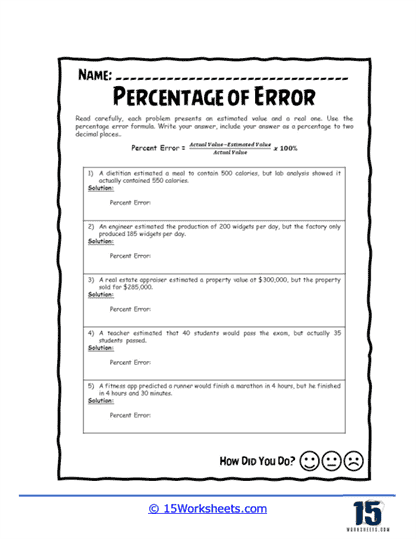
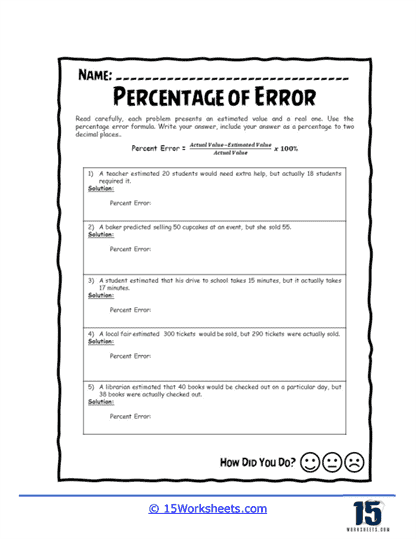
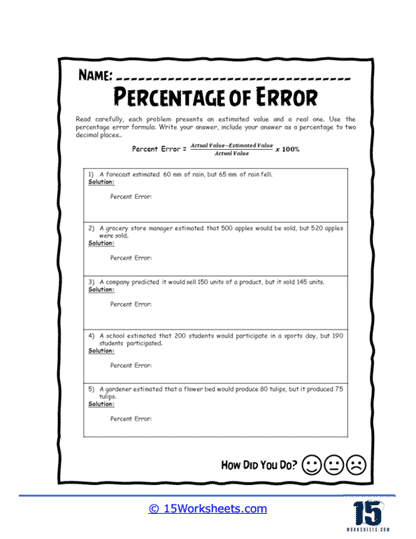
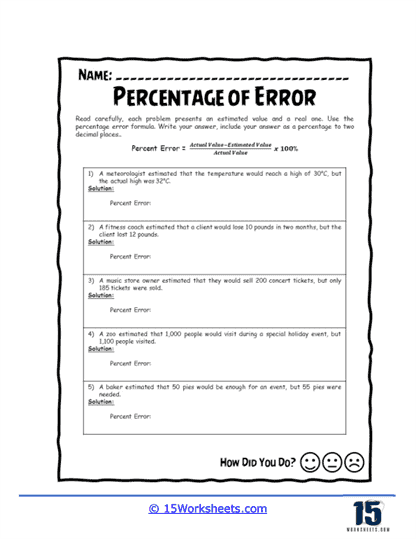
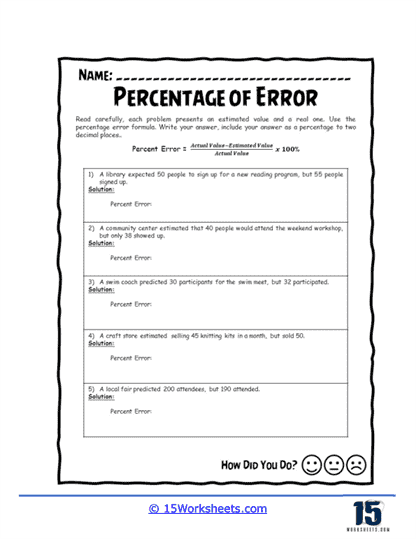
These worksheets are designed to help students understand the concept of error measurement in mathematics, particularly in situations where there is a difference between an estimated or measured value and the actual value. These worksheets focus on calculating the percentage error, which is the difference between the estimated and actual values expressed as a percentage of the actual value. The formula used is straightforward: subtract the estimated value from the actual value, divide the result by the actual value, multiply by 100, and express the result as a percentage. Students then round their answers to two decimal places, ensuring accuracy in their calculations. These worksheets are incredibly useful in teaching students how to analyze estimates versus reality and how to quantify the degree of error in measurements or predictions.
One common type of problem on these worksheets involves scenarios where estimates are made, but actual outcomes differ from the estimates. For example, students might be asked to calculate the percentage error in a weather forecast where the predicted rainfall was 20 millimeters, but the actual recorded rainfall was only 15 millimeters. Another example might involve a school estimating that 300 students would enroll for the new academic year, but only 280 actually enrolled. These scenarios help students see the relevance of percentage error in real-world situations, emphasizing the importance of precise calculations when making predictions or estimates.
Another type of problem found in these worksheets focuses on the difference between projected and actual outcomes in production or sales. A manufacturer, for instance, might estimate that their car model would achieve a fuel efficiency of 40 miles per gallon, but real-world tests show that the car only achieved 35 miles per gallon. Similarly, a baker might estimate that 200 loaves of bread would sell on a particular day, but only 175 loaves were sold. These examples give students a practical look at how errors in estimates can affect businesses, production goals, and financial outcomes, further underscoring the real-life applications of percentage error calculations.
In addition to predictions and outcomes, some worksheets incorporate problems involving scientific experiments, such as calculating the percentage error in energy output from a laboratory experiment. For instance, a physics experiment might estimate that 120 joules of energy would be produced, but the actual output was only 115 joules. This type of problem shows students the critical role that percentage error plays in scientific accuracy, teaching them how to compare experimental results with expected outcomes and how to account for discrepancies.
The variety of scenarios found in our percentage of error worksheets ensures that students are exposed to a wide range of contexts where percentage error is important. This includes fields like weather forecasting, manufacturing, baking, education, and scientific research. These contexts allow students to see how percentage error affects different industries and processes, and how calculating it can help improve accuracy and performance. The problems are typically written in everyday language, making it easy for students to understand and apply the concepts being taught.
Each problem on these worksheets is structured in a similar way. First, students are given an estimated or predicted value and an actual value. They are then required to apply the percentage error formula to calculate the difference between the two values. After calculating the error, students convert the result into a percentage, which provides a clear measure of how far off the estimate was from the actual outcome. By practicing this calculation repeatedly, students become more comfortable with the formula and the process of calculating percentage error, which can be applied to various subjects and areas of life.
The math skills taught in these worksheets extend beyond just the calculation of percentage error. Students also develop their abilities in subtraction, division, multiplication, and rounding. They learn how to approach word problems by extracting the necessary information and applying the appropriate formula to solve for the percentage error. These are essential skills in higher-level math, science, and everyday decision-making. In particular, learning to round answers to two decimal places helps students ensure precision, a critical skill in both academic and professional settings.
These worksheets also help students develop critical thinking skills. Each problem requires them to interpret a scenario, understand the relationship between the estimated and actual values, and calculate how far off the estimate was. By analyzing each situation and performing the necessary calculations, students improve their ability to assess accuracy, make informed decisions, and reflect on why certain estimates might differ from actual results. This type of reasoning is important not only in math and science but also in real-world applications where estimates and predictions are made regularly.
By focusing on the difference between estimates and actual outcomes, students also learn valuable lessons in precision, accuracy, and the importance of careful measurement. They come to understand that small differences can sometimes lead to significant percentage errors, and that improving estimates can lead to better outcomes in various fields. Whether it’s predicting the number of students who will enroll in a school, estimating how many loaves of bread will be sold, or calculating how much energy an experiment will produce, the skills learned in these worksheets are applicable to a wide range of disciplines and career paths.
Percentage of Error In The Real World
Percentage of Error is a powerful tool used across a wide range of industries to measure the accuracy of predictions and estimates compared to actual outcomes. It quantifies how much an estimate deviates from reality, providing valuable insight that allows professionals to refine their processes, improve accuracy, and make better-informed decisions. Below are expanded examples of how percentage error is applied in different sectors, each demonstrating the critical role it plays in day-to-day operations.
In scientific research and experiments, percentage error is a key metric for understanding how closely experimental results match theoretical predictions. Scientists in fields like chemistry, physics, and biology use this calculation to determine whether their experiments yielded the expected outcomes or if there are significant discrepancies. For example, if a chemist expects a certain reaction to produce 10 grams of a substance but only measures 9 grams after conducting the experiment, the percentage error quantifies the difference between the predicted and actual result. This allows scientists to identify potential sources of error, such as inaccuracies in measurements, external environmental factors, or procedural mistakes. By consistently measuring and analyzing percentage error, researchers can refine their experimental techniques, improve the reliability of their results, and contribute more accurate findings to the scientific community.
In manufacturing and production, companies rely heavily on forecasts and estimates to ensure their processes run efficiently. Factories often set targets for how many units of a product should be produced in a given time frame, based on demand forecasts or production capacity. However, real-world production may fall short of or exceed these estimates due to equipment malfunctions, supply chain issues, or labor shortages. For instance, if a factory estimates that it will produce 1,000 units of a product but only 950 are manufactured by the end of the day, calculating the percentage error allows the company to measure the shortfall. This helps managers identify inefficiencies in the production process and make adjustments to improve productivity or ensure more accurate forecasts in the future. Understanding percentage error in this context helps manufacturers optimize their operations, reduce waste, and better meet customer demand.
In weather forecasting, percentage error is used by meteorologists to evaluate the accuracy of their predictions. Forecasts play a crucial role in helping individuals, businesses, and governments prepare for weather events, but the inherent unpredictability of the atmosphere means that forecasts are not always perfect. For example, if a weather forecast predicts 20 millimeters of rainfall, but only 15 millimeters are recorded, the percentage error measures how far off the prediction was. By calculating this error, meteorologists can analyze the factors that led to the discrepancy—such as unexpected changes in wind patterns, humidity levels, or pressure systems. This helps weather experts refine their models and make more accurate predictions in the future, ultimately improving public safety and reducing the potential for weather-related disruptions in industries such as agriculture, transportation, and construction.
In the realm of business and finance, percentage error is used to measure the accuracy of financial forecasts. Businesses rely on revenue and sales projections to make decisions about budgeting, staffing, and investment. However, external factors such as market conditions, consumer behavior, or supply chain disruptions can lead to actual results that differ from projections. For instance, if a company forecasts $1 million in sales but only generates $900,000, the percentage error provides a clear indication of the deviation from the expected outcome. This allows financial managers to assess the reasons behind the discrepancy and adjust future forecasts to reflect more realistic expectations. Understanding the percentage error in financial forecasting is essential for businesses seeking to manage risk, allocate resources effectively, and maintain profitability.
In construction and engineering, percentage error is used to compare estimated project costs and timelines with actual expenses and completion dates. Construction projects are often subject to delays, cost overruns, or changes in scope, which can lead to significant deviations from the original estimates. For example, a construction company may estimate that a building project will cost $500,000, but the final cost might end up being $550,000. By calculating the percentage error, project managers can determine how far off their initial estimates were and analyze the factors that contributed to the increased costs, such as unexpected material price increases or labor shortages. This analysis helps engineers and project managers improve their cost estimation techniques for future projects, ensuring that budgets are more accurate and timelines are better adhered to. Minimizing percentage error in construction is critical for maintaining client trust and staying within budget.
In retail and inventory management, percentage error is often used to assess the accuracy of sales forecasts and inventory levels. Retailers make projections about how many units of a product they expect to sell based on factors such as past sales data, market trends, and seasonal demand. However, actual sales figures can sometimes fall short of or exceed these expectations due to consumer behavior or economic conditions. For example, if a store estimates it will sell 500 units of a product but only 450 units are sold, the percentage error helps managers understand how much the actual sales deviated from the forecast. This information allows retailers to make better decisions about ordering, inventory management, and promotions to avoid overstocking or understocking items. By consistently monitoring percentage error, retailers can fine-tune their forecasting processes and optimize inventory turnover.