Percent of Change Worksheets
About These 15 Worksheets
These worksheets are great for helping students understand how to calculate the percentage increase or decrease between two numerical values. These worksheets are designed to reinforce the concept of percentage change, which is a critical skill in both mathematics and real-world applications. By working through various types of problems, students gain a better understanding of how quantities evolve over time, how prices fluctuate, and how different values compare to one another in terms of their percentage change.
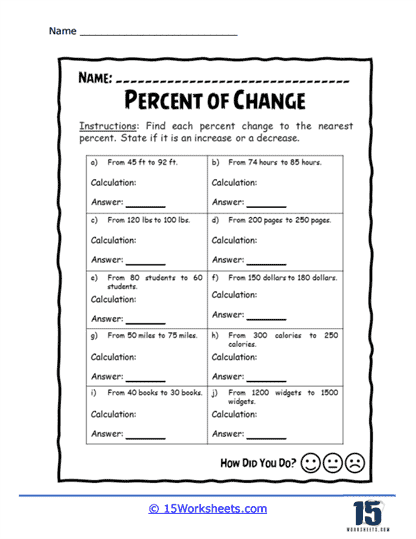
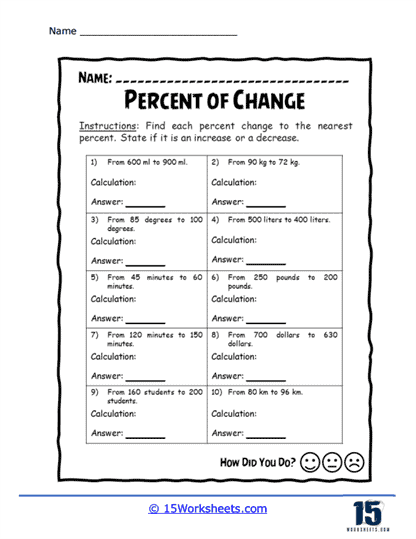
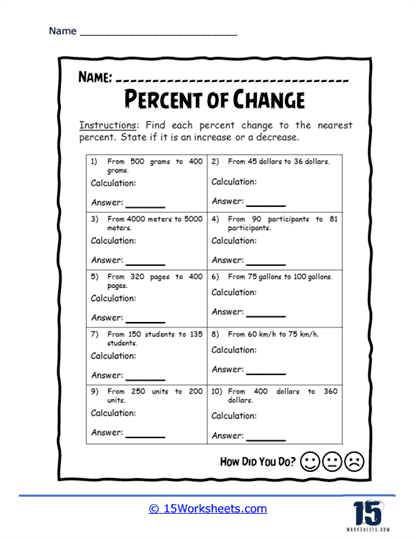
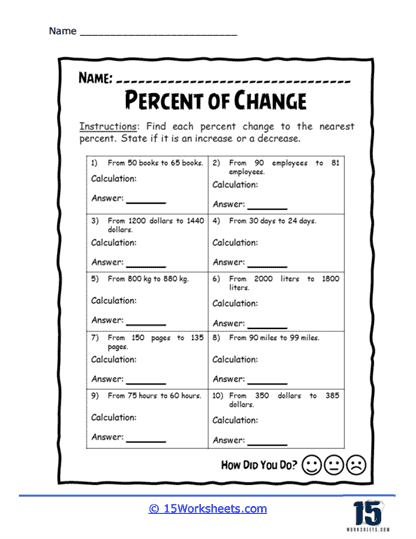
Each of these worksheet presents a series of problems where students are given two numbers: an initial value and a final value. The task is to calculate the percentage change from the initial value to the final value and determine whether this change is an increase or a decrease. For example, a problem might present a situation where a student’s test score increases from 75 to 90, and the student is asked to calculate the percentage increase. This type of problem reinforces the student’s ability to apply the percent change formula: Percent Change = (New Value – Old Value) / Old Value x 100. By consistently applying this formula, students develop a solid understanding of how percentage changes are calculated and what they represent.
One type of problem commonly found on these worksheets involves real-life scenarios where quantities increase, such as the number of attendees at an event or the distance run in a race. These problems help students connect the concept of percent change to everyday experiences, making the math more relatable and meaningful. For example, a problem might ask students to calculate the percentage increase when the number of people attending a concert rises from 500 to 600. By solving these problems, students learn how to apply mathematical concepts to situations they might encounter in their own lives, such as understanding how a price increase affects their budget or how a rise in temperature impacts their day.
Conversely, other problems on these worksheets involve scenarios where quantities decrease, such as a reduction in the number of employees at a company or a decrease in the amount of a product in a container. These problems are equally important because they teach students to recognize and calculate negative percentage changes. For instance, a problem might ask students to find the percentage decrease when the weight of a package drops from 150 to 120 pounds. By working through these problems, students gain a comprehensive understanding of how to handle both increases and decreases, which is essential for interpreting data in various fields, including business, economics, and science.
Some worksheets also include problems that require students to calculate the percentage change between more abstract values, such as time, distance, or weight. For example, students might be asked to calculate the percentage change when the duration of a task is reduced from 60 minutes to 45 minutes or when the distance traveled increases from 200 to 250 miles. These problems help students understand that the concept of percent change is not limited to just prices or quantities but can be applied to any measurable change over time or space. This broader application of percent change reinforces the versatility of the concept and prepares students for more advanced math and science courses.
This collection of worksheets will often present problems in a variety of formats to keep students engaged and to challenge their critical thinking skills. Some problems might be presented in a straightforward, numerical format, while others might be word problems that require students to interpret and analyze the information before calculating the percentage change. For example, a word problem might describe a situation where a store’s inventory decreases from 1,000 items to 850 items, and the student must determine the percentage decrease. These types of problems help students develop their ability to read and comprehend complex information, a skill that is essential for success in both academics and everyday life.
Another important feature of these worksheets is that they often require students to state whether the change they calculated is an increase or a decrease. This additional step ensures that students are not only performing the correct calculations but also understanding the context of the problem and interpreting the results accurately. For instance, after calculating the percentage change in a situation where the number of books in a library increases from 200 to 250, students would need to recognize that this is a percentage increase. This emphasis on context and interpretation helps students develop a deeper understanding of the material and prepares them for real-world situations where accurate data interpretation is crucial.
These worksheets will include problems that vary in difficulty, allowing students to build their skills gradually. A worksheet might start with simpler problems, such as calculating the percentage change from 50 to 60, and then progress to more complex scenarios, such as finding the percentage change when multiple variables are involved. This gradual increase in difficulty helps students build confidence in their abilities and ensures that they are mastering each concept before moving on to more challenging material. By the end of the worksheet, students should feel confident in their ability to calculate percentage changes in a variety of contexts and to apply this knowledge to real-life situations.
In addition to teaching students how to calculate percent change, these worksheets also help students develop a number of other important math skills. For instance, students practice their ability to work with fractions, decimals, and percentages, all of which are essential components of many mathematical concepts. They also learn to follow a systematic approach to problem-solving, which involves identifying the relevant information, performing the necessary calculations, and interpreting the results. This systematic approach is a valuable skill that students can apply to many different areas of math, science, and even everyday life.
These worksheets often encourage students to check their work and think critically about their answers. For example, after calculating a percentage change, students might be asked to consider whether their answer makes sense in the context of the problem. This type of critical thinking is essential for ensuring that students are not just performing calculations mechanically but are also understanding the material and applying it correctly. By developing this habit of checking their work and thinking critically, students become more independent learners and are better prepared for more advanced math courses and real-world problem-solving.