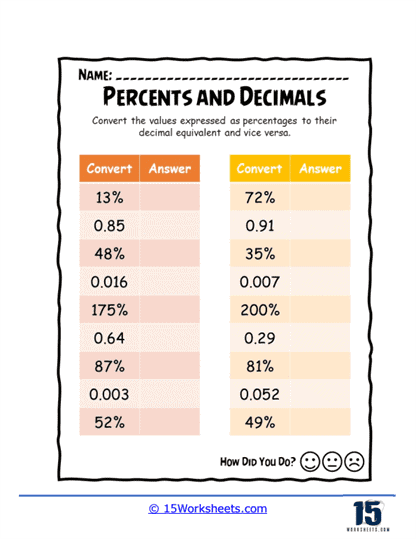
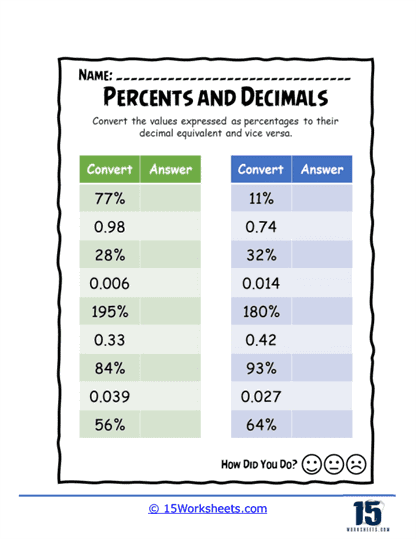
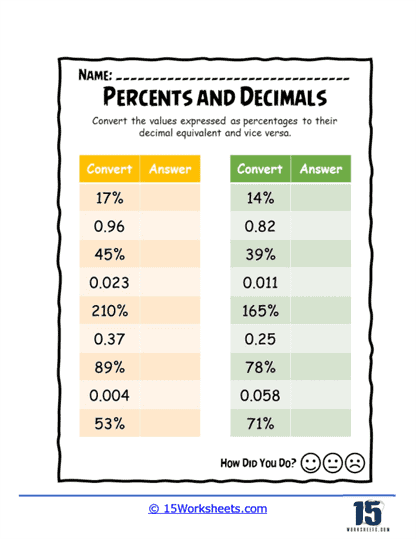
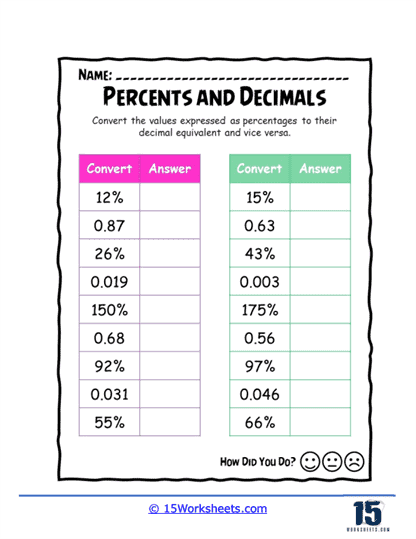
Percentages and Decimals Worksheets
About These 15 Worksheets
These worksheets will help bridge the understanding between fractions, percentages, and decimals. These worksheets help students develop crucial skills that are foundational not only in mathematics but also in real-world applications such as finance, science, and everyday decision-making.
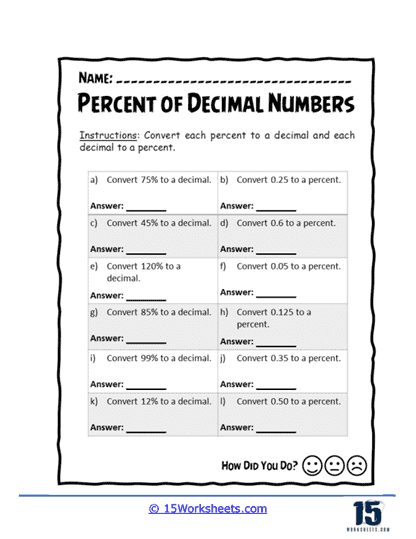
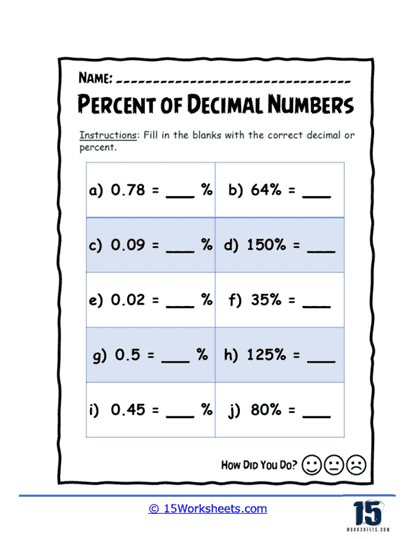
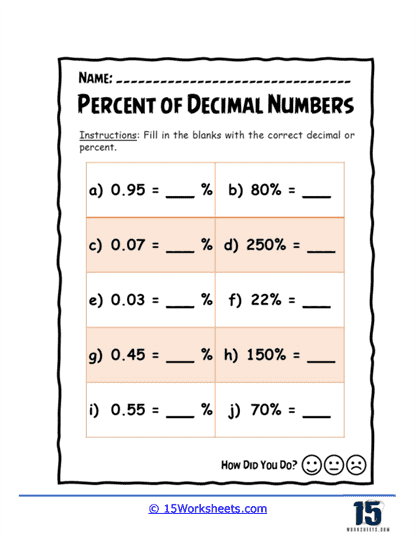
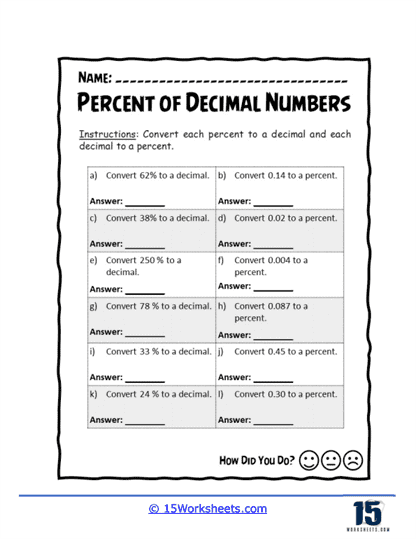
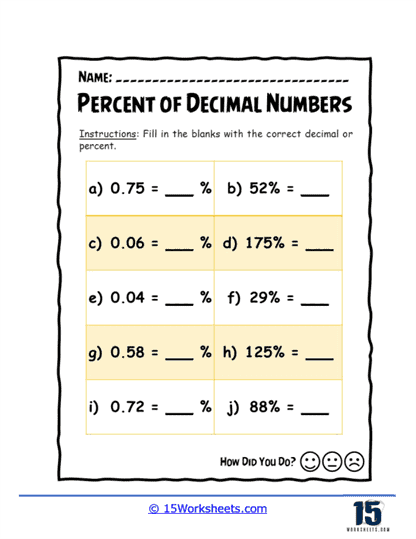
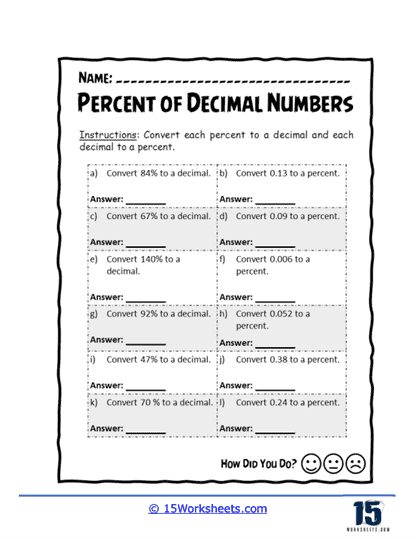
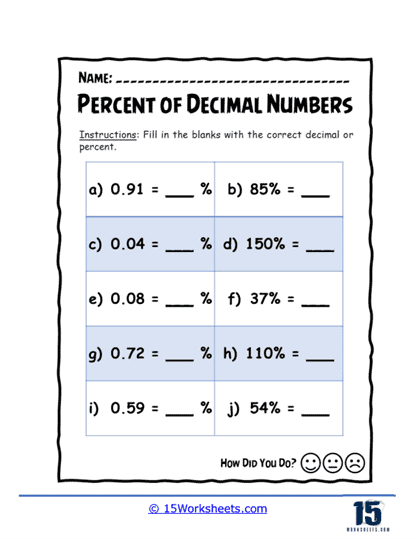
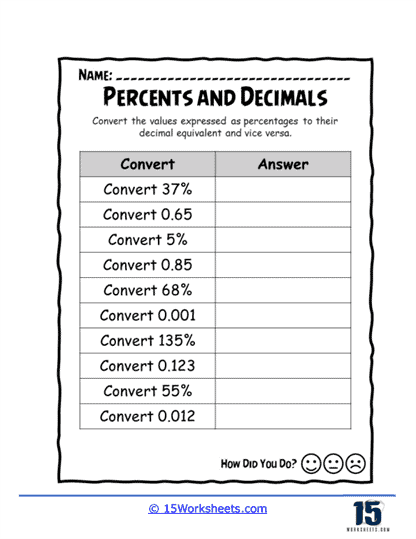
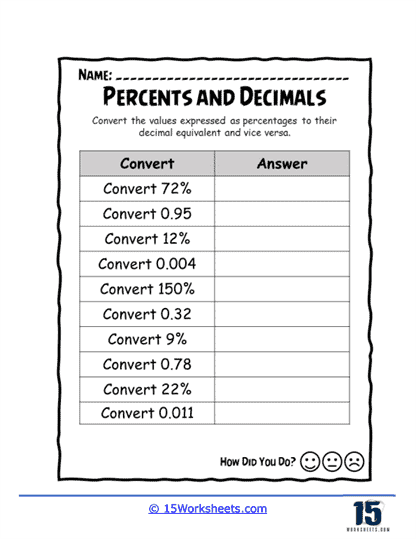
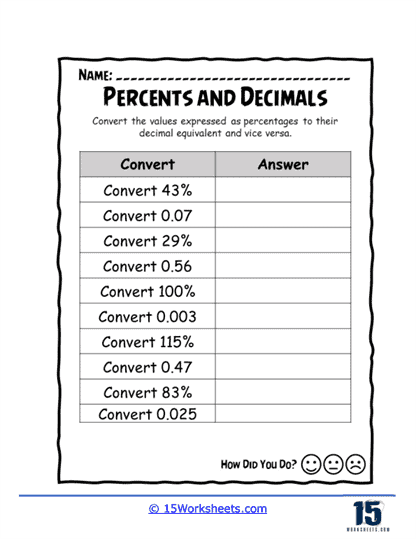
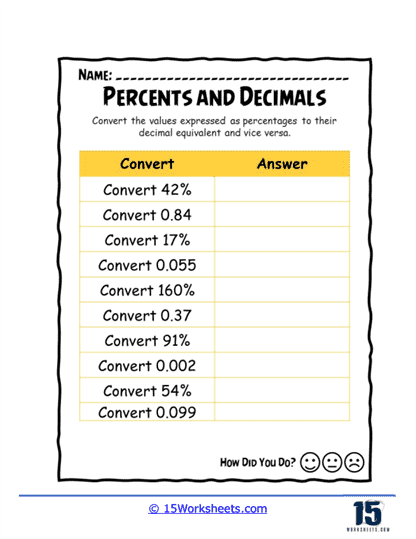
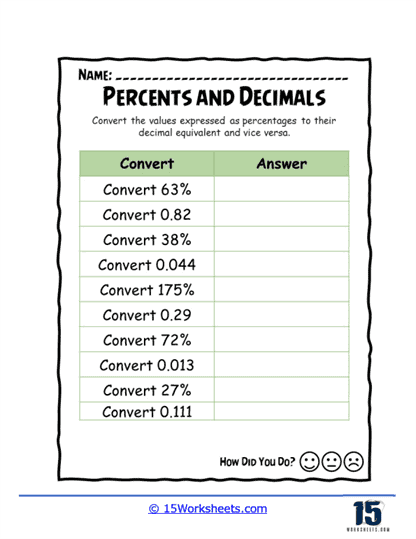
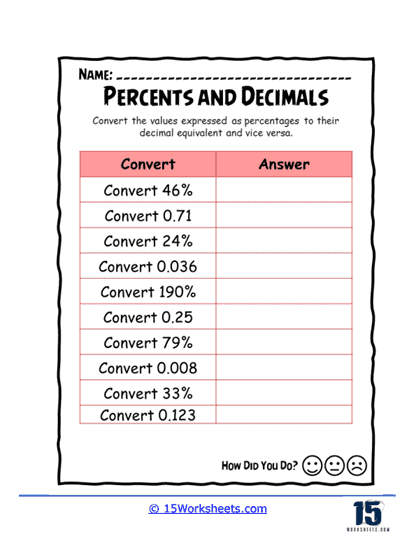
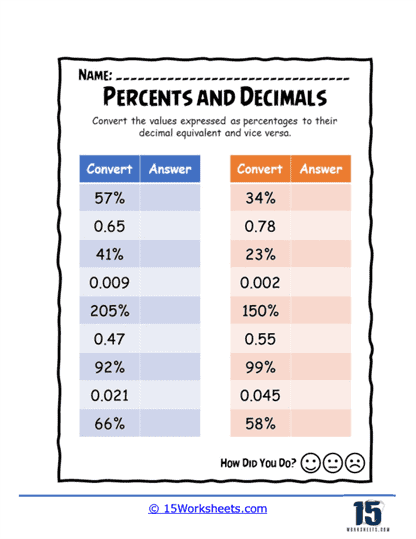
The worksheets are designed to guide students through the process of converting percentages to decimals and vice versa. This conversion skill is a cornerstone of understanding how different numerical representations relate to each other. For example, students may be asked to convert a percentage like 75% into a decimal, which would involve recognizing that 75% is equivalent to 0.75. Conversely, they might be required to convert a decimal like 0.25 back into a percentage, which would result in 25%. This practice reinforces the understanding that percentages are another way of expressing fractions out of 100, and decimals are just another form of these fractions.
Beyond simple conversion tasks, the worksheets include more complex problems that require students to apply these conversion skills in various contexts. For instance, students might encounter problems where they have to find a percentage of a given number or determine what percentage one number is of another. These types of problems help students understand how percentages and decimals function in different scenarios, such as calculating discounts during shopping, understanding interest rates, or analyzing data in graphs and charts.
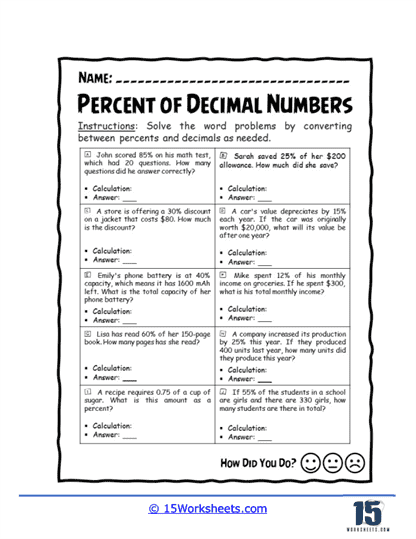
Some worksheets present word problems that incorporate percentages and decimals. These problems are particularly valuable because they teach students how to translate real-world situations into mathematical equations. For example, a problem might describe a situation where a store is offering a discount, and the student must calculate the final price after applying the percentage discount. Another problem might involve understanding what a certain percentage of a number represents in a context, such as how many questions a student answered correctly on a test or how much of a budget is allocated to a specific category. These word problems enhance critical thinking and problem-solving skills by requiring students to discern relevant information, set up appropriate equations, and perform the necessary calculations.
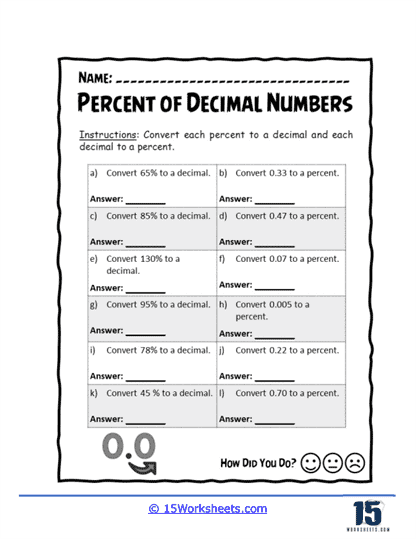
Our advanced worksheets will challenge students to tackle problems involving percentages greater than 100% or less than 1%, which are concepts that extend the basic understanding of percentages. For instance, converting 250% to a decimal (which results in 2.5) teaches students that percentages can represent values greater than the whole, while converting 0.5% to a decimal (which results in 0.005) illustrates how percentages can represent very small parts of a whole. These exercises push students to think beyond simple cases and understand the flexibility of percentage and decimal notation in representing different types of quantities.
The skills developed through these worksheets are cumulative. Early exercises might focus solely on conversion, helping students get comfortable with the mechanics of switching between decimals and percentages. As students gain confidence, the worksheets progress to more applied problems, including both numeric and word problems. This progression ensures that students build a deep understanding of the relationship between decimals and percentages, how they can be manipulated, and how they apply to real-life situations.
Converting a Percentage to a Decimal
Converting between percentages and decimals is a simple process that involves either dividing or multiplying by 100. When converting from a percentage to a decimal, you divide by 100.
To convert a percentage to a decimal, follow these steps:
Step #1 – Remove the percent sign (%): The first step is to remove the “%” symbol from the percentage value.
Step #2 – Divide by 100: After removing the percent sign, divide the number by 100. This step is equivalent to moving the decimal point two places to the left.
Example: Convert 75% to a Decimal
Step #1 – Remove the percent sign from 75%, so it becomes 75.
Step #2 – Divide 75 by 100.
75 ÷ 100 = 0.75
So, 75% is equivalent to 0.75 as a decimal.
Converting a Decimal to a Percentage
When converting from a decimal to a percentage, you multiply by 100. This operation is essentially about shifting the decimal point two places to the left (for percentage to decimal) or two places to the right (for decimal to percentage).
To convert a decimal to a percentage, follow these steps:
Step #1 – Multiply by 100: Start by multiplying the decimal by 100. This step is equivalent to moving the decimal point two places to the right.
Step #2 – Add the percent sign (%): After multiplying, add the “%” symbol to the resulting number.
Example 1: Convert 0.25 to a Percentage
Step #1 – Multiply 0.25 by 100.
0.25 x 100 = 25
Step #2 – Add the percent sign to the result.
So, 0.25 is equivalent to 25%.