Area of Trapezoids Worksheets

About Our Area of Trapezoids Worksheets

Understanding geometry is a crucial aspect of a student’s mathematical journey, and one of the essential topics in this domain is the area of trapezoids. This comprehensive worksheet collection is designed to provide structured, engaging, and practical exercises that reinforce the fundamental concepts related to trapezoidal area calculations. These worksheets help students develop confidence in solving geometry problems while enhancing their critical thinking and problem-solving skills.

Ideal for classroom instruction, homework assignments, homeschooling, or independent practice, this collection offers a variety of question formats, including step-by-step calculations, real-world application problems, and visually engaging activities. Whether you’re an educator looking for supplemental materials or a parent seeking extra practice for your child, this Area of Trapezoids Worksheet Collection is an invaluable resource.

What’s Inside?

This curated worksheet set provides a structured and scaffolded approach to mastering the concept of trapezoidal area calculations. The worksheets cater to students at various learning levels, ensuring that they grasp the topic progressively.

1. Foundational Understanding

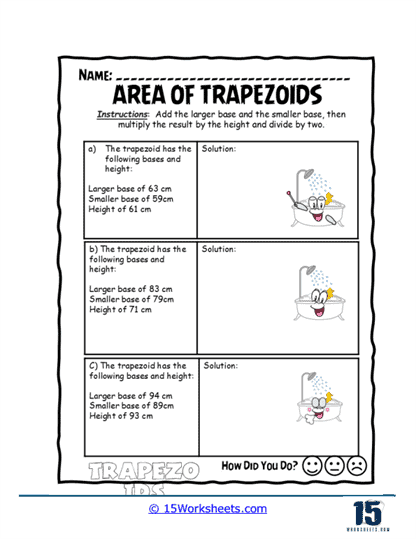
Introduction to the Formula – Students learn the standard formula for calculating the area of a trapezoid:

Area of a trapezoid = ½ (b₁ + b₂) x h

where b₁ and b₂ are the bases, and h is the height.

• Step-by-Step Guided Problems – Worksheets begin with simple, straightforward problems where students apply the formula with clearly labeled values.
• Visual Aids & Diagrams – Engaging graphics help students understand how different trapezoid dimensions affect area calculations.

2. Skill-Building Exercises

• Fill-in-the-Blank Problems – Students complete missing values within area formulas to reinforce their understanding.
• Multiple-Choice Questions – These questions assess conceptual knowledge and problem-solving strategies.
• Matching Activities – Students match trapezoid dimensions with their correct areas, promoting active engagement.
• Error Analysis Tasks – Students analyze incorrect solutions to identify common mistakes and misconceptions.

3. Real-World Applications

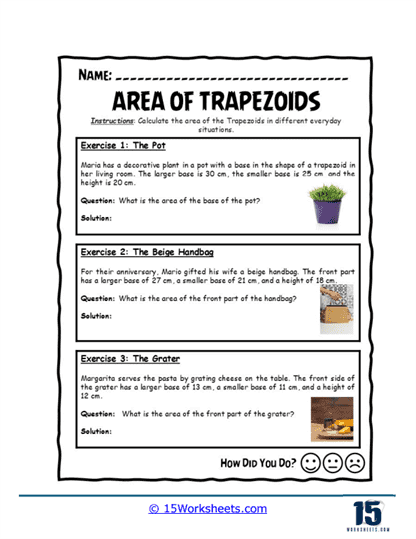
• Word Problems with Context – Students solve problems based on real-life scenarios, such as calculating the area of trapezoidal garden plots, architectural designs, and construction blueprints.
• Challenge Problems – Advanced questions require students to apply the area formula to composite shapes and solve for unknown variables.
• Cross-Curricular Connections – Some exercises integrate elements of physics, engineering, and geography, showing how trapezoids appear in different fields.

4. Engaging & Interactive Elements

• Stepwise Progression – Exercises are organized from basic to advanced, ensuring gradual mastery of the concept.
• Differentiated Learning – Varied question formats accommodate different learning styles and levels of mathematical proficiency.
• Answer Keys & Explanations – Detailed solutions help students self-assess their work and understand step-by-step processes.

Skills Developed

Mastering the area of trapezoids is not just about memorizing a formula; it involves a range of cognitive and mathematical skills that prepare students for higher-level mathematics and real-world problem-solving. The structured approach in these worksheets helps learners develop mathematical proficiency, critical thinking, and analytical reasoning, all of which are essential for academic success and everyday applications.

Building a Strong Foundation in Geometry

One of the key skills students develop is formula application. Geometry relies heavily on the correct application of formulas, and these worksheets ensure that students understand, practice, and confidently apply the trapezoidal area formula:

Area of a trapezoid = ½ (b₁ + b₂) x h

Through a variety of guided exercises, fill-in-the-blank questions, and problem-solving scenarios, students move beyond simple memorization. Instead, they develop a deeper conceptual understanding, learning how to manipulate the formula based on different given values.

Dimensional analysis plays a significant role in helping students comprehend the impact of changes in base length and height on the total area. By adjusting trapezoidal dimensions in their calculations, students build an intuitive sense of spatial relationships, which is a critical skill in fields like architecture, engineering, and physics.

These worksheets reinforce geometric reasoning by challenging students to recognize trapezoids within more complex figures. This skill is particularly useful when working with composite shapes, where students need to break down irregular figures into known components to determine total areas. By integrating these exercises, the worksheets foster the ability to think spatially and approach problems strategically.

Analytical Skills

Beyond calculations, these worksheets emphasize critical thinking and logical problem-solving. Many of the exercises go beyond straightforward formula application, requiring students to analyze word problems, identify missing values, and troubleshoot incorrect solutions.

For instance, analytical skills are developed through activities that require students to compare different trapezoids, determine patterns, and make predictions about how changes in base lengths or height affect the overall area. These exercises strengthen their ability to evaluate numerical relationships and use logical deduction-skills that are essential in advanced mathematics and data analysis.

Logical thinking is cultivated by structuring the worksheets in a progressive manner, where students begin with simpler problems before tackling more complex, multi-step questions. They must interpret problem statements, recognize key values, and logically sequence their calculations-all of which mimic real-world problem-solving scenarios.

Another key aspect is pattern recognition. Through repeated exposure to different types of trapezoids and real-world applications, students start to identify similarities and differences in problem structures. This ability to recognize mathematical patterns is not only crucial for solving geometry problems but also for algebra, data analysis, and scientific research, where recognizing trends and relationships is a fundamental skill.

Connecting Geometry to Everyday Life

A strong understanding of trapezoidal area calculations has practical implications beyond the classroom. These worksheets include real-world application problems that encourage students to see the relevance of geometry in their daily lives and future careers.

One of the most significant real-world connections is STEM readiness. Geometry is a foundational subject for careers in engineering, architecture, and design, where professionals must calculate areas of irregular shapes for construction projects, urban planning, and mechanical design. By mastering trapezoidal area calculations, students build a practical skill set that prepares them for higher education and professional fields requiring mathematical precision.

In addition to STEM applications, understanding the area of trapezoids is highly relevant for everyday problem-solving. Homeowners, landscapers, and DIY enthusiasts frequently encounter trapezoidal shapes when designing gardens, patios, or sloped driveways. Learning how to compute these areas ensures that students can estimate materials, optimize space, and make informed decisions in home improvement projects.

Data interpretation and measurement skills are enhanced through these exercises. In fields such as surveying, construction, and physics, professionals must measure and calculate areas accurately to ensure precision in mapping, land division, and structural integrity. By working through these worksheets, students gain exposure to real-world scenarios that prepare them for future academic and professional pursuits.

This collection is more than just a set of exercises-it is a comprehensive learning tool that fosters mathematical confidence, critical thinking, and real-world problem-solving abilities. By providing a structured and engaging approach, these worksheets enable students to progressively master the concept of trapezoidal area calculations, from understanding the fundamental formula to tackling complex application problems. Through interactive exercises, real-world scenarios, and step-by-step guidance, students gain a deeper appreciation for geometry and develop the skills necessary for academic success and practical applications. Whether in the classroom or at home, these worksheets provide an accessible and effective resource for reinforcing essential math concepts in a meaningful way.

Beyond academic benefits, these worksheets bridge the gap between theory and practice, demonstrating how mathematical principles apply to everyday life and future careers. From architects and engineers designing structures to homeowners planning renovations, the ability to calculate the area of trapezoidal spaces is a practical skill with broad relevance. By integrating critical thinking, pattern recognition, and logical reasoning, students not only strengthen their mathematical foundation but also prepare for STEM-related fields and lifelong problem-solving challenges. With this collection, educators, parents, and learners alike can feel confident in their ability to build lasting mathematical proficiency and real-world application skills.