Area of Parallelograms Worksheets
About Our Area of Parallelograms Worksheets
This collection of worksheets is designed to provide students with a structured, engaging, and hands-on approach to understanding and mastering the concept of calculating the area of a parallelogram. These worksheets offer a variety of exercises that guide students through the fundamental formula, real-world applications, and different problem-solving methods to strengthen their conceptual grasp of this essential geometry topic.
Perfect for teachers, homeschooling parents, and students, these printable worksheets cater to different learning styles by incorporating multiple question formats, visual problem-solving tasks, and interactive exercises to enhance comprehension and retention.
Key Features
Each worksheet is designed to reinforce concepts progressively while keeping students engaged with diverse question formats, interactive exercises, and real-world applications.
Step-by-Step Concept Reinforcement
Understanding the area of a parallelogram requires a strong grasp of the fundamental formula:
Area = Base x Height
To build this foundation, the worksheets begin with introductory exercises that teach students how to identify the base and height in different parallelograms. These exercises emphasize the importance of using the correct height measurement, especially when dealing with slanted or non-standard parallelogram orientations.
As students progress, they will:
- Learn to differentiate between the base and the height in varied layouts.
- Solve guided problems that break down the formula into step-by-step calculations.
- Work through error analysis exercises that help them spot and correct common mistakes.
- Apply their knowledge to real-world examples, making their learning experience more meaningful.
By following a structured learning path, students develop confidence in using the formula accurately and efficiently.
Diverse Question Formats for Better Understanding
Learning geometry isn’t just about memorizing formulas-it’s about understanding concepts from different angles. This worksheet collection includes a variety of question formats that cater to different learning styles, ensuring every student has a chance to excel.
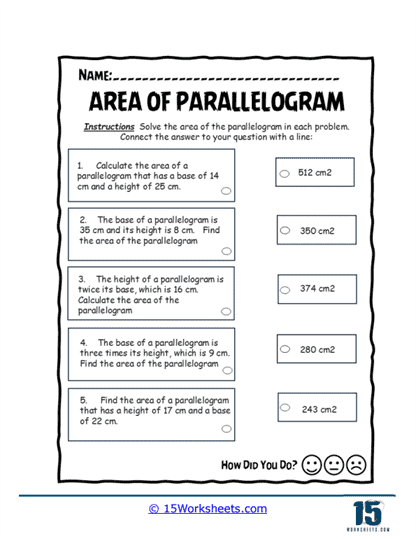
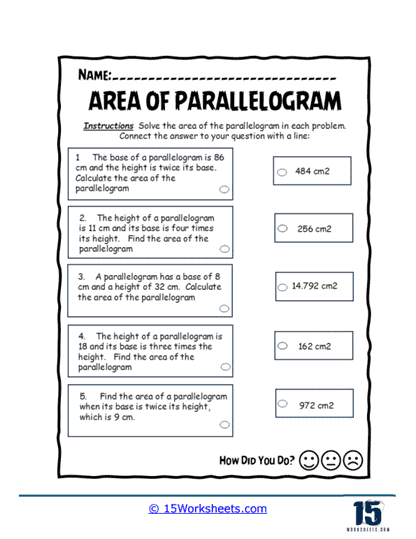
Multiple-Choice Questions – Ideal for quick recall and assessment, these questions help students solidify their understanding of the area formula and its applications. Questions vary from basic calculations to choosing the correct base or height in a given diagram.
Fill-in-the-Blank Exercises – These encourage students to actively think through the steps needed to compute the area, rather than just choosing from given options. Some exercises focus on unit conversions, reinforcing the importance of proper measurements in area calculations.
Matching Exercises – Students match parallelograms with their corresponding area values, reinforcing quick mental calculations and pattern recognition. These exercises also include matching questions to their correct problem-solving steps, reinforcing logical progression.
Word Problems & Real-Life Applications – Students apply the area formula in practical, real-world situations, such as:
- Calculating the area of a tabletop or floor space.
- Finding the area of a billboard or wall for painting estimates.
- Determining how much fabric or material is needed for a project.
These exercises bridge the gap between abstract math and everyday life, making the topic more relatable and useful.
Diagram-Based Questions – Students work with labeled parallelograms, identifying the base and height before calculating the area. Some problems include incorrect height placements, prompting students to analyze and choose the correct measurements. Rotated parallelograms challenge students to visualize height correctly, reinforcing spatial reasoning skills.
This variety in question formats engages students in different ways, ensuring deep comprehension and retention of the topic.
Practical Applications in Everyday Life
Understanding the area of a parallelogram isn’t just an academic exercise-it’s a practical skill with numerous real-world applications. These worksheets include scenarios and challenges that mimic real-life situations, helping students see the relevance of their learning.
Where do we use parallelogram area calculations in daily life?
Home Improvement & DIY Projects – Measuring the surface area of tables, countertops, and floors for renovation or decoration. Estimating paint or wallpaper coverage for walls with slanted ceilings.
Architecture & Engineering – Calculating land area for plot mapping and construction projects. Designing roof layouts, window placements, and tiling work.
Graphic Design & Art Projects – Determining canvas and poster sizes based on given area constraints. Understanding perspective and dimensions in design layouts.
Sports & Athletics – Computing field and track dimensions for layouts and renovations. Measuring parallelogram-shaped areas in courts or sports facilities.
Incorporating a structured, engaging, and practical approach, this area of parallelogram worksheet collection equips students with the essential skills needed to confidently tackle geometry problems. Through step-by-step concept reinforcement, diverse question formats, and real-world applications, these worksheets ensure that students not only master the formula but also develop critical thinking and problem-solving abilities. By presenting math in a visually engaging and relatable way, this collection makes learning interactive and enjoyable, fostering a deeper understanding of how geometry is applied in everyday life.
Whether used in classrooms, homeschooling environments, or independent study, these worksheets provide a valuable resource for reinforcing mathematical concepts in a way that is accessible and effective. By bridging the gap between abstract formulas and real-world problem-solving, this collection helps students gain a lifelong appreciation for the relevance of geometry. With each completed exercise, learners build the confidence and competence needed to apply their knowledge beyond the classroom, making math a skill they can use in practical, meaningful ways.