Properties of Multiplication Worksheets
About These 15 Worksheets
These worksheets focus on the foundational principles that govern multiplication, helping students recognize patterns and relationships that make working with numbers more intuitive and efficient. By breaking down the process into understandable segments, these worksheets are designed to foster deeper mathematical thinking and build the skills necessary for more complex problem-solving in the future.
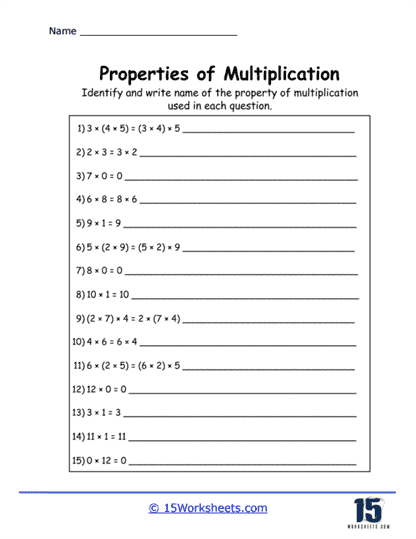
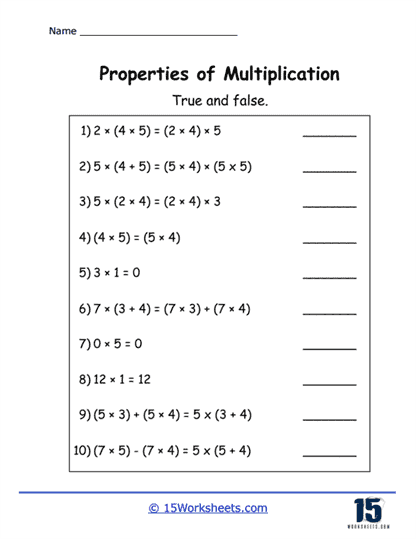
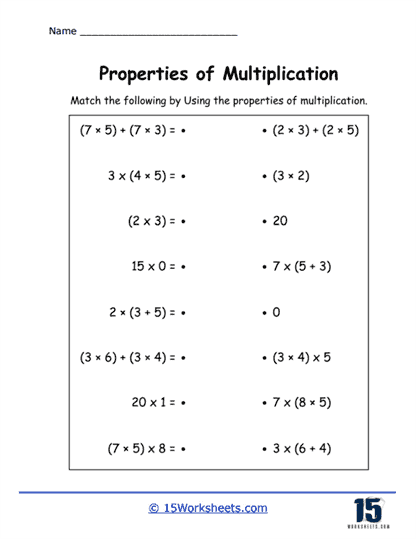
At the core of these worksheets, you’ll find a variety of exercises that cover the four fundamental properties of multiplication – the commutative, associative, distributive, and identity properties. Each property is essential for students to grasp, as they lay the groundwork for advanced mathematical concepts. The goal of these worksheets is not just to drill students on these properties but to help them see how these principles apply in different scenarios, ultimately leading to a stronger grasp of multiplication as a whole.
1. Commutative Property of Multiplication
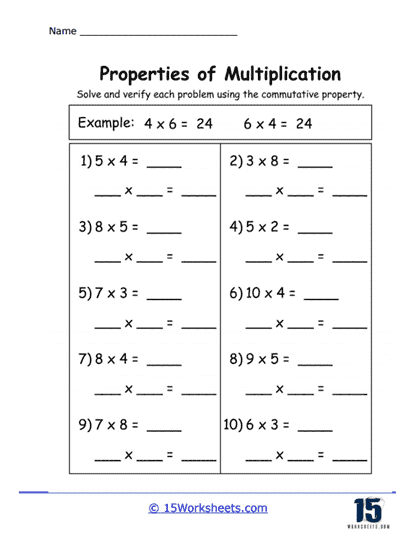
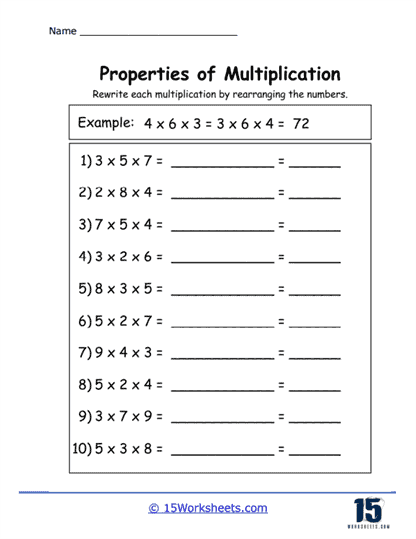
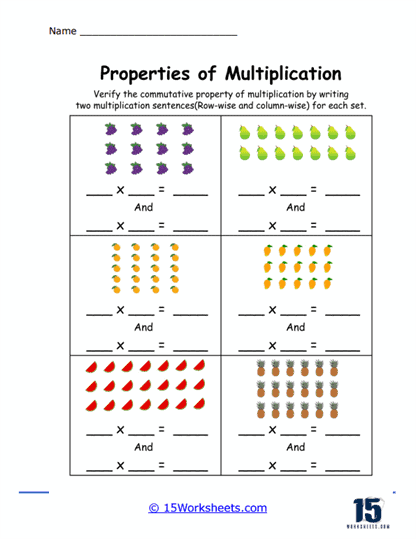
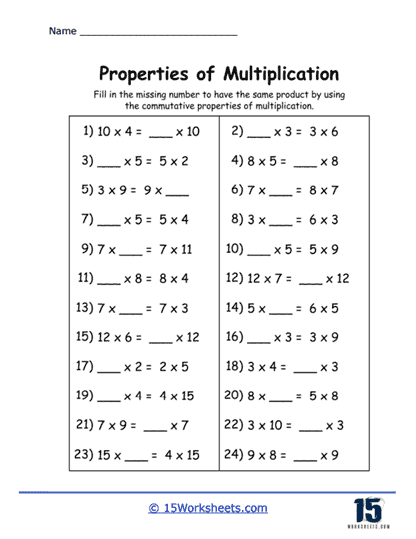
The commutative property of multiplication is typically the first property introduced to students, as it is straightforward and easy to grasp. This property states that the order in which two numbers are multiplied does not affect the product. In other words, a x b = b x a. Worksheets focusing on the commutative property often feature problems where students are asked to identify pairs of multiplication sentences that demonstrate this property. For example, a worksheet might present problems such as 4 x 3 = 12 and ask students to find the matching equation 3 x 4 = 12.
These exercises help students recognize that multiplication is flexible and that the order of factors can be swapped without changing the result. This understanding is critical as it allows students to approach multiplication problems with more confidence, knowing that they have multiple strategies to arrive at the correct answer. Moreover, recognizing the commutative property can also simplify more complex calculations, as students can rearrange factors in a way that makes the math easier to perform.
This property helps students understand that the order in which they multiply numbers doesn’t matter. It’s like rearranging a group of objects; the total number of objects stays the same no matter how you arrange them.
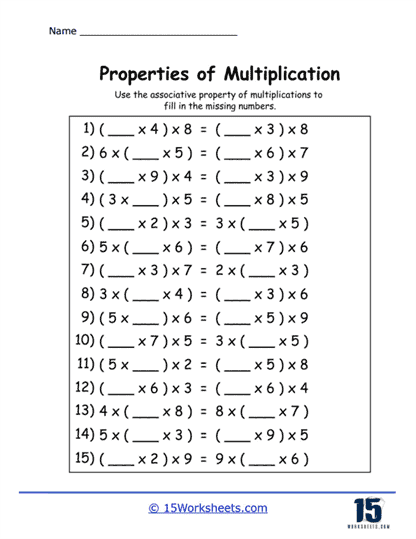
2. Associative Property of Multiplication
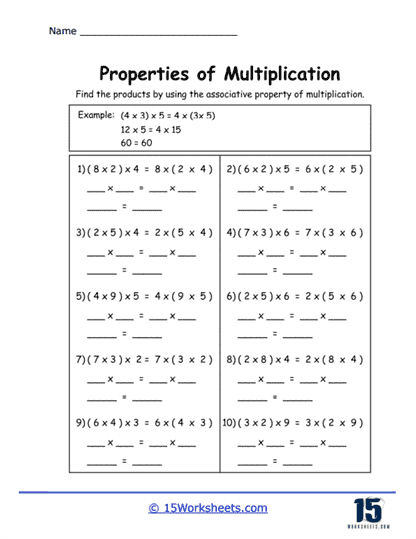
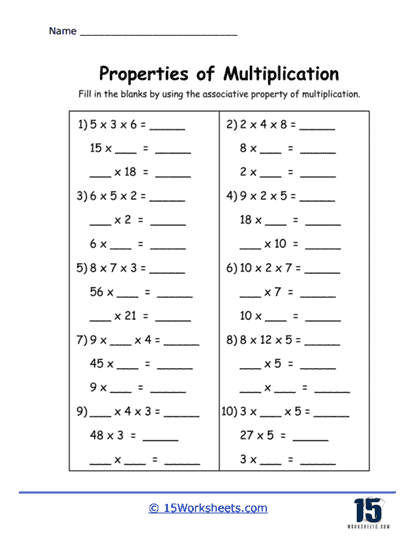
The associative property of multiplication states that the way in which numbers are grouped in a multiplication problem does not change the product. In mathematical terms, this means (a x b) x c = a x (b x c). Worksheets that focus on the associative property typically include problems where students must group numbers in different ways to verify that the product remains the same.
For instance, a worksheet might present a problem like (2 x 3) x 4 and ask students to regroup it as 2 x (3 x 4) to see that both yield the same product, which is 24. These exercises help students understand that grouping is a tool they can use to simplify calculations, especially when dealing with larger numbers or more factors. The associative property is particularly useful when students start working with mental math, as it allows them to break down complex problems into more manageable steps.
This property helps students see that they have flexibility in how they approach multiplication. It reassures them that no matter how they group the numbers when multiplying, the final product will always be the same.
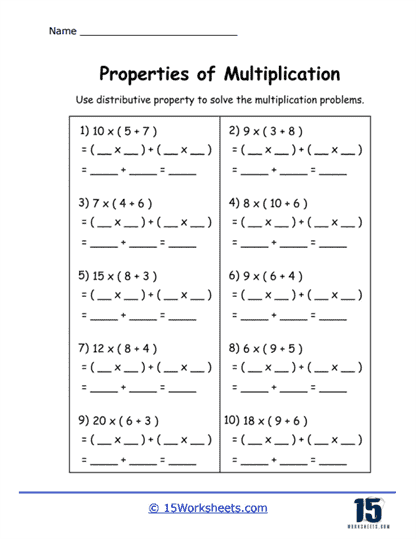
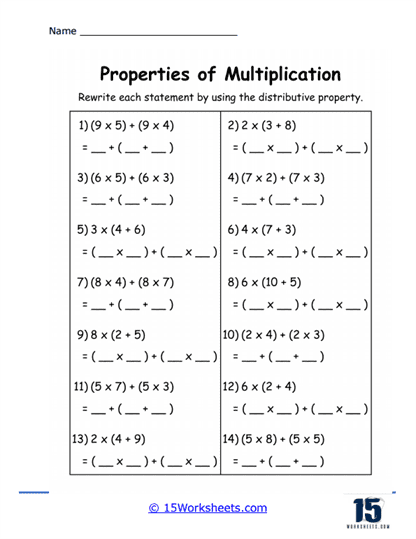
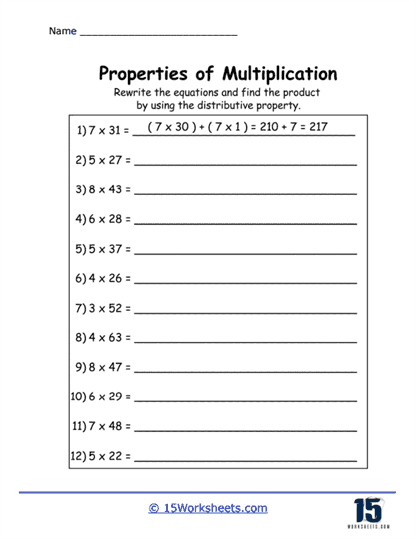
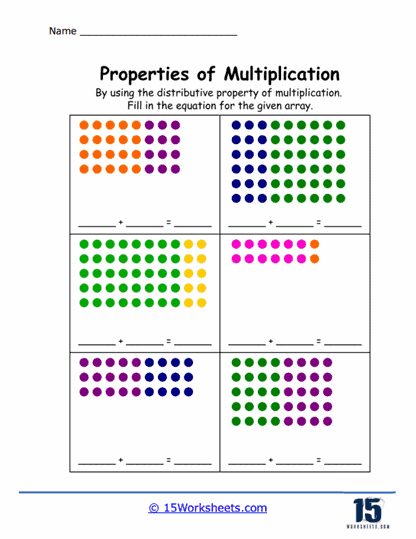
3. Distributive Property of Multiplication
The distributive property of multiplication is perhaps the most versatile and widely used property in mathematics. It states that multiplying a sum by a number is the same as multiplying each addend by the number and then adding the products. Formally, this can be expressed as a x (b + c) = (a x b) + (a x c).
Worksheets that cover the distributive property often include problems that require students to apply this principle to simplify multiplication involving addition. For example, a worksheet might ask students to expand and solve an expression like 3 x (4 + 5). Using the distributive property, students would calculate 3 x 4 + 3 x 5 to arrive at the correct answer, which is 27.
These types of problems teach students how to break down more challenging multiplication problems into easier steps, a skill that becomes increasingly important as they progress in their math education. The distributive property is also essential in algebra, where it is used to simplify expressions and solve equations. By practicing this property early on, students build a strong foundation for these more advanced topics.
The distributive property is very helpful when students need to break down more complicated multiplication problems into smaller, easier steps. It’s like splitting a big job into smaller tasks and then combining the results.
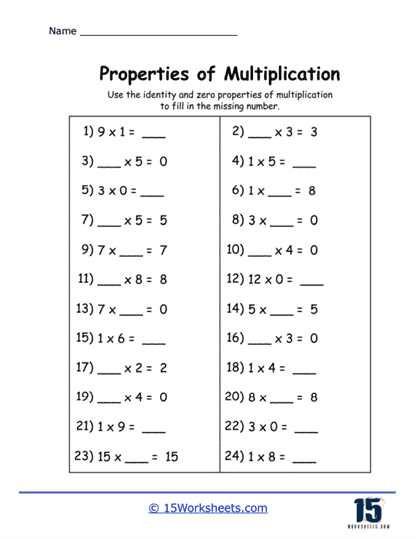
4. Identity Property of Multiplication
The identity property of multiplication states that any number multiplied by one remains unchanged, or a x 1 = a. This property is usually one of the simplest for students to understand, but it is still crucial for reinforcing the concept that multiplication has consistent rules.
Worksheets focusing on the identity property often include problems where students need to identify or complete equations that demonstrate this principle. For example, a problem might present an equation like 7 x 1 = and ask the student to fill in the blank. While this might seem elementary, it reinforces the idea that multiplication by one does not alter the value of a number, which is a key concept in maintaining accuracy in more complex calculations.
This property is simple but important because it teaches students that multiplying by 1 is like doing nothing to the number-it stays the same. This helps them understand the concept of multiplication more deeply. Additionally, the identity property is foundational for understanding other properties and operations, particularly when students begin to work with fractions and algebraic expressions. Recognizing that multiplying by one doesn’t change the value helps in simplifying expressions and solving equations where maintaining the integrity of the original number is critical.
5. The Zero Property of Multiplication
The zero property of multiplication tells us that any number multiplied by 0 is always 0. No matter how big or small the number is, multiplying it by 0 will give you nothing. This property is crucial because it shows that 0 is a special number in multiplication. It teaches students that when something is multiplied by zero, everything disappears, resulting in zero.
Example – Suppose you have 7 candies, and you multiply them by 0 – 7xx 0 = 0. This means if you have 7 groups but each group has 0 candies, you end up with no candies at all.
Understanding these key properties of multiplication-commutative, associative, distributive, identity, and zero-provides students with the foundational skills they need to work with numbers more effectively. These properties not only simplify calculations but also build confidence in students as they see the patterns and relationships that exist in mathematics. By grasping these concepts early on, students will be better prepared for more advanced mathematical thinking in the future.