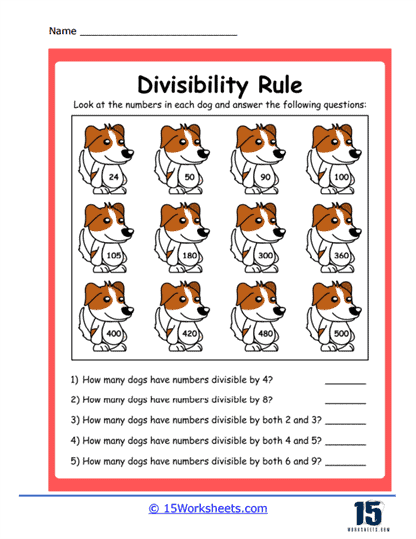
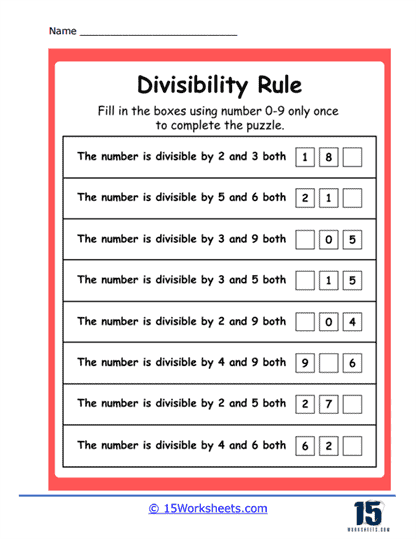
Divisibility Rules Worksheets
About These 15 Worksheets
Have you ever looked at a big number and wondered if it could be divided evenly by another number? For example, can the number 642 be divided by 2 without leaving any remainder? That’s where divisibility rules come in handy! They’re like quick shortcuts or magic tricks that help us figure out if one number can be divided by another without actually doing the division.
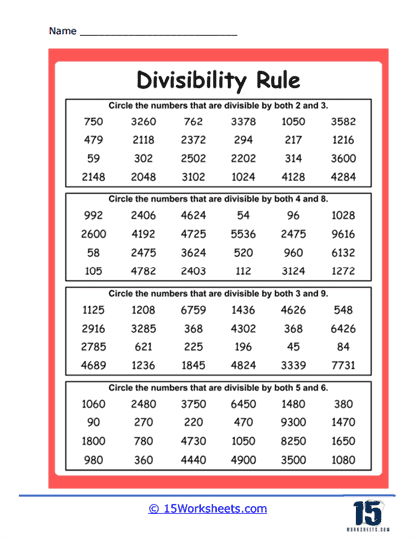
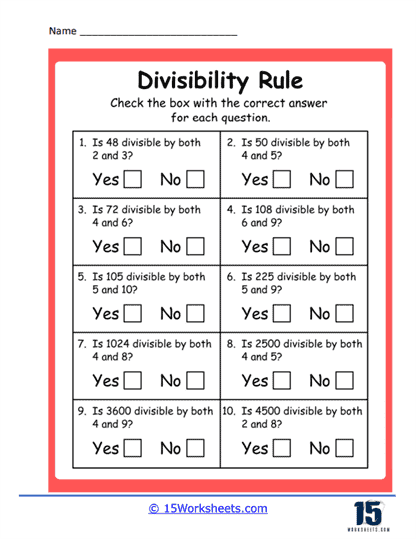
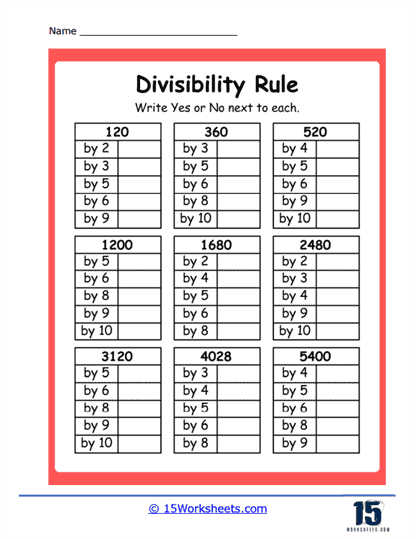
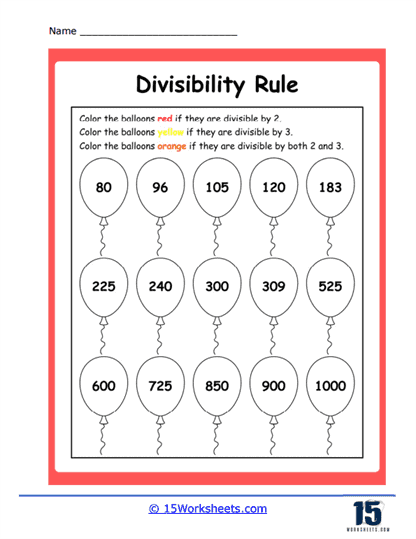
These worksheets often provide a variety of numbers to test students’ ability to apply the rules accurately. By practicing with these worksheets, students can become more proficient in recognizing and using these rules, which can help them in various math-related activities.
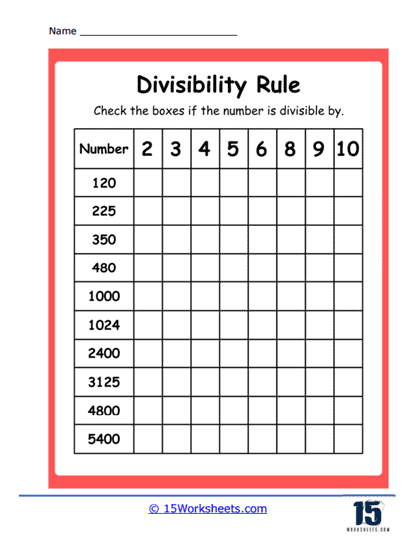
These worksheets include a series of numbers and ask students to determine if each number is divisible by a given divisor or not. The worksheets provide opportunities for students to apply the divisibility rules they’ve learned and identify patterns in numbers. This helps them develop stronger number sense and computational skills.
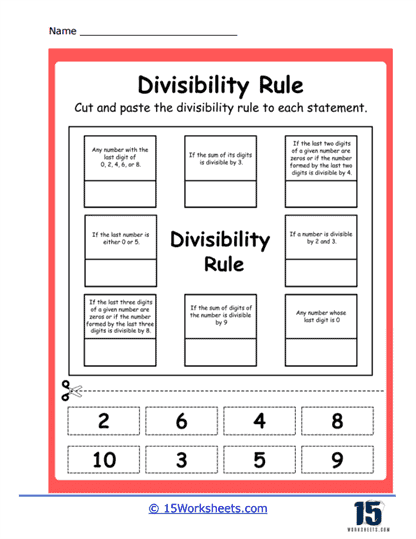
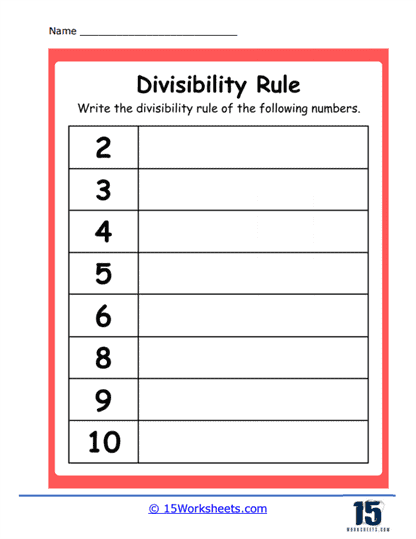
Divisibility rules are specific guidelines or criteria that help determine whether one number is evenly divisible by another number without the need for performing long division or using a calculator. These rules are based on the properties of numbers and can be helpful for quickly identifying factors and divisors of numbers.
How Does This Improve Your Math Skills?
Speed – Instead of doing long divisions for every number, you can quickly determine divisibility. This saves time! As you use these rules, you become more familiar with numbers and how they behave, which helps in other areas of math too.
Building Confidence – Sometimes, the problems on these worksheets can be like puzzles. They can challenge you to think and make decisions based on the rules. As you get better at using these rules, you’ll feel more confident about tackling bigger math challenges.
Where Can You Use These Skills in the Real World?
Shopping – Imagine you’re buying candy for a party and you want to divide them equally among your friends. By using divisibility rules, you can quickly figure out how to divide things without leaving anyone out!
Cooking – If you’re trying to split a recipe in half or double it, knowing divisibility can help make sure you’re using the right amounts.
Planning Events – If you’re organizing seats at a school event or deciding how many items to put in goodie bags, divisibility rules can help.
Everyday Decisions – From splitting chores at home to dividing game points, these rules are super handy!
Whether you’re shopping, cooking, or just playing games with friends, the skills you learn from these worksheets will come in handy in real life. So the next time you see a big number and wonder if it can be split evenly, remember the magic of divisibility rules!
What Are the Divisibility Rules?
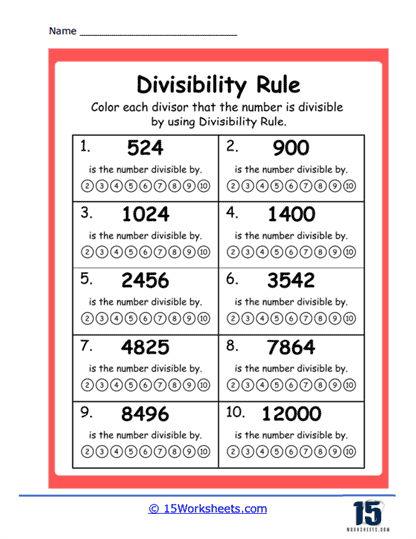
The rules of divisibility are fundamental principles in mathematics that determine whether one number can be evenly divided by another. These rules are essential for simplifying calculations, factoring numbers, and understanding the properties of various integer sets. In this explanation, we will explore the rules of divisibility in great detail, covering the rules for divisibility by integers 2 through 11.
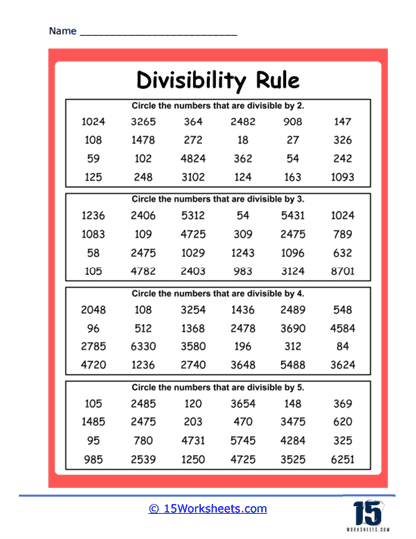
Divisibility by 2
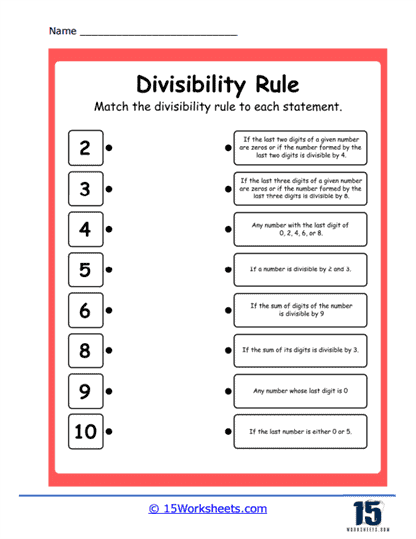
To check whether a number is divisible by 2, examine its last digit. If the last digit is even (0, 2, 4, 6, or 8), the number is divisible by 2. If the last digit is odd (1, 3, 5, 7, or 9), the number is not divisible by 2.
Example:
246 is divisible by 2 (even last digit).
357 is not divisible by 2 (odd last digit).
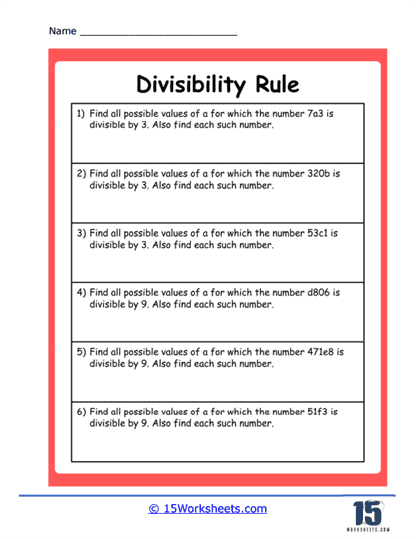
Divisibility by 3
To determine if a number is divisible by 3, add up its digits. If the sum is divisible by 3, then the original number is also divisible by 3.
Example:
132: 1 + 3 + 2 = 6, which is divisible by 3. So, 132 is divisible by 3.
457: 4 + 5 + 7 = 16, which is not divisible by 3. So, 457 is not divisible by 3.
Divisibility by 4
A number is divisible by 4 if its last two digits form a number divisible by 4. In other words, if the last two digits are a multiple of 4, the entire number is divisible by 4.
Example:
284 is divisible by 4 (84 is divisible by 4).
739 is not divisible by 4 (39 is not divisible by 4).
Divisibility by 5
A number is divisible by 5 if its last digit is either 0 or 5. If the last digit is any other digit, the number is not divisible by 5.
Example:
420 is divisible by 5 (ends with 0).
617 is not divisible by 5 (does not end with 0 or 5).
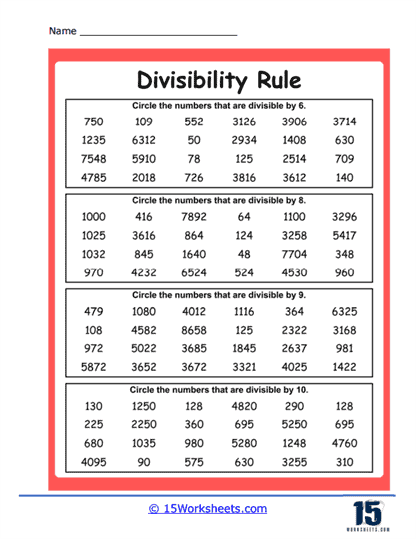
Divisibility by 6
A number is divisible by 6 if it is divisible by both 2 and 3. In other words, it must have an even last digit and have the sum of its digits divisible by 3.
Example:
126 is divisible by 6 (even last digit and the sum of digits is 1 + 2 + 6 = 9, which is divisible by 3).
371 is not divisible by 6 (odd last digit).
Divisibility by 7
Determining divisibility by 7 involves a more complex process. You can use a rule called the “divisibility by 7 rule,” which states that if you double the last digit and subtract it from the rest of the number without the last digit, the result should be divisible by 7 or equal to 0.
Example:
For 658, double the last digit (8 * 2 = 16) and subtract it from the rest of the number (65 – 16 = 49), which is divisible by 7. So, 658 is divisible by 7.
Divisibility by 8
A number is divisible by 8 if its last three digits form a number divisible by 8. In other words, if the last three digits are a multiple of 8, the entire number is divisible by 8.
Example:
2,496 is divisible by 8 (496 is divisible by 8).
723 is not divisible by 8.
Divisibility by 9
Similar to the rule for divisibility by 3, you can determine divisibility by 9 by adding up the digits. If the sum is divisible by 9, then the original number is also divisible by 9.
Example:
891: 8 + 9 + 1 = 18, which is divisible by 9. So, 891 is divisible by 9.
625: 6 + 2 + 5 = 13, which is not divisible by 9. So, 625 is not divisible by 9.
Divisibility by 10
A number is divisible by 10 if it ends with a 0. In other words, the last digit must be 0.
Example:
450 is divisible by 10 (ends with 0).
723 is not divisible by 10 (does not end with 0).
Divisibility by 11
To determine divisibility by 11, you can use a specific rule. Take the alternating sum of the digits (starting with the leftmost digit as positive) and check if the result is divisible by 11. If the alternating sum is divisible by 11 or equal to 0, then the original number is divisible by 11.
Example:
For 1,452: 1 – 4 + 5 – 2 = 0, which is divisible by 11. So, 1,452 is divisible by 11.
For 2,937: 2 – 9 + 3 – 7 = -11, which is divisible by 11. So, 2,937 is divisible by 11.
These rules of divisibility are powerful tools in number theory and arithmetic. They help simplify calculations, identify prime numbers, and find factors of numbers. By mastering these rules, you can quickly determine whether one number can be divided by another without the need for lengthy division calculations. These rules are essential for building a strong foundation in mathematics and are widely used in various mathematical disciplines and real-world applications.