Non-terminating Numbers

Worksheet Description

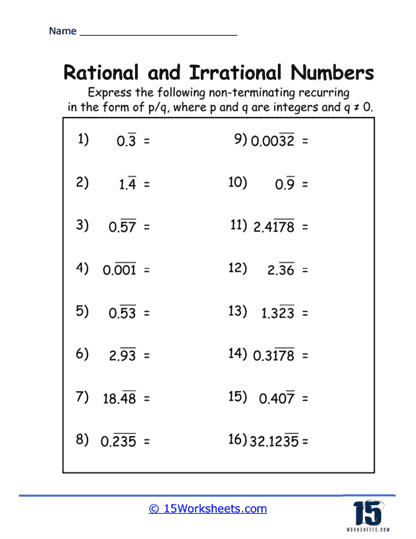
This worksheet is designed to practice converting non-terminating recurring decimals into their equivalent fractional form, expressed as
p/q, where p and q are integers and q is not zero. The numbers given include both simple recurring decimals and more complex ones, involving single and multiple repeating patterns. Students must apply the mathematical process of converting repeating decimals to fractions, which involves algebraic techniques like setting up equations to represent the decimals. The worksheet covers a fundamental aspect of rational numbers, emphasizing that even non-terminating decimals can have rational equivalents.

The worksheet teaches students how to express non-terminating recurring decimals as fractions, which is an essential skill in understanding the nature of rational numbers. It demonstrates the fact that all repeating decimals are rational numbers because they can be represented as the ratio of two integers. Through these exercises, students learn the method of writing a repeating decimal as an infinite geometric series and then using algebra to solve for a finite fractional representation. This not only enhances their number theory knowledge but also strengthens their algebraic manipulation skills, as they must work with equations and variables to find the fractional form.